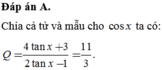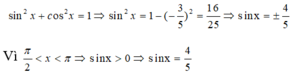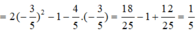Tính giá trị biểu thức A biết \(cosx=0,5;A=\dfrac{cosx+2sin^2x}{cos^2x-sinx}\)

Những câu hỏi liên quan
Biết \(sinx+cosx=m\).
Tính giá trị biểu thức sau theo m: \(sin^3x+cos^3x\)
sin x+cosx=m
=>(sinx+cosx)^2=m^2
=>1+2*cosx*sinx=m^2
=>2*sinx*cosx=m^2-1
=>\(sinx\cdot cosx=\dfrac{m^2-1}{2}\)
\(sin^3x+cos^3x=\left(sinx+cosx\right)^3-3\cdot sinx\cdot cosx\cdot\left(sinx+cosx\right)\)\(=m^3-3\cdot\dfrac{m^2-1}{2}\cdot m\)
\(=m^3-\dfrac{3m^3-3m}{2}\)
\(=\dfrac{2m^3-3m^3+3m}{2}=\dfrac{-m^3+3m}{2}\)
Đúng 0
Bình luận (0)
tính giá trị biểu thức P bằng sin2-sinx*cosx+2cos2x/2sin2x-cos2x biết tan x bằng 3
Lần sau bạn vào cái hình E để gửi câu hỏi nha!
\(P=\dfrac{sin^2\alpha-sin\alpha\cdot cos\alpha+2cos^2\alpha}{2sin^2\alpha-cos^2\alpha}\)
\(P=\dfrac{tan^2\alpha-tan\alpha+2}{2tan^2\alpha-1}\) (Chia cả tử và mẫu cho \(cos^2\alpha\))
\(P=\dfrac{3^2-3+2}{2\cdot3^2-1}=\dfrac{8}{17}\)
Chúc bn học tốt!
Đúng 1
Bình luận (0)
Cho biết cotx 1/2. Giá trị biểu thức A
2
sin
2
x
-
sin
x
.
cos
x
-
cos
2
x
bằng A. 6. B. 8. C. 10. D. 12.
Đọc tiếp
Cho biết cotx = 1/2. Giá trị biểu thức A= 2 sin 2 x - sin x . cos x - cos 2 x bằng
A. 6.
B. 8.
C. 10.
D. 12.
Chọn C.
Chia cả tử và mẫu cho sin2x ta được
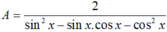

Đúng 0
Bình luận (0)
Cho tan²x+cot²x=2. Tính giá trị của biểu thức P=1/cosx–cosx/1+sin x
Xem chi tiết
\(tan^2x+cot^2x=2=2.tanx.cotx\)
\(\Leftrightarrow tan^2x+cot^2x-2tanx.cotx=0\)
\(\Leftrightarrow\left(tanx-cotx\right)^2=0\Leftrightarrow tanx=cotx=\dfrac{1}{tanx}\)
\(\Leftrightarrow tanx=\pm1\)
\(P=\dfrac{1}{cosx}-\dfrac{cosx}{1+sinx}=\dfrac{1+sinx-cos^2x}{cosx\left(1+sinx\right)}=\dfrac{sin^2x+sinx}{cosx\left(1+sinx\right)}\)
\(=\dfrac{sinx\left(1+sinx\right)}{cosx\left(1+sinx\right)}=tanx=\pm1\)
Đúng 1
Bình luận (0)
Cho \(sinx+cosx=m\) Tính theo m giá trị biểu thức
\(a,A=sinx.cosx\\ b,B=\left|sinx-cosx\right|\\ c,C=sin^4x+cos^4x\\ d,D=tan^2x+cot^2x\)
a: A=(sinx+cosx)^2-1=m^2-1
b: B=căn (sinx+cosx)^2-4sinxcosx=căn m^2-4(m^2-1)=căn -3m^2+4
c: C=(sin^2x+cos^2x)^2-2(sinx*cosx)^2=1-2m^2
Đúng 1
Bình luận (1)
D) tan2x + cot2x
= (1 - 2)(-sin2x/2 + 1/2)2):(-sin2x/2 + 1/2)2
= (1 - 2sin2x)/sin2x.cos2x
= (m2 - 3)/2
Đúng 1
Bình luận (0)
Giá trị của biểu thức
Q
4
sin
x
+
3
cos
x
2
sin
x
−
cos
x
biết tanx2 là A.
11
3
B. 2 C.
10
3
D. 4
Đọc tiếp
Giá trị của biểu thức Q = 4 sin x + 3 cos x 2 sin x − cos x biết tanx=2 là
A. 11 3
B. 2
C. 10 3
D. 4
Cho cos x = 3 5 , ( π 2 < x < π )
Tính giá trị biểu thức sau:
P = cos 2 x - 1 2 sin 2 x
1. Cho biết \(cosx=\dfrac{3}{4}\). Tính giá trị của biểu thức \(P=sin^22x\).
2. Giải phương trình \(cos2x-sin\left(x+\dfrac{\pi}{3}\right)=0\)
1: \(P=sin^22x=1-cos^22x\)
\(=1-\left(cos2x\right)^2\)
\(=1-\left(2cos^2x-1\right)^2\)
\(=1-\left(2\cdot\dfrac{9}{16}-1\right)^2\)
\(=1-\left(\dfrac{9}{8}-1\right)^2=1-\left(\dfrac{1}{8}\right)^2=\dfrac{63}{64}\)
2:
\(cos2x-sin\left(x+\dfrac{\Omega}{3}\right)=0\)
=>\(sin\left(x+\dfrac{\Omega}{3}\right)=cos2x=sin\left(\dfrac{\Omega}{2}-2x\right)\)
=>\(\left[{}\begin{matrix}x+\dfrac{\Omega}{3}=\dfrac{\Omega}{2}-2x+k2\Omega\\x+\dfrac{\Omega}{3}=\Omega-\dfrac{\Omega}{2}+2x+k2\Omega\end{matrix}\right.\)
=>\(\left[{}\begin{matrix}3x=\dfrac{\Omega}{6}+k2\Omega\\-x=\dfrac{1}{6}\Omega+k2\Omega\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{\Omega}{18}+\dfrac{k2\Omega}{3}\\x=-\dfrac{1}{6}\Omega-k2\Omega\end{matrix}\right.\)
Đúng 0
Bình luận (0)
Cho góc x, với cosx = 1/3. Tính giá trị của biểu thức: P = 3sin2x + cos2x.
Ta có : sin2 x + cos2 x = 1 ⇒ sin2 x = 1 – cos2 x.
⇒ P = 3.sin2 x + cos2 x
= 3.(1 – cos2x) + cos2 x
= 3 – 3.cos2x + cos2x
= 3 – 2.cos2x
= 3 – 2.(1/3)2
= 3 – 2/9
= 25/9.
Đúng 1
Bình luận (0)