P= cos2x. cot2x + 3cos2x - cot2x +2sin2x có giá trị là:
A.2 B. -2 C.3 D.-3
Những câu hỏi liên quan
Rút gọn biểu thức A = cos2x.cot2x + 3cos2x - cot2x + 2sin2x không phụ thuộc vào x và bằng
A. 2.
B. -2.
C. 1.
D. -1.
Chọn A.
Ta có: A = cos2x.cot2x + 3cos2x - cot2x + 2sin2x
=( cos2x.cot2x - cot2x) + (2sin2x + 2cos2x) + cos2x
= cot2x( cos2x - 1) + 2 + cos2x
= - cot2x. sin2x + 2 + cos2x
= -cos2x + 2 + cos2x = 2
Đúng 0
Bình luận (0)
giải phương trình:
a, \(tanx.sin^2x-2sin^2x=3\left(cos2x+sinxcosx\right)\)
b, \(5sinx-2=3\left(1-sinx\right)tan^2x\)
c,\(\frac{cos2x+3cot2x+4sinx}{cot2x-cos2x}=2\)
d, \(\frac{4sin^2x+6sin^2x-3cos2x-9}{cosx}=0\)
chứng minh
a> cot2x/1+cot2x . 1+tan2x/tan2x = tan2x+cot2x/1=tan4x
b>tan2x-cos2x/sin2x + cot2x-sin2x/cos2x = 2
a: \(VT=\dfrac{cot^2x}{1+cot^2x}\cdot\dfrac{1+tan^2x}{tan^2x}\)
\(=\dfrac{cot^2x}{\dfrac{1}{sin^2x}}\cdot\dfrac{\dfrac{1}{cos^2x}}{tan^2x}\)
\(=\dfrac{cot^2x}{tan^2x}\cdot\dfrac{1}{cos^2x}:\dfrac{1}{sin^2x}\)
\(=\dfrac{cot^2x}{tan^2x}\cdot\dfrac{sin^2x}{cos^2x}\)
\(=cot^2x\)
\(VP=\dfrac{tan^2x+cot^2x}{1+tan^4x}=\dfrac{\dfrac{sin^2x}{cos^2x}+\dfrac{cos^2x}{sin^2x}}{1+\dfrac{sin^4x}{cos^4x}}\)
\(=\dfrac{sin^4x+cos^4x}{sin^2x\cdot cos^2x}:\dfrac{cos^4x+sin^4x}{cos^4x}\)
\(=\dfrac{sin^4x+cos^4x}{sin^2x\cdot cos^2x}\cdot\dfrac{cos^4x}{cos^4x+sin^4x}=\dfrac{cos^2x}{sin^2x}=cot^2x\)
=>VT=VP
b:
\(\dfrac{tan^2x-cos^2x}{sin^2x}+\dfrac{cot^2x-sin^2x}{cos^2x}\)
\(=\dfrac{\left(\dfrac{sinx}{cosx}\right)^2-cos^2x}{sin^2x}+\dfrac{\left(\dfrac{cosx}{sinx}\right)^2-sin^2x}{cos^2x}\)
\(=\dfrac{sin^2x-cos^4x}{cos^2x\cdot sin^2x}+\dfrac{cos^2x-sin^4x}{sin^2x\cdot cos^2x}\)
\(=\dfrac{sin^2x+cos^2x-cos^4x-sin^4x}{cos^2x\cdot sin^2x}\)
\(=\dfrac{1-\left(cos^2x+sin^2x\right)^2+2\cdot cos^2x\cdot sin^2x}{cos^2x\cdot sin^2x}\)
\(=\dfrac{2\cdot cos^2x\cdot sin^2x}{cos^2x\cdot sin^2x}=2\)
Đúng 1
Bình luận (0)
Tập nghiệm của phương trình
c
o
t
2
x
+
2
sin
2
x
1
/
sin
2
x
là: A.
±
π
6
+
k
π
,
kπ
;
k
∈...
Đọc tiếp
Tập nghiệm của phương trình c o t 2 x + 2 sin 2 x = 1 / sin 2 x là:
A. ± π 6 + k π , kπ ; k ∈ ℤ
B. ± π 3 + k π ; k ∈ ℤ
C. ± π 3 + k π , kπ ; k ∈ ℤ
D. ± π 6 + k π ; k ∈ ℤ
Chọn B
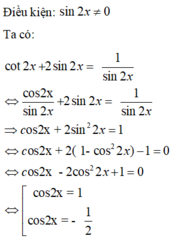
* Với cos 2x = 1 thì sin2x =0 ( không thỏa mãn điều kiện)
Đúng 0
Bình luận (0)
Biểu thức B
sin
4
x
+
cos
4
x
-
1
tan
2
x
+
c
o
t
2
x
+...
Đọc tiếp
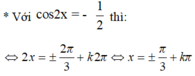
Biểu thức B= sin 4 x + cos 4 x - 1 tan 2 x + c o t 2 x + 2 có giá trị không đổi bằng:
A. 2
B. 1
C. -2
D. -1
Biểu thức
B
sin
4
x
+
cos
4
x
−
1
tan
2
x
+
cot...
Đọc tiếp
Biểu thức B = sin 4 x + cos 4 x − 1 tan 2 x + cot 2 x + 2 có giá trị không đổi bằng:
A. 2
B. 1
C. -2
D. -1
Câu 1 ) tìm TXD của các hàm số sau :
a) y = 2014/tan(1-x)
b) y = cotx/sinx + cos2x
c) y = cot2x/sinx + 2
a/ \(\left\{{}\begin{matrix}sin\left(1-x\right)\ne0\\cos\left(1-x\right)\ne0\end{matrix}\right.\) \(\Leftrightarrow sin\left(1-x\right).cos\left(1-x\right)\ne0\)
\(\Leftrightarrow sin\left(2-2x\right)\ne0\)
\(\Leftrightarrow2-2x\ne k\pi\Leftrightarrow x\ne1+\frac{k\pi}{2}\)
b và c bạn ghi như vầy ko biết mẫu số nằm ở đâu để tìm điều kiện
Đúng 0
Bình luận (0)
B tan2 x . (cot2x + cos2x + sin2x – 1 ) + 10 B sin2 230 + + sin2670 – cos600
Đọc tiếp
B = tan2 x . (cot2x + cos2x + sin2x – 1 ) + 10
B = sin2 230 + 
+ sin2670 – cos600
Tính giá trị của biểu thức:
a) tan10o . tan11o ... tan79o . tan80o
b) Cho tan x+ cot x=2. tính tan2x + cot2x ; tan3x + cot3x
\(a,\tan10.\tan11......\)
\(=\left(\tan10.tan80\right)\left(tan11.tan79\right)....\left(tan44.tan46\right).tan45\)
Mà 10 và 80, 11 và 79, ... là các góc phụ nhau .
\(=tan10.cot10....tan45=1\)
b, Ta có : \(\tan x+\cot x=2\)
\(\Rightarrow\tan^2x+2\tan x\cot x+\cot^2x=4\)
\(\Rightarrow\tan^2x+\cot^2x=4-2=2\)
Ta có : \(\tan^3x+\cot^3x=\left(\tan x+\cot x\right)\left(\tan^2x-\tan x\cot x+\cot^2x\right)=2\)
Đúng 0
Bình luận (0)
25 tháng 8 2020 lúc 19:12
giải pt
a) \(sin^2x+2sin^22x+sin^23x-2=0\)
b) \(2cosx.cos\left(x+\frac{\pi}{3}\right)+\sqrt{3}sin2x=1\)
c) \(5\left(1+cosx\right)=2+sin^4x-cos^4x\)
d) \(1+cot2x=\frac{1-cos2x}{sin^22x}\)
a/
\(\Leftrightarrow\frac{1}{2}-\frac{1}{2}cos2x+\frac{1}{2}-\frac{1}{2}cos6x-2\left(1-sin^22x\right)=0\)
\(\Leftrightarrow1-\frac{1}{2}\left(cos6x+cos2x\right)-2cos^22x=0\)
\(\Leftrightarrow1-cos4x.cos2x-2cos^22x=0\)
\(\Leftrightarrow2cos^22x-1+cos4x.cos2x=0\)
\(\Leftrightarrow cos4x+cos4x.cos2x=0\)
\(\Leftrightarrow cos4x\left(cos2x+1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}cos4x=0\\cos2x=-1\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}4x=\frac{\pi}{2}+k\pi\\2x=\pi+k2\pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\frac{\pi}{8}+\frac{k\pi}{4}\\x=\frac{\pi}{2}+k\pi\end{matrix}\right.\)
Đúng 0
Bình luận (0)
b/
\(\Leftrightarrow cos\left(2x+\frac{\pi}{3}\right)+cos\left(\frac{\pi}{3}\right)+\sqrt{3}sin2x=1\)
\(\Leftrightarrow cos2x.cos\left(\frac{\pi}{3}\right)-sin2x.sin\left(\frac{\pi}{3}\right)+\frac{1}{2}+\sqrt{3}sin2x=1\)
\(\Leftrightarrow\frac{1}{2}cos2x+\frac{\sqrt{3}}{2}sin2x=\frac{1}{2}\)
\(\Leftrightarrow cos\left(2x-\frac{\pi}{3}\right)=\frac{1}{2}\)
\(\Leftrightarrow\left[{}\begin{matrix}2x-\frac{\pi}{3}=\frac{\pi}{3}+k2\pi\\2x-\frac{\pi}{3}=-\frac{\pi}{3}+k2\pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\frac{\pi}{3}+k\pi\\x=k\pi\end{matrix}\right.\)
Đúng 0
Bình luận (0)
c/
\(\Leftrightarrow5+5cosx=2+\left(sin^2x-cos^2x\right)\left(sin^2x+cos^2x\right)\)
\(\Leftrightarrow3+5cosx=sin^2x-cos^2x\)
\(\Leftrightarrow3+5cosx=1-cos^2x-cos^2x\)
\(\Leftrightarrow2cos^2x+5cosx+2=0\)
\(\Leftrightarrow\left[{}\begin{matrix}cosx=-2\left(l\right)\\cosx=-\frac{1}{2}\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\frac{2\pi}{3}+k2\pi\\x=-\frac{2\pi}{3}+k2\pi\end{matrix}\right.\)
Đúng 0
Bình luận (0)
Xem thêm câu trả lời