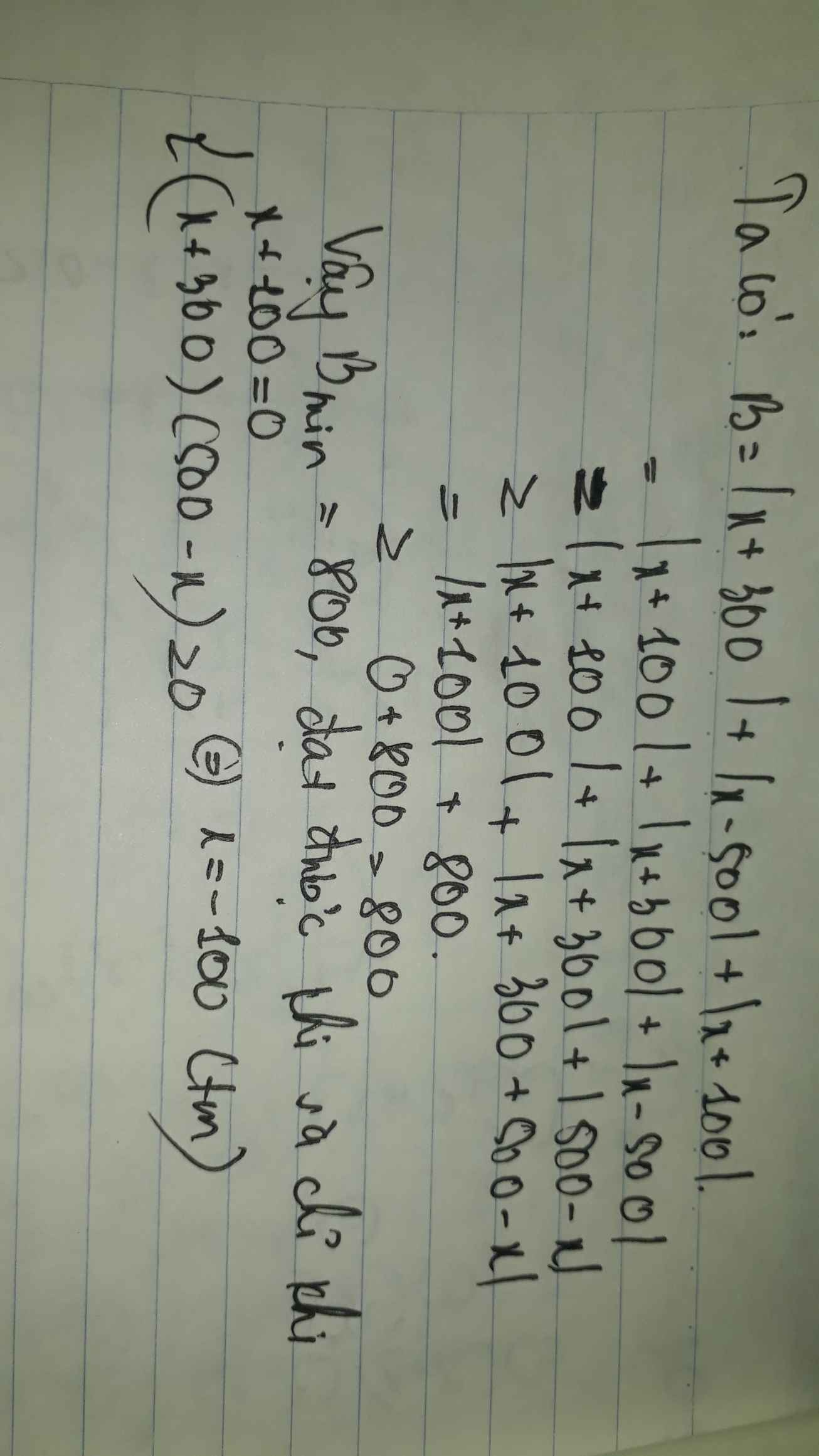tính GTNN của \(A=\left|x\right|+\left|x+8\right|\)

Những câu hỏi liên quan
Tìm gtnn của biểu thức
\(A=\left|x+5\right|+\left|x+2\right|+\left|x-7\right|+\left|x-8\right|\)
Ta có tính chất :
\(\left|a\right|+\left|b\right|\ge\left|a+b\right|\)
\(\rightarrow A=\left|x+5\right|+\left|x+2\right|+\left|x-7\right|+\left|x-8\right|\ge\left|x+5+x+2+x-7+x-8\right|\)
\(\rightarrow A\ge\left|4x-8\right|\)
Vì \(\left|4x-8\right|\ge0\forall x\in R\) nên :
\(\rightarrow A\ge0\forall x\in R\)
Dấu "= " xảy ra khi :
\(\left|4x-8\right|=0\) \(\Leftrightarrow4x-8=0\)
\(\Leftrightarrow x=2\)
Vậy \(A_{min}=0\Leftrightarrow x=2\)
Đúng 1
Bình luận (0)
Bài 1 : Tìm GTNN của : \(A=\left|x+8\right|+\left|2x+7\right|+\left|3x+6\right|+\left|4x-7\right|+\left|3x-6\right|+\left|2x-7\right|+\left|x-8\right|-100\)
Tìm GTNN của A=\(\left|x+8\right|+\left|x+13\right|+\left|x+50\right|\)
\(\left|x+8\right|+\left|x+13\right|=\left|x+8\right|+\left|-x-13\right|\)
Áp dụng bđt \(\left|a\right|+\left|b\right|\ge\left|a+b\right|\) ta có :
\(\left|x+8\right|+\left|-x-13\right|\ge\left|x+8-x-13\right|=\left|-5\right|=5\)
\(\Rightarrow A\ge\left|x+50\right|+5\ge5\)
Dấu "=" xảy ra <=> |x + 50| = 0 => x = - 50
Vậy gtnn của A là 5 tại x = - 50
Đúng 0
Bình luận (0)
Tìm GTNN của biểu thức: \(A=\frac{\left(x^2+18\left|x\right|+32\right)\left(x^2+9\left|x\right|+8\right)}{x^2}\)
Với \(x\ne0\), đặt \(\left|x\right|=a>0\)
\(A=\frac{\left(a^2+18a+32\right)\left(a^2+9a+8\right)}{a^2}=\frac{\left(a+2\right)\left(a+16\right)\left(a+1\right)\left(a+8\right)}{a^2}\)
\(A=\frac{\left(a+2\right)\left(a+8\right)\left(a+1\right)\left(a+16\right)}{a^2}=\frac{\left(a^2+10a+16\right)\left(a^2+17a+16\right)}{a^2}\)
\(A=\frac{\left(a^2+16+10a\right)}{a}.\frac{\left(a^2+16+17a\right)}{a}=\left(a+\frac{16}{a}+10\right)\left(a+\frac{16}{a}+17\right)\)
\(\Rightarrow A\ge\left(2\sqrt{a.\frac{16}{a}}+10\right)\left(2\sqrt{a.\frac{16}{a}}+17\right)=\left(8+10\right)\left(8+17\right)=450\)
\(\Rightarrow A_{min}=450\) khi \(a^2=16\Rightarrow a=4\Rightarrow x=\pm4\)
Đúng 0
Bình luận (0)
Bài 1 : Tìm GTNN của : \(A=\left|x+8\right|+\left|2x+7\right|+\left|3x+6\right|+\left|4x-7\right|+\left|3x-6\right|+\left|2x-7\right|+\left|x-8\right|-100\)
Tính GTNN của B=\(\left|x+300\right|+\left|x-500\right|+\left|x+100\right|\)
Timg GTNN của các bt:
a. \(A=\left|x-2000\right|+\left|x-2001\right|+\left|x-2002\right|\)
b. \(\sqrt{\left(x-8\right)^2}+\sqrt{\left(x-9\right)^2}+\sqrt{\left(x-10\right)^2}+\sqrt{\left(x+11\right)^2}\)
a) \(\left|x-2000\right|+\left|x-2002\right|=\left|x-2000\right|+\left|2002-x\right|\)
\(\ge\left|x-2000+2002-x\right|=2\) (1)
Dấu "=" \(\Leftrightarrow\left(x-2000\right)\left(2002-x\right)\ge0\)
\(\Leftrightarrow2000\le x\le2002\)
+ \(\left|x-2001\right|\ge0\forall x\). "=" \(\Leftrightarrow x=2001\) (2)
Từ (1) và (2) suy ra \(A\ge2\)
Dấu "=" \(\Leftrightarrow x=2001\)
b) \(B=\left|x-8\right|+\left|x-9\right|+\left|x-10\right|+\left|x+11\right|\)
+ \(\left|x-10\right|+\left|x+11\right|=\left|x+11\right|+\left|10-x\right|\)
\(\ge\left|x+11+10-x\right|=21\) (3)
Dấu "=" \(\Leftrightarrow\left(x+11\right)\left(10-x\right)\ge0\Leftrightarrow-11\le x\le10\)
+ \(\left|x-8\right|+\left|x-9\right|\ge\left|x-8+9-x\right|=1\) (4)
"=" \(\Leftrightarrow\left(x-8\right)\left(9-x\right)\ge0\Leftrightarrow8\le x\le9\)
Từ (3) và (4) suy ra \(B\ge22\)
"=" \(\Leftrightarrow8\le x\le9\)
Đúng 0
Bình luận (0)
Tìm GTNN của biểu thức:
a) \(\left|x+5\right|+\left|x+17\right|\)
b) \(\left|x+8\right|+\left|x+13\right|+\left|x+50\right|\)
c) \(\left|x+5\right|+\left|x+2\right|+\left|x-7\right|+\left|x-8\right|\)
d) \(\left|x+3\right|+\left|x-2\right|+\left|x-5\right|\)
Tìm GTNN của biểu thức:
a) A = |x+5|+|x+17|
Giải
Ta có : A = |x+5|+|x+17| \(\ge\) |x+5+x+17|
A = |-x-5|+|x+17| \(\ge\) |-x-5+x+17| = | -12 | = 12
Dấu bằng xảy ra khi - 17 \(\le\) x \(\le\) -5
Vậy MinA=12 khi - 17 \(\le\) x \(\le\) -5
b) B = |x+8|+|x+13|+|x+50|
Giải
B = |x+8|+|x+13|+|x+50| \(\ge\) (| x+8|+|-50-x |)+|x+13|
= (| x+8-50-x |)+|x+13|
= |-42| + |x+13|
= 42 + |x+13| \(\ge\) 42
Vậy MinB = 42 khi và chỉ khi:
\(\left\{{}\begin{matrix}x+8\ge0\\x+13=0\\x+50\ge0\end{matrix}\right.\) \(\Rightarrow\left\{{}\begin{matrix}x\ge-8\\x=-13\\x\ge-50\end{matrix}\right.\) \(\Rightarrow x=-13\)
c) C = |x+5|+|x+2|+|x−7|+|x−8|
Giải
C = |x+5|+|x+2|+|x−7|+|x−8|
\(\ge\) |x+5| + |x+2| + |7-x| + |8-x|
\(\ge\) |x+5+7-x| + |x+2+8-x|
\(\ge\) |12| + |10|
\(\ge\) 12 + 10 \(\ge\) 22
Vậy MinC = 22 khi và chỉ khi :
-5 \(\le\) x \(\le\) 8 và -2 \(\le\) x \(\le\) 7 \(\Leftrightarrow\) -2 \(\le\) x \(\le\) 7
d) D = |x+3|+|x−2|+|x−5|
Giải
D = |x+3|+|x−2|+|x−5|
\(\ge\) ( |x+3|+|5-x| ) + |x-2| \(\ge\) | x+3+5-x | + | x-2 | \(\ge\) | 8 | + | x-2 | \(\ge\) 8 + | x-2 | \(\ge\) 8 Vậy MinD = 8 khi và chỉ khi: \(\left\{{}\begin{matrix}x+3\ge0\\x-2=0\\5-x\ge0\end{matrix}\right.\) \(\Rightarrow\left\{{}\begin{matrix}x\ge-3\\x=2\\x\le5\end{matrix}\right.\) \(\Rightarrow x=2\)
Đúng 0
Bình luận (0)
Tìm GTNN của biểu thức:
a) A = |x+5|+|x+17|
Giải
Ta có : A = |x+5|+|x+17| ≥≥|x+5+x+17|
A = |-x-5|+|x+17| ≥ |-x-5+x+17| = | -12 | = 12
Dấu bằng xảy ra khi - 17 ≤ x ≤ -5
Vậy MinA=12 khi - 17 ≤ x ≤ -5
b) B = |x+8|+|x+13|+|x+50|
Giải
B = |x+8|+|x+13|+|x+50| ≥ (| x+8|+|-50-x |)+|x+13|
= (| x+8-50-x |)+|x+13|
= |-42| + |x+13|
= 42 + |x+13| ≥≥42
Vậy MinB = 42 khi và chỉ khi:
x+8 ≥ 0 ⇒x ≥ −8
x+13 = 0 => x = −13 .Vậy x=-13
x+50 ≥ 0 => x ≥ −50
c) C = |x+5|+|x+2|+|x−7|+|x−8|
Giải
C = |x+5|+|x+2|+|x−7|+|x−8|
=> |x+5| + |x+2| + |7-x| + |8-x|
≥ |x+5+7-x| + |x+2+8-x| = |12| + |10| =12 + 10 = 22
Vậy MinC = 22 khi và chỉ khi :
-5 ≤ x ≤ 8 và -2 ≤ x ≤ 7 ⇔ -2 ≤ x ≤ 7
Đúng 0
Bình luận (0)
Tìm GTNN của biểu thức:
a) A = |x+5|+|x+17|
Giải
Ta có : A = |x+5|+|x+17| ≥≥ |x+5+x+17|
A = |-x-5|+|x+17| ≥≥ |-x-5+x+17| = | -12 | = 12
Dấu bằng xảy ra khi - 17 ≤≤ x ≤≤ -5
Vậy MinA=12 khi - 17 ≤≤ x ≤≤ -5
b) B = |x+8|+|x+13|+|x+50|
Giải
B = |x+8|+|x+13|+|x+50| ≥≥ (| x+8|+|-50-x |)+|x+13|
= (| x+8-50-x |)+|x+13|
= |-42| + |x+13|
= 42 + |x+13| ≥≥ 42
Vậy MinB = 42 khi và chỉ khi:
⎧⎪⎨⎪⎩x+8≥0x+13=0x+50≥0{x+8≥0x+13=0x+50≥0 ⇒⎧⎪⎨⎪⎩x≥−8x=−13x≥−50⇒{x≥−8x=−13x≥−50 ⇒x=−13⇒x=−13
c) C = |x+5|+|x+2|+|x−7|+|x−8|
Giải
C = |x+5|+|x+2|+|x−7|+|x−8|
\(\ge\) |x+5| + |x+2| + |7-x| + |8-x|
≥≥ |x+5+7-x| + |x+2+8-x|
≥≥ |12| + |10|
≥≥ 12 + 10 ≥≥ 22
Vậy MinC = 22 khi và chỉ khi :
-5 ≤≤ x ≤≤ 8 và -2 x ≤≤ 7 ⇔⇔ -2 ≤≤ x ≤≤ 7
d) D = |x+3|+|x−2|+|x−5|
Giải
D = |x+3|+|x−2|+|x−5|
≥≥ ( |x+3|+|5-x| ) + |x-2| ≥≥ | x+3+5-x | + | x-2 | ≥≥ | 8 | + | x-2 | ≥≥ 8 + | x-2 | ≥≥ 8 Vậy MinD = 8 khi và chỉ khi: ⎧⎪⎨⎪⎩x+3≥0x−2=05−x≥0{x+3≥0x−2=05−x≥0 ⇒⎧⎪⎨⎪⎩x≥−3x=2x≤5⇒{x≥−3x=2x≤5 ⇒x=2
Đúng 0
Bình luận (0)
Tìm GTNN của biểu thức sau :
A=\(\left|x+8\right|+\left|x+13\right|+\left|x+50\right|+2018\)
Với mọi x ta có :
\(\left|x+50\right|=\left|-x-50\right|\)
\(\Leftrightarrow\left|x+8\right|+\left|x+50\right|=\left|x+8\right|+\left|-50-x\right|\)
\(\Leftrightarrow\left|x+8\right|+\left|-x-50\right|\ge\left|\left(x+8\right)+\left(-x-50\right)\right|\)
\(\Leftrightarrow\left|x+8\right|+\left|-x-50\right|\ge42\)
Mà \(\left|x+13\right|\ge0\)
\(\Leftrightarrow\left|x+8\right|+\left|-x-50\right|+\left|x+13\right|+2018\ge2060\)
\(\Leftrightarrow A\ge2060\)
Dấu "=" xảy ra khi :
\(\left\{{}\begin{matrix}\left(x+8\right)\left(-x-50\right)\ge0\left(1\right)\\\left|x+13\right|=0\left(2\right)\end{matrix}\right.\)
Từ \(\left(1\right)\Leftrightarrow\left[{}\begin{matrix}\left\{{}\begin{matrix}x+8\ge0\\-x-50\ge0\end{matrix}\right.\\\left\{{}\begin{matrix}x+8\le0\\-x-50\le0\end{matrix}\right.\end{matrix}\right.\) \(\Leftrightarrow\left[{}\begin{matrix}\left\{{}\begin{matrix}x\ge-8\\-50\ge x\end{matrix}\right.\\\left\{{}\begin{matrix}x\le-8\\-50\le x\end{matrix}\right.\end{matrix}\right.\) \(\Leftrightarrow\left[{}\begin{matrix}x\in\varnothing\\-50\le x\le-8\end{matrix}\right.\)
\(\Leftrightarrow-50\le x\le-8\left(I\right)\)
Từ \(\left(2\right)\Leftrightarrow x+13=0\)
\(\Leftrightarrow x=-13\left(II\right)\)
Từ \(\left(I\right)+\left(II\right)\Leftrightarrow A_{Min}=2060\Leftrightarrow x=-13\)
Đúng 0
Bình luận (0)