Giải hệ phương trình:
{ x .(x + 3y) = 4 ; 4y2 = 5 - xy}
Giải hệ phương trình sau: x+3y=4 3x-2y=1
\(\left\{{}\begin{matrix}x+3y=4\\3x-2y=1\end{matrix}\right.\)
<=> \(\left\{{}\begin{matrix}3x+9y=12\\3x-2y=1\end{matrix}\right.\)
\(\Leftrightarrow11y=11\)
=> y = 1 => \(x=4-3.1=1\)
Giải hệ phương trình :
x+2y=5 và 2x-3y=-4
Có: \(\left\{{}\begin{matrix}x+2y=5\\2x-3y=-4\end{matrix}\right.\)\(\Leftrightarrow\left\{{}\begin{matrix}2x+4y=10\left(1\right)\\2x-3y=-4\left(2\right)\end{matrix}\right.\)
Lấy (1) trừ (2) vế theo vế, ta có: 7y=14 hay y=2 \(\Rightarrow x=1\)
Vậy nghiệm của hệ phương trình là (1;2)
\(\left\{{}\begin{matrix}x+2y=5\\2x-3y=-4\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}3x+6y=15\\4x-6y=-8\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}7x=7\\x+2y=5\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x=1\\1+2y=5\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}x=1\\y=2\end{matrix}\right.\)
`{(x + 2y = 5),(2x - 3y = -4):}`
`<=>{(2x + 4y = 10),(2x - 3y = -4):}`
`<=>{(7y = 14),(x + 2y = 5):}`
`<=>{(y = 2),(x + 2 . 2 = 5):}`
`<=>{(x = 1),(y = 2):}`
Vậy hệ ptr có nghiệm `(x ; y) = ( 1; 2)`
Giải các hệ phương trình sau bằng phương pháp thế:
a ) x + y 5 = 0 x 5 + 3 y = 1 − 5 b ) ( 2 − 3 ) x − 3 y = 2 + 5 3 4 x + y = 4 − 2 3
Bài toán giải hệ phương trình bằng phương pháp thế có 2 cách trình bày.
Cách 1:
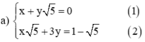
Từ (1) ta rút ra được x = -y√5 (*)
Thế (*) vào phương trình (2) ta được :
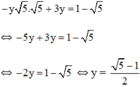
Thay y = 5 - 1 2 vào (*) ta được: x = − 5 − 1 2 ⋅ 5 = 5 − 5 2
Vậy hệ phương trình có nghiệm 5 − 5 2 ; 5 − 1 2
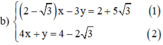
Từ (2) ta rút ra được y = -4x + 4 - 2 √3 (*)
Thế (*) vào phương trình (1) ta được:
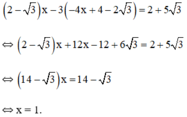
Thay x = 1 vào (*) ta được y = -4.1 + 4 - 2√3 = -2√3
Vậy hệ phương trình có nghiệm duy nhất (1; -2√3)
Cách 2 :
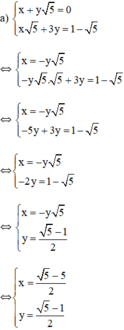
Vậy hệ phương trình có nghiệm duy nhất 5 − 5 2 ; 5 − 1 2
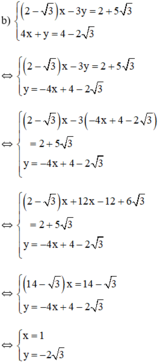
Vậy hệ phương trình có nghiệm duy nhất (1; -2√3)
Kiến thức áp dụng
Giải hệ phương trình 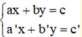 ta làm như sau:
ta làm như sau:
Bước 1: Từ một phương trình (coi là phương trình thứ nhất), ta biểu diễn x theo y (hoặc y theo x) ta được phương trình (*). Sau đó, ta thế (*) vào phương trình thứ hai để được một phương trình mới ( chỉ còn một ẩn).
Bước 2: Dùng phương trình mới ấy thay thế cho phương trình thứ hai, phương trình (*) thay thế cho phương trình thứ nhất của hệ ta được hệ phương trình mới tương đương .
Bước 3: Giải hệ phương trình mới ta tìm được nghiệm của hệ phương trình.
giải hệ phương trình:
(x-y)^2+5(x-y)=6 và 2x+3y=4
a) giải hệ phương trình {x+y=3 {2x-3y=1 b) giải phương trình x^ -7x +10=0
\(a,\left\{{}\begin{matrix}x+y=3\\2x-3y=1\end{matrix}\right.\\ \Leftrightarrow\left\{{}\begin{matrix}2x+2y=6\\2x-3y=1\end{matrix}\right.\\ \Leftrightarrow\left\{{}\begin{matrix}5y=5\\2x-3y=1\end{matrix}\right.\\ \Leftrightarrow\left\{{}\begin{matrix}y=1\\2x-3.1=1\end{matrix}\right.\\ \Leftrightarrow\left\{{}\begin{matrix}x=2\\y=1\end{matrix}\right.\)
Vậy \(\left(x;y\right)=\left(2;1\right)\)
b, \(x^2-7x+10=0\\ \Leftrightarrow x^2-5x-2x+10=0\\ \Leftrightarrow x\left(x-5\right)-2\left(x-5\right)=0\\ \Leftrightarrow\left(x-2\right)\left(x-5\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x-2=0\\x-5=0\end{matrix}\right.\\ \Leftrightarrow\left[{}\begin{matrix}x=2\\x=5\end{matrix}\right.\)
\(a,\)\(\left\{{}\begin{matrix}x+y=3\\2x-3y=1\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}3x+3y=9\\2x-3y=1\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}5x=10\\2x-3y=1\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x=2\\2.2-3y=1\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x=2\\y=1\end{matrix}\right.\)
Vậy hệ pt có nghiệm duy nhất \(\left(x;y\right)=\left(2;1\right)\)
\(b,x^2-7x+10=0\)
\(\Delta=b^2-4ac=\left(-7\right)^2-4.10=9>0\)
\(\Rightarrow\) Pt có 2 nghiệm \(x_1,x_2\)
Ta có :
\(\left\{{}\begin{matrix}x_1=\dfrac{-b+\sqrt{\Delta}}{2a}=\dfrac{7+3}{2}=5\\x_2=\dfrac{-b-\sqrt{\Delta}}{2a}=\dfrac{7-3}{2}=2\end{matrix}\right.\)
Vậy \(S=\left\{5;2\right\}\)
giải các hệ phương trình
9x-6y=4 và 3(4x-3y)=-3x+y+7
3(x+1)+2y=-x và 5(x+y)=-3x+y-5
2(2x+3y)=3(2x-3y)+10 và 4x-3y=4(6y-2x)+3
Bài 1: Giải hệ phương trình sau
\(\left\{{}\begin{matrix}\dfrac{1}{2x-y}+\left(x+3y\right)=\dfrac{3}{2}\\\dfrac{4}{2x-y}-5\left(x+3y\right)=-2\end{matrix}\right.\)
Bài 2: Cho phương trình: x\(^2\)+(m-1)x-m\(^2\)-2=0
a) CMR: phương trình luôn có 2 nghiệm phân biệt \(\forall\)m
b) Tìm m để biểu thức A=\(\left(\dfrac{x_1}{x_2}\right)^3+\left(\dfrac{x_2}{x_1}\right)^3\) đạt giá trị lớn nhất.
Bài 2:
a) Ta có: \(\Delta=\left(m-1\right)^2-4\cdot1\cdot\left(-m^2-2\right)\)
\(=m^2-2m+1+4m^2+8\)
\(=5m^2-2m+9>0\forall m\)
Do đó, phương trình luôn có hai nghiệm phân biệt với mọi m
Bài 1:
ĐKXĐ \(2x\ne y\)
Đặt \(\dfrac{1}{2x-y}=a;x+3y=b\)
HPT trở thành
\(\left\{{}\begin{matrix}a+b=\dfrac{3}{2}\\4a-5b=-2\end{matrix}\right.\\ \Leftrightarrow\left\{{}\begin{matrix}a=\dfrac{3}{2}-b\\4\left(\dfrac{3}{2}-b\right)-5b=-2\end{matrix}\right.\\ \Leftrightarrow\left\{{}\begin{matrix}a=\dfrac{3}{2}-b\\6-9b=-2\end{matrix}\right.\\ \Leftrightarrow\left\{{}\begin{matrix}b=\dfrac{8}{9}\\a=\dfrac{11}{18}\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x+3y=\dfrac{8}{9}\\2x-y=\dfrac{18}{11}\end{matrix}\right.\\ \Leftrightarrow\left\{{}\begin{matrix}y=2x-\dfrac{18}{11}\\x+3\left(2x-\dfrac{18}{11}\right)=\dfrac{8}{9}\end{matrix}\right.\\ \Leftrightarrow\left\{{}\begin{matrix}x=\dfrac{82}{99}\\y=\dfrac{2}{99}\end{matrix}\right.\)
Bài 1:Cho biểu thức P=√x + 1/√x - 2 + 2√x/√x +2 + 2+5√x /4-x
a)Rút gọn P
b)Tìm x để P=2
Bài 2:Cho hệ phương trình x+my=9 và mx-3y=4
a)Giải hệ phương trình với m=3
b)Tìm m để hệ phương trính có nghiệm x=-1,y=3
Bài 1:
a) Ta có: \(P=\dfrac{\sqrt{x}+1}{\sqrt{x}-2}+\dfrac{2\sqrt{x}}{\sqrt{x}+2}+\dfrac{2+5\sqrt{x}}{4-x}\)
\(=\dfrac{\left(\sqrt{x}+1\right)\left(\sqrt{x}+2\right)}{\left(\sqrt{x}-2\right)\cdot\left(\sqrt{x}+2\right)}+\dfrac{2\sqrt{x}\left(\sqrt{x}-2\right)}{\left(\sqrt{x}+2\right)\left(\sqrt{x}-2\right)}-\dfrac{2+5\sqrt{x}}{\left(\sqrt{x}+2\right)\left(\sqrt{x}-2\right)}\)
\(=\dfrac{x+3\sqrt{x}+2+2x-4\sqrt{x}-2-5\sqrt{x}}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+2\right)}\)
\(=\dfrac{3x-6\sqrt{x}}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+2\right)}\)
\(=\dfrac{3\sqrt{x}\left(\sqrt{x}-2\right)}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+2\right)}\)
\(=\dfrac{3\sqrt{x}}{\sqrt{x}+2}\)
b)
ĐKXĐ: \(\left\{{}\begin{matrix}x\ge0\\x\ne4\end{matrix}\right.\)
Để P=2 thì \(\dfrac{3\sqrt{x}}{\sqrt{x}+2}=2\)
\(\Leftrightarrow3\sqrt{x}=2\left(\sqrt{x}+2\right)\)
\(\Leftrightarrow3\sqrt{x}=2\sqrt{x}+4\)
\(\Leftrightarrow3\sqrt{x}-2\sqrt{x}=4\)
\(\Leftrightarrow\sqrt{x}=4\)
hay x=16(nhận)
Vậy: Để P=2 thì x=16
2.
a, \(m=3\), hệ phương trình trở thành:
\(\left\{{}\begin{matrix}x+3y=9\\3x-3y=4\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}4x=13\\y=\dfrac{3x-4}{3}\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x=\dfrac{13}{4}\\y=\dfrac{23}{12}\end{matrix}\right.\)
b, \(\left(x;y\right)=\left(-1;3\right)\) là nghiệm của hệ, suy ra:
\(\left\{{}\begin{matrix}-1+3m=9\\-m-9=4\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}m=\dfrac{10}{3}\\m=-13\end{matrix}\right.\)
\(\Rightarrow\) Không tồn tại giá trị m thỏa mãn
cho hệ phương trình: mx + 3y= - 4,
x- 2y=5
a/ giải hệ pt với m=2
b/ tìm m để hệ pt không có nghiệm
a: Khi m=2 thì hệ phương trình sẽ trở thành:
\(\left\{{}\begin{matrix}2x+3y=-4\\x-2y=5\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}2x+3y=-4\\2x-4y=10\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}7y=-14\\x-2y=5\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}y=-2\\x=2y+5=-4+5=1\end{matrix}\right.\)
b: Để hệ phương trình không có nghiệm thì \(\dfrac{m}{1}=\dfrac{3}{-2}< >-\dfrac{4}{5}\)
=>\(\dfrac{m}{1}=\dfrac{3}{-2}\)
=>\(m=\dfrac{3}{-2}=-\dfrac{3}{2}\)
giải hệ phương trình sau
\(\left\{{}\begin{matrix}2x-3y=4\\x+2y=-5\end{matrix}\right.\)
\(\left\{{}\begin{matrix}2x-3y=4\\x+2y=-5\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}2x-3y=4\\2x+4y=-10\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}-7y=14\\x+2y=-5\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}y=-2\\x+2.\left(-2\right)=-5\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}y=-2\\x=-1\end{matrix}\right.\)
Vậy nghiệm của hệ phương trình là \(\left\{{}\begin{matrix}y=-2\\x=-1\end{matrix}\right.\)
Ta có: \(\left\{{}\begin{matrix}2x-3y=4\\x+2y=-5\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}2x-3y=4\\-2x-4y=10\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}-7y=14\\2x-3y=4\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}y=-2\\2x-3\cdot\left(-2\right)=4\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}y=-2\\2x+6=4\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}y=-2\\2x=-2\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}y=-2\\x=-1\end{matrix}\right.\)
Vậy: Hệ phương trình có nghiệm duy nhất là \(\left\{{}\begin{matrix}x=-1\\y=-2\end{matrix}\right.\)