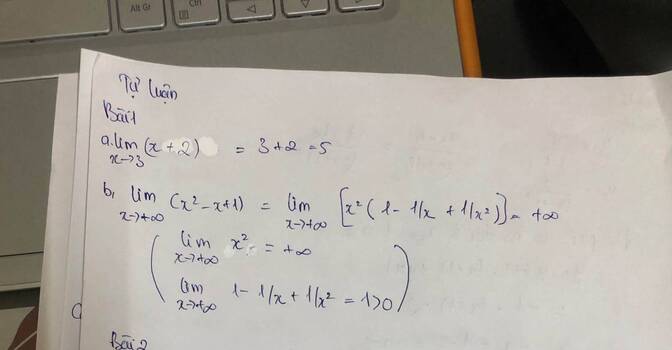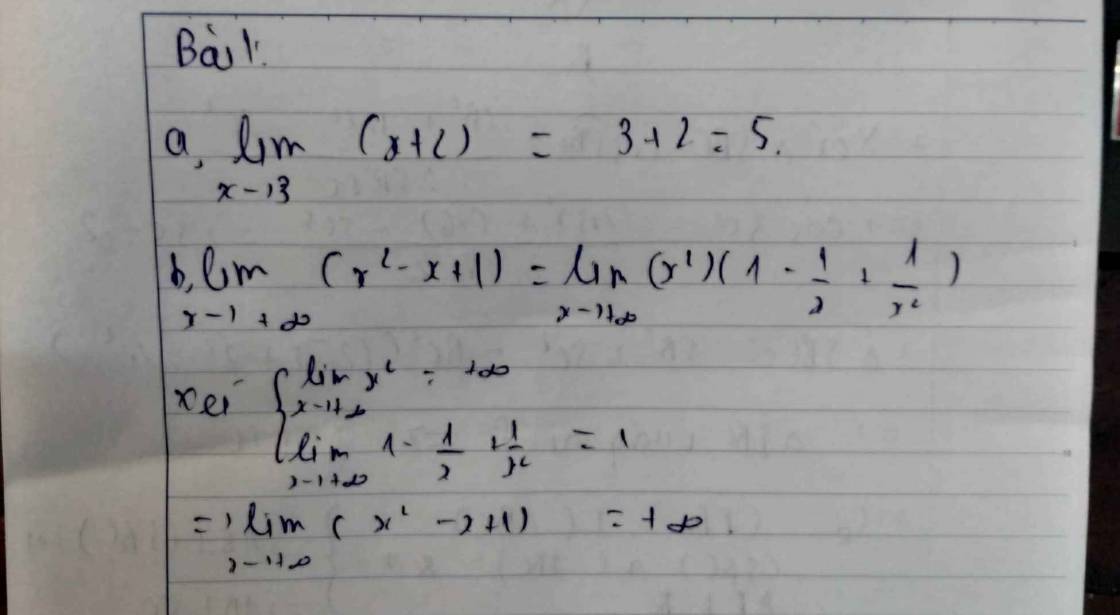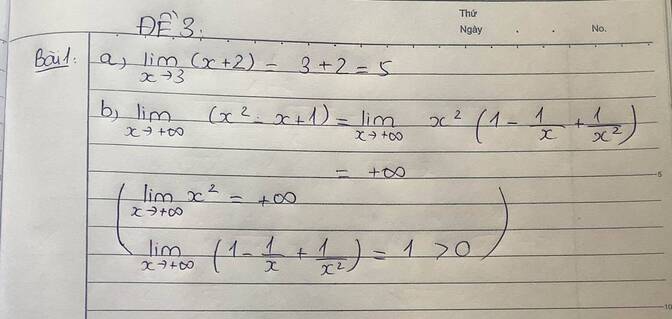Tính các giới hạn sau lim x → 1 4 x 5 + 9 x + 7 3 x 6 + x 3 + 1

Những câu hỏi liên quan
Tính các giới hạn sau:
a) $\underset{x\to 3}{\mathop{\lim }}\,\left( x+2 \right);$
b) $\underset{x\to +\infty }{\mathop{\lim }}\,\left( {{x}^{2}}-x+1 \right).$
4. Tính giới hạn \(\lim\limits_{x\rightarrow0}\dfrac{\sqrt{x^2+1}-x-1}{2x^2-x}_{ }\)
5. Tính giới hạn:
a) \(\lim\limits_{x\rightarrow2}\dfrac{x-2}{x^2-4}_{ }\)
b) \(\lim\limits_{x\rightarrow3^-}\dfrac{x+3}{x-3}_{ }\)
\(\lim\limits_{x\rightarrow0}\dfrac{\sqrt{x^2+1}-\left(x+1\right)}{2x^2-x}=\lim\limits_{x\rightarrow0}\dfrac{\left(\sqrt{x^2+1}-\left(x+1\right)\right)\left(\sqrt{x^2+1}+x+1\right)}{x\left(2x-1\right)\left(\sqrt{x^2+1}+x+1\right)}\)
\(=\lim\limits_{x\rightarrow0}\dfrac{-2x}{x\left(2x-1\right)\left(\sqrt{x^2+1}+x+1\right)}\)
\(=\lim\limits_{x\rightarrow0}\dfrac{-2}{\left(2x-1\right)\left(\sqrt{x^2+1}+x+1\right)}\)
\(=\dfrac{-2}{\left(0-1\right)\left(\sqrt{1}+1\right)}=1\)
a. \(\lim\limits_{x\rightarrow2}\dfrac{x-2}{x^2-4}=\lim\limits_{x\rightarrow2}\dfrac{x-2}{\left(x-2\right)\left(x+2\right)}=\lim\limits_{x\rightarrow2}\dfrac{1}{x+2}=\dfrac{1}{4}\)
b. \(\lim\limits_{x\rightarrow3^-}\dfrac{x+3}{x-3}=\lim\limits_{x\rightarrow3^-}\dfrac{-x-3}{3-x}\)
Do \(\lim\limits_{x\rightarrow3^-}\left(-x-3\right)=-6< 0\)
\(\lim\limits_{x\rightarrow3^-}\left(3-x\right)=0\) và \(3-x>0;\forall x< 3\)
\(\Rightarrow\lim\limits_{x\rightarrow3^-}\dfrac{-x-3}{3-x}=-\infty\)
Đúng 1
Bình luận (0)
Tính các giới hạn
a) \(\lim\limits_{x\rightarrow2}\dfrac{x+3}{x^2+x+4}=\dfrac{1}{2}\)
b) \(\lim\limits_{x\rightarrow-3}\dfrac{x^2+5x+6}{x^2+3x}=\dfrac{1}{3}\)
a/ \(\lim\limits_{x\rightarrow2}\dfrac{2+3}{4+2+4}=\dfrac{5}{10}=\dfrac{1}{2}\)
b/ \(\lim\limits_{x\rightarrow-3}\dfrac{\left(x+2\right)\left(x+3\right)}{x\left(x+3\right)}=\lim\limits_{x\rightarrow-3}\dfrac{x+2}{x}=\dfrac{-3+2}{-3}=\dfrac{1}{3}\)
Đúng 1
Bình luận (0)
Tính các giới hạn sau:a) mathop {lim }limits_{x to + infty } frac{{9x + 1}}{{3x - 4}}; b) mathop {lim }limits_{x to - infty } frac{{7x - 11}}{{2x + 3}}; c) mathop {lim }limits_{x to + infty } frac{{sqrt {{x^2} + 1} }}{x};d) mathop {lim }limits_{x to - infty } frac{{sqrt {{x^2} + 1} }}{x}; e) mathop {lim }limits_{x to {6^ - }} frac{1}{{x - 6}}; g) mathop {lim }limits_{x to {7^ + }} frac{1}{{x - 7}}.
Đọc tiếp
Tính các giới hạn sau:
a) \(\mathop {\lim }\limits_{x \to + \infty } \frac{{9x + 1}}{{3x - 4}};\)
b) \(\mathop {\lim }\limits_{x \to - \infty } \frac{{7x - 11}}{{2x + 3}};\)
c) \(\mathop {\lim }\limits_{x \to + \infty } \frac{{\sqrt {{x^2} + 1} }}{x};\)
d) \(\mathop {\lim }\limits_{x \to - \infty } \frac{{\sqrt {{x^2} + 1} }}{x};\)
e) \(\mathop {\lim }\limits_{x \to {6^ - }} \frac{1}{{x - 6}};\)
g) \(\mathop {\lim }\limits_{x \to {7^ + }} \frac{1}{{x - 7}}.\)
a) \(\mathop {\lim }\limits_{x \to + \infty } \frac{{9x + 1}}{{3x - 4}} = \mathop {\lim }\limits_{x \to + \infty } \frac{{x\left( {9 + \frac{1}{x}} \right)}}{{x\left( {3 - \frac{4}{x}} \right)}} = \mathop {\lim }\limits_{x \to + \infty } \frac{{9 + \frac{1}{x}}}{{3 - \frac{4}{x}}} = \frac{{9 + 0}}{{3 - 0}} = 3\)
b) \(\mathop {\lim }\limits_{x \to - \infty } \frac{{7x - 11}}{{2x + 3}} = \mathop {\lim }\limits_{x \to - \infty } \frac{{x\left( {7 - \frac{{11}}{x}} \right)}}{{x\left( {2 + \frac{3}{x}} \right)}} = \mathop {\lim }\limits_{x \to - \infty } \frac{{7 - \frac{{11}}{x}}}{{2 + \frac{3}{x}}} = \frac{{7 - 0}}{{2 + 0}} = \frac{7}{2}\)
c) \(\mathop {\lim }\limits_{x \to + \infty } \frac{{\sqrt {{x^2} + 1} }}{x} = \mathop {\lim }\limits_{x \to + \infty } \frac{{x\sqrt {1 + \frac{1}{{{x^2}}}} }}{x} = \mathop {\lim }\limits_{x \to + \infty } \sqrt {1 + \frac{1}{{{x^2}}}} = \sqrt {1 + 0} = 1\)
Đúng 0
Bình luận (0)
d) \(\mathop {\lim }\limits_{x \to - \infty } \frac{{\sqrt {{x^2} + 1} }}{x} = \mathop {\lim }\limits_{x \to - \infty } \frac{{ - x\sqrt {1 + \frac{1}{{{x^2}}}} }}{x} = \mathop {\lim }\limits_{x \to - \infty } - \sqrt {1 + \frac{1}{{{x^2}}}} = - \sqrt {1 + 0} = - 1\)
e) Ta có: \(\left\{ \begin{array}{l}1 > 0\\x - 6 < 0,x \to {6^ - }\end{array} \right.\)
Do đó, \(\mathop {\lim }\limits_{x \to {6^ - }} \frac{1}{{x - 6}} = - \infty \)
g) Ta có: \(\left\{ \begin{array}{l}1 > 0\\x + 7 > 0,x \to {7^ + }\end{array} \right.\)
Do đó, \(\mathop {\lim }\limits_{x \to {7^ + }} \frac{1}{{x - 7}} = + \infty \)
Đúng 0
Bình luận (0)
Tính các giới hạn sau :
1/\(\lim\limits_{x\rightarrow-\infty}\left(\sqrt{4x^2-3x+1}+2x\right)\)
2/\(lim\left(\sqrt{4n^2+2n+1}-2n+2020\right)\)
2: \(=lim\left(\dfrac{4n^2+2n+1-4n^2}{\sqrt{4n^2+2n+1}+2n}+2020\right)\)
\(=lim\left(\dfrac{2n+1}{\sqrt{4n^2+2n+1}+2n}+2020\right)\)
\(=lim\left(\dfrac{2+\dfrac{1}{n}}{\sqrt{4+\dfrac{2}{n}+\dfrac{1}{n^2}}+2}+2020\right)\)
\(=\dfrac{2}{2+2}+2020=\dfrac{2}{4}+2020=2020.5\)
Đúng 0
Bình luận (1)
Tìm các giới hạn sau :
a, lim\(\dfrac{2x^2+x-6}{x^3+8}\) khi x→-2
b, lim\(\dfrac{x^4-x^2-72}{x^2-2x-3}\) khi x→3
c, lim\(\dfrac{x^5+1}{x^3+1}\) khi x→-1
d, lim \(\left(\dfrac{2}{x^2-1}-\dfrac{1}{x-1}\right)\) khi x→1
a) \(\lim\limits_{x\rightarrow-2}\dfrac{2x^2+x-6}{x^3+8}=\lim\limits_{x\rightarrow-2}\dfrac{\left(2x-3\right)\left(x+2\right)}{\left(x+2\right)\left(x^2-2x+4\right)}\\ =\lim\limits_{x\rightarrow-2}\dfrac{2x-3}{x^2-2x+4}=-\dfrac{7}{12}\).
b) \(\lim\limits_{x\rightarrow3}\dfrac{x^4-x^2-72}{x^2-2x-3}=\lim\limits_{x\rightarrow3}\dfrac{\left(x^2+8\right)\left(x+3\right)\left(x-3\right)}{\left(x-3\right)\left(x+1\right)}\\ =\lim\limits_{x\rightarrow3}\dfrac{\left(x^2+8\right)\left(x+3\right)}{x+1}=\dfrac{51}{2}\).
c) \(\lim\limits_{x\rightarrow-1}\dfrac{x^5+1}{x^3+1}=\lim\limits_{x\rightarrow-1}\dfrac{\left(x+1\right)\left(x^4-x^3+x^2-x+1\right)}{\left(x+1\right)\left(x^2-x+1\right)}\\ =\lim\limits_{x\rightarrow-1}\dfrac{x^4-x^3+x^2-x+1}{x^2-x+1}=\dfrac{5}{3}\).
d) \(\lim\limits_{x\rightarrow1}\left(\dfrac{2}{x^2-1}-\dfrac{1}{x-1}\right)=\lim\limits_{x\rightarrow1}\left(\dfrac{2}{\left(x-1\right)\left(x+1\right)}-\dfrac{x+1}{\left(x-1\right)\left(x+1\right)}\right)\\ =\lim\limits_{x\rightarrow1}\dfrac{1-x}{\left(x-1\right)\left(x+1\right)}=\lim\limits_{x\rightarrow1}\dfrac{-1}{x+1}=-\dfrac{1}{2}\).
Đúng 1
Bình luận (1)
tính các giới hạn sau:
a. \(lim\dfrac{\sqrt{x+1}-x+1}{x^2-5x+6}\)
x->3
b. \(lim\left|x^3-3x\right|\)
x->-2
Câu a.
\(^{lim}_{x\rightarrow3}\dfrac{\sqrt{x+1}-x+1}{x^2-5x+6}\)
Nhân liên hợp ta đc:
\(^{lim}_{x\rightarrow3}\dfrac{x+1-\left(x-1\right)^2}{(x^2-5x+6)\cdot\left(\sqrt{x+1}+x-1\right)}\)
\(=^{lim}_{x\rightarrow3}\dfrac{-x^2+3x}{\left(x-3\right)\left(x-2\right)\left(\sqrt{x+1}+x-1\right)}\)
\(=^{lim}_{x\rightarrow3}\dfrac{-x}{\left(x-2\right)\cdot\left(\sqrt{x+1}+x-1\right)}\)
\(=\dfrac{-3}{\left(3-2\right)\cdot\left(\sqrt{3+1}+3-1\right)}=-\dfrac{3}{4}\)
Đúng 1
Bình luận (0)
Câu b.
\(^{lim}_{x\rightarrow-2}\left|x^3-3x\right|\)
\(=\left|\left(-2\right)^3-3\cdot\left(-2\right)\right|=\left|-2\right|=2\)
Câu này đơn giản chỉ thay số thôi nhé, nó ở dạng đa thức nữa!
Đúng 1
Bình luận (0)
Tính các giới hạn sau:
a) \(\lim\limits_{x\rightarrow0^-}\dfrac{2\left|x\right|+x}{x^2-x}\)
b) \(\lim\limits_{x\rightarrow-\infty}\left(\sqrt{x^2-x}-\sqrt{x^2-1}\right)\)
c) \(\lim\limits_{x\rightarrow-\infty}\dfrac{\sqrt[3]{1+x^4+x^6}}{\sqrt{1+x^3+x^4}}\)
a: \(\lim\limits_{x->0^-^-}\dfrac{-2x+x}{x\left(x-1\right)}=lim_{x->0^-}\left(\dfrac{-x}{x\left(x-1\right)}\right)\)
\(=lim_{x->0^-}\left(\dfrac{-1}{x-1}\right)=\dfrac{-1}{0-1}=\dfrac{-1}{-1}=1\)
b: \(=lim_{x->-\infty}\left(\dfrac{x^2-x-x^2+1}{\sqrt{x^2-x}+\sqrt{x^2-1}}\right)\)
\(=lim_{x->-\infty}\left(\dfrac{-x+1}{\sqrt{x^2-x}+\sqrt{x^2-1}}\right)\)
\(=lim_{x->-\infty}\left(\dfrac{-1+\dfrac{1}{x}}{-\sqrt{1-\dfrac{1}{x^2}}-\sqrt{1-\dfrac{1}{x^2}}}\right)=\dfrac{-1}{-2}=\dfrac{1}{2}\)
Đúng 0
Bình luận (1)
BÀI 3. Tính các giới hạn sau:
a) \(\lim\limits_{x\rightarrow-\infty}\dfrac{2x^3-5x^2+1}{7x^2-x+4}\)
b) \(\lim\limits_{x\rightarrow+\infty}x\sqrt{\dfrac{x^2+2x+3}{3x^4+4x^2-5}}\)
a: \(=lim_{x->-\infty}\dfrac{2x-5+\dfrac{1}{x^2}}{7-\dfrac{1}{x}+\dfrac{4}{x^2}}\)
\(=\dfrac{2x-5}{7}\)
\(=\dfrac{2}{7}x-\dfrac{5}{7}\)
\(=-\infty\)
b: \(=lim_{x->+\infty}x\sqrt{\dfrac{1+\dfrac{1}{x}+\dfrac{3}{x^2}}{3x^2+4-\dfrac{5}{x^2}}}\)
\(=lim_{x->+\infty}x\sqrt{\dfrac{1}{3x^2+4}}=+\infty\)
Đúng 1
Bình luận (0)
Tìm các giới hạn sau:
a) \(\mathop {\lim }\limits_{x \to {4^ + }} \frac{1}{{x - 4}}\);
c) \(\mathop {\lim }\limits_{x \to {2^ - }} \frac{x}{{2 - x}}\).
a) Áp dụng giới hạn một bên thường dùng, ta có : \(\mathop {\lim }\limits_{x \to {4^ + }} \frac{1}{{x - 4}} = + \infty \)
b) \(\mathop {\lim }\limits_{x \to {2^ + }} \frac{x}{{2 - x}} = \mathop {\lim }\limits_{x \to {2^+ }} \frac{{ - x}}{{x - 2}} = \mathop {\lim }\limits_{x \to {2^ + }} \left( { - x} \right).\mathop {\lim }\limits_{x \to {2^ + }} \frac{1}{{x - 2}}\)
Ta có: \(\mathop {\lim }\limits_{x \to {2^ + }} \left( { - x} \right) = - \mathop {\lim }\limits_{x \to {2^ + }} x = - 2;\mathop {\lim }\limits_{x \to {2^ +}} \frac{1}{{x - 2}} = +\infty \)
\( \Rightarrow \mathop {\lim }\limits_{x \to {2^ - }} \frac{x}{{2 - x}} = - \infty \)
Đúng 0
Bình luận (0)