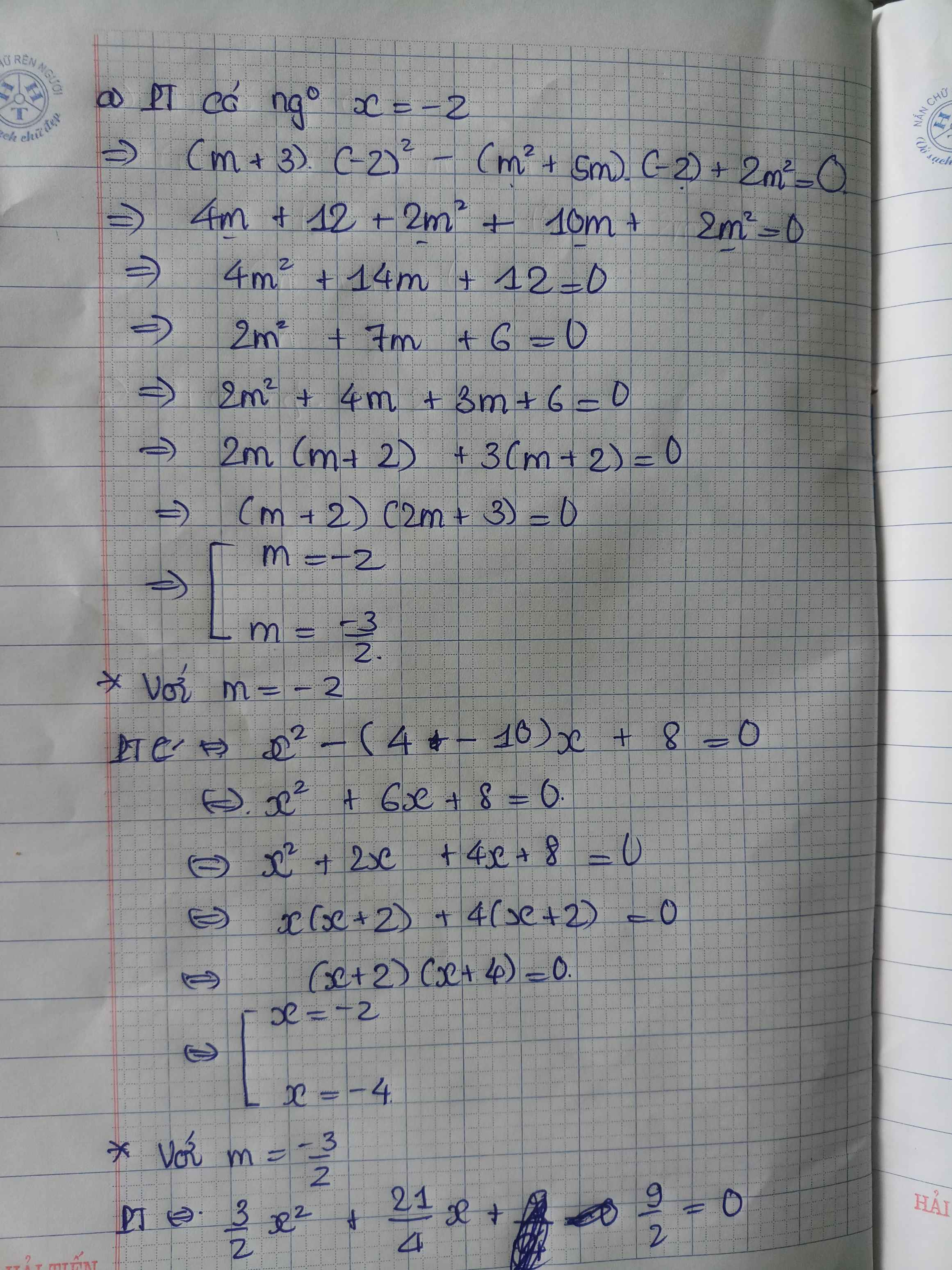tìm m để bất phương trình sau có tập nghiệm là R \(-x^2+\left(2m-1\right)x+m< 0\)

Những câu hỏi liên quan
Tìm tham số m để bất phương trình sau có tập nghiệm là
R:\(x^2+\left(m-2\right)x+m+1>0\)
Để bất phương trình có tập nghiệm là R thì \(\left(m-2\right)^2-4\left(m+1\right)< 0\)
\(\Rightarrow m^2-4m+4-4m-4< 0\)
=>m(m-8)<0
=>0<m<8
Đúng 2
Bình luận (0)
Để bất phương trình đã cho có tập nghiệm là R thì
\(\left\{{}\begin{matrix}a>0\\\Delta\le0\end{matrix}\right.\) (với a là hệ số của x2 và bằng 1, thỏa)
\(\Rightarrow\) (m-2)2-4.(m+1)\(\le\)0 \(\Leftrightarrow\) m2-8m\(\le\)0 \(\Leftrightarrow\) 0\(\le\)m\(\le\)8.
Đúng 0
Bình luận (0)
Cho bất phương trình: \(\left(2m-1\right)x^3+\left(3-3m\right)x^2+\left(m-4\right)x+2\ge0\)
Tìm m để tập nghiệm chứa \(\left(0;+\infty\right)\)
- Với \(m=\dfrac{1}{2}\) ko thỏa mãn
- Với \(m\ne\dfrac{1}{2}\)
\(\Leftrightarrow\left(2m-1\right)x^3-\left(2m-1\right)x^2-\left(m-2\right)x^2+\left(m-4\right)x+2\ge0\)
\(\Leftrightarrow\left(2m-1\right)x^2\left(x-1\right)-\left(x-1\right)\left[\left(m-2\right)x+2\right]\ge0\)
\(\Leftrightarrow\left(x-1\right)\left[\left(2m-1\right)x^2-\left(m-2\right)x-2\right]\ge0\) (1)
Do (1) luôn chứa 1 nghiệm \(x=1\in\left(0;+\infty\right)\) nên để bài toán thỏa mãn thì cần 2 điều sau đồng thời xảy ra:
+/ \(2m-1>0\Rightarrow m>\dfrac{1}{2}\)
+/ \(\left(2m-1\right)x^2-\left(m-2\right)x-2=0\) có 2 nghiệm trong đó \(x_1\le0\) và \(x_2=1\)
Thay \(x=1\) vào ta được:
\(\left(2m-1\right)-\left(m-2\right)-2=0\Leftrightarrow m=1\)
Khi đó: \(x^2+x-2=0\) có 2 nghiệm \(\left[{}\begin{matrix}x_1=-2< 0\left(thỏa\right)\\x_2=1\end{matrix}\right.\)
Vậy \(m=1\)
Đúng 1
Bình luận (0)
Tìm m để bất phương trình: \(\left(m-2\right)x^2+2\left(m+1\right)x+2m>0\) vô nghiệm
- Với \(m=2\) BPT luôn có nghiệm
- Với \(m\ne2\) BPT vô nghiệm khi và chỉ khi:
\(\left\{{}\begin{matrix}m-2< 0\\\Delta'=\left(m+1\right)^2-2m\left(m-2\right)\le0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}m< 2\\-m^2+6m+1\le0\end{matrix}\right.\)
\(\Rightarrow m\le3-\sqrt{10}\)
Đúng 1
Bình luận (0)
Tìm m để hai bất phương trình sau có cùng tập nghiệm:
\(x^2\left(x-5\right)>4-5x\) và \(mx-5>x-2m\)
Tìm m để các phương trình sau (dùng công thức nghiệm thu gọn)
a.\(x^2+2\left(m-2\right)x+m^2-3=0\) có nghiệm
b.\(\left(2m-1\right)x-4mx+2m+3=0\) có nghiệm kép
c.\(4x^2-2\left(2m-1\right)x+m^2=0\) vô nghiệm
a: \(\Leftrightarrow\left(2m-4\right)^2-4\left(m^2-3\right)>=0\)
\(\Leftrightarrow4m^2-16m+16-4m^2+12>=0\)
=>-16m>=-28
hay m<=7/4
b: \(\Leftrightarrow16m^2-4\left(2m-1\right)\left(2m+3\right)=0\)
\(\Leftrightarrow16m^2-4\left(4m^2+4m-3\right)=0\)
=>4m-3=0
hay m=3/4
c: \(\Leftrightarrow\left(4m-2\right)^2-4\cdot4\cdot m^2< 0\)
=>-16m+4<0
hay m>1/4
Đúng 0
Bình luận (0)
Tìm m để các hệ bất phương trình sau có nghiệm\(\left\{{}\begin{matrix}3x^2\text{-2(1+m)x+2m-1 < 0}\\mx+2-m\le0\end{matrix}\right.\)
a) Tìm m để phương trình\(\left(m+3\right)x^2-\left(m^2+5m\right)x+2m^2=0\) có nghiệm x=-2
tìm nghiệm còn lại
b Tìm m để phương trình \(\left(m^2-1\right)x^2-2mx+m^2+m+4=0\) có nghiệm x=2
Tìm nghiệm còn
lại?
b) Thay x=2 vào pt, ta được:
\(4\left(m^2-1\right)-4m+m^2+m+4=0\)
\(\Leftrightarrow4m^2-4-4m+m^2+m+4=0\)
\(\Leftrightarrow5m^2-3m=0\)
\(\Leftrightarrow m\left(5m-3\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}m=0\\m=\dfrac{3}{5}\end{matrix}\right.\)
Áp dụng hệ thức Vi-et, ta được:
\(x_1+x_2=\dfrac{2m}{m^2-1}\)
\(\Leftrightarrow\left[{}\begin{matrix}x_2+2=0\\x_2+2=\dfrac{6}{5}:\left(\dfrac{36}{25}-1\right)=\dfrac{30}{11}\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x_2=-2\\x_2=\dfrac{8}{11}\end{matrix}\right.\)
Đúng 1
Bình luận (0)
Câu 1: Tìm m để biểu thức sau luôn âm: (m-4)x2+ (m+1)x + 2m-1
Câu 2: Tìm m để bất phương trình sau có nghiệm đúng với mọi x:
a/ \(\dfrac{3x^2-5x+4}{\left(m-4\right)x^2+\left(1+m\right)x+2m-1}>0\)
b/ \(-4< \dfrac{2x^2+mx-4}{-x^2+x-1}< 6\)
GIÚP MÌNH VỚI Ạ!!!
2.
b, \(-4< \dfrac{2x^2+mx-4}{-x^2+x-1}< 6\)
\(\Leftrightarrow\left\{{}\begin{matrix}-4< \dfrac{2x^2+mx-4}{-x^2+x-1}\left(1\right)\\\dfrac{2x^2+mx-4}{-x^2+x-1}< 6\left(2\right)\end{matrix}\right.\)
\(\left(1\right)\Leftrightarrow4\left(x^2-x+1\right)>2x^2+mx-4\)
\(\Leftrightarrow2x^2-\left(m+4\right)x+8>0\)
Yêu cầu bài toán thỏa mãn khi \(\Delta=m^2+8m-48< 0\Leftrightarrow-12< m< 4\)
\(\left(2\right)\Leftrightarrow-6\left(x^2-x+1\right)< 2x^2+mx-4\)
\(\Leftrightarrow8x^2+\left(m-6\right)x+2>0\)
Yêu cầu bài toán thỏa mãn khi \(\Delta=m^2-12m-28< 0\Leftrightarrow-2< x< 14\)
Vậy \(m\in\left(-2;4\right)\)
Đúng 3
Bình luận (0)
2.
a, Yêu cầu bài toán thỏa mãn khi phương trình \(\left(m-4\right)x^2+\left(1+m\right)x+2m-1>0\) có nghiệm đúng với mọi x
\(\Leftrightarrow\left\{{}\begin{matrix}m-4>0\\\Delta=m^2+2m+1-4\left(m-4\right)\left(2m-1\right)< 0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}m>4\\\left[{}\begin{matrix}m< \dfrac{3}{7}\\m>5\end{matrix}\right.\end{matrix}\right.\)
\(\Leftrightarrow m>5\)
Đúng 3
Bình luận (0)
1.
Yêu cầu bài toán thỏa mãn khi:
\(\left\{{}\begin{matrix}m-4< 0\\\Delta=-7m^2+38m-15< 0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}m< 4\\\left[{}\begin{matrix}m>5\\m< \dfrac{3}{7}\end{matrix}\right.\end{matrix}\right.\)
\(\Rightarrow m< \dfrac{3}{7}\)
Đúng 1
Bình luận (0)
Tìm m để bất phương trình sau có nghiệm: \(x^2-2\left(m+1\right)x+4\left(m+1\right)< 0\)
Bất phương trình đã cho có nghiệm khi:
\(\Delta'=m^2+2m+1-4m-4=m^2-2m-3< 0\)
\(\Leftrightarrow-1< m< 3\)
Đúng 2
Bình luận (0)