x4 -8x2-9=0
tìm x
chi tiết hộ mik

Những câu hỏi liên quan
x4 - x3 - 7x2 - x 6 = 0tìm x hộ mk với
Xem chi tiết
tìm x
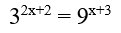
chi tiết nha
\(3^{2x+2}=9^{x+3}\)
\(\Rightarrow3^{2x+2}=\left(3^2\right)^{x+3}\)
\(\Rightarrow3^{2x+2}=3^{2x+6}\)
\(\Rightarrow2x+3=2x+6\)
\(\Rightarrow2x-2x=6-3\)
\(\Rightarrow0=3\) (vô lý)
Vậy không có x phù hợp
Đúng 1
Bình luận (0)
Giải phương trình sau:x4-8x2-9=0
ta có:
x4-8x2-9=0
x4+9x2-x2-9=0
x4-x2+9x2-9=0
x2(x2-1)+9(x2-10=0
(x2-1)(x2+9)=0
=>x2-1=0=>x=1
=>x2+9=0=>x=-3
Đúng 0
Bình luận (0)
\(X^4-8x^2-9=0\left(1\right)\)
Đặt:\(t=x^2\left(t\ge0\right)\)
\(Pt\left(1\right)\Leftrightarrow t^2-8t-9=0\)
Tự giải pt bậc 2 ta đc \(\orbr{\begin{cases}t_1=9\left(n\right)\\t_2=-1\left(l\right)\end{cases}}\)
Với:\(t_{1=9\Rightarrow x^2=9\Rightarrow x=\orbr{\begin{cases}3\\-3\end{cases}}}\)
Đúng 0
Bình luận (0)
16x^2-(4x+1)^2=0
tìm x giải hộ mik
16x2 - (4x+1)2 = 0
16x2 - (16x2+8x+1) = 0
16x2 -16x2 - 8x-1=0
-8x-1=0
-8x=1
x= 1/-8
Đúng 1
Bình luận (0)
\(\Leftrightarrow-8x-4=0\)
hay \(x=-\dfrac{1}{2}\)
Đúng 0
Bình luận (0)
F(x)=x4+5x2-4x+x5-x4-8x2+3+2x3+2
Thu gọn và sắp xếp phải k ạ?
`F(x)= (x^4-x^4)+(5x^2-8x^2)-4x+x^5+3+2x^3+2`
`F(x) = -3x^2-4x+x^5+3+2x^3+2`
`F(x)= x^5+2x^3-3x^2-4x+3+2`
Đúng 1
Bình luận (1)
\(F\left(x\right)=x^4+5x^2-4x+x^5-x^4-8x^2+3+2x^3+2\)
\(F\left(x\right)=x^5+\left(x^4-x^4\right)+2x^3+\left(5x^2-8x^2\right)-4x+\left(3+2\right)\)
\(F\left(x\right)=x^5+2x^3-3x^2-4x+5\)
Đúng 0
Bình luận (0)
Rút gọn các phân thức sau1) 9 - ( x + 5)2 / x2 + 4x + 42) 32x - 8x2 + 2x3 / x3 + 643) 5x3 + 5x / x4 -14) 3x2 - 12x + 12 / x4 - 8x5) 2a2 - 2ab / ac + ad - bc -bd6) x2 - xy / y2 - x27) 2 - 2a / a3 - 18) x7 - x4 / x6 - 19) ( x + 2 )2 - ( x - 2)2 / 16x10) 24,5x2 - 0,5y2 / 3,5x2 - 0,5xy11) a3 - 3a2 + 2a - 6 / a2 +212) ( a - b) ( c - d) / (b2- a2) ( d2 - c2)Giúp mình với ạ, mình cảm ơn !
Đọc tiếp
Rút gọn các phân thức sau
1) 9 - ( x + 5)2 / x2 + 4x + 4
2) 32x - 8x2 + 2x3 / x3 + 64
3) 5x3 + 5x / x4 -1
4) 3x2 - 12x + 12 / x4 - 8x
5) 2a2 - 2ab / ac + ad - bc -bd
6) x2 - xy / y2 - x2
7) 2 - 2a / a3 - 1
8) x7 - x4 / x6 - 1
9) ( x + 2 )2 - ( x - 2)2 / 16x
10) 24,5x2 - 0,5y2 / 3,5x2 - 0,5xy
11) a3 - 3a2 + 2a - 6 / a2 +2
12) ( a - b) ( c - d) / (b2- a2) ( d2 - c2)
Giúp mình với ạ, mình cảm ơn !
1: \(=\dfrac{-\left[\left(x+5\right)^2-9\right]}{\left(x+2\right)^2}=\dfrac{-\left(x+5-3\right)\left(x+5+3\right)}{\left(x+2\right)^2}\)
\(=\dfrac{-\left(x+2\right)\left(x+8\right)}{\left(x+2\right)^2}=\dfrac{-\left(x+8\right)}{x+2}\)
2: \(=\dfrac{2x\left(x^2-4x+16\right)}{\left(x+4\right)\left(x^2-4x+16\right)}=\dfrac{2x}{x+4}\)
3: \(=\dfrac{5x\left(x^2+1\right)}{\left(x^2-1\right)\left(x^2+1\right)}=\dfrac{5x}{x^2-1}\)
4: \(=\dfrac{3\left(x^2-4x+4\right)}{x\left(x^3-8\right)}=\dfrac{3\left(x-2\right)^2}{x\left(x-2\right)\left(x^2+2x+4\right)}\)
\(=\dfrac{3\left(x-2\right)}{x\left(x^2+2x+4\right)}\)
5: \(=\dfrac{2a\left(a-b\right)}{a\left(c+d\right)-b\left(c+d\right)}=\dfrac{2a\left(a-b\right)}{\left(c+d\right)\left(a-b\right)}=\dfrac{2a}{c+d}\)
6: \(=\dfrac{x\left(x-y\right)}{\left(x-y\right)\left(x+y\right)}\cdot\left(-1\right)=\dfrac{-x}{x+y}\)
7: \(=\dfrac{2\left(1-a\right)}{-\left(1-a^3\right)}=\dfrac{-2\left(1-a\right)}{\left(1-a\right)\left(1+a+a^2\right)}=-\dfrac{2}{1+a+a^2}\)
8: \(=\dfrac{x^4\left(x^3-1\right)}{\left(x^3-1\right)\left(x^3+1\right)}=\dfrac{x^4}{x^3+1}\)
9: \(=\dfrac{\left(x+2-x+2\right)\left(x+2+x-2\right)}{16x}=\dfrac{4\cdot2x}{16x}=\dfrac{1}{2}\)
10: \(=\dfrac{0.5\left(49x^2-y^2\right)}{0.5x\left(7x-y\right)}=\dfrac{1}{x}\cdot\dfrac{\left(7x-y\right)\left(7x+y\right)}{7x-y}\)
\(=\dfrac{7x+y}{x}\)
Đúng 0
Bình luận (0)
Giải phương trình : 2sin2x.cos3x-sin5x=0
Tìm m để phương trình sau có nghiệm : 2cos3x-3m+1=0
Mn giải chi tiết ghúp mik nha . Thanks
b: =>cos3x=(3m-1)/2
Để phương trình có nghiệm thì -1<=(3m-1)/2<=1
=>-2<=3m-1<=2
=>-1<=3m<=3
=>-1/3<=m<=1
a: 2*sin2x*cos3x-sin5x=0
=>2*1/2*(sin5x+sin(-x))-sin5x=0
=>sin(-x)=0
=>sin x=0
=>x=kpi
Đúng 2
Bình luận (0)
1) x4 - 8x2 + 4x + 3 = 0
2) x4 - 3x3 - 7x2 + 24x - 8 = 0
3) x4 - x3 - x2 + x + 1 = 0
Giải phương trình??? sử dụng Hooc-ne cho nhanh nhá :v
1) \(x^4-8x^2+4x+3=0\)
( dùng máy tính ta đoán được 1 nghiệm chính xác là -3 )
\(\Leftrightarrow\left(x+3\right)\left(x^3-3x^2+x+1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x+3=0\\x^3-3x^2+x+1=0\left(2\right)\end{matrix}\right.\)
Tiếp tục dùng máy tính ta tìm được 1 nghiệm chính xác của pt ( 2 ) là 1
\(\Leftrightarrow\left(x+3\right)\left(x-1\right)\left(x^2-2x-1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x+3=0\\x-1=0\\x^2-2x-1=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=-3\\x=1\\x=1+\sqrt{2}\\x=1-\sqrt{2}\end{matrix}\right.\)
rồi mấy câu còn lại tương tự
Đúng 1
Bình luận (0)
Hàm số
y
−
x
4
+
8
x
2
−
3
đạt cực đại tại A.
x
−
3
B.
x
13
C.
x
0
D.
x
±
2
Đọc tiếp
Hàm số y = − x 4 + 8 x 2 − 3 đạt cực đại tại
A. x = − 3
B. x = 13
C. x = 0
D. x = ± 2
Đáp án D
Ta có: y ' = − 4 x 3 + 16 x = 0 ⇔ x = 0 x = ± 2
Hàm số có a < 0 ⇒ hàm số đạt cực đại tại x = ± 2
Đúng 0
Bình luận (0)
2x^2−(4m+3)x+2m^2−1=0
tìm tất cả các giá trị của tham số m để pt có 2 nghiệm phân biệt trong đó có ột nghiệm bằng 1
ai đó giải cực kì chi tiết hộ e với rảnh thì hộ e
PT có 2 nghiệm phân biệt
`<=>(4m+3)^2-8(2m^2-1)>0`
`<=>16m^2+24m+9-16m^2+8>0`
`<=>24m+17>0`
`<=>24m> -17`
`<=>m>(-17)/24`
PT có 1 nghiệm =1 thì ta thay x=1 thì pt =
`=>2.1-(4m+3).1+2m^2-1=0`
`<=>2m^2-1-(4m+3)+2=0`
`<=>2m^2+1-4m-3=0`
`<=>2m^2-4m-2=0`
`<=>m^2-2m-1=0`
`a=1,b=-2,c=-1`
`Delta'=1+1=2`
`=>x_1=1+sqrt2(tm),1-sqrt2(tm)`
Vậy `m=1+-sqrt2` thì PT có 2 nghiệm phân biệt có 1 nghiệm = 1
Đúng 1
Bình luận (1)
PT có 1 nghiệm là `1 <=> 2-(4m+3)+2m^2-1=0`
`<=> 2m^2-4m-2=0`
`<=>m=1 \pm \sqrt2`.
Đúng 1
Bình luận (10)
viet : \(\left\{{}\begin{matrix}1+x_2=\dfrac{4m+3}{2}\\1\cdot x_2=\dfrac{2m^2-1}{2}\end{matrix}\right.\Rightarrow2\left(m-1\right)^2=0\Rightarrow m=1\)
Đúng 0
Bình luận (1)





























