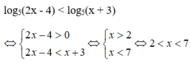Giải bất phương trình: 3 – 2x > 4

Những câu hỏi liên quan
Giải bất phương trình: 2 x + 3 - 4 ≥ 4 - x - 3
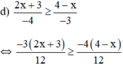
(Quy đồng mẫu)
⇔ -3(2x + 3) ≥ -4(4 – x )(nhân cả hai về với 12 > 0).
⇔ -6x – 9 ≥ -16 + 4x
⇔ 16 – 9 ≥ 4x + 6x (Chuyển vế và đổi dấu hạng tử -6x và -16)
⇔ 7 ≥ 10x
⇔ 0,7 ≥ x hay x ≤ 0,7
Vậy bất phương trình có nghiệm x ≤ 0,7.
Đúng 0
Bình luận (0)
giải bất phương trình (x-1)x(3-2x)/2x-4 > 0
\(y=\dfrac{\left(x-1\right)\left(3-2x\right)}{2x-4}>0\)
nghiệm của y: x - 1 = 0 <=> x = 1
3 - 2x = 0 <=> x = 3/2
y không xác định: 2x - 4 = 0 <=> x = 2
| x | -∞ 1 3/2 2 +∞ |
| x - 1 | - 0 + | + | + |
| 3 - 2x | - | - 0 + | + |
| 2x - 4 | - | - | - 0 + |
| dấu y | - 0 + 0 - || + |
vậy: \(S=\left(1;\dfrac{3}{2}\right)\cup\left(2;+\text{∞}\right)\)
Đúng 0
Bình luận (0)
Giải bất phương trình: 1 x + 2 x + 4 < 3 x + 3
Điều kiện xác định x ≠ 0; x ≠ –3; x ≠ –4.
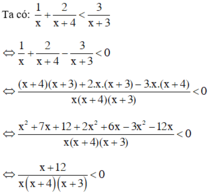
Đặt 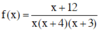 . Ta có bảng xét dấu sau:
. Ta có bảng xét dấu sau:

Dựa vào bảng xét dấu ta thấy
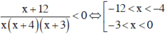
Vậy tập nghiệm của bất phương trình là S = (–12; –4) ∪ (–3; 0).
Đúng 0
Bình luận (0)
giải bất phương trình 2x-3/x-1<1/3
giải bất phương trình 2x-3/x-1 > 1/3
\(\dfrac{2x-3}{x-1}< \dfrac{1}{3}\left(đk:x\ne1\right)\)
\(\Leftrightarrow6x-9< x-1\Leftrightarrow5x< 8\Leftrightarrow x< \dfrac{8}{5}\) và ĐK \(x\ne1\)
\(\dfrac{2x-3}{x-1}>\dfrac{1}{3}\left(đk:x\ne1\right)\)
\(\Leftrightarrow x-1< 6x-9\Leftrightarrow5x>8\Leftrightarrow x>\dfrac{8}{5}\) và ĐK \(x\ne1\)
Đúng 1
Bình luận (0)
Giải phương trìnhsau x/2x-6-x/2x+2=2x/(x+1)(x-3) Giải bất phương trình sau 12x+1/12_< 9x+1/3 - 8x+1/4
\(\dfrac{x}{2x-6}-\dfrac{x}{2x+2}=\dfrac{2x}{\left(x+1\right)\left(x-3\right)}\left(ĐKXĐ:x\ne-1,x\ne3\right)\)
\(\Leftrightarrow\dfrac{x}{2\left(x-3\right)}-\dfrac{x}{2\left(x+1\right)}=\dfrac{2x}{\left(x+1\right)\left(x-3\right)}\)
\(\Leftrightarrow\dfrac{x\left(x+1\right)}{2\left(x+1\right)\left(x-3\right)}-\dfrac{x\left(x-3\right)}{2\left(x+1\right)\left(x-3\right)}=\dfrac{2x\cdot2}{2\left(x+1\right)\left(x-3\right)}\)
\(\Rightarrow x\left(x+1\right)-x\left(x-3\right)=4x\)
\(\Leftrightarrow x^2+x-x^2+3x=4x\)
\(\Leftrightarrow x^2+x-x^2+3x-4x=0\)
\(\Leftrightarrow0x=0\)
Phương trình có vô số nghiệm , trừ x = -1,x = 3
Vậy ...
\(\dfrac{12x+1}{12}< \dfrac{9x+1}{3}-\dfrac{8x+1}{4}\)
\(\Leftrightarrow12\cdot\dfrac{12x+1}{12}< 12\cdot\dfrac{9x+1}{3}-12\cdot\dfrac{8x+1}{4}\)
\(\Leftrightarrow12x+1< 4\left(9x+1\right)-3\left(8x+1\right)\)
\(\Leftrightarrow12x+1< 36x+4-24x-3\)
\(\Leftrightarrow12x+1< 12x+1\)
\(\Leftrightarrow12x-12x< 1-1\)
\(\Leftrightarrow0x< 0\)
Vậy S = {x | x \(\in R\)}
Đúng 2
Bình luận (0)
Giải bất phương trình log 5 ( 2 x - 4 ) < log 5 ( x + 3 )
A. 2 < x < 7
B. -3 < x < 7
C. -3 < x < 2
D. x < 7
1) Giải các phương trình sau : a) x-3/x=2-x-3/x+3 b) 3x^2-2x-16=0 2) Giải bất phương trình sau: 4x-3/4>3x-5/3-2x-7/12
\(a,\dfrac{x-3}{x}=\dfrac{x-3}{x+3}\)\(\left(đk:x\ne0,-3\right)\)
\(\Leftrightarrow\dfrac{x-3}{x}-\dfrac{x-3}{x+3}=0\)
\(\Leftrightarrow\dfrac{\left(x-3\right)\left(x+3\right)-x\left(x-3\right)}{x\left(x+3\right)}=0\)
\(\Leftrightarrow x^2-9-x^2+3x=0\)
\(\Leftrightarrow3x-9=0\)
\(\Leftrightarrow3x=9\)
\(\Leftrightarrow x=3\left(n\right)\)
Vậy \(S=\left\{3\right\}\)
Đúng 1
Bình luận (0)
\(b,\dfrac{4x-3}{4}>\dfrac{3x-5}{3}-\dfrac{2x-7}{12}\)
\(\Leftrightarrow\dfrac{4x-3}{4}-\dfrac{3x-5}{3}+\dfrac{2x-7}{12}>0\)
\(\Leftrightarrow\dfrac{3\left(4x-3\right)-4\left(3x-5\right)+2x-7}{12}>0\)
\(\Leftrightarrow12x-9-12x+20+2x-7>0\)
\(\Leftrightarrow2x+4>0\)
\(\Leftrightarrow2x>-4\)
\(\Leftrightarrow x>-2\)
Đúng 1
Bình luận (0)
Bài 1: Giải các bất phương trình và phương trình sau :
a) 2(3-4x) = 10-(2x – 5)
Giải các bất phương trình và phương trình sau :
a) 3(2-4x) = 11-(3x – 1)
Bài 1:
a) Ta có: \(2\left(3-4x\right)=10-\left(2x-5\right)\)
\(\Leftrightarrow6-8x-10+2x-5=0\)
\(\Leftrightarrow-6x+11=0\)
\(\Leftrightarrow-6x=-11\)
hay \(x=\dfrac{11}{6}\)
b) Ta có: \(3\left(2-4x\right)=11-\left(3x-1\right)\)
\(\Leftrightarrow6-12x-11+3x-1=0\)
\(\Leftrightarrow-9x-6=0\)
\(\Leftrightarrow-9x=6\)
hay \(x=-\dfrac{2}{3}\)
Đúng 2
Bình luận (0)
giải bất phương trình :
\(\sqrt{x+2}+\sqrt{4-x}>\sqrt{2x+3}\)
ĐKXĐ: \(-\dfrac{3}{2}\le x\le4\)
BPT tương đương:
\(6+2\sqrt{\left(x+2\right)\left(4-x\right)}>2x+3\)
\(\Leftrightarrow2\sqrt{-x^2+2x+8}>2x-3\)
\(\Leftrightarrow\left[{}\begin{matrix}x< \dfrac{3}{2}\\\left\{{}\begin{matrix}x\ge\dfrac{3}{2}\\4\left(-x^2+2x+8\right)>4x^2-12x+9\end{matrix}\right.\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x< \dfrac{3}{2}\\\left\{{}\begin{matrix}x\ge\dfrac{3}{2}\\8x^2-20x-23< 0\end{matrix}\right.\end{matrix}\right.\)
\(\Rightarrow-\dfrac{3}{2}\le x< \dfrac{5+\sqrt{71}}{4}\)
Đúng 0
Bình luận (0)