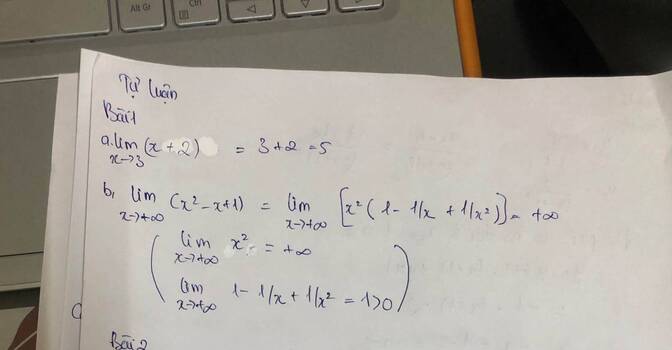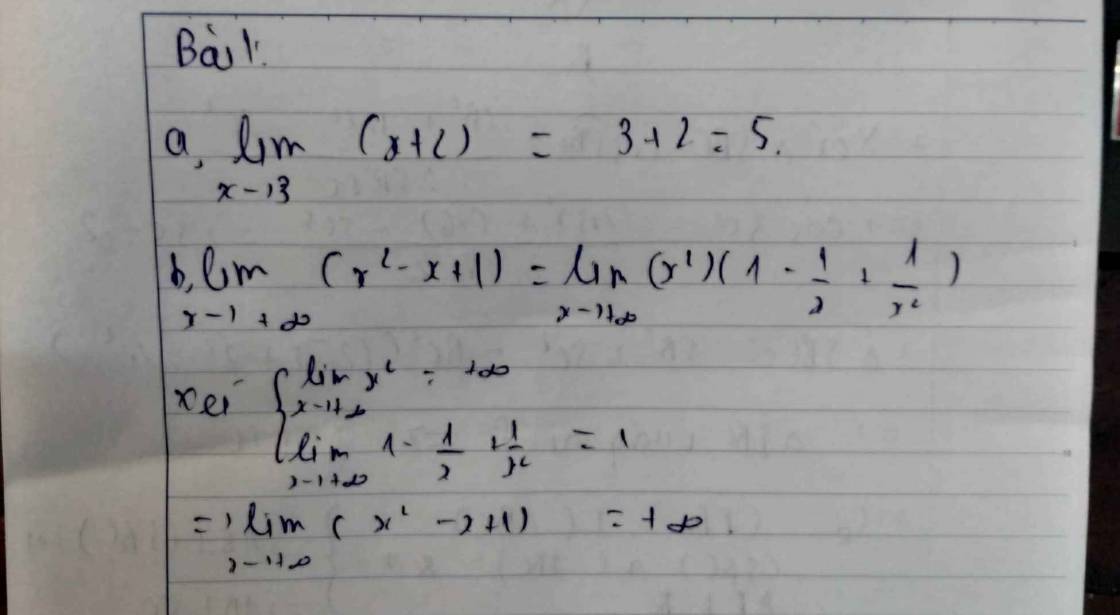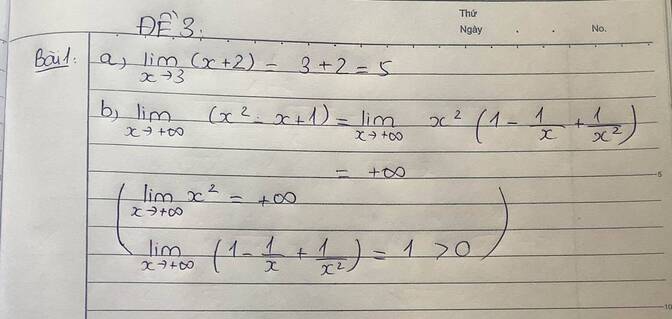Tính các giới hạn lim x → π 3 2 sin 2 x + sin x - 1 2 sin 2 x - 3 sin x + 1
Tuyển Cộng tác viên Hoc24 nhiệm kì 26 tại đây: https://forms.gle/dK3zGK3LHFrgvTkJ6

Những câu hỏi liên quan
tính các giới hạn sau:
a. \(lim\dfrac{\sqrt{x+1}-x+1}{x^2-5x+6}\)
x->3
b. \(lim\left|x^3-3x\right|\)
x->-2
Câu a.
\(^{lim}_{x\rightarrow3}\dfrac{\sqrt{x+1}-x+1}{x^2-5x+6}\)
Nhân liên hợp ta đc:
\(^{lim}_{x\rightarrow3}\dfrac{x+1-\left(x-1\right)^2}{(x^2-5x+6)\cdot\left(\sqrt{x+1}+x-1\right)}\)
\(=^{lim}_{x\rightarrow3}\dfrac{-x^2+3x}{\left(x-3\right)\left(x-2\right)\left(\sqrt{x+1}+x-1\right)}\)
\(=^{lim}_{x\rightarrow3}\dfrac{-x}{\left(x-2\right)\cdot\left(\sqrt{x+1}+x-1\right)}\)
\(=\dfrac{-3}{\left(3-2\right)\cdot\left(\sqrt{3+1}+3-1\right)}=-\dfrac{3}{4}\)
Đúng 1
Bình luận (0)
Câu b.
\(^{lim}_{x\rightarrow-2}\left|x^3-3x\right|\)
\(=\left|\left(-2\right)^3-3\cdot\left(-2\right)\right|=\left|-2\right|=2\)
Câu này đơn giản chỉ thay số thôi nhé, nó ở dạng đa thức nữa!
Đúng 1
Bình luận (0)
Tính các giới hạn sau:
a) \(\mathop {\lim }\limits_{x \to 2} \left( {{x^2} - 4x + 3} \right);\)
b) \(\mathop {\lim }\limits_{x \to 3} \frac{{{x^2} - 5x + 6}}{{x - 3}};\)
c) \(\mathop {\lim }\limits_{x \to 1} \frac{{\sqrt x - 1}}{{x - 1}}.\)
a) \(\mathop {\lim }\limits_{x \to 2} \left( {{x^2} - 4x + 3} \right) = \mathop {\lim }\limits_{x \to 2} {x^2} - \mathop {\lim }\limits_{x \to 2} \left( {4x} \right) + 3 = {2^2} - 4.2 + 3 = - 1\)
b) \(\mathop {\lim }\limits_{x \to 3} \frac{{{x^2} - 5x + 6}}{{x - 3}} = \mathop {\lim }\limits_{x \to 3} \frac{{\left( {x - 3} \right)\left( {x - 2} \right)}}{{x - 3}} = \mathop {\lim }\limits_{x \to 3} \left( {x - 2} \right) = \mathop {\lim }\limits_{x \to 3} x - 2 = 3 - 2 = 1\)
c) \(\mathop {\lim }\limits_{x \to 1} \frac{{\sqrt x - 1}}{{x - 1}} = \mathop {\lim }\limits_{x \to 1} \frac{{\sqrt x - 1}}{{\left( {\sqrt x - 1} \right)\left( {\sqrt x + 1} \right)}} = \mathop {\lim }\limits_{x \to 1} \frac{1}{{\sqrt x + 1}} = \frac{1}{{\sqrt 1 + 1}} = \frac{1}{2}\)
Đúng 0
Bình luận (0)
Tính các giới hạn sau:
a) $\underset{x\to 3}{\mathop{\lim }}\,\left( x+2 \right);$
b) $\underset{x\to +\infty }{\mathop{\lim }}\,\left( {{x}^{2}}-x+1 \right).$
Tính các giới hạn sau:
a) \(\mathop {\lim }\limits_{x \to 3} \left( {2{x^2} - x} \right)\);
b) \(\mathop {\lim }\limits_{x \to - 1} \frac{{{x^2} + 2x + 1}}{{x + 1}}\).
a) Đặt \(f\left( x \right) = 2{x^2} - x\).
Hàm số \(y = f\left( x \right)\) xác định trên \(\mathbb{R}\).
Giả sử \(\left( {{x_n}} \right)\) là dãy số bất kì thỏa mãn \({x_n} \to 3\) khi \(n \to + \infty \). Ta có:
\(\lim f\left( {{x_n}} \right) = \lim \left( {2x_n^2 - {x_n}} \right) = 2.\lim x_n^2 - \lim {x_n} = {2.3^2} - 3 = 15\).
Vậy \(\mathop {\lim }\limits_{x \to 3} \left( {2{x^2} - x} \right) = 15\).
b) Đặt \(f\left( x \right) = \frac{{{x^2} + 2x + 1}}{{x + 1}}\).
Hàm số \(y = f\left( x \right)\) xác định trên \(\mathbb{R}\).
Giả sử \(\left( {{x_n}} \right)\) là dãy số bất kì thỏa mãn \({x_n} \to - 1\) khi \(n \to + \infty \). Ta có:
\(\lim f\left( {{x_n}} \right) = \lim \frac{{x_n^2 + 2{x_n} + 1}}{{{x_n} + 1}} = \lim \frac{{{{\left( {{x_n} + 1} \right)}^2}}}{{{x_n} + 1}} = \lim \left( {{x_n} + 1} \right) = \lim {x_n} + 1 = - 1 + 1 = 0\).
Vậy \(\mathop {\lim }\limits_{x \to - 1} \frac{{{x^2} + 2x + 1}}{{x + 1}} = 0\).
Đúng 0
Bình luận (0)
Tính các giới hạn
\(\lim\limits_{x\rightarrow0}\dfrac{\sqrt{x^2+x+1}-\sqrt[3]{x^3+1}}{x}\)
làm bài lim xem nào :)))
P/s Sở Kiều :))
Hướng làm thôi chứ gõ công thức lâu vl
\(\lim\limits_{x\rightarrow0}\dfrac{\sqrt{x^2+x+1}-\sqrt[3]{x^3+1}}{x}\)
\(=\lim\limits_{x\rightarrow0}\dfrac{\sqrt{x^2+x+1}-1}{x}+\dfrac{1-\sqrt[3]{x^3+1}}{x}\)
Đến đây liên hợp là xong phần của bạn đó ;)
Đúng 1
Bình luận (5)
Tính các giới hạn sau:
a) \(\lim\limits_{x\rightarrow1^+}\dfrac{x^3+x+1}{x-1}\)
b) \(\lim\limits_{x\rightarrow-1^+}\dfrac{3x+2}{x+1}\)
c) \(\lim\limits_{x\rightarrow2^-}\dfrac{x-15}{x-2}\)
Lời giải:
a. \(\lim\limits_{x\to 1+}(x^3+x+1)=3>0\)
\(\lim\limits_{x\to 1+}(x-1)=0\) và $x-1>0$ khi $x>1$
\(\Rightarrow \lim\limits_{x\to 1+}\frac{x^3+x+1}{x-1}=+\infty\)
b.
\(\lim\limits_{x\to -1+}(3x+2)=-1<0\)
\(\lim\limits_{x\to -1+}(x+1)=0\) và $x+1>0$ khi $x>-1$
\(\Rightarrow \lim\limits_{x\to -1+}\frac{3x+2}{x+1}=-\infty\)
c.
\(\lim\limits_{x\to 2-}(x-15)=-17<0\)
\(\lim\limits_{x\to 2-}(x-2)=0\) và $x-2<0$ khi $x<2$
\(\Rightarrow \lim\limits_{x\to 2-}\frac{x-15}{x-2}=+\infty\)
Đúng 1
Bình luận (0)
Tính các giới hạn
a) \(\lim\limits_{x\rightarrow2}\dfrac{x+3}{x^2+x+4}=\dfrac{1}{2}\)
b) \(\lim\limits_{x\rightarrow-3}\dfrac{x^2+5x+6}{x^2+3x}=\dfrac{1}{3}\)
a/ \(\lim\limits_{x\rightarrow2}\dfrac{2+3}{4+2+4}=\dfrac{5}{10}=\dfrac{1}{2}\)
b/ \(\lim\limits_{x\rightarrow-3}\dfrac{\left(x+2\right)\left(x+3\right)}{x\left(x+3\right)}=\lim\limits_{x\rightarrow-3}\dfrac{x+2}{x}=\dfrac{-3+2}{-3}=\dfrac{1}{3}\)
Đúng 1
Bình luận (0)
4. Tính giới hạn \(\lim\limits_{x\rightarrow0}\dfrac{\sqrt{x^2+1}-x-1}{2x^2-x}_{ }\)
5. Tính giới hạn:
a) \(\lim\limits_{x\rightarrow2}\dfrac{x-2}{x^2-4}_{ }\)
b) \(\lim\limits_{x\rightarrow3^-}\dfrac{x+3}{x-3}_{ }\)
\(\lim\limits_{x\rightarrow0}\dfrac{\sqrt{x^2+1}-\left(x+1\right)}{2x^2-x}=\lim\limits_{x\rightarrow0}\dfrac{\left(\sqrt{x^2+1}-\left(x+1\right)\right)\left(\sqrt{x^2+1}+x+1\right)}{x\left(2x-1\right)\left(\sqrt{x^2+1}+x+1\right)}\)
\(=\lim\limits_{x\rightarrow0}\dfrac{-2x}{x\left(2x-1\right)\left(\sqrt{x^2+1}+x+1\right)}\)
\(=\lim\limits_{x\rightarrow0}\dfrac{-2}{\left(2x-1\right)\left(\sqrt{x^2+1}+x+1\right)}\)
\(=\dfrac{-2}{\left(0-1\right)\left(\sqrt{1}+1\right)}=1\)
a. \(\lim\limits_{x\rightarrow2}\dfrac{x-2}{x^2-4}=\lim\limits_{x\rightarrow2}\dfrac{x-2}{\left(x-2\right)\left(x+2\right)}=\lim\limits_{x\rightarrow2}\dfrac{1}{x+2}=\dfrac{1}{4}\)
b. \(\lim\limits_{x\rightarrow3^-}\dfrac{x+3}{x-3}=\lim\limits_{x\rightarrow3^-}\dfrac{-x-3}{3-x}\)
Do \(\lim\limits_{x\rightarrow3^-}\left(-x-3\right)=-6< 0\)
\(\lim\limits_{x\rightarrow3^-}\left(3-x\right)=0\) và \(3-x>0;\forall x< 3\)
\(\Rightarrow\lim\limits_{x\rightarrow3^-}\dfrac{-x-3}{3-x}=-\infty\)
Đúng 1
Bình luận (0)
Tính các giới hạn sau:
a) \(\mathop {\lim }\limits_{x \to - 3} \left( {4{x^2} - 5x + 6} \right)\);
b) \(\mathop {\lim }\limits_{x \to 2} \frac{{2{x^2} - 5x + 2}}{{x - 2}}\);
c) \(\mathop {\lim }\limits_{x \to 4} \frac{{\sqrt x - 2}}{{{x^2} - 16}}\).
a) \(\mathop {\lim }\limits_{x \to - 3} \left( {4{x^2} - 5x + 6} \right) = 4.{\left( { - 3} \right)^2} - 5.\left( { - 3} \right) + 6 = 57\)
b) \(\mathop {\lim }\limits_{x \to 2} \frac{{2{x^2} - 5x + 2}}{{x - 2}} = \mathop {\lim }\limits_{x \to 2} \frac{{\left( {x - 2} \right)\left( {2x - 1} \right)}}{{x - 2}} = \mathop {\lim }\limits_{x \to 2} \left( {2x - 1} \right) = 2.2 - 1 = 3\)
c) \(\begin{array}{c}\mathop {\lim }\limits_{x \to 4} \frac{{\sqrt x - 2}}{{{x^2} - 16}} = \mathop {\lim }\limits_{x \to 4} \frac{{\sqrt x - 2}}{{\left( {x - 4} \right)\left( {x + 4} \right)}} = \mathop {\lim }\limits_{x \to 4} \frac{{\sqrt x - 2}}{{\left( {\sqrt x - 2} \right)\left( {\sqrt x + 2} \right)\left( {x + 4} \right)}} = \mathop {\lim }\limits_{x \to 4} \frac{1}{{\left( {\sqrt x + 2} \right)\left( {x + 4} \right)}}\\ = \frac{1}{{\left( {\sqrt 4 + 2} \right)\left( {4 + 4} \right)}} = \frac{1}{{32}}\end{array}\)
Đúng 0
Bình luận (0)
BÀI 3. Tính các giới hạn sau:
a) \(\lim\limits_{x\rightarrow-\infty}\dfrac{2x^3-5x^2+1}{7x^2-x+4}\)
b) \(\lim\limits_{x\rightarrow+\infty}x\sqrt{\dfrac{x^2+2x+3}{3x^4+4x^2-5}}\)
a: \(=lim_{x->-\infty}\dfrac{2x-5+\dfrac{1}{x^2}}{7-\dfrac{1}{x}+\dfrac{4}{x^2}}\)
\(=\dfrac{2x-5}{7}\)
\(=\dfrac{2}{7}x-\dfrac{5}{7}\)
\(=-\infty\)
b: \(=lim_{x->+\infty}x\sqrt{\dfrac{1+\dfrac{1}{x}+\dfrac{3}{x^2}}{3x^2+4-\dfrac{5}{x^2}}}\)
\(=lim_{x->+\infty}x\sqrt{\dfrac{1}{3x^2+4}}=+\infty\)
Đúng 1
Bình luận (0)