tìm tập xác điịnh của hàm số y=tan\(\frac{\Pi}{x}\)

Những câu hỏi liên quan
Tìm tập xác định của các hàm số sau:
\(\begin{array}{l}a)\;y = \frac{1}{{cosx}}\\b)\;y = tan(x + \frac{\pi }{4})\\c)\;y = \frac{1}{{2 - si{n^2}x}}\end{array}\)
a, ĐK: \(cos\left(x\right)\ne0\Leftrightarrow x\ne\dfrac{\pi}{2}+k\pi\)
Vậy tập xác định của hàm số là: \(D=R\backslash\left\{\dfrac{\pi}{2}+k\pi,k\in Z\right\}\)
b, ĐK: \(cos\left(x+\dfrac{\pi}{4}\right)\ne0\Leftrightarrow x+\dfrac{\pi}{4}\ne\dfrac{\pi}{2}+k\pi\Leftrightarrow x\ne\dfrac{\pi}{4}+k\pi\)
Vậy tập xác định của hàm số là \(D=R\backslash\left\{\dfrac{\pi}{4}+k\pi,k\in Z\right\}\)
c, ĐK: \(2-sin^2\left(x\right)\ne0\Leftrightarrow sin^2\left(x\right)\ne2\)
Vì \(0\le sin^2\left(x\right)\le1\Rightarrow sin^2\left(x\right)\ne2\forall x\)
Vậy tập xác định của hàm số là \(D=R\)
Đúng 1
Bình luận (0)
c1 tập xác định của hàm số \(y=\dfrac{sin2x+cosx}{tanx-sinx}\)
c2 tập xác định của hàm số \(y=\sqrt{1+cot^22x}\)
c3 tập xác định của hàm số \(y=cot\left(x-\dfrac{\pi}{4}\right)+tan\left(x-\dfrac{\pi}{4}\right)\)
1.
ĐKXĐ: \(\left\{{}\begin{matrix}cosx\ne0\\tanx-sinx\ne0\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}cosx\ne0\\\dfrac{sinx}{cosx}-sinx\ne0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}cosx\ne0\\sinx\ne0\\cosx\ne1\end{matrix}\right.\) \(\Leftrightarrow sin2x\ne0\Leftrightarrow x\ne\dfrac{k\pi}{2}\)
2.
ĐKXĐ: \(sin2x\ne0\Leftrightarrow x\ne\dfrac{k\pi}{2}\)
3.
ĐKXĐ: \(\left\{{}\begin{matrix}sin\left(x-\dfrac{\pi}{4}\right)\ne0\\cos\left(x-\dfrac{\pi}{4}\right)\ne0\end{matrix}\right.\)
\(\Leftrightarrow sin\left(2x-\dfrac{\pi}{2}\right)\ne0\Leftrightarrow cos2x\ne0\)
\(\Leftrightarrow x\ne\dfrac{\pi}{4}+\dfrac{k\pi}{2}\)
Đúng 2
Bình luận (5)
câu 2 ..... \(\dfrac{cos^22x}{sin^22x}=cot^22x\) nên suy ra sin2x khác 0 đúng hơm
còn câu 3, tui ko hiểu chỗ sin(2x-pi/4).. sao ở đây rớt xuống dợ
Đúng 1
Bình luận (2)
tìm tập xác định của mỗi hàm số sau : a) y = \(\sqrt{\frac{1-\sin x}{1+\cos x}}\) ; b) y = \(\tan\left(2x+\frac{\pi}{3}\right)\).
a)\(\forall x\Rightarrow sinx\le1\Rightarrow1-sinx\ge0\)
cosx\(\ge-1\Rightarrow1+cosx\ge0\)
ĐK:cosx\(\ne-1\Leftrightarrow x\ne\pi+k2\pi\)
\(\Rightarrow D=\left\{R\backslash\left\{\pi+k2\pi\right\}\right\}\)
b)ĐK:\(cos\left(2x+\frac{\pi}{3}\right)\ne0\Leftrightarrow2x+\frac{\pi}{3}\ne\frac{\pi}{2}+k\pi\Leftrightarrow x\ne\frac{\pi}{12}+\frac{k\pi}{2}\)
\(\Rightarrow D=\left\{R\text{\}\left\{\frac{\pi}{12}+\frac{k\pi}{2}\right\}\right\}\)
Đúng 0
Bình luận (0)
tìm tập xác định của hàm số lượng giác saua)ydfrac{tanleft(2x-dfrac{pi}{4}right)}{sqrt{1-sinleft(x-dfrac{pi}{8}right)}}b)ydfrac{tanleft(x-dfrac{pi}{4}right)}{1-cosleft(x+dfrac{pi}{3}right)}c)ydfrac{3}{cosx-cos3x}d)ydfrac{4}{sin^2x-cos^2x}e)ydfrac{1+cotleft(dfrac{pi}{3}+xright)}{tan^2left(3x-dfrac{pi}{4}right)}
Đọc tiếp
tìm tập xác định của hàm số lượng giác sau
a)\(y=\dfrac{tan\left(2x-\dfrac{\pi}{4}\right)}{\sqrt{1-sin\left(x-\dfrac{\pi}{8}\right)}}\)
b)\(y=\dfrac{tan\left(x-\dfrac{\pi}{4}\right)}{1-cos\left(x+\dfrac{\pi}{3}\right)}\)
c)\(y=\dfrac{3}{cosx-cos3x}\)
d)\(y=\dfrac{4}{sin^2x-cos^2x}\)
e)\(y=\dfrac{1+cot\left(\dfrac{\pi}{3}+x\right)}{tan^2\left(3x-\dfrac{\pi}{4}\right)}\)
tìm tập xác định của hàm số
a) y =\(tan\left(x-\dfrac{2\pi}{3}\right)\)
b) y = \(cot5x\)
c) y = \(cot7x\)
tìm tập xác định của hàm số
a) y = \(tan\left(2x-\dfrac{\pi}{3}\right)\)
b) y = \(tan\left(3x-\dfrac{\pi}{4}\right)\)
tìm tập xác định của các hàm số:
1.y=sin2x
2.y=\(\dfrac{1-cosx}{sinx}\)
3.y=\(\dfrac{1-2sinx}{cos2x}\)
4.y=tan\(\left(x+\dfrac{\pi}{4}\right)\)
1. \(D=R\)
2. \(sinx\ne0\Leftrightarrow x\ne k\pi\Rightarrow D=R\backslash\left\{k\pi|k\in R\right\}\)
3. \(cos2x\ne0\Leftrightarrow2x\ne\dfrac{\pi}{2}+k\pi\Leftrightarrow x\ne\dfrac{\pi}{4}+\dfrac{k\pi}{2}\Rightarrow D=R\backslash\left\{\dfrac{\pi}{4}+\dfrac{k\pi}{2}|k\in R\right\}\)
4. \(cos\left(x+\dfrac{\pi}{4}\right)\ne0\Leftrightarrow x+\dfrac{\pi}{4}\ne\dfrac{\pi}{2}+k\pi\Leftrightarrow x\ne\dfrac{\pi}{4}+k\pi\Rightarrow D=R\backslash\left\{\dfrac{\pi}{4}+k\pi|k\in R\right\}\)
Đúng 2
Bình luận (1)
tìm tập xác định của mỗi hàm số sau : a) y = \(\sqrt{\frac{1-\sin x}{1+\cos x}}\) ; b) y = \(\tan\) \(\left(2x+\frac{\pi}{3}\right)\).
Cho hàm số y tan xa) Xét tính chẵn, lẻ của hàm sốb) Hoàn thành bảng giá trị của hàm số y tan x trên khoảng;left( { - frac{pi }{2};frac{pi }{2}} right). x - frac{pi }{3} - frac{pi }{4} - frac{pi }{6}0frac{pi }{6}frac{pi }{4}frac{pi }{3}y tan x???????Bằng cách lấy nhiều điểm Mleft( {x;tan x} right) với x in left( { - frac{pi }{2};frac{pi }{2}} right) và nối lại ta được đồ thị hàm số y tan x trên khoảng left( { - frac{pi }{2};frac{pi }{2}} right).c) Bằng cách làm tương tự c...
Đọc tiếp
Cho hàm số \(y = \tan x\)
a) Xét tính chẵn, lẻ của hàm số
b) Hoàn thành bảng giá trị của hàm số \(y = \tan x\) trên khoảng\(\;\left( { - \frac{\pi }{2};\frac{\pi }{2}} \right)\).
\(x\) | \( - \frac{\pi }{3}\) | \( - \frac{\pi }{4}\) | \( - \frac{\pi }{6}\) | 0 | \(\frac{\pi }{6}\) | \(\frac{\pi }{4}\) | \(\frac{\pi }{3}\) |
\(y = \tan x\) | ? | ? | ? | ? | ? | ? | ? |
Bằng cách lấy nhiều điểm \(M\left( {x;\tan x} \right)\) với \(x \in \left( { - \frac{\pi }{2};\frac{\pi }{2}} \right)\) và nối lại ta được đồ thị hàm số \(y = \tan x\) trên khoảng \(\left( { - \frac{\pi }{2};\frac{\pi }{2}} \right)\).
c) Bằng cách làm tương tự câu b cho các đoạn khác có độ dài bằng chu kỳ \(T = \pi \), ta được đồ thị của hàm số \(y = \tan x\) như hình dưới đây.
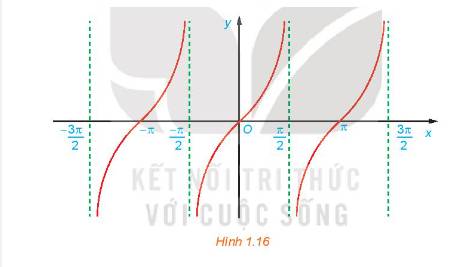
Từ đồ thị ở Hình 1.16, hãy tìm tập giá trị và các khoảng đồng biến của hàm số \(y = \tan x\).
a) Tập xác định của hàm số là \(D = \mathbb{R}\;\backslash \left\{ {\frac{\pi }{2} + k\pi {\rm{|}}\;k\; \in \;\mathbb{Z}} \right\}\)
Do đó, nếu x thuộc tập xác định D thì –x cũng thuộc tập xác định D
Ta có: \(f\left( { - x} \right) = \tan \left( { - x} \right) = - \tan x = - f\left( x \right),\;\forall x\; \in \;D\)
Vậy \(y = \tan x\) là hàm số lẻ.
b)
\(x\) | \( - \frac{\pi }{3}\) | \( - \frac{\pi }{4}\) | \( - \frac{\pi }{6}\) | \(0\) | \(\frac{\pi }{6}\) | \(\frac{\pi }{4}\) | \(\frac{\pi }{3}\) |
\(\tan x\) | \( - \sqrt 3 \) | \( - 1\) | \( - \frac{{\sqrt 3 }}{3}\) | \(0\) | \(\frac{{\sqrt 3 }}{3}\) | \(1\) | \(\sqrt 3 \) |
c) Từ đồ thị trên, ta thấy hàm số \(y = \tan x\) có tập xác định là \(\mathbb{R}\backslash \left\{ {\frac{\pi }{2} + k\pi {\rm{|}}\;k\; \in \;\mathbb{Z}} \right\}\), tập giá trị là \(\mathbb{R}\) và đồng biến trên mỗi khoảng \(\left( { - \frac{\pi }{2} + k\pi ;\frac{\pi }{2} + k\pi } \right)\).
Đúng 0
Bình luận (0)



















