a.

ĐKXĐ: \(x\ge-1\)

\(\Leftrightarrow\left(\sqrt{x+1}+1\right)\left(\sqrt{x+1}+2x-5\right)=x+1-1\)

\(\Leftrightarrow\left(\sqrt{x+1}+1\right)\left(\sqrt{x+1}+2x-5\right)=\left(\sqrt{x+1}+1\right)\left(\sqrt{x+1}-1\right)\)

\(\Leftrightarrow\sqrt{x+1}+2x-5=\sqrt{x+1}-1\)

\(\Leftrightarrow2x-5=-1\)

\(\Leftrightarrow x=2\)

b.

ĐKXĐ: \(x\ge-\dfrac{5}{3}\)

\(6x+10+4\sqrt{6x+10}+4=4x^2+20x+25\)

\(\Leftrightarrow\left(\sqrt{6x+10}+4\right)^2=\left(2x+5\right)^2\)

\(\Leftrightarrow\left[{}\begin{matrix}\sqrt{6x+10}+4=2x+5\\\sqrt{6x+10}+4=-2x-5\end{matrix}\right.\)

\(\Leftrightarrow\left[{}\begin{matrix}\sqrt{6x+10}=2x+1\left(1\right)\\\sqrt{6x+10}=-2x-9< 0\left(loại\right)\end{matrix}\right.\)

(1) \(\Leftrightarrow6x+10=4x^2+4x+1\) \(\left(x\ge-\dfrac{1}{2}\right)\)

\(\Leftrightarrow4x^2-2x-9=0\)

\(\Rightarrow x=\dfrac{1+\sqrt{37}}{4}\)

a) ĐKXĐ : \(x\ge-3\)\(pt\Leftrightarrow x^2-2x+1=x+3-4\sqrt{x+3}+4\Leftrightarrow\left(x-1\right)^2=\left(\sqrt{x+3}-2\right)^2\Leftrightarrow x-1=\sqrt{x+3}-2\Leftrightarrow x+1=\sqrt{x+3}\Leftrightarrow\left(x+1\right)^2=x+3\left(x\ge-1\right)\Leftrightarrow x^2+2x+1=x+3\Leftrightarrow x^2+x-2=0\Leftrightarrow\left[{}\begin{matrix}x=1\left(tmdk\right)\\x=-2\left(kotm\right)\end{matrix}\right.\)

a) Phương trình bậc hai  4 x 2   +   4 x   +   1   =   0

Có a = 4; b’ = 2; c = 1;  Δ ’   =   ( b ’ ) 2   –   a c   =   2 2   –   4 . 1   =   0

Phương trình có nghiệm kép là:

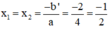
b) Phương trình  13852 x 2   –   14 x   +   1   =   0

Có a = 13852; b’ = -7; c = 1;

Δ ’   =   ( b ’ ) 2   –   a c   =   ( - 7 ) 2   –   13852 . 1   =   - 13803   <   0

Vậy phương trình vô nghiệm.

c) Phương trình bậc hai  5 x 2   –   6 x   +   1   =   0

Có: a = 5; b’ = -3; c = 1.;  Δ ’   =   ( b ’ ) 2   –   a c   =   ( - 3 ) 2   –   5 . 1   =   4   >   0

Phương trình có hai nghiệm phân biệt:

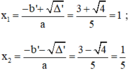
d) Phương trình bậc hai: 

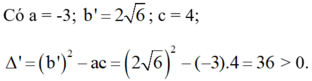
Phương trình có hai nghiệm phân biệt :

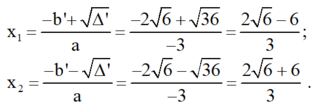
Kiến thức áp dụng

Phương trình ax2 + bx + c = 0 (a ≠ 0) có biệt thức Δ = b2 – 4ac.

+ Nếu Δ > 0, phương trình có hai nghiệm phân biệt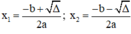 

+ Nếu Δ = 0, phương trình có nghiệm kép  ;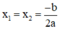

+ Nếu Δ < 0, phương trình vô nghiệm.

a: ĐKXĐ: \(x\ge1\)

b: ĐKXĐ: \(x< 0\)

c: ĐKXĐ: \(\left[{}\begin{matrix}x\ge11\\x\le3\end{matrix}\right.\)

1) ĐKXĐ: \(\left\{{}\begin{matrix}2x+11\ge0\\x-1\ge0\end{matrix}\right.\)\(\Leftrightarrow x\ge1\)

2) ĐKXĐ: \(\left\{{}\begin{matrix}-5x\ge0\\x\ne0\end{matrix}\right.\)\(\Leftrightarrow x< 0\)

3) ĐKXĐ: \(7x^2+1\ge0\left(đúng\forall x\right)\Leftrightarrow x\in R\)

4) ĐKXĐ: \(x^2-14x+33\ge0\Leftrightarrow\left(x-11\right)\left(x-3\right)\ge0\)

\(\Leftrightarrow\left[{}\begin{matrix}\left\{{}\begin{matrix}x-11\ge0\\x-3\ge0\end{matrix}\right.\\\left\{{}\begin{matrix}x-11\le0\\x-3\le0\end{matrix}\right.\end{matrix}\right.\)\(\Leftrightarrow\left[{}\begin{matrix}x\ge11\\x\le3\end{matrix}\right.\)

5) ĐKXĐ: 

+) \(-x^2+6x+16\ge0\)

\(\Leftrightarrow-\left(x^2-6x+9\right)+25\ge0\)

\(\Leftrightarrow\left(x-3\right)^2\le25\Leftrightarrow-5\le x-3\le5\)

\(\Leftrightarrow-2\le x\le8\)

+) \(3x^2\ne0\Leftrightarrow x\ne0\)

\(\Rightarrow\left\{{}\begin{matrix}-2\le x\le8\\x\ne0\end{matrix}\right.\)

a/

Đặt \(\left\{{}\begin{matrix}\sqrt[3]{x-1}=a\\\sqrt[3]{27-14x}=b\end{matrix}\right.\) ta được hệ:

\(\left\{{}\begin{matrix}2a+b=1\\14a^3+b^3=13\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}b=1-2a\\14a^3+b^3=13\end{matrix}\right.\)

\(\Rightarrow14a^3+\left(1-2a\right)^3=13\)

\(\Leftrightarrow a^3+2a^2-a-2=0\)

\(\Leftrightarrow\left(a-1\right)\left(a+1\right)\left(a+2\right)=0\)

\(\Leftrightarrow\left[{}\begin{matrix}x-1=1\\x-1=-1\\x-1=-8\end{matrix}\right.\) \(\Leftrightarrow...\)

b/ ĐKXĐ: ...

\(VT=\sqrt{x-2}+\sqrt{4-x}\le\sqrt{2\left(x-2+4-x\right)}=2\)

\(VP=\left(x-3\right)^2+2\ge2\)

Đẳng thức xảy ra khi và chỉ khi \(x=3\)

Bài 2:

a) \(3x^2-7x-10=\left(x+1\right)\left(3x-10\right)\)

b) \(x^2+6x+9-4y^2=\left(x+3\right)^2-\left(2y\right)^2=\left(x+3-2y\right)\left(x+3+2y\right)\)

c) \(x^2-2xy+y^2-5x+5y=\left(x-y\right)^2-5\left(x-y\right)=\left(x-y\right)\left(x-y-5\right)\)

d) \(4x^2-y^2-6x+3y=\left(2x-y\right)\left(2x+y\right)-3\left(2x-y\right)=\left(2x-y\right)\left(2x+y-3\right)\)

e) \(1-2a+2bc+a^2-b^2-c^2=\left(a-1\right)^2-\left(b-c\right)^2=\left(a-1-b+c\right)\left(a-1+b-c\right)\)

f) \(x^3-3x^2-4x+12=\left(x+2\right)\left(x-3\right)\left(x-2\right)\)

g) \(x^4+64=\left(x^2+8\right)^2-16x^2=\left(x^2+8-4x\right)\left(x^2+6+4x\right)\)h) \(x^4-5x^2+4=\left(x+2\right)\left(x+1\right)\left(x-1\right)\left(x-2\right)\)

i) \(\left(x+1\right)\left(x+3\right)\left(x+5\right)\left(x+7\right)+16=\left(x^2+8x+7\right)\left(x^2+8x+15\right)+16=\left(x^2+8x+7\right)^2+8\left(x^2+8x+7\right)+16=\left(x^2+8x+11\right)^2\)

a: \(3x^2-7x-10\)

\(=3x^2+3x-10x-10\)

\(=\left(x+1\right)\left(3x-10\right)\)

b: \(x^2+6x+9-4y^2\)

\(=\left(x+3\right)^2-4y^2\)

\(=\left(x+3-2y\right)\left(x+3+2y\right)\)

c: \(x^2-2xy+y^2-5x+5y\)

\(=\left(x-y\right)^2-5\left(x-y\right)\)

\(=\left(x-y\right)\left(x-y-5\right)\)

a) 

b) 

c) 

d) 

e) 

f) 

g) h) 

i) 

\(M=343-8x^3-64+8x^3=279\\ N=8x^3-1-1+8x^3=16x^3=16\cdot1000=16000\)

\(M=343-8x^3-\left(64-8x^3\right)=343-64=279\)

(biểu thức này ko phụ thuộc vào biến x nên ko cần thay)

\(N=8x^3-1-1+8x^3=16x^3\)

Thay \(x=10\)

\(N=16\cdot10^3=16\cdot1000=16000\)