cho B = \(\dfrac{5}{\sqrt{x-1}}\) , tìm x thuộc Z để B có giá trị nguyên

Những câu hỏi liên quan
Cho \(B=\dfrac{5}{\sqrt{x}-1}\) Tìm x thuộc Z để B có giá trị nguyên.
Để B có nghĩa thì x ≥ 0 và x ≠ 1
\(B=\dfrac{5}{\sqrt{x}-1}\) nguyên khi \(\sqrt{x}-1\) thuộc ước của 5
⇒ \(\sqrt{x}-1\) ∈ \(\left\{1,-1,5,-5\right\}\)
\(TH1:\sqrt{x}-1=1\Rightarrow x=4\)
\(TH2:\sqrt{x}-1=-1\Rightarrow x=0\)
\(TH3:\sqrt{x}-1=5\Rightarrow x=36\)
\(TH4:\sqrt{x}-1=-5\Rightarrow x=-4\) (loại vì x ≥ 0)
Vậy \(x\in\left\{0,4,36\right\}\)
Đúng 3
Bình luận (0)
\(ĐK:x\ge0;x\ne1\\ B\in Z\Leftrightarrow\sqrt{x}-1\inƯ\left(5\right)=\left\{-1;1;5\right\}\left(\sqrt{x}-1\ge-1\right)\\ \Leftrightarrow\sqrt{x}\in\left\{0;2;6\right\}\\ \Leftrightarrow x\in\left\{0;4;36\right\}\left(tm\right)\)
Đúng 3
Bình luận (0)
tìm \(x\) thuộc \(Z\) để
\(F=\dfrac{5}{\sqrt{x}+1}\) có giá trị nguyên
Để \(F=\dfrac{5}{\sqrt{x}+1}\) có giá trị nguyên thì \(5⋮\left(\sqrt{x}+1\right)\)
Suy ra \(\left(\sqrt{x}+1\right)\inƯ\left(5\right)\) hay \(\left(\sqrt{x}+1\right)\in\left\{1;-1;5;-5\right\}\)
Ta có bảng:
| \(\sqrt{x}+1\) | 1 | -1 | 5 | -5 |
| x | 0 | không có | 2 | không có |
Vậy để \(F=\dfrac{5}{\sqrt{x}+1}\) có giá trị nguyên thì \(x\in\left\{0;2\right\}\)
Đúng 1
Bình luận (0)
Cho hai bt A=\(\dfrac{\sqrt{x}+2}{\sqrt{x}-2}\)và B=\(\left(\dfrac{\sqrt{x}}{x-4}+\dfrac{1}{\sqrt{x}-2}\right):\dfrac{\sqrt{x}+2}{x-4}\)
a) rút gọn B
b)tìm x thuộc Z để C= A(B-2) có giá trị nguyên
a) \(B=\left(\dfrac{\sqrt{x}}{x-4}+\dfrac{1}{\sqrt{x}-2}\right):\dfrac{\sqrt{x}+2}{x-4}\left(đk:x\ge0,x\ne4\right)\)
\(=\dfrac{\sqrt{x}+\sqrt{x}+2}{x-4}.\dfrac{x-4}{\sqrt{x}+2}=\dfrac{2\sqrt{x}+2}{\sqrt{x}+2}\)
c) \(C=A\left(B-2\right)=\dfrac{\sqrt{x}+2}{\sqrt{x}-2}\left(\dfrac{2\sqrt{x}+2}{\sqrt{x}+2}-2\right)\)
\(=\dfrac{\sqrt{x}+2}{\sqrt{x}-2}.\dfrac{-2}{\sqrt{x}+2}=\dfrac{-2}{\sqrt{x}-2}\in Z\)
\(\Rightarrow\sqrt{x}-2\inƯ\left(2\right)=\left\{1;-1;2-2\right\}\)
\(\Rightarrow\sqrt{x}\in\left\{3;1;4;0\right\}\)
\(\Rightarrow x\in\left\{0;1;9;16\right\}\)
Đúng 1
Bình luận (0)
Cho \(B=\dfrac{2}{\sqrt{x}+3}+\dfrac{2\sqrt{x}}{x-4\sqrt{x}+3}+\dfrac{\sqrt{x}}{\sqrt{x}-1}\)
Tìm \(x\in Z\) để B có giá trị nguyên
\(B=\dfrac{2}{\sqrt{x}-3}+\dfrac{2\sqrt{x}}{x-4\sqrt{x}+3}+\dfrac{\sqrt{x}}{\sqrt{x}-1}\)
\(=\dfrac{2\sqrt{x}-2+2\sqrt{x}+x-3\sqrt{x}}{\left(\sqrt{x}-1\right)\left(\sqrt{x}-3\right)}\)
\(=\dfrac{\sqrt{x}+2}{\sqrt{x}-3}\)
Để B nguyên thì \(\sqrt{x}-3\in\left\{1;-1;5\right\}\)
\(\Leftrightarrow\sqrt{x}\in\left\{4;2;8\right\}\)
hay \(x\in\left\{16;4;64\right\}\)
Đúng 0
Bình luận (0)
tìm \(x\) thuộc \(Z\) để :
\(A=\dfrac{9}{\sqrt{x}-5}\) có giá trị nguyên
Bài 13 : Cho A =\(\dfrac{\sqrt{x}+1}{\sqrt{x}-3}\). Tìm x thuộc Z để A có giá trị là một số nguyên
Để A có giá trị là một số nguyên thì:
\(\left(\sqrt{x}+1\right)⋮\left(\sqrt{x}-3\right)\)
\(\Leftrightarrow\left(\sqrt{x}-3\right)+4⋮\left(\sqrt{x}-3\right)\)
\(\Leftrightarrow4⋮\left(\sqrt{x}-3\right)\)
Vì \(x\in Z\) nên \(\left(\sqrt{x}-3\right)\inƯ\left(4\right)=\left\{\pm1,\pm2,\pm4\right\}\)
Ta có bảng sau:
| \(\sqrt{x}-3\) | 1 | -1 | 2 | -2 | 4 | -4 |
| \(\sqrt{x}\) | 4 | 2 | 5 | 1 | 7 | -1 |
| x | 16 | 4 | 25 | 1 | 49 | (loại) |
Vậy ....
Đúng 0
Bình luận (0)
Ta có: \(A=\dfrac{\sqrt{x}+1}{\sqrt{x}-3}=\dfrac{\left(\sqrt{x}-3\right)+4}{\sqrt{x}-3}=\dfrac{\sqrt{x}-3}{\sqrt{x}-3}=\dfrac{4}{\sqrt{x}-3}=1+\dfrac{4}{\sqrt{x}-3}\)
Để A có giá trị là một số nguyên khi:
\(4⋮\sqrt{x}-3\) hay \(\sqrt{x}-3\inƯ\left(4\right)=\left\{\pm1;\pm2;\pm4\right\}\)
Do đó:
\(\sqrt{x}-3=-1\Rightarrow\sqrt{x}=-1+3=2\Rightarrow x=4\)
\(\sqrt{x}-3=1\Rightarrow\sqrt{x}=1+3=4\Rightarrow x=16\)
\(\sqrt{x}-3=-2\Rightarrow\sqrt{x}=-2+3=1\Rightarrow x=1\)
\(\sqrt{x}-3=2\Rightarrow\sqrt{x}=2+3=5\Rightarrow x=25\)
\(\sqrt{x}-3=-4\Rightarrow\sqrt{x}=-4+3=-1\) ( loại )
\(\sqrt{x}-3=4\Rightarrow\sqrt{x}=4+3=7\Rightarrow x=49\)
Vậy để A là một số nguyên khi \(x\in\left\{4;16;1;25;49\right\}\)
Đúng 0
Bình luận (0)
Cho B = \(\frac{5}{\sqrt{x}-1}\). Tìm x thuộc Z để B có giá trị nguyên
\(B=\frac{5}{\sqrt{x}-1}\)
Để B nguyên thì: \(\sqrt{x}-1\inƯ\left(5\right)\)
Mà: Ư(5)={-1;1;-5;-5}
=> \(\sqrt{x}-1\in\left\{1;-1;5-;5\right\}\)
Ta có bảng sau:
| \(\sqrt{x}-1\) | 1 | -1 | 5 | -5 |
| x | 4 | 0 | 36 | loại |
Vậy x={0;4;16}
Đúng 0
Bình luận (0)
Tìm x thuộc Z để biểu thức có giá trị nguyên: A=\(\dfrac{2\sqrt{x}+3}{3\sqrt{x}-1}\)
Để A nguyên thì \(2\sqrt{x}+3⋮3\sqrt{x}-1\)
\(\Leftrightarrow6\sqrt{x}+9⋮3\sqrt{x}-1\)
\(\Leftrightarrow3\sqrt{x}-1\in\left\{-1;1;11\right\}\)
\(\Leftrightarrow3\sqrt{x}\in\left\{0;12\right\}\)
hay \(x\in\left\{0;16\right\}\)
Đúng 0
Bình luận (2)
a, cho A dfrac{sqrt{x+1}}{sqrt{x-3}}. tìm x để A có giá trị nguyên ( x ϵ Z)b, Thực hiện phép tính: {[(2sqrt{2})^2 : 2,4] x [5,25 : (sqrt{7})^2]} : {[2dfrac{1}{7} : dfrac{left(sqrt{5}right)^2}{7}] : [2^2 : dfrac{left(2sqrt{2}right)^2}{sqrt{81}}]}
Đọc tiếp
a, cho A = \(\dfrac{\sqrt{x+1}}{\sqrt{x-3}}\). tìm x để A có giá trị nguyên ( x ϵ Z)
b, Thực hiện phép tính: {[(2\(\sqrt{2}\))\(^2\) : 2,4] x [5,25 : (\(\sqrt{7}\))\(^2\)]} : {[2\(\dfrac{1}{7}\) : \(\dfrac{\left(\sqrt{5}\right)^2}{7}\)] : [2\(^2\) : \(\dfrac{\left(2\sqrt{2}\right)^2}{\sqrt{81}}\)]}
a: Sửa đề: \(A=\dfrac{\sqrt{x}+1}{\sqrt{x}-3}\)
ĐKXĐ: \(\left\{{}\begin{matrix}x>=0\\x\ne9\end{matrix}\right.\)
Để A là số nguyên thì \(\sqrt{x}+1⋮\sqrt{x}-3\)
=>\(\sqrt{x}-3+4⋮\sqrt{x}-3\)
=>\(4⋮\sqrt{x}-3\)
=>\(\sqrt{x}-3\in\left\{1;-1;2;-2;4;-4\right\}\)
=>\(\sqrt{x}\in\left\{4;2;5;1;7;-1\right\}\)
=>\(\sqrt{x}\in\left\{4;2;5;1;7\right\}\)
=>\(x\in\left\{16;4;25;1;49\right\}\)
b: 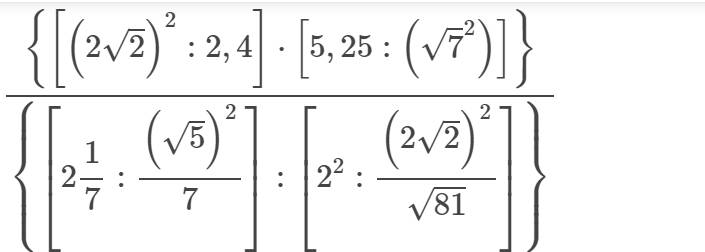
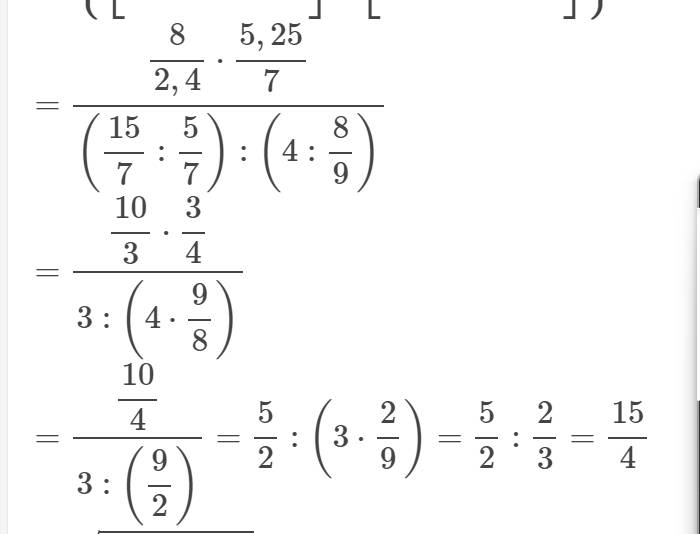
Đúng 0
Bình luận (0)
bai 1
A= \(\frac{\sqrt{x}-3}{2}\) . tìm x thuộc Z và x<30 để A có giá trị nguyên
bài 2
B = \(\frac{5}{\sqrt{x}-1}\)tìm x thuộc Z để B có gía trị nguyên

























