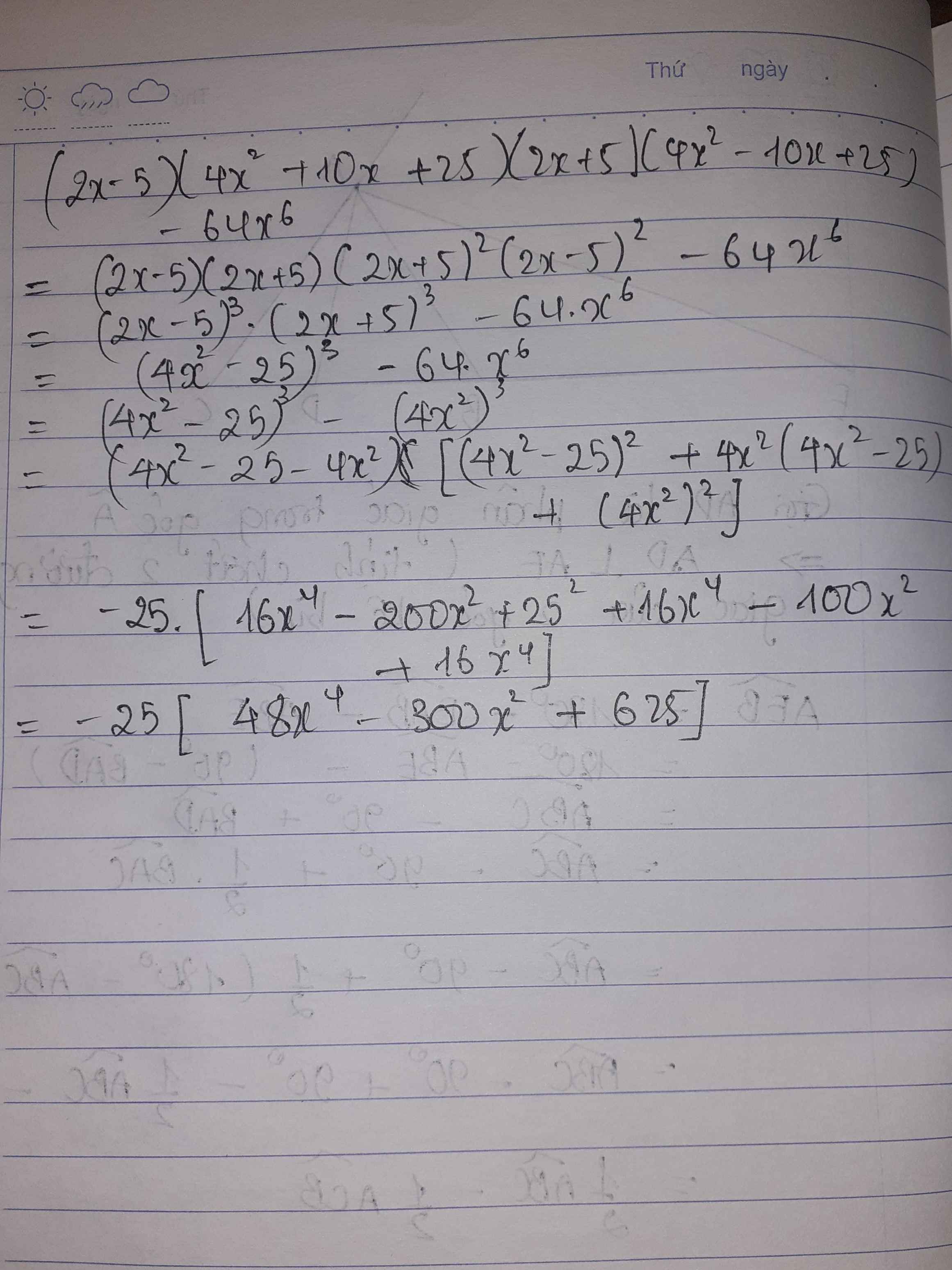`#3107.101107`

a)

`x^2 + 6x + 10`

`= (x^2 + 2*x*3 + 3^2) + 1`

`= (x + 3)^2 + 1`

Vì `(x + 3)^2 \ge 0` `AA` `x`

`=> (x + 3)^2 + 1 \ge 1` `AA` `x`

Vậy, GTNN của bt là 1 khi `(x + 3)^2 = 0`

`<=> x + 3 = 0`

`<=> x = -3`

b)

`4x^2 - 4x + 5`

`= [(2x)^2 - 2*2x*1 + 1^2] + 4`

`= (2x - 1)^2 + 4`

Vì `(2x - 1)^2 \ge 0` `AA` `x`

`=> (2x - 1)^2 + 4 \ge 4` `AA` `x`

Vậy, GTNN của bt là `4` khi `(2x - 1)^2 = 0`

`<=> 2x - 1 = 0`

`<=> 2x = 1`

`<=> x = 1/2`

c)

`x^2 - 3x + 1`

`= (x^2 - 2*x*3/2 + 9/4) - 5/4`

`= (x - 3/2)^2 - 5/4`

Vì `(x - 3/2)^2 \ge 0` `AA` `x`

`=> (x - 3/2)^2 - 5/4 \ge -5/4` `AA` `x`

Vậy, GTNN của bt là `-5/4` khi `(x - 3/2)^2 = 0`

`<=> x - 3/2 = 0`

`<=> x = 3/2`

\(a,\Leftrightarrow x\left(2x-7\right)+2\left(2x-7\right)=0\\ \Leftrightarrow\left(x+2\right)\left(2x-7\right)=0\Leftrightarrow\left[{}\begin{matrix}x=-2\\x=\dfrac{7}{2}\end{matrix}\right.\\ b,\Leftrightarrow x\left(x^2-9\right)=0\\ \Leftrightarrow x\left(x-3\right)\left(x+3\right)=0\Leftrightarrow\left[{}\begin{matrix}x=0\\x=3\\x=-3\end{matrix}\right.\\ c,\Leftrightarrow\left(2x-1\right)\left(2x+1\right)-2\left(2x-1\right)^2=0\\ \Leftrightarrow\left(2x-1\right)\left(2x+1-4x+2\right)=0\\ \Leftrightarrow\left(2x-1\right)\left(-2x+3\right)=0\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{1}{2}\\x=\dfrac{3}{2}\end{matrix}\right.\\ d,\Leftrightarrow x^2\left(x-1\right)-4\left(x-1\right)^2=0\\ \Leftrightarrow\left(x-1\right)\left(x^2-4x+4\right)=0\\ \Leftrightarrow\left(x-1\right)\left(x-2\right)^2=0\Leftrightarrow\left[{}\begin{matrix}x=1\\x=2\end{matrix}\right.\)

a) \(4x^2+12x+1=\left(4x^2+12x+9\right)-8=\left(2x+3\right)^2-8\ge-8\)

\(ĐTXR\Leftrightarrow x=-\dfrac{3}{2}\)

b) \(4x^2-3x+10=\left(4x^2-3x+\dfrac{9}{16}\right)+\dfrac{151}{16}=\left(2x-\dfrac{3}{4}\right)^2+\dfrac{151}{16}\ge\dfrac{151}{16}\)

\(ĐTXR\Leftrightarrow x=\dfrac{3}{8}\)

c) \(2x^2+5x+10=\left(2x^2+5x+\dfrac{25}{8}\right)+\dfrac{55}{8}=\left(\sqrt{2}x+\dfrac{5\sqrt{2}}{4}\right)^2+\dfrac{55}{8}\ge\dfrac{55}{8}\)

\(ĐTXR\Leftrightarrow x=-\dfrac{5}{4}\)

d) \(x-x^2+2=-\left(x^2-x+\dfrac{1}{4}\right)+\dfrac{9}{4}=-\left(x-\dfrac{1}{2}\right)^2+\dfrac{9}{4}\le\dfrac{9}{4}\)

\(ĐTXR\Leftrightarrow x=\dfrac{1}{2}\)

e) \(2x-2x^2=-2\left(x^2-x+\dfrac{1}{4}\right)+\dfrac{1}{2}=-2\left(x-\dfrac{1}{2}\right)^2+\dfrac{1}{2}\le\dfrac{1}{2}\)

\(ĐTXR\Leftrightarrow x=\dfrac{1}{2}\)

f) \(4x^2+2y^2+4xy+4y+5=\left(4x^2+4xy+y^2\right)+\left(y^2+4y+4\right)+1=\left(2x+y\right)^2+\left(y+2\right)^2+1\ge1\)

\(ĐTXR\Leftrightarrow\) \(\left\{{}\begin{matrix}x=1\\y=-2\end{matrix}\right.\)

a: Ta có: \(4x^2+12x+1\)

\(=4x^2+12x+9-8\)

\(=\left(2x+3\right)^2-8\ge-8\forall x\)

Dấu '=' xảy ra khi \(x=-\dfrac{3}{2}\)

b: Ta có: \(4x^2-3x+10\)

\(=4\left(x^2-\dfrac{3}{4}x+\dfrac{5}{2}\right)\)

\(=4\left(x^2-2\cdot x\cdot\dfrac{3}{8}+\dfrac{9}{64}+\dfrac{151}{64}\right)\)

\(=4\left(x-\dfrac{3}{8}\right)^2+\dfrac{151}{16}\ge\dfrac{151}{16}\forall x\)

Dấu '=' xảy ra khi \(x=\dfrac{3}{8}\)

c: Ta có: \(2x^2+5x+10\)

\(=2\left(x^2+\dfrac{5}{2}x+5\right)\)

\(=2\left(x^2+2\cdot x\cdot\dfrac{5}{4}+\dfrac{25}{16}+\dfrac{55}{16}\right)\)

\(=2\left(x+\dfrac{5}{4}\right)^2+\dfrac{55}{8}\ge\dfrac{55}{8}\forall x\)

Dấu '=' xảy ra khi \(x=-\dfrac{5}{4}\)

a) \(\Leftrightarrow4x^2-10x-4x^2-3x=19\\ \Leftrightarrow-13x=19\\ \Leftrightarrow x=-\dfrac{19}{13}\)

b) \(\Leftrightarrow\left(x-7\right)\left(3x+5\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x=7\\x=-\dfrac{5}{3}\end{matrix}\right.\)

Ta có: \(\left(2x-5\right)\left(4x^2+10x+25\right)\left(2x+5\right)\left(4x^2-10x+25\right)-64x^6\)

\(=\left(8x^3-125\right)\left(8x^3+125\right)-64x^6\)

\(=64x^6-15625-64x^6\)

=-15625

a. (2x + 1)² - 4x² + 2x² - 2 = 0

<=> (2x + 1 - 2x)(2x + 1 + 2x) + 2(x² - 1) = 0

<=> (4x + 1) + 2x² - 2 = 0

<=> 4x + 1 + 2x² - 2 = 0

<=> 2x² + 4x - 2 + 1 = 0

<=> 2x² + 4x - 1 = 0

<=> 2x² + 4x = 1

<=> 2x(x + 2) = 1

Vì 1 chỉ có tích là 1 . 1 nên:

<=> \(\left[{}\begin{matrix}2x=1\\x+2=1\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{1}{2}\\x=-1\end{matrix}\right.\)

\(a,\Leftrightarrow4x^2+4x+1-4x^2+2x^2-2=0\\ \Leftrightarrow2x^2+4x-1=0\\ \Leftrightarrow2\left(x^2+2x+1\right)-3=0\\ \Leftrightarrow2\left(x+1\right)^2-3=0\\ \Leftrightarrow\left(x+1\right)^2=\dfrac{3}{2}\\ \Leftrightarrow\left[{}\begin{matrix}x+1=\sqrt{\dfrac{3}{2}}\\x+1=-\sqrt{\dfrac{3}{2}}\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{-2-\sqrt{6}}{2}\\x=\dfrac{-2+\sqrt{6}}{2}\end{matrix}\right.\)

\(b,\left(x-2\right)\left(x+2\right)-\left(x+3\right)^2-2x-5=0\\ \Leftrightarrow x^2-4-x^2-6x-9-2x-5=0\\ \Leftrightarrow-8x=18\\ \Leftrightarrow x=-\dfrac{9}{4}\)

Lời giải:

$4x^2-2x-1=0$

$\Leftrightarrow [(2x)^2-2.2x.\frac{1}{2}+(\frac{1}{2})^2]-\frac{5}{4}=0$

$\Leftrightarrow (2x-\frac{1}{2})^2=\frac{5}{4}$

$\Rightarrow 2x-\frac{1}{2}=\pm \frac{\sqrt{5}}{2}$

$\Leftrightarrow 2x=\frac{1\pm \sqrt{5}}{2}$

$\Rightarrow x=\frac{1\pm \sqrt{5}}{4}$

$x^4-4x^2-32=0$

$\Leftrightarrow (x^2-2)^2-36=0$

$\Leftrightarrow (x^2-2-6)(x^2-2+6)=0$
$\Leftrightarrow (x^2-8)(x^2+4)=0$

Vì $x^2+4>0$ với mọi $x$ nên $x^2-8=0$

$\Leftrightarrow x=\pm 2\sqrt{2}$

a) Ta có: \(4x^2-2x-1=0\)

\(\Delta=\left(-2\right)^2-4\cdot4\cdot\left(-1\right)=4+16=20\)

Vì \(\Delta>0\) nên phương trình có hai nghiệm phân biệt là:

\(\left\{{}\begin{matrix}x_1=\dfrac{2-2\sqrt{5}}{8}=\dfrac{1-\sqrt{5}}{4}\\x_2=\dfrac{2+2\sqrt{5}}{8}=\dfrac{1+\sqrt{5}}{4}\end{matrix}\right.\)

b) Ta có: \(x^4-4x^2-32=0\)

\(\Leftrightarrow x^4-8x^2+4x^2-32=0\)

\(\Leftrightarrow x^2=8\)
hay \(x\in\left\{2\sqrt{2};-2\sqrt{2}\right\}\)

\(a,\left(3x-7\right)^2=\left(2-2x\right)^2\)

a,\(=>\left(3x-7\right)^2-\left(2-2x\right)^2=0\)

\(< =>\left(3x-7+2-2x\right)\left(3x-7-2+2x\right)=0\)

\(< =>\left(x-5\right)\left(5x-9\right)=0=>\left[{}\begin{matrix}x=5\\x=1,8\end{matrix}\right.\)

b, \(x^2-8x+6=0< =>x^2-2.4x+16-10=0\)

\(< =>\left(x-4\right)^2-\sqrt{10}^2=0\)

\(=>\left(x-4+\sqrt{10}\right)\left(x-4-\sqrt{10}\right)=0\)

\(=>\left[{}\begin{matrix}x=4-\sqrt{10}\\x=4+\sqrt{10}\end{matrix}\right.\)

c, \(4x^2-2x-1=0\)

\(< =>\left(2x\right)^2-2.2.\dfrac{1}{2}x+\dfrac{1}{4}-\dfrac{5}{4}=0\)

\(=>\left(2x-\dfrac{1}{2}\right)^2-\left(\dfrac{\sqrt{5}}{2}\right)^2=0\)

\(=>\left(2x+\dfrac{-1+\sqrt{5}}{2}\right)\left(2x-\dfrac{1+\sqrt{5}}{2}\right)=0\)

\(=>\left[{}\begin{matrix}x=\dfrac{1-\sqrt{5}}{4}\\x=\dfrac{1+\sqrt{5}}{4}\end{matrix}\right.\)

d,\(x^4-4x^2-32=0\)

đặt \(t=x^2\left(t\ge0\right)=>t^2-4t-32=0\)

\(< =>t^2-2.2t+4-6^2=0\)

\(=>\left(t-2\right)^2-6^2=0=>\left(t-8\right)\left(t+4\right)=0\)

\(=>\left[{}\begin{matrix}t=8\left(tm\right)\\t=-4\left(loai\right)\end{matrix}\right.\)\(=>x=\pm\sqrt{8}\)