quá đúng

1234567890-01234567890-=qưertyuiop[]\';;lkjhfgdsazxcvbnm,./\'l;[]7894561230.+-

a) A=\cos x-\cos x+\sin ^{2} x \cdot \dfrac{1}{\cos ^{2} x}-\tan ^{2} x=0A=cosx−cosx+sin2x⋅cos2x1​−tan2x=0

b) B=\dfrac{1}{\sin x} \cdot \sqrt{\dfrac{1-\cos x+1+\cos x}{(1-\cos x)(1+\cos x)}}-\sqrt{2}B=sinx1​⋅(1−cosx)(1+cosx)1−cosx+1+cosx​​−2​

\begin{aligned}&=\dfrac{1}{\sin x} \cdot \sqrt{\dfrac{2}{1-\cos ^{2} x}}-\sqrt{2}=\dfrac{1}{\sin x} \cdot \sqrt{\dfrac{2}{\sin ^{2} x}}-\sqrt{2} \\&=\sqrt{2}\left(\dfrac{1}{\sin ^{2} x}-1\right)=\sqrt{2} \cot ^{2} x\end{aligned}​=sinx1​⋅1−cos2x2​​−2​=sinx1​⋅sin2x2​​−2​=2​(sin2x1​−1)=2​cot2x​

\(B=\dfrac{1-4\sin^2x\cdot\cos^2x}{\sin^2x+2\sin x\cdot\cos x+\cos^2}+2\sin x\cdot\cos x\\ B=\dfrac{1-4\sin^2x\cdot\cos^2x}{2\sin x\cdot\cos x}+2\sin x\cdot\cos x\\ B=\dfrac{1-4\sin^2x\cdot\cos^2x+4\sin^2x\cdot\cos^2x}{2\sin x\cdot\cos x}=\dfrac{1}{2\sin x\cdot\cos x}\)

a:\(a\cdot sin0+b\cdot cos0+c\cdot sin90\)

\(=a\cdot0+b\cdot1+c\cdot1\)

=b+c

b: \(a\cdot cos90+b\cdot sin90+c\cdot sin180\)

\(=a\cdot0+b\cdot1+c\cdot0\)

=b

c: \(a^2\cdot sin90+b^2\cdot cos90+c^2\cdot cos180\)

\(=a^2\cdot1+b^2\cdot0+c^2\left(-1\right)\)

\(=a^2-c^2\)

????

\(=cosa\cdot sina-1-1+sina\cdot cosa+2\)

\(=2\cdot sina\cdot cosa=sin2a\)

\(\Leftrightarrow3sin^2x-2sinx.cosx-5cos^2x=0\)

Nhận thấy \(cosx=0\) ko phải nghiệm, chia 2 vế cho \(cos^2x\)

\(3tan^2x-2tanx-5=0\)

\(\Leftrightarrow\left[{}\begin{matrix}tanx=-1\\tanx=\frac{5}{3}\end{matrix}\right.\)

\(\Rightarrow\left[{}\begin{matrix}x=-45^0+k180^0\\x=arctan\left(\frac{5}{3}\right)+k180^0\end{matrix}\right.\)

1.

\(\Leftrightarrow2sinx.cosx+2cosx=0\)

\(\Leftrightarrow2cosx\left(sinx+1\right)=0\)

\(\Leftrightarrow\left[{}\begin{matrix}cosx=0\\sinx=-1\end{matrix}\right.\)

\(\Leftrightarrow cosx=0\) (do \(cosx=0\Leftrightarrow sinx=\pm1\) bao hàm luôn cả pt \(sinx=-1\))

\(\Leftrightarrow x=\dfrac{\pi}{2}+k\pi\)

2.

\(\Leftrightarrow\left[{}\begin{matrix}2x-10^0=60^0+k360^0\\2x-10^0=120^0+n360^0\end{matrix}\right.\)

\(\Leftrightarrow\left[{}\begin{matrix}x=35^0+k180^0\\x=65^0+n180^0\end{matrix}\right.\)

Do \(-120^0< x< 90^0\Rightarrow\left\{{}\begin{matrix}-120^0< 35^0+k180^0< 90^0\\-120^0< 65^0+n180^0< 90^0\end{matrix}\right.\)

\(\Rightarrow\left\{{}\begin{matrix}k=0\\n=\left\{-1;0\right\}\end{matrix}\right.\)

\(\Rightarrow\left[{}\begin{matrix}x=35^0\\x=-115^0\\x=65^0\end{matrix}\right.\)

3. Làm tương tự câu 2

4.

\(\Leftrightarrow\dfrac{1}{2}-\dfrac{1}{2}cos\left(10x+\dfrac{4\pi}{5}\right)-\left(\dfrac{1}{2}+\dfrac{1}{2}cos\left(\dfrac{x}{2}-2\pi\right)\right)=0\)

\(\Leftrightarrow cos\left(10x+\dfrac{4\pi}{5}\right)+cos\left(\dfrac{x}{2}-2\pi\right)=0\)

\(\Leftrightarrow cos\left(10x+\dfrac{4\pi}{5}\right)+cos\left(\dfrac{x}{2}\right)=0\)

\(\Leftrightarrow cos\left(10x+\dfrac{4\pi}{5}\right)=-cos\left(\dfrac{x}{2}\right)=cos\left(\pi-\dfrac{x}{2}\right)\)

\(\Leftrightarrow\left[{}\begin{matrix}10x+\dfrac{4\pi}{5}=\pi-\dfrac{x}{2}+k2\pi\\10x+\dfrac{4\pi}{5}=\dfrac{x}{2}-\pi+k2\pi\end{matrix}\right.\)

\(\Leftrightarrow...\)

a: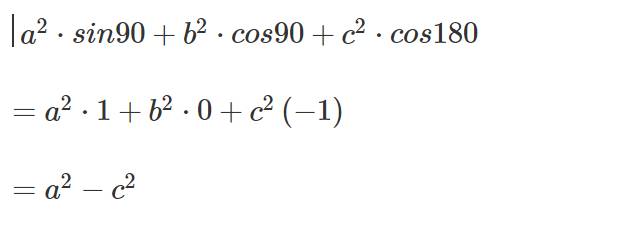 

b: \(B=3-sin^290^0+2\cdot cos^260^0-3\cdot tan^245^0\)

\(=3-1+2\cdot\left(\dfrac{1}{2}\right)^2-3\cdot1^2\)

\(=2-3+2\cdot\dfrac{1}{4}=-1+\dfrac{1}{2}=-\dfrac{1}{2}\)

c: \(C=sin^245^0-2\cdot sin^250^0+3\cdot cos^245^0-2\cdot sin^240^0+4\cdot tan55\cdot tan35\)

\(=\left(\dfrac{\sqrt{2}}{2}\right)^2+3\cdot\left(\dfrac{\sqrt{2}}{2}\right)^2-2\cdot\left(sin^250^0+sin^240^0\right)+4\)

\(=\dfrac{1}{2}+3\cdot\dfrac{1}{2}-2+4\)

\(=2-2+4=4\)

= cos \(_{^{ }\beta}\).cos\(\beta\).(-cot\(\beta\)) vậy dấu của A là dấu trừ