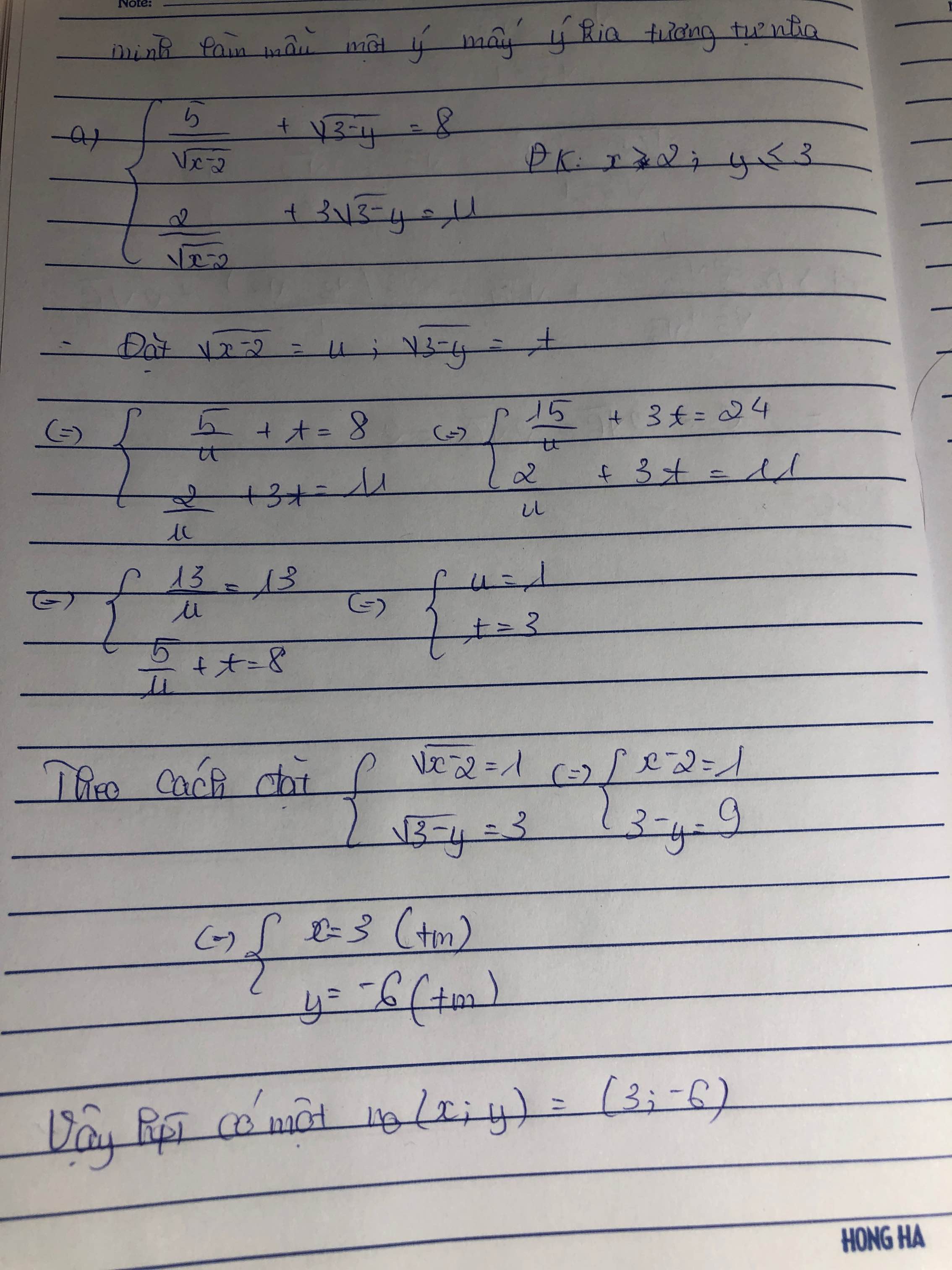Bài 1: ĐKXĐ: $2\leq x\leq 4$
PT $\Leftrightarrow (\sqrt{x-2}+\sqrt{4-x})^2=2$

$\Leftrightarrow 2+2\sqrt{(x-2)(4-x)}=2$
$\Leftrightarrow (x-2)(4-x)=0$

$\Leftrightarrow x-2=0$ hoặc $4-x=0$

$\Leftrightarrow x=2$ hoặc $x=4$ (tm)

Bài 2:
PT $\Leftrightarrow 4x^3(x-1)-3x^2(x-1)+6x(x-1)-4(x-1)=0$

$\Leftrightarrow (x-1)(4x^3-3x^2+6x-4)=0$
$\Leftrightarrow x=1$ hoặc $4x^3-3x^2+6x-4=0$

Với $4x^3-3x^2+6x-4=0(*)$

Đặt $x=t+\frac{1}{4}$ thì pt $(*)$ trở thành:
$4t^3+\frac{21}{4}t-\frac{21}{8}=0$

Đặt $t=m-\frac{7}{16m}$ thì pt trở thành:

$4m^3-\frac{343}{1024m^3}-\frac{21}{8}=0$
$\Leftrightarrow 4096m^6-2688m^3-343=0$

Coi đây là pt bậc 2 ẩn $m^3$ và giải ta thu được \(m=\frac{\sqrt[3]{49}}{4}\) hoặc \(m=\frac{-\sqrt[3]{7}}{4}\)

Khi đó ta thu được \(x=\frac{1}{4}(1-\sqrt[3]{7}+\sqrt[3]{49})\)

Nãy mình tìm được một cách giải tương tự cho câu 2.

PT \(\Leftrightarrow\left(x-1\right)\left(4x^3-3x^2+6x-4\right)=0\)

\(\Leftrightarrow\left[{}\begin{matrix}x-1=0\\4x^3-3x^2+6x-4=0\left(1\right)\end{matrix}\right.\)

Vậy pt có 1 nghiệm bằng 1.

\(\left(1\right)\Rightarrow8x^3-6x^2+12x-8=0\)

\(\Leftrightarrow7x^3+x^3-6x^2+12x-8=0\)

\(\Leftrightarrow\left(x-2\right)^3=-7x^3\)

\(\Leftrightarrow x-2=-\sqrt[3]{7}x\)

\(\Leftrightarrow x=\dfrac{2}{1+\sqrt[3]{7}}\)

Vậy pt có nghiệm \(S=\left\{1;\dfrac{2}{1+\sqrt[3]{7}}\right\}\)

Lưu ý: Nghiệm của người kia hoàn toàn tương đồng với nghiệm của mình (\(\dfrac{2}{1+\sqrt[3]{7}}=\dfrac{1}{4}\left(1-\sqrt[3]{7}+\sqrt[3]{49}\right)\))

1) Ta có: \(\left\{{}\begin{matrix}3\sqrt{x}-\sqrt{y}=5\\2\sqrt{x}+3\sqrt{y}=18\end{matrix}\right.\)

\(\Leftrightarrow\left\{{}\begin{matrix}9\sqrt{x}-3\sqrt{y}=15\\2\sqrt{x}+3\sqrt{y}=18\end{matrix}\right.\)

\(\Leftrightarrow\left\{{}\begin{matrix}11\sqrt{x}=33\\3\sqrt{x}-\sqrt{y}=5\end{matrix}\right.\)

\(\Leftrightarrow\left\{{}\begin{matrix}\sqrt{x}=3\\\sqrt{y}=4\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=9\\y=16\end{matrix}\right.\)

Vậy: Hệ phương trình có nghiệm duy nhất là \(\left\{{}\begin{matrix}x=9\\y=16\end{matrix}\right.\)

2) Ta có: \(\left\{{}\begin{matrix}\sqrt{x+3}-2\sqrt{y+1}=2\\2\sqrt{x+3}+\sqrt{y+1}=4\end{matrix}\right.\)

\(\Leftrightarrow\left\{{}\begin{matrix}-2\sqrt{x+3}+4\sqrt{y+1}=-4\\2\sqrt{x+3}+\sqrt{y+1}=4\end{matrix}\right.\)

\(\Leftrightarrow\left\{{}\begin{matrix}5\sqrt{y+1}=0\\\sqrt{x+3}-2\sqrt{y+1}=2\end{matrix}\right.\)

\(\Leftrightarrow\left\{{}\begin{matrix}\sqrt{y+1}=0\\\sqrt{x+3}=2\end{matrix}\right.\)

\(\Leftrightarrow\left\{{}\begin{matrix}y+1=0\\x+3=4\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}y=-1\\x=1\end{matrix}\right.\)

Vậy: Hệ phương trình có nghiệm duy nhất là \(\left\{{}\begin{matrix}x=1\\y=-1\end{matrix}\right.\)

4. Đk: \(x,y\ge0\)

\(\left\{{}\begin{matrix}\sqrt{x}+\sqrt{y+1}=1\\\sqrt{y}+\sqrt{x+1}=1\end{matrix}\right.\left(1\right)\)

Ta có: \(\left\{{}\begin{matrix}\sqrt{x}+\sqrt{y+1}\ge0+1=1\\\sqrt{y}+\sqrt{x+1}\ge0+1=1\end{matrix}\right.\left(2\right)\)

\(\left(1\right),\left(2\right)\Rightarrow\left\{{}\begin{matrix}\sqrt{x}=0,\sqrt{x+1}=1\\\sqrt{y}=0,\sqrt{y+1}=1\end{matrix}\right.\)

\(\Leftrightarrow\left\{{}\begin{matrix}x=0\\y=0\end{matrix}\right.\)<tmđk>

Vậy hệ pt có nghiệm \(\left(x,y\right)=\left(0;0\right)\)

\(\left\{{}\begin{matrix}2\left(xy+1\right)=x\left(x+y\right)+2\left(1\right)\\3xy-x+3=\sqrt{x+2y+1}+\sqrt{x+4y+4}\left(2\right)\end{matrix}\right.\)

Đk: \(x+2y+1\ge0,x+4y+4\ge0\)

\(\left(1\right)\Rightarrow2xy+2=x^2+xy+2\)

\(\Leftrightarrow x^2-xy=0\) \(\Leftrightarrow\left[{}\begin{matrix}x=0\\x=y\end{matrix}\right.\) 

*Khi \(x=0\), thay vào (2) ta được pt: \(\sqrt{2y+1}+\sqrt{4y+4}=3\)

Giải bằng phương pháp bình phương 2 vế ta được \(y=0\).

Thay \(x=y=0\) vào đk hoàn toàn thỏa mãn.

*Khi \(x=y\), thay vào (2) ta được pt: \(3x^2-x+3=\sqrt{3x+1}+\sqrt{5x+4}\) .

Mình không giải được nhưng pt có nghiệm \(x=0\) nên suy ra \(y=0\)Vậy hệ pt ban đầu có nghiệm \(\left(x,y\right)=\left(0;0\right)\).

a.

ĐKXĐ: \(\left\{{}\begin{matrix}x\ge2\\y\ge3\end{matrix}\right.\)

\(\left\{{}\begin{matrix}3\sqrt{x-2}+3\sqrt{y-3}=9\\2\sqrt{x-2}-3\sqrt{y-3}=-4\end{matrix}\right.\)

\(\Leftrightarrow\left\{{}\begin{matrix}3\sqrt{x-2}+3\sqrt{y-3}=9\\5\sqrt{x-2}=5\end{matrix}\right.\)

\(\Leftrightarrow\left\{{}\begin{matrix}3\sqrt{x-2}+3\sqrt{y-3}=9\\\sqrt{x-2}=1\end{matrix}\right.\)

\(\Leftrightarrow\left\{{}\begin{matrix}\sqrt{x-2}=1\\\sqrt{y-3}=2\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}x=3\\y=7\end{matrix}\right.\)

b.

ĐKXĐ: \(\left\{{}\begin{matrix}x\ne-1\\y\ne-4\end{matrix}\right.\)

\(\left\{{}\begin{matrix}\dfrac{15x}{x+1}+\dfrac{10}{y+4}=20\\\dfrac{4x}{x+1}-\dfrac{10}{y+4}=8\end{matrix}\right.\)

\(\Leftrightarrow\left\{{}\begin{matrix}\dfrac{15x}{x+1}+\dfrac{10}{y+4}=20\\\dfrac{19x}{x+1}=28\end{matrix}\right.\)

\(\Leftrightarrow\left\{{}\begin{matrix}\dfrac{x}{x+1}=\dfrac{28}{19}\\\dfrac{1}{y+4}=-\dfrac{4}{19}\end{matrix}\right.\)

\(\Leftrightarrow\left\{{}\begin{matrix}19x=28x+28\\4y+16=-19\end{matrix}\right.\)

\(\Leftrightarrow\left\{{}\begin{matrix}x=-\dfrac{28}{9}\\y=-\dfrac{35}{4}\end{matrix}\right.\)

\(\left\{{}\begin{matrix}\dfrac{x+2}{y-1}=\dfrac{x-4}{y+2}\\\dfrac{2x+3}{y-1}=\dfrac{4x+1}{2y+1}\end{matrix}\right.\)

⇔\(\left\{{}\begin{matrix}\left(x+2\right)\left(y+2\right)=\left(y-1\right)\left(x-\text{4}\right)\\\left(2x+3\right)\left(2y+1\right)=\left(y-1\right)\left(4x+1\right)\end{matrix}\right.\)

⇔\(\left\{{}\begin{matrix}xy+2x+2y+4=xy-4y-x+4\\4xy+2x+6y+3=4xy-4x+y-1\end{matrix}\right.\)

⇔\(\left\{{}\begin{matrix}3x+6y=0\\6x+5y=-4\end{matrix}\right.\)

⇔\(\left\{{}\begin{matrix}x=-\dfrac{8}{7}\\y=\dfrac{4}{7}\end{matrix}\right.\)(TM)

\(\left\{{}\begin{matrix}5\left(x-y\right)-3\left(2x+3y\right)=12\\3\left(x+2y\right)-4\left(x+2y\right)=5\end{matrix}\right.\)

⇔\(\left\{{}\begin{matrix}5x-5y-6x-9y=12\\3x+6y-4x-8y=5\end{matrix}\right.\)

⇔\(\left\{{}\begin{matrix}-x-14y=12\\-x-2y=5\end{matrix}\right.\)

⇔\(\left\{{}\begin{matrix}x=-\dfrac{26}{3}\\y=-\dfrac{7}{12}\end{matrix}\right.\)

Vậy HPT có nghiệm (x;y) = (\(-\dfrac{26}{3};-\dfrac{7}{12}\))

\(1,\Leftrightarrow\left\{{}\begin{matrix}x=y+5\\2y+10+y=11\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=\dfrac{16}{3}\\y=\dfrac{1}{3}\end{matrix}\right.\\ 2,\Leftrightarrow\left\{{}\begin{matrix}3x=1-2y\\1-2y+y=2\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=1\\y=-1\end{matrix}\right.\\ 3,\Leftrightarrow\left\{{}\begin{matrix}x=y+2\\3y+6+2y=11\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=3\\y=1\end{matrix}\right.\)

\(\left\{{}\begin{matrix}\sqrt{xy+\dfrac{x-y}{x^2+y^2+1}}+\sqrt{x}=y+\sqrt{y}\left(1\right)\\\left|x-1\right|+\left|y-2\right|=1+x^2-y^2\left(2\right)\end{matrix}\right.\)

ĐKXĐ: \(\left\{{}\begin{matrix}x\ge0\\y\ge0\\xy+\dfrac{x-y}{x^2+y^2+1}\ge0\end{matrix}\right.\)

\(\left(1\right)\Leftrightarrow\sqrt{xy+\dfrac{x-y}{x^2+y^2+1}}-y=\sqrt{y}-\sqrt{x}\)

\(\Leftrightarrow\dfrac{y\left(x-y\right)+\dfrac{x-y}{x^2+y^2+1}}{\sqrt{xy+\dfrac{x-y}{x^2+y^2+1}}+y}=\dfrac{x-y}{-xy}\Leftrightarrow\left(x-y\right)\left[\dfrac{y+\dfrac{1}{x^2+y^2+1}}{\sqrt{xy+\dfrac{x-y}{x^2+y^2+1}}+y}+xy\right]=0\Leftrightarrow x=y\).

Thay x = y vào (2) ta có \(\left|y-1\right|+\left|y-2\right|=1\). (*)

Ta có \(\left|y-1\right|+\left|y-2\right|=\left|y-1\right|+\left|2-y\right|\ge y-1+2-y=1\).

Mà đẳng thức xảy ra ở (1) nên ta phải có \(1\le y\le2\). (TMĐK)

Vậy pt đã cho có vô số nghiệm \(x=y=k\) với \(1\le k\le2\)