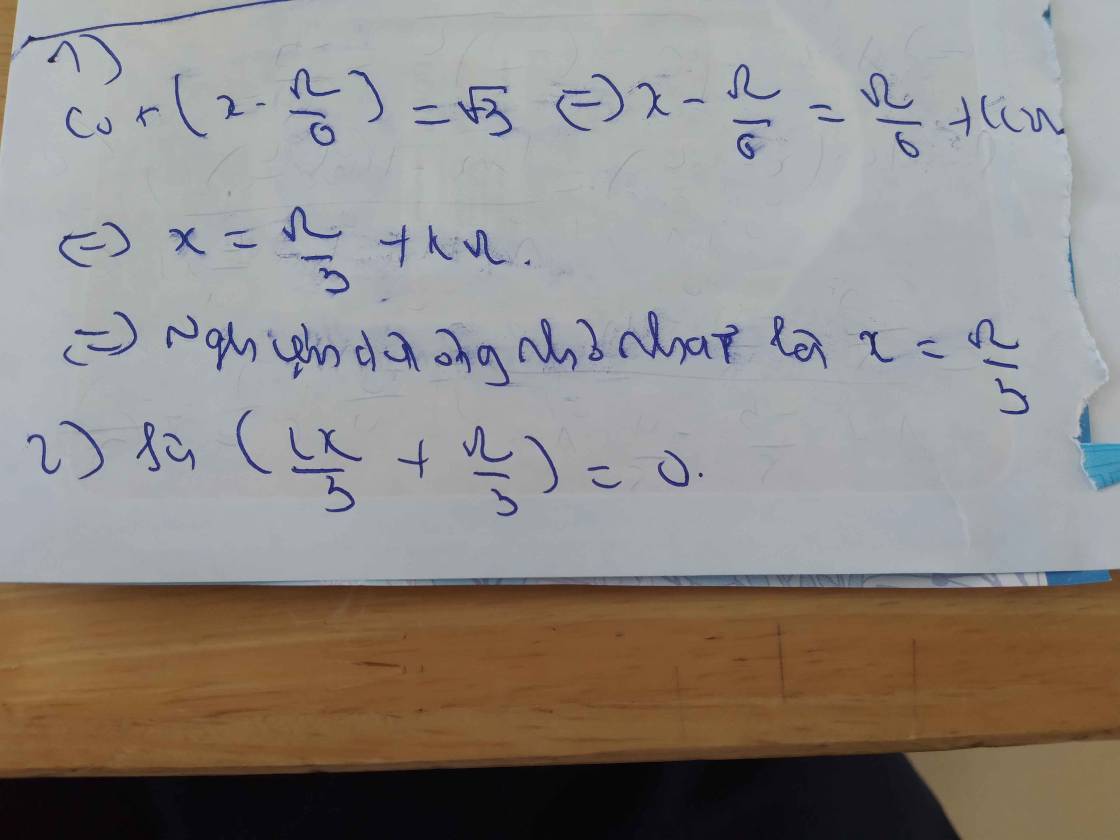\(\text{Phương trình cos5x=\dfrac{1}{\sqrt{2}} có bao nhiêu nghiệm thuộc đoạn [−50\pi ;0] ?}\)

Những câu hỏi liên quan
Phương trình \(sin\left(x^2-5x\right)=\dfrac{-\sqrt{3}}{2}\) có bao nhiêu nghiệm thuộc \(\left[0;\dfrac{\pi}{2}\right]\)
Giải các phương trình sau:
a) \(2sin\left(x+\dfrac{\pi}{5}\right)+\sqrt{3}=0\)
b)\(sin\left(2x-50\text{°}\right)=\dfrac{\sqrt{3}}{2}\)
c)\(\sqrt{3}tan\left(2x-\dfrac{\pi}{3}\right)-1=0\)
a: \(2\cdot sin\left(x+\dfrac{\Omega}{5}\right)+\sqrt{3}=0\)
=>\(2\cdot sin\left(x+\dfrac{\Omega}{5}\right)=-\sqrt{3}\)
=>\(sin\left(x+\dfrac{\Omega}{5}\right)=-\dfrac{\sqrt{3}}{2}\)
=>\(\left[{}\begin{matrix}x+\dfrac{\Omega}{5}=-\dfrac{\Omega}{3}+k2\Omega\\x+\dfrac{\Omega}{5}=\dfrac{4}{3}\Omega+k2\Omega\end{matrix}\right.\)
=>\(\left[{}\begin{matrix}x=-\dfrac{8}{15}\Omega+k2\Omega\\x=\dfrac{4}{3}\Omega-\dfrac{\Omega}{5}+k2\Omega=\dfrac{17}{15}\Omega+k2\Omega\end{matrix}\right.\)
b: \(sin\left(2x-50^0\right)=\dfrac{\sqrt{3}}{2}\)
=>\(\left[{}\begin{matrix}2x-50^0=60^0+k\cdot360^0\\2x-50^0=300^0+k\cdot360^0\end{matrix}\right.\)
=>\(\left[{}\begin{matrix}2x=110^0+k\cdot360^0\\2x=350^0+k\cdot360^0\end{matrix}\right.\)
=>\(\left[{}\begin{matrix}x=55^0+k\cdot180^0\\x=175^0+k\cdot180^0\end{matrix}\right.\)
c: \(\sqrt{3}\cdot tan\left(2x-\dfrac{\Omega}{3}\right)-1=0\)
=>\(\sqrt{3}\cdot tan\left(2x-\dfrac{\Omega}{3}\right)=1\)
=>\(tan\left(2x-\dfrac{\Omega}{3}\right)=\dfrac{1}{\sqrt{3}}\)
=>\(2x-\dfrac{\Omega}{3}=\dfrac{\Omega}{6}+k2\Omega\)
=>\(2x=\dfrac{1}{2}\Omega+k2\Omega\)
=>\(x=\dfrac{1}{4}\Omega+k\Omega\)
Đúng 1
Bình luận (1)
1. Tìm tham số m để phương trình 3cos2x-7=2m có nghiệm?
2. Trên đoạn \([0;2\pi]\) , phương trình \(2cos^2x-\sqrt{3}cosx=0\)có bao nhiêu nghiệm?
3. Có tất cả bao nhiêu giá trị nguyên dương của tham số m để hàm số \(y=\sqrt{2cosx-3m+14}\) xác định với mọi x thuộc R?
Help me!!!
1.
\(3cos2x-7=2m\)
\(\Leftrightarrow cos2x=\dfrac{2m-7}{3}\)
Phương trình đã cho có nghiệm khi:
\(-1\le\dfrac{2m-7}{3}\le1\)
\(\Leftrightarrow2\le m\le5\)
Đúng 1
Bình luận (0)
2.
\(2cos^2x-\sqrt{3}cosx=0\)
\(\Leftrightarrow cosx\left(2cosx-\sqrt{3}\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}cosx=0\\cosx=\dfrac{\sqrt{3}}{2}\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{\pi}{2}+k\pi\\x=\pm\dfrac{\pi}{6}+k2\pi\end{matrix}\right.\)
\(\Rightarrow\) Có 4 nghiệm \(\dfrac{\pi}{2};\dfrac{3\pi}{2};\dfrac{\pi}{6};\dfrac{11\pi}{6}\) thuộc đoạn \(\left[0;2\pi\right]\)
Đúng 1
Bình luận (0)
Số nghiệm của phương trình: \(sin2x+\sqrt{3}cos2x=\sqrt{3}\) trên khoảng \(\left(0;\dfrac{\pi}{2}\right)\)là bao nhiêu ?
Pt \(\Leftrightarrow2sin\left(2x+\dfrac{\pi}{3}\right)=\sqrt{3}\)
\(\Leftrightarrow sin\left(2x+\dfrac{\pi}{3}\right)=\dfrac{\sqrt{3}}{2}\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{\pi}{6}+k\pi\\x=k\pi\end{matrix}\right.\)\(\left(k\in Z\right)\)
\(x\in\left(0;\dfrac{\pi}{2}\right)\)\(\Rightarrow\left[{}\begin{matrix}0< \dfrac{\pi}{6}+k\pi< \dfrac{\pi}{2}\\0< k\pi< \dfrac{\pi}{2}\end{matrix}\right.\)\(\left(k\in Z\right)\)
\(\Leftrightarrow\left[{}\begin{matrix}-\dfrac{1}{6}< k< \dfrac{1}{3}\\0< k< \dfrac{1}{2}\end{matrix}\right.\)\(\left(k\in Z\right)\)\(\Leftrightarrow\left[{}\begin{matrix}k=0\\k\in\varnothing\end{matrix}\right.\)
Vậy có 1 nghiệm thỏa mãn
Đúng 4
Bình luận (0)
Trên khoảng (\(\dfrac{\pi}{2};2\pi\)) , phương trình \(cos\left(\dfrac{\pi}{6}-2x\right)=sinx\) có bao nhiêu nghiệm
\(cos\left(\dfrac{\pi}{6}-2x\right)=cos\left(\dfrac{\pi}{2}-x\right)\)
\(\Rightarrow\left[{}\begin{matrix}\dfrac{\pi}{6}-2x=\dfrac{\pi}{2}-x+k2\pi\\\dfrac{\pi}{6}-2x=x-\dfrac{\pi}{2}+k2\pi\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}x=-\dfrac{\pi}{3}+k2\pi\\x=\dfrac{2\pi}{9}+\dfrac{k2\pi}{3}\end{matrix}\right.\)
\(\Rightarrow x=\left\{\dfrac{8\pi}{9};\dfrac{14\pi}{9};\dfrac{5\pi}{3}\right\}\) có 3 nghiệm
Đúng 2
Bình luận (0)
1) nghiệm dương nhỏ nhất của phương trình \(cot\left(x-\dfrac{\pi}{6}\right)=\sqrt{3}\) là
2) phương trình \(sin\left(\dfrac{2x}{3}+\dfrac{\pi}{3}\right)=0\) có nghiệm là
3) họ nghiệm của phương trình \(cot\)(2x - 30 độ) = \(\sqrt{3}\) là
Tìm tất cả giá trị của tham số m để phương trình \(\left(sinx-2m+1\right)\left(2cosx-1\right)=0\)
a) Có 2 nghiệm thuộc \([-\dfrac{\pi}{2};\dfrac{5\pi}{6}]\)
b) Có 3 nghiệm thuộc \([-\dfrac{\pi}{2};\dfrac{5\pi}{6}]\)
Có tất cả bao nhiêu giá trị nguyên của tham số m để phương trình
mcos4x + 10sin2xcos2x = 15-m có đúng một nghiệm thuộc đoạn \(\left[\dfrac{-\pi}{6};\dfrac{\pi}{6}\right]\)
Tìm giá trị của m để phương trình \(\dfrac{sinx-m}{2cosx+\sqrt{3}}=0\) có đúng hai nghiệm thuộc \((0;\dfrac{5\pi}{2}]\)