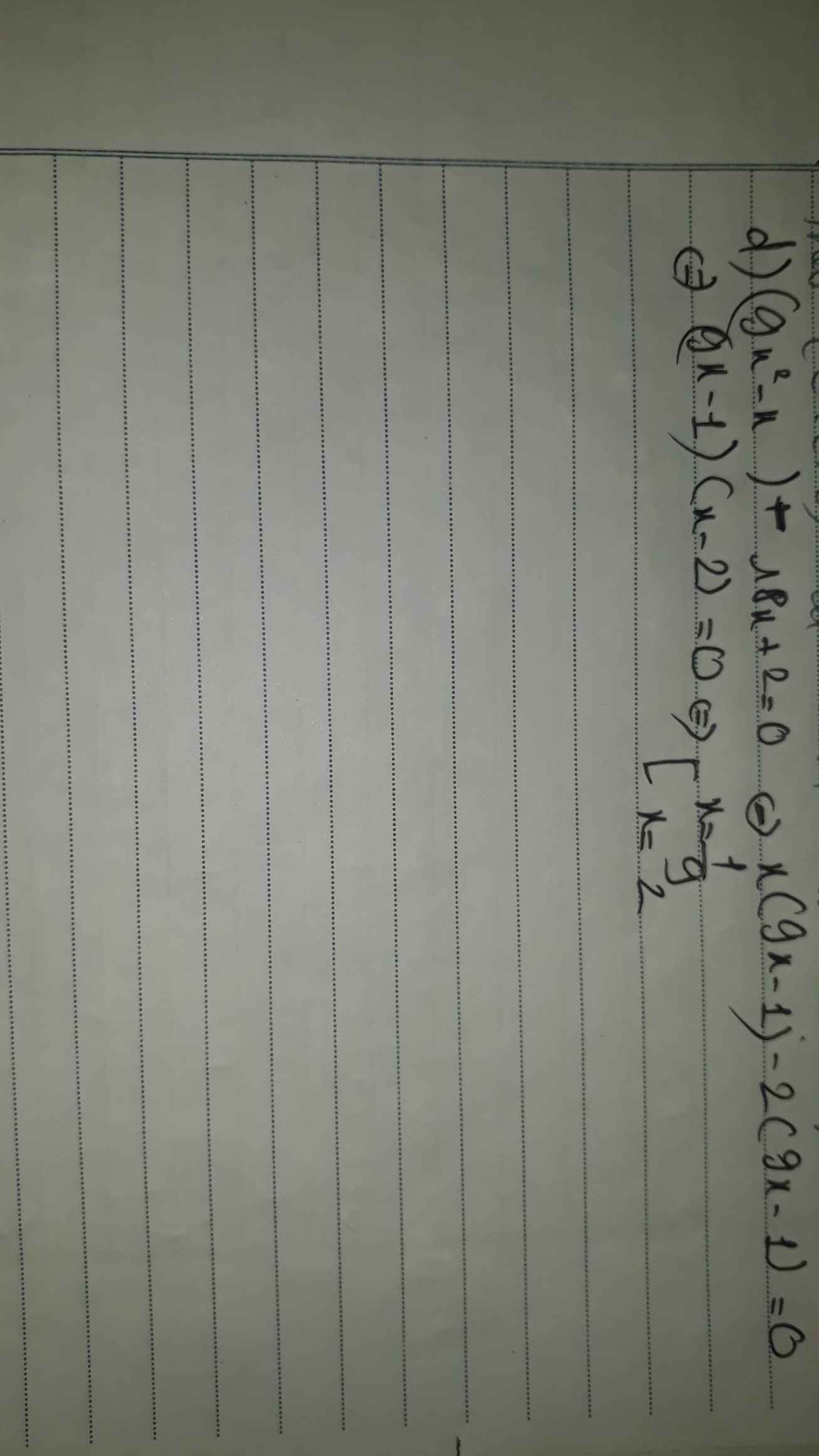so sanh : A= 9x2-12x+8..... B = 2

Những câu hỏi liên quan
cho A = 1+2+22+23+...+22014+22015
a thu gon A
b so sanh A voi 9x22013
Viết biểu thức sau dưới dạng tích
a) x 2 + 2x +1
b) 9x2 +y2 - 6xy
c) x 2 – 9
d) x 3 +12x2 +48x +64
e) x 3 - 6x2 +12x -8 f
) x 3 + 8
g) 8x3 – y
\(a,=\left(x+1\right)^2\\ b,=\left(3x-y\right)^2\\ c,=\left(x-3\right)\left(x+3\right)\\ d,=\left(x+4\right)^3\\ e,=\left(x-2\right)^3\\ f,=\left(x+2\right)\left(x^2-2x+4\right)\\ g,=\left(2x-y\right)\left(4x^2+2xy+y^2\right)\)
Đúng 0
Bình luận (0)
Tìm x:
a) x3-6x2+12x-8=\(\dfrac{-1}{1000}\)
b) x3-81x=0
c)x(7-2x)-7+2x=0
d)(9x2-x)-18x+2=0
tìm x biết
a,-25+49x2=0
b,16x2-25(x-2)2
c,(3x-2)2-9(x+4)(x+4)=2
d,x3-6x2+12x-8=0
e,-27+27x-9x2+x3=0
a: 49x^2-25=0
=>(7x-5)(7x+5)=0
=>7x-5=0 hoặc 7x+5=0
=>x=5/7 hoặc x=-5/7
b: Đề thiếu vế phải rồi bạn
c: (3x-2)^2-9(x+4)(x-4)=2
=>9x^2-12x+4-9(x^2-16)=2
=>9x^2-12x+4-9x^2+144=2
=>-12x+148=2
=>-12x=-146
=>x=146/12=73/6
d: x^3-6x^2+12x-8=0
=>(x-2)^3=0
=>x-2=0
=>x=2
e: x^3-9x^2+27x-27=0
=>(x-3)^3=0
=>x-3=0
=>x=3
Đúng 0
Bình luận (1)
a) \(-25+49x^2=0\)
\(\Leftrightarrow49x^2-25=0\)
\(\Leftrightarrow\left(7x\right)^2-5^2=0\)
\(\Leftrightarrow\left(7x-5\right)\left(7x+5\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}7x-5=0\\7x+5=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}7x=5\\7x=-5\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{5}{7}\\x=-\dfrac{5}{7}\end{matrix}\right.\)
b) \(16x^2-25\left(x-2\right)^2=0\)
\(\Leftrightarrow\left(4x\right)^2-\left[5\left(x-2\right)\right]^2=0\)
\(\Leftrightarrow\left(4x-5x+10\right)\left(4x+5x-10\right)=0\)
\(\Leftrightarrow\left(10-x\right)\left(9x-10\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}10-x=0\\9x=10\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=10\\x=\dfrac{10}{9}\end{matrix}\right.\)
c) \(\left(3x-2\right)^2-9\left(x+4\right)\left(x+4\right)=2\)
\(\Leftrightarrow9x^2-12x+4-9\left(x^2+8x+16\right)=2\)
\(\Leftrightarrow9x^2-12x+4-9x^2-72x-144=2\)
\(\Leftrightarrow-84x-140=2\)
\(\Leftrightarrow-84x=142\)
\(\Leftrightarrow x=-\dfrac{142}{84}\)
\(\Leftrightarrow x=-\dfrac{71}{42}\)
d) \(x^3-6x^2+12x-8=0\)
\(\Leftrightarrow x^3-3\cdot2\cdot x^2+3\cdot2^2\cdot x-2^3=0\)
\(\Leftrightarrow\left(x-2\right)^3=0\)
\(\Leftrightarrow x-2=0\)
\(\Leftrightarrow x=2\)
e) \(-27+27x-9x^2+x^3=0\)
\(\Leftrightarrow x^3-9x^2+27x-27=0\)
\(\Leftrightarrow\left(x-3\right)^3=0\)
\(\Leftrightarrow x-3=0\)
\(\Leftrightarrow x=3\)
Đúng 1
Bình luận (0)
Mình sửa lại câu c một chút nha:
c: (3x-2)^2-9(x+4)(x+4)=2
=>(3x-2)^2-9(x+4)^2=2
=>(3x-2)^2-(3x+12)^2=2
=>(3x-2-3x-12)(3x-2+3x+12)=2
=>-14*(6x+10)=2
=>6x+10=-1/7
=>6x=-71/7
=>x=-71/42
Đúng 0
Bình luận (0)
Xem thêm câu trả lời
Phân tích đa thức sau thành nhân tử A) 12x³-9x2+3x B) x2-y²+6x+9
a ) x=0; x = -(căn bậc hai(7)*i-3)/8;x = (căn bậc hai(7)*i+3)/8;
b ) -(y-x-3)*(y+x+3)
Đúng 0
Bình luận (0)
a) \(12x^3-9x^2+3x\)
\(=3x\left(4x^2-3x+1\right)\)
b) \(x^2-y^2+6x+9\)
\(=\left(x^2+6x+9\right)-y^2\)
\(=\left(x+3\right)^2-y^2\)
\(=\left(x+y+3\right)\left(x-y+3\right)\)
Đúng 0
Bình luận (0)
1) Phân tích đa thức thành nhân tử:
a) x3 - 4x2 - 12x + 27
b) 9x2 + 6x - 8
c) x2 - 7xy + 10y2
d) x8 + x7 + 1
a) x3 - 4x2 - 12x + 27
= \(\left(x^3+3x^2\right)-\left(7x^2+21x\right)+\left(9x+27\right)\)
= \(\left(x+3\right)\left(x^2-7x+9\right)\)
b) 9x2 + 6x - 8
=\(9x^2-6x+12x-8=3x\left(3x-2\right)+4\left(3x-2\right)\)
=\(\left(3x-2\right)\left(3x+4\right)\)
c) x2 - 7xy + 10y2
=\(x^2-5xy-2xy+10y^2=x\left(x-5y\right)-2y\left(x-5y\right)\)
=\(\left(x-5y\right)\left(x-2y\right)\)
Đúng 0
Bình luận (0)
a) x3 - 4x2 - 12x + 27
=x3 + 3x2 - 7x2 - 21x + 9x + 27
= x2(x+3) - 7x(x+3) + 9(x+3)
= (x2 - 7x + 9)(x + 3)
b) 9x2 + 6x - 8
= 9x2 - 6x + 12x - 8
= 3x(3x - 2) + 4(3x - 2)
= (3x + 4)(3x - 2)
c) x2 - 7xy + 10y2
= x2 - 5xy - 2xy + 10y2
= x(x - 5y) - 2y(x - 5y)
= (x - 2y)(x - 5y)
d) x8 + x7 + 1
Ta thêm vào các số hạng x6, x5, x4, x3, x2, x và cùng bớt đi các số hạng ấy ta có:
= x8 - x6 + x5 - x3 + x2 + x7 - x5 + x4 -x2 +x + x6 - x4 + x3 - x + 1
= x2(x6 - x4 + x3 - x + 1) + x(x6 - x4 + x3 - x + 1) + x6 - x4 + x3 - x + 1
= (x2 + x + 1)(x6 - x4 + x3 - x + 1)
Đúng 0
Bình luận (2)
a) \(x^3-4x^2-12x+27\)
\(=\left(x^3+27\right)-\left(4x^2+12x\right)\)
\(=\left(x+3\right)\left(x^2-3x+9\right)-4x\left(x+3\right)\)
\(=\left(x+3\right)\left(x^2-3x+9-4x\right)\)
\(=\left(x+3\right)\left(x^2-7x+9\right)\)
b) \(9x^2+6x-8\)
\(=9x^2+6x+1-9\)
\(=\left(3x+1\right)^2-9\)
\(=\left(3x+1-9\right)\left(3x+1+9\right)\)
\(=\left(3x+8\right)\left(3x+10\right)\)
c) \(x^2-7xy+10y^2\)
\(=x^2-2xy-5xy+10y^2\)
\(=x\left(x-2y\right)-5y\left(x-2y\right)\)
\(=\left(x-2y\right)\left(x-5\right)\)
Đúng 0
Bình luận (1)
bài 1 tính
2 3 phần 4 : 1 1phần 3
bài 2 tính nhanh
a) 12x -7/11x5/6x22/7 b) -8/15x7/9x5/8x(-18)
c) -5/11x13/28-13/28x6/11 d) 4/9.7/11+4/9x6/11-4/9x2/11
e) 11/22-3/16x8/18+1/18
giúp mik với ah =))
bài 1:
\(2\dfrac{3}{4}:1\dfrac{1}{3}=\dfrac{11}{4}:\dfrac{4}{3}=\dfrac{33}{16}\)
Đúng 1
Bình luận (1)
Thực hiện các phép tính sau:a)
P
(
4
x
2
−
1
)
1
2
x
−
1
−
1
2
x
+
1
−...
Đọc tiếp
Thực hiện các phép tính sau:
a) P = ( 4 x 2 − 1 ) 1 2 x − 1 − 1 2 x + 1 − 1 với x ≠ ± 1 2 ;
b) Q = 3 x + 3 − 9 x 2 + 6 x + 9 : 3 x 2 − 9 + 1 3 − x với x ≠ 0 và x ≠ ± 3
a) Ta có P = ( 4 x 2 − 1 ) ( 2 x + 1 ) − ( 2 x − 1 ) − ( 4 x 2 − 1 ) ( 2 x + 1 ) ( 2 x − 1 ) = 3 − 4 x 2
b) Ta có Q = 3 x ( x + 3 ) . ( x + 3 ) ( x − 3 ) − x = 9 − 3 x x + 3
Đúng 0
Bình luận (0)
CMR: các bt sau luôn có gt dương vs mọi gt của biến
a, 9x2 - 6x + 2
b, x2 + x + 1
c, 2x2 + 2x + 1
CMR: bt sau luôn âm vs mọi gt của biến
-9x2 + 12x - 15
câu a: 9x^2-6x+2=(3x-1)^2+1>=1>0 mọi x
câu b:x^2+x+1=(x-1/2)^2+3/4>0 với mới x
Đúng 0
Bình luận (0)