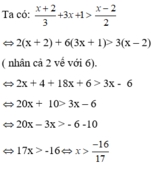giải bất phương trình |x-|x-1||>3x+|x|

Những câu hỏi liên quan
1.Giải các phương trình sau : a,7x+35=0 b, 8-x/x-7 -8 =1/x-7 2.giải bất phương trình sau : 18-3x(1-x)_< 3x^2-3x
a: 7x+35=0
=>7x=-35
=>x=-5
b: \(\dfrac{8-x}{x-7}-8=\dfrac{1}{x-7}\)
=>8-x-8(x-7)=1
=>8-x-8x+56=1
=>-9x+64=1
=>-9x=-63
hay x=7(loại)
Đúng 1
Bình luận (0)
a, \(7x=-35\Leftrightarrow x=-5\)
b, đk : x khác 7
\(8-x-8x+56=1\Leftrightarrow-9x=-63\Leftrightarrow x=7\left(ktm\right)\)
vậy pt vô nghiệm
2, thiếu đề
Đúng 0
Bình luận (0)
1.
\(a,7x+35=0\\ \Rightarrow7x=-35\\ \Rightarrow x=-5\\ b,ĐKXĐ:x\ne7\\ \dfrac{8-x}{x-7}-8=\dfrac{1}{x-7}\\ \Leftrightarrow\dfrac{8-x}{x-7}-\dfrac{8\left(x-7\right)}{x-7}-\dfrac{1}{x-7}=0\\ \Leftrightarrow\dfrac{8-x-8x+56-1}{x-7}=0\\ \Rightarrow-9x+63=0\\ \Leftrightarrow-9x=-63\\ \Leftrightarrow x=7\left(ktm\right)\)
2.đề thiếu
Đúng 0
Bình luận (0)
Bài 1: Giải các bất phương trình:
3(1 - x)> \(\dfrac{7-3x^2}{x+1}\)
Bài 2. Giải và biện luận bất phương trình
( m2 - 4 ) x +3 > ( 2m -1) x +m
cho hàm số \(f\left(x\right)=x^3-3x^2+2\)
a, giải bất phương trình \(f'\left(x\right)\le0\)
b, giải phương trình \(f'=\left(x^2-3x+2\right)=0\)
c, đặt \(g\left(x\right)=f\left(1-2x\right)+x^2-x+2022\) giải bất phương trình\(g'\left(x\right)\ge0\)
\(a,f'\left(x\right)=3x^2-6x\\ f'\left(x\right)\le0\Leftrightarrow3x^2-6x\le0\\ \Leftrightarrow3x\left(x-2\right)\le0\Leftrightarrow0\le x\le2\)
Đúng 2
Bình luận (0)
Lời giải:
a. $f'(x)\leq 0$
$\Leftrightarrow 3x^2-6x\leq 0$
$\Leftrightarrow x(x-2)\leq 0$
$\Leftrightarrow 0\leq x\leq 2$
b.
$f'(x)=x^2-3x+2=0$
$\Leftrightarrow 3x^2-6x=x^2-3x+2=0$
$\Leftrightarrow 3x(x-2)=(x-1)(x-2)=0$
$\Leftrightarrow x-2=0$
$\Leftrightarrow x=2$
c.
$g(x)=f(1-2x)+x^2-x+2022$
$g'(x)=(1-2x)'f(1-2x)'_{1-2x}+2x-1$
$=-2[3(1-2x)^2-6(1-2x)]+2x-1$
$=-24x^2+2x+5$
$g'(x)\geq 0$
$\Leftrightarrow -24x^2+2x+5\geq 0$
$\Leftrightarrow (5-12x)(2x-1)\geq 0$
$\Leftrightarrow \frac{-5}{12}\leq x\leq \frac{1}{2}$
Đúng 1
Bình luận (0)
Giải bất phương trình:
x
+
2
3
+
3
x
+
1
x
-
2
2
A.
x
-
16
17
B.
x
6
17
C.
x
-
16
17
D. ...
Đọc tiếp
Giải bất phương trình: x + 2 3 + 3 x + 1 > x - 2 2
A. x < - 16 17
B. x > 6 17
C. x > - 16 17
D. x > - 6 11
1) Giải các phương trình sau : a) x-3/x=2-x-3/x+3 b) 3x^2-2x-16=0 2) Giải bất phương trình sau: 4x-3/4>3x-5/3-2x-7/12
\(a,\dfrac{x-3}{x}=\dfrac{x-3}{x+3}\)\(\left(đk:x\ne0,-3\right)\)
\(\Leftrightarrow\dfrac{x-3}{x}-\dfrac{x-3}{x+3}=0\)
\(\Leftrightarrow\dfrac{\left(x-3\right)\left(x+3\right)-x\left(x-3\right)}{x\left(x+3\right)}=0\)
\(\Leftrightarrow x^2-9-x^2+3x=0\)
\(\Leftrightarrow3x-9=0\)
\(\Leftrightarrow3x=9\)
\(\Leftrightarrow x=3\left(n\right)\)
Vậy \(S=\left\{3\right\}\)
Đúng 1
Bình luận (0)
\(b,\dfrac{4x-3}{4}>\dfrac{3x-5}{3}-\dfrac{2x-7}{12}\)
\(\Leftrightarrow\dfrac{4x-3}{4}-\dfrac{3x-5}{3}+\dfrac{2x-7}{12}>0\)
\(\Leftrightarrow\dfrac{3\left(4x-3\right)-4\left(3x-5\right)+2x-7}{12}>0\)
\(\Leftrightarrow12x-9-12x+20+2x-7>0\)
\(\Leftrightarrow2x+4>0\)
\(\Leftrightarrow2x>-4\)
\(\Leftrightarrow x>-2\)
Đúng 1
Bình luận (0)
Giải các bất phương trình sau:
x + x > 2 x + 3 x - 1
Giải bất phương trình: \(\dfrac{x-2}{x+1}\)≥ \(\dfrac{3x+2}{x-1}-2\)
\(BPT\Leftrightarrow\dfrac{\left(x-2\right)\left(x-1\right)}{\left(x+1\right)\left(x-1\right)}\ge\dfrac{\left(3x+2\right)\left(x+1\right)}{\left(x+1\right)\left(x-1\right)}-\dfrac{2\left(x+1\right)\left(x-1\right)}{\left(x+1\right)\left(x-1\right)}\)
\(\Rightarrow x^2-x-2x+2-3x^2-3x-2x-2-2x^2-2\ge0\)
\(\Leftrightarrow-4x^2-8x-2\ge0\)
\(\Leftrightarrow x^2+2x+\dfrac{1}{2}\ge0\)
\(\Leftrightarrow\left(x+1\right)^2-\dfrac{1}{2}\ge0\)
Vậy bất phương trình luôn đúng \(\forall x\).
Đúng 0
Bình luận (0)
ĐKXĐ: \(x\ne1,-1\)
Ta có: \(\dfrac{x-2}{x+1}\ge\dfrac{3x+2}{x-1}-2\)
\(\dfrac{x-2}{x+1}\ge\dfrac{3x+2-2\left(x-1\right)}{x-1}\)
\(\dfrac{x-2}{x+1}-\dfrac{3x+2-2x+2}{x-1}\ge0\)
\(\dfrac{x-2}{x+1}-\dfrac{x+4}{x-1}\ge0\)
\(\dfrac{\left(x-2\right)\left(x-1\right)-\left(x-4\right)\left(x+1\right)}{x^2-1}\ge0\)
\(\dfrac{x^2-3x+2-x^2+3x+4}{x^2-1}\ge0\)
\(\dfrac{6}{x^2-1}\ge0\)
\(\Rightarrow x^2-1>0\Leftrightarrow x^2>1\Leftrightarrow\left\{{}\begin{matrix}x< -1\\x>1\end{matrix}\right.\)(TM)
Đúng 3
Bình luận (0)
Biểu thức vế trái có nghĩa khi
\(x\ne-2;x\ne1\\ \dfrac{x-2}{x+2}+\dfrac{x+1}{x-1}\Leftrightarrow\dfrac{x-2}{x+2}+\dfrac{x+1}{x-1}-2>0\\ \dfrac{\left(x-2\right)\left(x-1\right)+\left(x+2\right)\left(x+1\right)-2\left(x+2\right)\left(x-1\right)}{\left(x+2\right)\left(x-1\right)}\\ >0\\ \Leftrightarrow\dfrac{8-2x}{\left(x+2\right)\left(x-1\right)}>0\\ \Leftrightarrow\dfrac{4-x}{\left(x+2\right)\left(x-1\right)}>0\)
\(\Leftrightarrow\left(x+2\right)\left(x-1\right)\left(x-4\right)< 0\)
Lập bảng xét dấu
| x | -2 | 1 | 4 |
| x+2 | - 0 + | + | + |
| x-1 | - - | 0 + | + |
| x-4 | - - | - | 0 + |
| VT | - 0 + | 0 - | 0 + |
Vậy nghiệm của bất pt là
\(x< -2.hay.1< x< 4\)
Đúng 0
Bình luận (0)
Bài I: 1) Giải các phương trình a/8 + 4x = 3x – 1
2) Giải các bất phương trình a) 10 - 5(x + 3) > 3(x - 1)
1) Ta có: \(4x+8=3x-1\)
\(\Leftrightarrow4x-3x=-1-8\)
\(\Leftrightarrow x=-9\)
2) Ta có: \(10-5\left(x+3\right)>3\left(x-1\right)\)
\(\Leftrightarrow10-5x-15-3x+3>0\)
\(\Leftrightarrow-8x>2\)
hay \(x< \dfrac{-1}{4}\)
Đúng 1
Bình luận (0)
Giải bất phương trình sau: (2x - 1)(x + 3) - 3x + 1 ≤ (x - 1)(x + 3) + x2 - 5
(2x – 1)(x + 3) – 3x + 1 ≤ (x – 1)(x + 3) + x2 – 5
⇔ 2x2 + 6x - x – 3 – 3x + 1 ≤ x2 + 3x - x – 3 + x2 – 5
⇔ 2x2 + 2x – 2 ≤ 2x2 + 2x – 8
⇔ 6 ≤ 0 (Vô lý).
Vậy BPT vô nghiệm.
Đúng 0
Bình luận (0)