Thực hiện phép tính :
(x^2-2xy+2(y^2)).(x^2+2xy+2(y^2)
Thực hiện phép tính:
a/(x^2+y^2-2xy)+(x^2+y^2 +2xy)
b/(x^2+y^2-2xy) - (x^2+y^2+2xy)
a.
(x^2 + y^2 - 2xy) + (x^2 + y^2 + 2xy)
= x^2 + y^2 - 2xy + x^2 + y^2 + 2xy
= (x^2 + x^2) + (y^2 + y^2) + (2xy - 2xy)
= 2x^2 + 2y^2
b.
(x^2 + y^2 - 2xy) - (x^2 + y^2 + 2xy)
= x^2 + y^2 - 2xy - x^2 - y^2 - 2xy
= (x^2 - x^2) + (y^2 - y^2) - (2xy + 2xy)
= -4xy
Thực hiện phép tính: \(\dfrac{x}{{x + y}} + \dfrac{{2xy}}{{{x^2} - {y^2}}} - \dfrac{y}{{x + y}}\)
`x/(x+y) + (2xy)/(x^2-y^2) - y(x+y)`
`= (x(x-y))/(x^2-y^2) + (2xy)/(x^2-y^2) - (y(x-y))/(x^2-y^2)`
`= (x^2 - xy + 2xy - xy + y^2)/(x^2-y^2)`
`= (x^2+y^2)/(x^2-y^2)`
\(\dfrac{x}{x+y}+\dfrac{2xy}{x^2-y^2}-\dfrac{y}{x+y}\)
\(=\dfrac{x-y}{x+y}+\dfrac{2xy}{\left(x+y\right)\left(x-y\right)}\)
\(=\dfrac{\left(x-y\right)^2}{\left(x+y\right)\left(x-y\right)}+\dfrac{2xy}{\left(x+y\right)\left(x-y\right)}\)
\(=\dfrac{x^2-2xy+y^2+2xy}{\left(x+y\right)\left(x-y\right)}\)
\(=\dfrac{x^2+y^2}{x^2-y^2}\)
\(MTC:x^2-y^2=\left(x+y\right)\left(x-y\right)\\ =\dfrac{x\left(x-y\right)}{\left(x-y\right)\left(x+y\right)}+\dfrac{2xy}{x^2-y^2}-\dfrac{y\left(x-y\right)}{\left(x+y\right)\left(x-y\right)}\\ =\dfrac{x\left(x-y\right)+2xy-y\left(x-y\right)}{x^2-y^2}\\ =\dfrac{x^2-xy+2xy-xy+y^2}{x^2-y^2}=\dfrac{x^2+y^2}{x^2-y^2}\)
Bài 3:
3: \(6x\left(x-y\right)-9y^2+9xy\)
\(=6x\left(x-y\right)+9xy-9y^2\)
\(=6x\left(x-y\right)+9y\left(x-y\right)\)
\(=\left(x-y\right)\left(6x+9y\right)\)
\(=3\left(2x+3y\right)\left(x-y\right)\)
Bài 4:
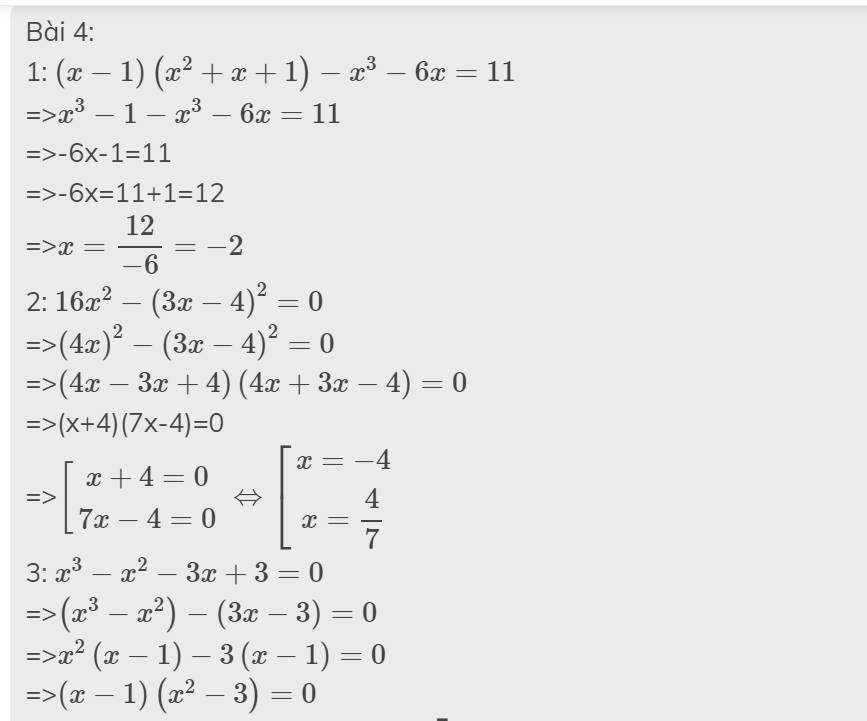

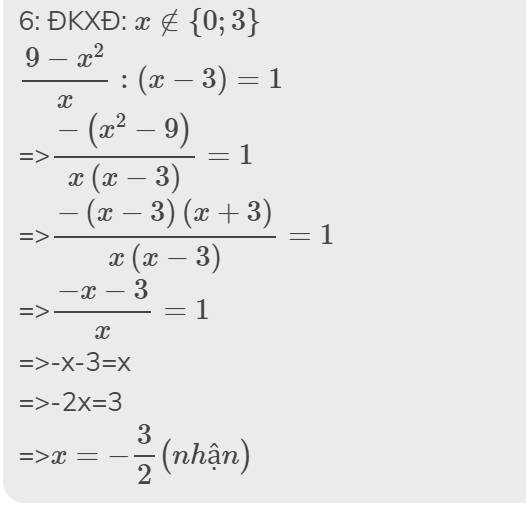
thực hiện phép cộng
\(\dfrac{3}{x^2+2xy+y^2}\)+ \(\dfrac{4}{2xy-x^2-y^2}\)+\(\dfrac{5}{x^2-y^2}\)
giúp mình với
Thực hiện phép tính :
( x + y ) ( x^2 + 2xy + y^2 )
giải cách dễ hiểu nhất nhé
`@` `\text {Ans}`
`\downarrow`
\(( x + y ) ( x^2 + 2xy + y^2 )\)
`= x(x^2 +2xy + y^2) + y(x^2 + 2xy + y^2)`
`= x^3 + 2x^2y + xy^2 + x^2y + 2xy^2 + y^3`
`= x^3 + 3x^2y + 3xy^2 + y^3`
Thực hiện phép tính
x^2/[(x-y)^2(x+y)] - 2xy^2/(x^4-2x^2y^2+y^4)+y^2/[(x^2-y^2)(x+y)]
giúp mk với nhé
sáng mai nộp rồi
ai nhanh tay mk sẽ k cho
nhân đơn thức và đa thức
bài1: thực hiện phép tính sau
[(x2-2xy+2xy2).(x+2y)-(x2+4y2).(x-y)]2xy=
[(x2-2xy+2xy2).(x+2y)-(x2+4y2).(x-y)]2xy
=( x3 + 2x2y-2x2y-4xy2+2x2y2+4xy3-x3+x2y-4xy2+4y3 )2xy
=2xy(2x2y2-8xy2+4xy3+x2y+4y3)
= 4x3y3-16x2y3+8x2y4+2x3y2+8xy4
Trả lời:
[ ( x2 - 2xy + 2xy2 ) ( x + 2y ) - ( x2 + 4y2 ) ( x - y ) ] 2xy
= [ ( x3 + 2x2y - 2x2y - 4xy2 + 2x2y2 + 4xy3 ) - ( x3 - x2y + 4xy2 - 4y3 ) ] 2xy
= ( x3 + 2x2y - 2x2y - 4xy2 + 2x2y2 + 4xy3 - x3 + x2y - 4xy2 + 4y3 ) 2xy
= ( x2y - 8xy2 + 2x2y2 + 4xy3 + 4y3 ) 2xy
= 2x3y2 - 16x2y3 + 4x3y3 + 8x2y4 + 8xy4
Bài 3 ( 3đ) : Thực hiện phép tính
\(\dfrac{y}{x-y}-\dfrac{x^3-xy^2}{x^2+y^2}.\left(\dfrac{x}{x^2-2xy+y^2}-\dfrac{y}{x^2-y^2}\right)\)
Ta có: \(\dfrac{y}{x-y}-\dfrac{x^3-xy^2}{x^2+y^2}\cdot\left(\dfrac{x}{x^2-2xy+y^2}-\dfrac{y}{x^2-y^2}\right)\)
\(=\dfrac{y}{x-y}-\dfrac{x\left(x^2-y^2\right)}{x^2+y^2}\cdot\left(\dfrac{x\left(x+y\right)}{\left(x-y\right)^2\cdot\left(x+y\right)}-\dfrac{y\cdot\left(x-y\right)}{\left(x-y\right)^2\cdot\left(x+y\right)}\right)\)
\(=\dfrac{y}{x-y}-\dfrac{x\left(x-y\right)\left(x+y\right)}{x^2+y^2}\cdot\dfrac{x^2+xy-xy+y^2}{\left(x-y\right)^2\left(x+y\right)}\)
\(=\dfrac{y}{x-y}-\dfrac{x\cdot\left(x^2+y^2\right)}{\left(x^2+y^2\right)\cdot\left(x-y\right)}\)
\(=\dfrac{y}{x-y}-\dfrac{x}{x-y}\)
\(=\dfrac{y-x}{x-y}=\dfrac{-\left(x-y\right)}{x-y}=-1\)
Thực hiện phép tính sau
A.(2x^2y-3xy+4xy^2)÷(2xy)
B.1/xy-x^2-1/y^2-xy
C.[x/xy-y^2- 2x-y/x^2-xy]:(1/x-1/y)
a: \(=x-\dfrac{3}{2}+2y\)
b: \(=\dfrac{1}{x\left(y-x\right)}-\dfrac{1}{y\left(y-x\right)}=\dfrac{y-x}{xy\left(y-x\right)}=\dfrac{1}{xy}\)