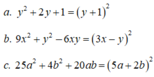a) \(16x^2+8xy+y^2=\left(4x+y\right)^2\)

b) \(4x^2-2xy+\dfrac{1}{4}y^2=\left(2x-\dfrac{1}{2}y\right)^2\)

c) \(x^2+x+\dfrac{1}{4}=\left(x+\dfrac{1}{2}\right)^2\)

d) \(9x^2-6xy+y^2=\left(3x-y\right)^2\)

\(A=\left(6x-3y\right)+\left(4x^2-4xy+y^2\right)=3\left(2x-y\right)+\left(2x-y\right)^2=\left(2x-y\right)\left(2+2x-y\right)\)

\(B=9x^2-\left(y^2-4y+4\right)=9x^2-\left(y-2\right)^2=\left(3x-y+2\right)\left(3x+y-2\right)\)

\(C=-25x^2+y^2-6y+9=\left(y^2-6y+9\right)-25x^2=\left(y-3\right)^2-\left(5x\right)^2=\left(y-3-5x\right)\left(y-3+5x\right)\)\(D=x^2-4x-y^2-8y-12=\left(x^2-4x+4\right)-\left(y^2+8y+16\right)=\left(x-2\right)^2-\left(y+4\right)^2=\left(x-2-y-4\right)\left(x-2+y+4\right)=\left(x-y-6\right)\left(x+y+2\right)\)

a: Ta có: \(A=\left(6x-3y\right)+\left(4x^2-4xy+y^2\right)\)

\(=3\left(2x-y\right)+\left(2x-y\right)^2\)

\(=\left(2x-y\right)\left(2x-y+3\right)\)

b: Ta có: \(B=9x^2-\left(y^2-4y+4\right)\)

\(=9x^2-\left(y-2\right)^2\)

\(=\left(3x-y+2\right)\left(3x+y-2\right)\)

\(A=\left(2x-1\right)^2+9\ge9\\ A_{min}=9\Leftrightarrow x=\dfrac{1}{2}\\ B=2\left(x^2-2\cdot\dfrac{3}{4}x+\dfrac{9}{16}\right)+\dfrac{1}{8}=2\left(x-\dfrac{3}{4}\right)^2+\dfrac{1}{8}\ge\dfrac{1}{8}\\ B_{min}=\dfrac{1}{8}\Leftrightarrow x=\dfrac{3}{4}\\ C=\left(4x^2+4xy+y^2\right)+2\left(2x+y\right)+1+\left(y^2+4y+4\right)-4\\ C=\left[\left(2x+y\right)^2+2\left(2x+y\right)+1\right]+\left(y+2\right)^2-4\\ C=\left(2x+y+1\right)^2+\left(y+2\right)^2-4\ge-4\\ C_{min}=-4\Leftrightarrow\left\{{}\begin{matrix}2x=-1-y\\y=-2\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=-\dfrac{3}{2}\\y=-2\end{matrix}\right.\)

\(D=\left(3x-1-2x\right)^2=\left(x-1\right)^2\ge0\\ D_{min}=0\Leftrightarrow x=1\\ G=\left(9x^2+6xy+y^2\right)+\left(y^2+4y+4\right)+1\\ G=\left(3x+y\right)^2+\left(y+2\right)^2+1\ge1\\ G_{min}=1\Leftrightarrow\left\{{}\begin{matrix}3x=-y\\y=-2\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=\dfrac{2}{3}\\y=-2\end{matrix}\right.\)

\(H=\left(x^2-2xy+y^2\right)+\left(x^2+2x+1\right)+\left(2y^2+4y+2\right)+2\\ H=\left(x-y\right)^2+\left(x+1\right)^2+2\left(y+1\right)^2+2\ge2\\ H_{min}=2\Leftrightarrow\left\{{}\begin{matrix}x=y\\x=-1\\y=-1\end{matrix}\right.\Leftrightarrow x=y=-1\)

Ta luôn có \(\left(x-y\right)^2+\left(y-z\right)^2+\left(z-x\right)^2\ge0\)

\(\Leftrightarrow2x^2+2y^2+2z^2-2xy-2yz-2xz\ge0\\ \Leftrightarrow x^2+y^2+z^2\ge xy+yz+xz\\ \Leftrightarrow x^2+y^2+z^2+2xy+2yz+2xz\ge3xy+3yz+3xz\\ \Leftrightarrow\left(x+y+z\right)^2\ge3\left(xy+yz+xz\right)\\ \Leftrightarrow\dfrac{3^2}{3}\ge xy+yz+xz\\ \Leftrightarrow K\le3\\ K_{max}=3\Leftrightarrow x=y=z=1\)

b: \(8x^2-48x+6xy-36y\)

\(=8x\left(x-6\right)+6y\left(x-6\right)\)

\(=2\left(x-6\right)\left(4x+3y\right)\)

d: \(a^2-2ab+b^2-4\)

\(=\left(a-b\right)^2-4\)

\(=\left(a-b-2\right)\left(a-b+2\right)\)

1b.=2((x+y)+(x+y)(x-y)+(x-y))=2(x²-y²+x+y+x-y)=2(x²-y²+2x)=2x²-2y²+4x

2a.=4xy+4xy+2y=8xy+2y=2y(4x+1)

b.=(3x)²+2.3x.y+y²-(2z)²=(3x+y)²-(2z)²=(3x+y-2z)(3x+y+2z)

c.=x²-x-7x+7=x(x-1)-7(x-1)=(x-1)(x-7)

\(\left(x+y\right)^2+2\left(x+y\right)\left(x-y\right)+\left(x-y\right)^2\)

\(=\left(x+y+x-y\right)^2\)

\(=\left(2x\right)^2\)

\(=4x^2\)

hk tốt

^^

Cách 1 : Xác định các hệ số a, b, c.

a) x2 + y2 – 2x – 2y – 2 = 0 có hệ số a = 1 ; b = 1 ; c = –2

⇒ tâm I (1; 1) và bán kính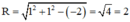 

b) 16x2 + 16y2 + 16x – 8y –11 = 0

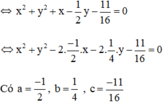
⇒ Đường tròn có tâm 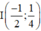 , bán kính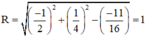 

c) x2 + y2 - 4x + 6y - 3 = 0

⇔ x2 + y2 - 2.2x - 2.(-3).y - 3 = 0

có hệ số a = 2, b = -3,c = -3

⇒ Đường tròn có tâm I(2 ; –3), bán kính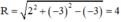 

Cách 2 : Đưa về phương trình chính tắc :

a) x2 + y2 - 2x - 2y - 2 = 0

⇔ (x2 - 2x + 1) + (y2 - 2y +1) = 4

⇔(x-1)2 + (y-1)2 = 4

Vậy đường tròn có tâm I(1 ; 1) và bán kính R = 2.

b) 16x2 + 16y2 + 16x - 8y - 11 = 0

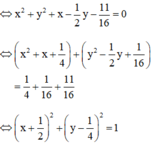
Vậy đường tròn có tâm 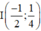 và bán kính R = 1.

c) x2 + y2 - 4x + 6y -3 = 0

⇔ (x2 - 4x + 4) + (y2 + 6y + 9) = 4 + 9 + 3

⇔ (x - 2)2 + (y + 3)2 = 16

Vậy đường tròn có tâm I( 2 ; –3) và bán kính R = 4.

\(A=x^3-8-128-x^3=-136\\ B=8x^3+27y^3-27x^3+8y^3=-19x^3+35y^3\)

\(A=\left(x-2\right)\left(x^2+2x+4\right)-\left(128+x^3\right)=x^3-8-128-x^3=-136\)

\(B=\left(2x+3y\right)\left(4x^2-6xy+9y^2\right)-\left(3x-2y\right)\left(9x^2+6xy+4y^2\right)=8x^3+27y^3-27x^3+8y^3=-19x^3+35y^3\)

\(A=x^3+2x^2+4x-2x^2-4x-8-128-x^3\)
\(A=-136\)
\(B=\left(2x+3y\right)\left(2x-3y\right)^2-\left(3x-2y\right)\left(3x+2y\right)^2\)
\(B=\left(2x+3y\right)\left(2x-3y\right)\left(2x-3y\right)-\left(3x-2y\right)\left(3x+2y\right)\left(3x+2y\right)\)
\(B=\left(4x^2-9y^2\right)\left(2x-3y\right)-\left(9x^2-4y^2\right)\left(3x+2y\right)\)
\(B=8x^3-12x^2y-18xy^2-27y^3-27x^3-18x^2y+12xy^2+8y^3\)
\(B=-19x^3-30x^2y-6xy^2-19y^3\)
\(B=-19\left(x^3-y^3\right)-6xy\left(5x+y\right)\)

\(9x^2+6xy+y^2-25\)

\(=\left(3x+y\right)^2-25\)

\(=\left(3x+y-5\right)\left(3x+y+5\right)\)

\(9x^2+6xy+y^2-25=\left(3x+y\right)^2-25=\left(3x+y-5\right)\left(3x+y+5\right)\)

9x²+6xy+y²-25= (3x)²+2.3x.y+y² -25

                        = (3x+y)²-5²

                        = (3x+y-5).(3x+y+5)

Học tốt!