Giúp e câu 2 vs ạ :( Cho pt: 4x

Những câu hỏi liên quan
Giải PT (có đặt ẩn phụ)
a. x^2 +2y^2=4x+4y-6
(giúp mik vs ạ)
=>x^2-4x+2y^2-4y+6=0
=>x^2-4x+4+2y^2-4y+2=0
=>(x-2)^2+2(y-1)^2=0
=>x=2 và y=1
Đúng 0
Bình luận (0)
1) Tìm m để pt : -2x2 - 3x - m + 1 = 0 có 2 nghiệm âm phân biệt.
2) Tìm m để pt : -3x2 - 4x -2m + 1 = 0 có 2 nghiệm âm.
MN GIÚP E BÀI NÀY VỚI Ạ. E ĐANG CẦN GẤP Ạ.
\(1,\Leftrightarrow\left\{{}\begin{matrix}\Delta=\left(-3\right)^2-4\left(-2\right)\left(-m+1\right)>0\\x_1+x_2=\dfrac{3}{-2}< 0\\x_1x_2=\dfrac{-m+1}{-2}>0\end{matrix}\right.\\ \Leftrightarrow\left\{{}\begin{matrix}17-8m>0\\-m+1< 0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}m< \dfrac{17}{8}\\m>1\end{matrix}\right.\Leftrightarrow1< m< \dfrac{17}{8}\)
\(2,\Leftrightarrow\left\{{}\begin{matrix}\Delta=\left(-4\right)^2-4\left(-3\right)\left(-2m+1\right)\ge0\\x_1+x_2=\dfrac{4}{-3}< 0\\x_1x_2=\dfrac{-2m+1}{-3}>0\end{matrix}\right.\\ \Leftrightarrow\left\{{}\begin{matrix}28-24m\ge0\\-2m+1< 0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}m\le\dfrac{7}{6}\\m>\dfrac{1}{2}\end{matrix}\right.\Leftrightarrow\dfrac{1}{2}< m\le\dfrac{7}{6}\)
Đúng 2
Bình luận (1)
Cho pt : x² -4x + m=0 (*). Tìm m để phương trình (*) có 2 nghiệm x1, x2 thỏa mãn hệ thức 2x1 + x2 = 7
Giải chi tiết giúp e ạ, vắn tắt quá e ko hiểu được ạ😭
\(x^2-4x+m=0\)
Để pt có 2 nghiệm \(x_1,x_2\Leftrightarrow\Delta\ge0\Leftrightarrow\left(-4\right)^2-4m\ge0\Leftrightarrow m\le4\)
Theo Vi-ét, ta có :
\(\left\{{}\begin{matrix}x_1+x_2=-\dfrac{b}{a}=4\\x_1x_2=\dfrac{c}{a}=m\end{matrix}\right.\)
Ta có :
\(2x_1+x_2=7\)
\(\Leftrightarrow\left\{{}\begin{matrix}2=\dfrac{x_1+x_2}{2}\\2x_1+x_2=7\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x_1+x_2=4\\2x_1+x_2=7\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x_1=3\\x_2=1\end{matrix}\right.\)
Thay \(x_1x_2=m\Leftrightarrow m=3.1=3\left(tmdk\right)\)
Vậy m = 3 thì pt có 2 nghiệm \(x_1,x_2\) thỏa mãn \(2x_1+x_2=7\)
Đúng 2
Bình luận (0)
2sin^2.2x-3cos2x+6sin^2-9=0. Giúp e giải pt này vs ạ
Đề là: \(2sin^22x-3cos2x+6sin^2x-9=0\) đúng không nhỉ?
\(\Leftrightarrow2\left(1-cos^22x\right)-3cos2x+3\left(1-cos2x\right)-9=0\)
\(\Leftrightarrow-2cos^22x-6cos2x-4=0\)
\(\Leftrightarrow cos^22x+3cos2x+2=0\)
\(\Leftrightarrow\left[{}\begin{matrix}cos2x=-1\\cos2x=-2\left(loại\right)\end{matrix}\right.\)
\(\Rightarrow...\)
Đúng 1
Bình luận (0)
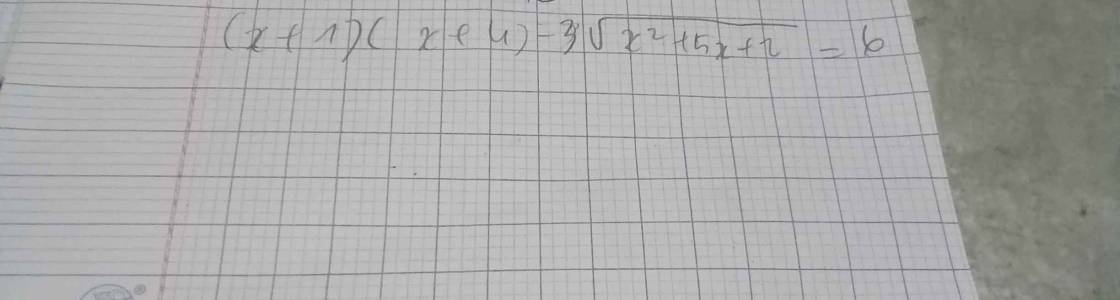 giải pt này giúp e vs ạ
giải pt này giúp e vs ạ
ĐKXĐ: \(x^2+5x+2>=0\)
=>\(\left[{}\begin{matrix}x>=\dfrac{-5+\sqrt{17}}{2}\\x< =\dfrac{-5-\sqrt{17}}{2}\end{matrix}\right.\)
\(\left(x+1\right)\left(x+4\right)-3\sqrt{x^2+5x+2}=6\)
=>\(x^2+5x+4-3\sqrt{x^2+5x+2}-6=0\)
=>\(x^2+5x+2-3\sqrt{x^2+5x+2}-4=0\)(1)
Đặt \(\sqrt{x^2+5x+2}=a\)(a>=0)
Phương trình (1) trở thành:
\(a^2-3a-4=0\)
=>(a-4)(a+1)=0
=>\(\left[{}\begin{matrix}a=4\left(nhận\right)\\a=-1\left(loại\right)\end{matrix}\right.\)
=>\(x^2+5x+2=4^2=16\)
=>\(x^2+5x-14=0\)
=>\(\left(x+7\right)\left(x-2\right)=0\)
=>\(\left[{}\begin{matrix}x=-7\left(nhận\right)\\x=2\left(nhận\right)\end{matrix}\right.\)
Đúng 1
Bình luận (0)
Tìm số a để đa thức 2x^3-4x^2+6x+a chia hết cho đa thức x+2 Giải giúp e vs e đang cần gấp lắm ạ
Để \(2x^3-4x^2+6x+a⋮x+2\)
\(\Leftrightarrow2x^3-4x^2+6x+a=\left(x+2\right)\cdot a\left(x\right)\)
Thay \(x=-2\)
\(\Leftrightarrow2\left(-2\right)^3-4\left(-2\right)^2+6\left(-2\right)+a=0\\ \Leftrightarrow-16-16-12+a=0\\ \Leftrightarrow-44+a=0\Leftrightarrow a=44\)
Đúng 3
Bình luận (0)
Tìm x:(làm rõ ra từng cái giúp e vs ạ)
(x - 2)(4x + 1) - 4x(x + 7) = 1
\(\Leftrightarrow4x^2+x-8x-2-4x^2-27x=1\)
=>-34x=3
hay x=-3/34
Đúng 1
Bình luận (0)
\(\left(x-2\right)\left(4x+1\right)-4x\left(x+7\right)=1\\ \Rightarrow4x^2-8x+x-2-4x^2-28x=1\\ \Rightarrow-35x=3\\ \Rightarrow x=\dfrac{-3}{35}\)
Đúng 1
Bình luận (0)
(x - 2)(4x + 1) - 4x(x+7) = 1
⇔ 4x2 + x - 8x - 2 - 4x2 - 28x = 1
⇔ - 35x = 3
⇔ x = - 3/35
Đúng 1
Bình luận (0)
GIÚP EM BÀI GIẢI PT VÀ CÂU C,D,E BÀI 2 VỚI Ạ..
\(b,B=\dfrac{\sqrt{x}+2}{\sqrt{x}-3}+\dfrac{\sqrt{x}-8}{x-5\sqrt{x}+6}\left(x\ge0;x\ne4;x\ne9\right)\\ B=\dfrac{\left(\sqrt{x}+2\right)\left(\sqrt{x}-2\right)+\sqrt{x}-8}{\left(\sqrt{x}-2\right)\left(\sqrt{x}-3\right)}\\ B=\dfrac{x-4+\sqrt{x}-8}{\left(\sqrt{x}-2\right)\left(\sqrt{x}-3\right)}=\dfrac{\left(\sqrt{x}-3\right)\left(\sqrt{x}-4\right)}{\left(\sqrt{x}-2\right)\left(\sqrt{x}-3\right)}=\dfrac{\sqrt{x}-4}{\sqrt{x}-2}\)
\(c,B< A\Leftrightarrow\dfrac{\sqrt{x}-4}{\sqrt{x}-2}< \dfrac{\sqrt{x}+1}{\sqrt{x}-2}\Leftrightarrow\dfrac{\sqrt{x}-4}{\sqrt{x}-2}-\dfrac{\sqrt{x}+1}{\sqrt{x}-2}< 0\\ \Leftrightarrow\dfrac{-5}{\sqrt{x}-2}< 0\Leftrightarrow\sqrt{x}-2>0\left(-5< 0\right)\\ \Leftrightarrow x>4\\ d,P=\dfrac{B}{A}=\dfrac{\sqrt{x}-4}{\sqrt{x}-2}:\dfrac{\sqrt{x}+1}{\sqrt{x}-2}=\dfrac{\sqrt{x}-4}{\sqrt{x}+1}=1-\dfrac{5}{\sqrt{x}+1}\in Z\\ \Leftrightarrow5⋮\sqrt{x}+1\Leftrightarrow\sqrt{x}+1\inƯ\left(5\right)=\left\{-5;-1;1;5\right\}\\ \Leftrightarrow\sqrt{x}\in\left\{-6;-2;0;4\right\}\\ \Leftrightarrow x\in\left\{0;16\right\}\left(\sqrt{x}\ge0\right)\)
\(e,P=1-\dfrac{5}{\sqrt{x}+1}\)
Ta có \(\sqrt{x}+1\ge1,\forall x\Leftrightarrow\dfrac{5}{\sqrt{x}+1}\ge5\Leftrightarrow1-\dfrac{5}{\sqrt{x}+1}\le-4\)
\(P_{max}=-4\Leftrightarrow x=0\)
Đúng 2
Bình luận (0)
Tìm nghiệm nguyên của pt \(x^2+5y^2-4xy+4x-8y-12=0\)
*Làm bằng cách sử dụng \(\Delta\) hoặc Δ' giúp e với ạ
PT <=> \(x^2-4x\left(y-1\right)+5y^2-8y-12=0\)
Xét \(\Delta'=\left[-2\left(y-1\right)\right]^2-1.\left(5y^2-8y-12\right)\)
= \(4\left(y^2-2y+1\right)-5y^2+8y+12\)
= \(-y^2+16\)
Để PT có nghiệm <=> \(\Delta'\ge0< =>-y^2+16\ge0\)
<=> \(y^2\le16\) <=> \(-4\le y\le4\)
Mà y nguyên
<=> \(y\in\left\{-4;-3;-2;-1;0;1;2;3;4\right\}\)
Đến đây bn thay y vào PT để tìm x nhé
Đúng 1
Bình luận (2)






















