
giải chi tiết ra giúp em với ạ!!
giải chi tiết ra giúp em với ạ

giải chi tiết ra giúp em với ạ
a: Xét tứ giác ADHE có \(\widehat{ADH}=\widehat{AEH}=\widehat{DAE}=90^0\)
nên ADHE là hình chữ nhật
Suy ra: AH=DE
b: Xét ΔABC vuông tại A có AH là đường cao
nên \(AH^2=HB\cdot HC\)
c: Xét ΔAHB vuông tại H có HD là đường cao
nên \(AD\cdot AB=AH^2\left(1\right)\)
Xét ΔAHC vuông tại H có HE là đường cao
nên \(AE\cdot AC=AH^2\left(2\right)\)
Từ (1) và (2) suy ra \(AD\cdot AB=AE\cdot AC\)
hay AD/AC=AE/AB
Xét ΔADE vuông tại A và ΔACB vuông tại A có
AD/AC=AE/AB
Do đó: ΔADE\(\sim\)ΔACB
Giải chi tiết ra giúp em với ! em cảm ơn ạ !!
a) \(I_4=\int\dfrac{3x+5}{2x^2+x+10}dx\)
\(=\int\dfrac{\dfrac{3}{4}\left(4x+1\right)+\dfrac{17}{4}}{2x^2+x+10}dx=\dfrac{3}{4}\int\dfrac{\left(4x+1\right)dx}{2x^2+x+10}+\dfrac{17}{4}\int\dfrac{dx}{2x^2+x+10}\)
\(=\dfrac{3}{4}\int\dfrac{d\left(2x^2+x+10\right)}{2x^2+x+10}+\dfrac{17}{8}\int\dfrac{dx}{x^2+\dfrac{x}{2}+5}\)
\(=\dfrac{3}{4}\ln\left(2x^2+x+10\right)+\dfrac{17}{8}\int\dfrac{dx}{\left(x+\dfrac{1}{4}\right)^2+\dfrac{79}{16}}\)
\(=\dfrac{3}{4}\ln\left(2x^2+x+10\right)+\dfrac{17}{8}\int\dfrac{dx}{\left(x+\dfrac{1}{4}\right)^2+\dfrac{79}{16}}\)
\(=\dfrac{3}{4}\ln\left(2x^2+x+10\right)+\dfrac{17}{8}\int\dfrac{d\left(x+\dfrac{1}{4}\right)}{\left(x+\dfrac{1}{4}\right)^2+\left(\dfrac{\sqrt{79}}{4}\right)^2}\)
\(=\dfrac{3}{4}\ln\left(2x^2+x+10\right)+\dfrac{17}{8}.\dfrac{4}{\sqrt{79}}arctan\left(\dfrac{4x+1}{\sqrt{79}}\right)+C\)
\(=\dfrac{3}{4}\ln\left(2x^2+x+10\right)+\dfrac{17}{2\sqrt{79}}arctan\left(\dfrac{4x+1}{\sqrt{79}}\right)+C\)
b) \(I_5=\int\dfrac{4x-1}{6x^2+9x+4}dx\)
\(=\int\dfrac{\dfrac{1}{3}\left(12x+9\right)-4}{6x^2+9x+4}dx\)
\(=\dfrac{1}{3}\int\dfrac{\left(12x+9\right)dx}{6x^2+9x+4}-4\int\dfrac{dx}{6x^2+9x+4}\)
\(=\dfrac{1}{3}\int\dfrac{d\left(6x^2+9x+4\right)}{6x^2+9x+4}-4\int\dfrac{dx}{\left(3x+1\right)^2+3}\)
\(=\dfrac{1}{3}\ln\left(6x^2+9x+4\right)-\dfrac{4}{3}\int\dfrac{d\left(3x+1\right)}{\left(3x+1\right)^2+\left(\sqrt{3}\right)^2}\)
\(=\dfrac{1}{3}\ln\left(6x^2+9x+4\right)-\dfrac{4}{3}.\dfrac{1}{\sqrt{3}}arctan\left(\dfrac{3x+1}{\sqrt{3}}\right)+C\)
Câu 23, 24 và 25 ạ
Giải chi tiết ra giúp em với ạ

23.
Ta sẽ tìm điểm \(I\left(a;b;c\right)\) sao cho \(\overrightarrow{IA}+\overrightarrow{IB}+\overrightarrow{IC}+\overrightarrow{ID}=\overrightarrow{0}\) (1)
\(\left\{{}\begin{matrix}\overrightarrow{IA}=\left(-2-a;2-b;6-c\right)\\\overrightarrow{IB}=\left(-3-a;1-b;8-c\right)\\\overrightarrow{IC}=\left(-1-a;-b;7-c\right)\\\overrightarrow{ID}=\left(1-a;2-b;3-c\right)\end{matrix}\right.\)
\(\Rightarrow\overrightarrow{IA}+\overrightarrow{IB}+\overrightarrow{IC}+\overrightarrow{ID}=\left(-5-4a;5-4b;24-4c\right)\)
(1) thỏa mãn khi: \(\left\{{}\begin{matrix}-5-4a=0\\5-4b=0\\24-4c=0\end{matrix}\right.\) \(\Rightarrow\left\{{}\begin{matrix}a=-\dfrac{5}{4}\\b=\dfrac{5}{4}\\c=6\end{matrix}\right.\)
\(\Rightarrow I\left(-\dfrac{5}{4};\dfrac{5}{4};6\right)\)
Khi đó:
\(T=MA^2+MB^2+MC^2+MD^2=\left(\overrightarrow{MI}+\overrightarrow{IA}\right)^2+\left(\overrightarrow{MI}+\overrightarrow{IB}\right)^2+\left(\overrightarrow{MI}+\overrightarrow{IC}\right)^2+\left(\overrightarrow{MI}+\overrightarrow{ID}\right)^2\)
\(=4MI^2+IA^2+IB^2+IC^2+ID^2+2\overrightarrow{MI}\left(\overrightarrow{IA}+\overrightarrow{IB}+\overrightarrow{IC}+\overrightarrow{ID}\right)\)
\(=4MI^2+IA^2+IB^2+IC^2+ID^2\) (do \(\overrightarrow{IA}+\overrightarrow{IB}+\overrightarrow{IC}+\overrightarrow{ID}=\overrightarrow{0}\))
\(IA^2+IB^2+IC^2+ID^2\) cố định nên \(T_{min}\) khi \(MI_{min}\)
\(\Leftrightarrow M\) trùng I
\(\Rightarrow M\left(-\dfrac{5}{4};\dfrac{5}{4};6\right)\Rightarrow x+y+z=-\dfrac{5}{4}+\dfrac{5}{4}+6=6\)
24.
\(a+b=4\Rightarrow b=4-a\)
ABCD là hình chữ nhật \(\Rightarrow\overrightarrow{AB}=\overrightarrow{DC}\)
\(\Rightarrow C\left(a;a;0\right)\)
Tương tự ta có: \(C'\left(a;a;b\right)\)
M là trung điểm CC' \(\Rightarrow M\left(a;a;\dfrac{b}{2}\right)\)
\(\Rightarrow\left\{{}\begin{matrix}\overrightarrow{A'B}=\left(a;0;-b\right)=\left(a;0;a-4\right)\\\overrightarrow{A'D}=\left(0;a;-b\right)=\left(0;a;a-4\right)\\\overrightarrow{A'M}=\left(a;a;-\dfrac{b}{2}\right)=\left(a;a;\dfrac{a-4}{2}\right)\end{matrix}\right.\)
Theo công thức tích có hướng:
\(\left[\overrightarrow{A'B};\overrightarrow{A'D}\right]=\left(-a^2+4a;-a^2+4a;a^2\right)\)
\(\Rightarrow V=\dfrac{1}{6}\left|\left[\overrightarrow{A'B};\overrightarrow{A'D}\right].\overrightarrow{A'M}\right|=\dfrac{1}{6}\left|a\left(-a^2+4a\right)+a\left(-a^2+4a\right)+\dfrac{a^2\left(a-4\right)}{2}\right|\)
\(=\dfrac{1}{4}\left|a^3-4a^2\right|=\dfrac{1}{4}\left(4a^2-a^3\right)\)
Xét hàm \(f\left(a\right)=\dfrac{1}{4}\left(4a^2-a^3\right)\) trên \(\left(0;4\right)\)
\(f'\left(a\right)=\dfrac{1}{4}\left(8a-3a^2\right)=0\Rightarrow\left[{}\begin{matrix}a=0\left(loại\right)\\a=\dfrac{8}{3}\end{matrix}\right.\)
\(\Rightarrow f\left(a\right)_{max}=f\left(\dfrac{8}{3}\right)=\dfrac{64}{27}\)
Mn oi giải giúp em với ạ mn giải chi tiết giúp em với ạ
1.I think you ought to give up playing video games. (should)
-->I think you should give up playing video games.
2.It's necessary for me to finish the work on time. (have to)
--->I have to finish the work on time.
3.It wasn't necessary for you to clean that car. (have to)
--->You didn't have to clean that car.
4.It was quite unnecessary for you to adopt a green lifestyle. (have to)
---->You didn't have to adopt a green lifestyle.
5.It was careless of you to leave the windows open last night. (shouldn't)
----->You shouldn't have left the windows open last night.
6.It is advisable for each member in the family to share the housework equally. (should)
---->Each member in the family should share the housework equally.
7.You are required to come back home before 10 p.m. (must)
--->You must come back home before 10 p.m.
8.Lina is advised to prepare carefully in the morning. (should)
----->Lina should prepare carefully in the morning.
9.Tu is responsible for picking up litter. (have to)
------>Tu has to pick up litter.
1.You are required to ask your parents for permission before staying out late. (must)
You must ask your parents for permission before staying out late.
2.I think you ought to give up smoking immediately. (should)
I think you should give up smoking immediately.
3.It is not a good idea for me to stay up late. (shouldn't)
I shouldn't stay up late.
4.It wasn't necessary for you to send these letters. (have to)
You didn't have to send these letters.
5.It was unnecessary for Tim to finish the work. (have to)
Tim didn't have to finish the work.
6.It was careless of you to leave your children alone at home. (shouldn't)
You shouldn't have left your children alone at home.
Bằng 1 cách nào đó 1 câu hỏi từ 2023 ở đây và tôi vẫn trả lời nó sau 1 năm :v
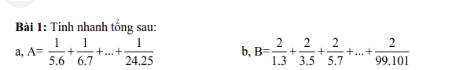
giúp em với ạ ( giải chi tiết giúp em ạ)
A = 1/(5.6) + 1/(6.7) + ... + 1/(24.25)
= 1/5 - 1/6 + 1/6 - 1/7 + ... + 1/24 - 1/25
= 1/5 - 1/25
= 4/25
B = 2/(1.3) + 2/(3.5) + 2/(5.7) + ... + 2/(99.101)
= 1 - 1/3 + 1/3 - 1/5 + 1/5 - 1/7 + ... + 1/99 - 1/101
= 1 - 1/101
= 100/101
`a) A = 1/(5.6) + 1/(6.7)+...+1/(24.25)`
`= 1/5 - 1/6 + 1/6 - 1/7 +...+1/24-1/25`
`= 1/5-1/25`
`= 5/25 - 1/25`
`= 4/25`
Vậy:`A = 4/25`
`b) B = 2/(1.3)+2/(3.5)+...+2/(99.101)`
`= 1- 1/3 + 1/3 - .... +1/99-1/101`
`= 1 - 1/101`
`= 100/101`
Vậy: `B = 100/101`
Dạ giải chi tiết ra giúp em vs ạ ❤️❤️❤️Em cảm ơn trc ạ
a) Ta có: O hóa trị II, ta gọi hóa trị Mn cần tìm trong hợp chất MnO2 là x: \(Mn^xO_2^{II}\)
Theo quy tắc hóa trị, ta có:
1.x=2.II
=>x= (2.II)/1= IV
=> Hóa trị x của Mn cần tìm trong hợp chất MnO2 là IV.
a) Ta có: (PO4) hóa trị II, ta gọi hóa trị Mn cần tìm trong hợp chất là y: \(Ba^y_3\left(PO_4\right)^{III}_2\)
Theo quy tắc hóa trị, ta có:
3.y=III.2
=>y=(III.2)/3=II
=> Hóa trị y của Ba cần tìm trong hợp chất Ba3(PO4)2 là II.

giải chi tiết giúp em với ạ em cảm ơn ạ
a, Xét tứ giác ADHE có ^ADH = ^AEH = ^DAE = 900
=> tứ giác ADHE là hcn
=> AH = DE (2 đường chéo bằng nhau)
b, Xét tam giác AHB và tam giác CHA ta có
^AHB = ^CHA = 900
^HAB = ^HCA ( cùng phụ ^HAC )
Vậy tam giác AHB~ tam giác CHA (g.g)
\(\dfrac{AH}{CH}=\dfrac{HB}{AH}\Rightarrow AH^2=BH.CH\)
c, Xét tam giác AHD và tam giác ABH có
^ADH = ^AHB = 900
^A _ chung
Vậy tam giác AHD ~ tam giác ABH (g.g)
\(\dfrac{AH}{AB}=\dfrac{AD}{AH}\Rightarrow AH^2=AD.AB\)(1)
tương tự tam giác AEH ~ tam giác AHC (g.g)
\(\dfrac{AE}{AH}=\dfrac{AH}{AC}\Rightarrow AH^2=AE.AC\left(2\right)\)
Từ (1) ; (2) suy ra \(AD.AB=AE.AC\Rightarrow\dfrac{AD}{AC}=\dfrac{AE}{AB}\)
Xét tam giác ADE và tam giác ACB
^A _ chung
\(\dfrac{AD}{AC}=\dfrac{AE}{AB}\left(cmt\right)\)
Vậy tam giác ADE ~ tam giác ACB (c.g.c)
-329 + (115-101) - (15-440) Giúp em với ạ (giải chi tiết giúp em ạ