Giải phương trình:
\(2\sqrt{3}sin3x.cos3x-4cos^32x=2sinx-3cos2x\)
Giải các phương trình sau 3 cos 2 x - 2 sin x + 2 = 0
3 cos 2 x - 2 sin x + 2 = 0 ⇔ 3 ( 1 - sin 2 x ) - 2 sin x + 2 = 0 ⇔ 3 sin 2 x + 2 sin x - 5 = 0 ⇔ ( sin x - 1 ) ( 3 sin x + 5 ) = 0 ⇔ sin x = 1 ⇔ x = π / 2 + k 2 π , k ∈ Z
Giải phương trình \(3cos2x-\sqrt{3}sin2x+2sinx-2\sqrt{3}cosx+1=0\)
\((2sinx-1)(2sin2x-1)=3-4cos^2x\) . giải phương trình
Pt \(\Leftrightarrow\left(2sinx-1\right)\left(2sin2x-1\right)=3-4\left(1-sin^2x\right)\)
\(\Leftrightarrow2sin2x\left(2sinx-1\right)-2sinx+1=-1+4sin^2x\)
\(\Leftrightarrow2sin2x\left(2sinx-1\right)-\left(4sin^2x+2sinx-2\right)=0\)
\(\Leftrightarrow2sin2x\left(2sinx-1\right)-2\left(2sinx-1\right)\left(sinx+1\right)=0\)
\(\Leftrightarrow2\left(2sinx-1\right)\left(sin2x-sinx-1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}sinx=\dfrac{1}{2}\left(1\right)\\sin2x=sinx+1\left(2\right)\end{matrix}\right.\)
Từ (1) \(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{\pi}{6}+k2\pi\\x=\dfrac{5\pi}{6}+k2\pi\end{matrix}\right.\),\(k\in Z\)
Từ (2)\(\Leftrightarrow2sinx.cosx-sinx-1=0\)
(Cái này tạm thời nghĩ ko ra,tối làm :)
Tìm tập nghiệm của phương trình: \(\dfrac{\sqrt[]{3}sin^2x-2sinxcosx-\sqrt{3}cos^2x}{\left(2sinx+3\right)\left(4cos^2x-3\right)}=0\)
ĐKXĐ: \(cos2x\ne\dfrac{1}{2}\Leftrightarrow x\ne\pm\dfrac{\pi}{6}+k\pi\)
\(\sqrt{3}sin^2x-2sinx.cosx-\sqrt{3}cos^2x=0\)
\(\Leftrightarrow-sin2x-\sqrt{3}\left(cos^2x-sin^2x\right)=0\)
\(\Leftrightarrow sin2x+\sqrt{3}cos2x=0\)
\(\Leftrightarrow\dfrac{1}{2}sin2x+\dfrac{\sqrt{3}}{2}cos2x=0\)
\(\Leftrightarrow sin\left(2x+\dfrac{\pi}{3}\right)=0\)
\(\Leftrightarrow2x+\dfrac{\pi}{3}=k\pi\)
\(\Leftrightarrow x=-\dfrac{\pi}{6}+\dfrac{k\pi}{2}\)
Nghiệm này bao gồm 2 họ nghiệm: \(\left[{}\begin{matrix}x=-\dfrac{\pi}{6}+k\pi\\x=\dfrac{\pi}{3}+k\pi\end{matrix}\right.\)
Do đó sau khi loại nghiệm theo ĐKXĐ ta được nghiệm của pt là: \(x=\dfrac{\pi}{3}+k\pi\)
Phương trình 3 cos 2 x − 2 sin x + 2 = 0 có nghiệm là
A. x = π 2 + k 2 π
B. x = − π 2 + k 2 π
C. x = π 3 + k 2 π x = − π 3 + k 2 π
D. x = π 6 + k 2 π x = − π 6 + k 2 π
Phương trình 3 cos 2 x -2sinx+2 = 0 co nghiệm là
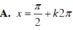
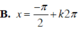
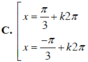
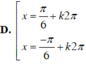
Nghiệm của phương trình 2 sin x ( cos x - 1 ) = 3 cos 2 x là:
A. π 3 + k 2 π , k ∈ ℤ hoặc 4 π 9 + k 2 π 3 , k ∈ ℤ
B. π 3 + k π , k ∈ ℤ hoặc 4 π 9 + k π 3 , k ∈ ℤ
C. - π 3 + k 2 π , k ∈ ℤ hoặc 2 π 9 + k 2 π 3 , k ∈ ℤ
D. - π 3 + k π , k ∈ ℤ hoặc 2 π 9 + k π 3 , k ∈ ℤ
a)\(4sin^3xcos3x+4cos^3xsin3x+3\sqrt{3}cos4x=3\)
b)\(2sin^2x\left(4sin^4x-1\right)=cos2x\left(7cos^22x+3cos2x-4\right)\)
2 câu này giải như nào ạ
a
\(\Leftrightarrow\left(3sinx-sin3x\right)cos3x+\left(3cosx+cos3x\right)sin3x+3\sqrt{3}cos4x=3\)
\(\Leftrightarrow\left(sinx.cos3x+sin3x.cosx\right)+\sqrt{3}cos4x=1\)
\(\Leftrightarrow sin4x+\sqrt{3}cos4x=1\)
Tới đây thôi, mình lười ghi rồi =))
b
\(\Leftrightarrow\left(1-cos2x\right)\left(2sin^2x-1\right)\left(2sin^2+1\right)=cos2x\left(7cos^22x+3cos2x-4\right)\)
\(\Leftrightarrow\left(1-cos2x\right)\left(-cos2x\right)\left(2-cos2x\right)=cos2x\left(7cos^22x+3cos2x+4\right)\)
\(\Leftrightarrow-cos^22x+3cos2x-2=7cos^22x+3cos2x+4\)
\(\Leftrightarrow4cos^22x+3=0\)
=> pt vô nghiệm
Giải phương trình:
\(\sqrt{18x-27}-\dfrac{1}{2}\sqrt{32x-48}+3\sqrt{\dfrac{16x-24}{2}}=1\)
\(ĐK:x\ge\dfrac{3}{2}\\ PT\Leftrightarrow3\sqrt{2x-3}-2\sqrt{2x-3}+6\sqrt{2x-3}=1\\ \Leftrightarrow7\sqrt{2x-3}=1\\ \Leftrightarrow\sqrt{2x-3}=\dfrac{1}{7}\\ \Leftrightarrow2x-3=\dfrac{1}{49}\Leftrightarrow x=\dfrac{74}{49}\left(tm\right)\)