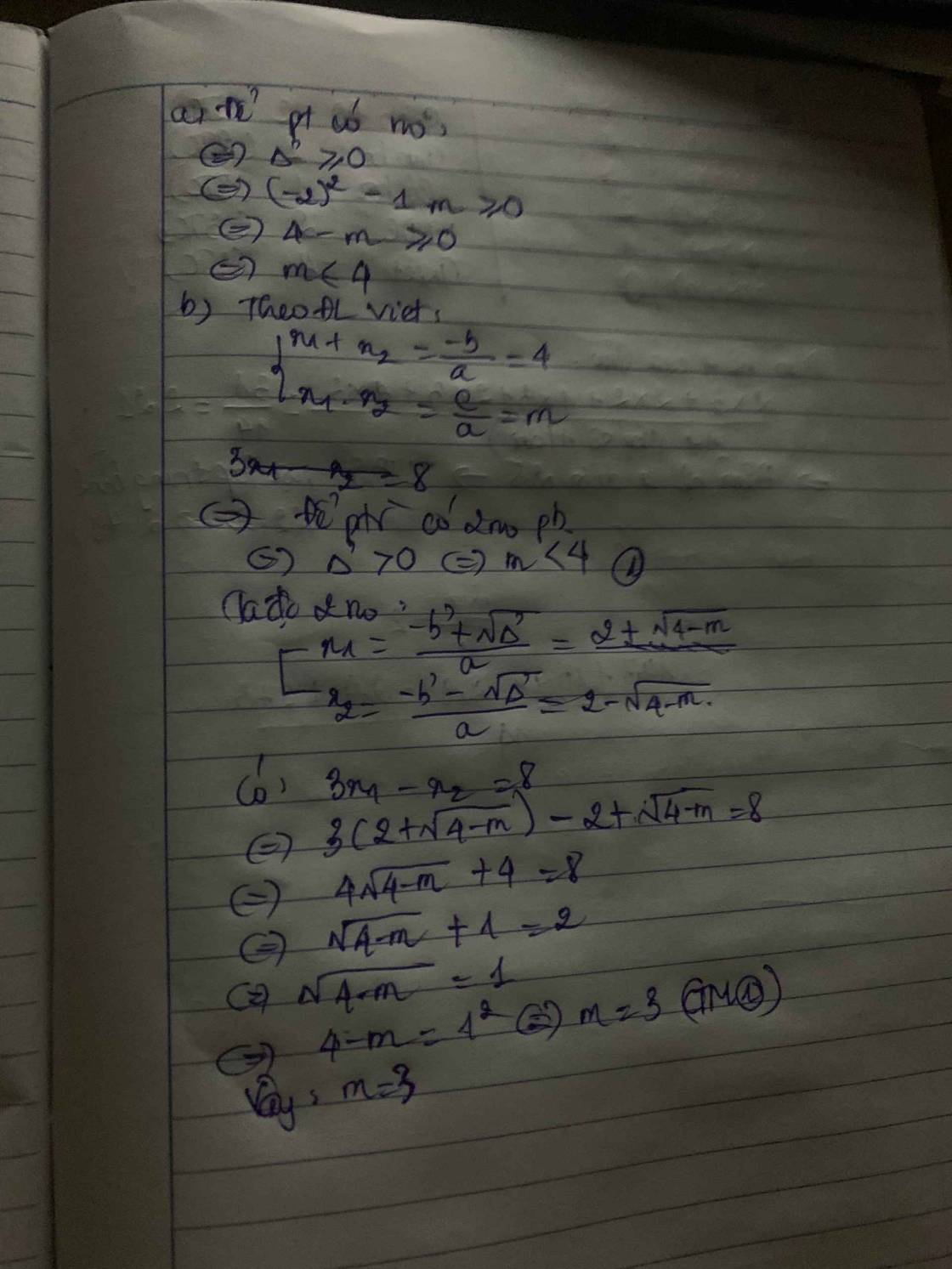Tìm m để pt sau có nghiệm: m.Sin^2(2x)+Cos(4x)=m

Những câu hỏi liên quan
Cho phương trình
m
.
sin
x
-
1
-
3
m
.
cos
x
m
-
2
. Tìm m để phương trình có nghiệm.
Đọc tiếp
Cho phương trình m . sin x - 1 - 3 m . cos x = m - 2 . Tìm m để phương trình có nghiệm.
![]()
![]()
![]()
![]()
Điều kiện của tham số m để phương trình m.sin x – 3.cos x 5 có nghiệm là
Đọc tiếp
Điều kiện của tham số m để phương trình m.sin x – 3.cos x = 5 có nghiệm là
![]()
![]()
![]()
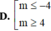
Tìm m để phương trình
2
sin
2
x
+
m
.
sin
2
x
2
m
vô nghiệm A.
m
0
;
m
≥
4
3
B.
m
≤
0
;
m
≥
4
3
C.
0
≤
m
≤...
Đọc tiếp
Tìm m để phương trình 2 sin 2 x + m . sin 2 x = 2 m vô nghiệm
A. m < 0 ; m ≥ 4 3
B. m ≤ 0 ; m ≥ 4 3
C. 0 ≤ m ≤ 4 3
D. m < 0 m > 4 3
Đáp án D
2 sin 2 x + m sin 2 x = 2 m ⇔ 1 − cos 2 x + m sin 2 x = 2 m ⇔ m sin 2 x − cos 2 x = 2 m − 1
Để phương trình vô nghiệm
⇔ 2 m − 1 2 > m 2 + 1
⇔ 3 m 2 − 4 m > 0 ⇔ m ∈ − ∞ ; 0 ∪ 4 3 ; + ∞
Đúng 0
Bình luận (0)
cho pt 2x^2=4x+5(m-1)=0. Tìm m để pt có 2 nghiệm phân biệt x1,x2 nhỏ hơn 3
B6:Tìm các giá trị của m để pt sau có nghiệm \(x_1x_2\) thỏa mãn; \(x_1^2+x_2^2+3x_1x_2=2\)
a)\(x^2-4x+m-1=0\)
b)\(x^2-2x+m-1=0\)
cho phương trình \(m^2\)x +6= 4x+3m a) giải pt khi m=3 b) tìm m để pt có nghiệm x= 1,5 c) tìm m để pt có nghiệm vô nghiệm vô số nghiệm d) tìm m nguyên để pt trên có nghiệm
a) Thay m=3 vào pt ta được:
\(9x+6=4x+9\Leftrightarrow x=\dfrac{3}{5}\)
Vậy...
b) Thay x=-1,5 vào pt ta được:
\(m^2\left(-1,5\right)+6=4.\left(-1,5\right)+3m\)
\(\Leftrightarrow\dfrac{-3}{2}m^2-3m+12=0\)\(\Leftrightarrow\left[{}\begin{matrix}m=2\\m=-4\end{matrix}\right.\)
Vậy...
c)Pt \(\Leftrightarrow x\left(m^2-4\right)=3m-6\)
Để pt vô nghiệm \(\Leftrightarrow\left\{{}\begin{matrix}3m-6\ne0\\m^2-4=0\end{matrix}\right.\)\(\Leftrightarrow\left\{{}\begin{matrix}m\ne2\\m=\pm2\end{matrix}\right.\)\(\Rightarrow m=-2\)
Để pt có vô số nghiệm \(\Leftrightarrow\left\{{}\begin{matrix}3m-6=0\\m^2-4=0\end{matrix}\right.\)\(\Leftrightarrow\left\{{}\begin{matrix}m=2\\m=\pm2\end{matrix}\right.\)\(\Rightarrow m=2\)
d)Để pt có nghiệm \(\Leftrightarrow m^2-4\ne0\Leftrightarrow m\ne\pm2\)
\(\Rightarrow x=\dfrac{3m-6}{m^2-4}=\dfrac{3\left(m-2\right)}{\left(m-2\right)\left(m+2\right)}=\dfrac{3}{m+2}\)
Để \(x\in Z\Leftrightarrow\dfrac{3}{m+2}\in Z\)
Vì \(m\in Z\Leftrightarrow m+2\in Z\).Để \(\dfrac{3}{m+2}\in Z\Leftrightarrow m+2\inƯ\left(3\right)=\left\{-1;-3;1;3\right\}\)
\(\Leftrightarrow m=\left\{-3;-5;-1;1\right\}\) (tm)
Vậy...
Đúng 3
Bình luận (0)
tìm m để pt có nghiệm thuộc \(\left[-1;1\right]\)
x^4+2x^3+5x^2+4x-1-m=0
\(x^4+2x^3+5x^2+4x-1-m=0\)
\(\Leftrightarrow\left(x^2+x\right)^2+4\left(x^2+x\right)-1-m=0\left(1\right)\)
\(đặt:x^2+x=t\ge\dfrac{-\Delta}{4a}=-\dfrac{1}{4}\)
\(\left(1\right)\Leftrightarrow t^2+4t-1-m=0\) có nghiệm trên \([-\dfrac{1}{4};\text{+∞})\)
\(f\left(t\right)=t^2+4t-1=m\)
\(f\left(-\dfrac{b}{2a}\right)=-5\)
\(f\left(-\dfrac{1}{4}\right)=-\dfrac{31}{16}\Rightarrow m\ge-\dfrac{31}{16}\Rightarrow\left[{}\begin{matrix}t=\dfrac{-b}{2a}=-2\Rightarrow x^2+x+2=0\left(vô-nghiệm\right)\left(loại\right)\\\left\{{}\begin{matrix}t1=\dfrac{-4+\sqrt{20+4m}}{2}=-2+\sqrt{5+m}\\t2=\dfrac{-4-\sqrt{20+4m}}{2}=-2-\sqrt{5+m}\end{matrix}\right.\end{matrix}\right.\)
\(x^2+x=t1=-2+\sqrt{5+m}\Leftrightarrow f\left(x\right)=x^2+x+2=\sqrt{5+m}\) có nghiệm thuộc \(\left[-1;1\right]\)
\(\Rightarrow f\left(-\dfrac{b}{2a}\right)=\dfrac{7}{4}\)
\(f\left(-1\right)=2;f\left(1\right)=4\)
\(\Rightarrow\dfrac{7}{4}\le\sqrt{5+m}\le4\Leftrightarrow\dfrac{-31}{16}\le m\le11\)
\(x^2+x=t2=-2-\sqrt{5+m}\Leftrightarrow f\left(x\right)=x^2+x+2=-\sqrt{5+m}\)
có nghiệm trên \(\left[-1;1\right]\)
\(x^2+x+2>0\Rightarrow x^2+x+2=-\sqrt{5+m}< 0\left(vô-lí\right)\Rightarrow vô-nghiệm\forall m\)
\(\Rightarrow\dfrac{-31}{16}\le m\le11\) thì pt có nghiệm thuộc \(\left[-1;1\right]\)
Đúng 0
Bình luận (0)
Cho pt x^2-4x+m=0 a)tìm m để pt có nghiệm b)tìm m để pt có nghiệm thỏa 3x1-x2=8
Tìm m để phương trình sau có nghiệm: msin²2x - 3sin2xcos2x + cos²2x = 2
\(\Leftrightarrow\dfrac{m}{2}\left(1-cos4x\right)-\dfrac{3}{2}sin4x+\dfrac{1+cos4x}{2}=2\)
\(\Leftrightarrow\left(1-m\right)cos4x-3sin4x=3-m\)
Theo điều kiện có nghiệm của pt lượng giác bậc nhất, pt đã cho có nghiệm khi:
\(\left(1-m\right)^2+\left(-3\right)^2\ge\left(3-m\right)^2\)
\(\Leftrightarrow4m+1\ge0\Leftrightarrow m\ge-\dfrac{1}{4}\)
Đúng 0
Bình luận (0)