Câu 1 đề sai, chắc chắn 1 trong 2 cái \(cot^2x\) phải có 1 cái là \(cos^2x\)

2.

\(\dfrac{1-sinx}{cosx}-\dfrac{cosx}{1+sinx}=\dfrac{\left(1-sinx\right)\left(1+sinx\right)-cos^2x}{cosx\left(1+sinx\right)}=\dfrac{1-sin^2x-cos^2x}{cosx\left(1+sinx\right)}\)

\(=\dfrac{1-\left(sin^2x+cos^2x\right)}{cosx\left(1+sinx\right)}=\dfrac{1-1}{cosx\left(1+sinx\right)}=0\)

3.

\(\dfrac{tanx}{sinx}-\dfrac{sinx}{cotx}=\dfrac{tanx.cotx-sin^2x}{sinx.cotx}=\dfrac{1-sin^2x}{sinx.\dfrac{cosx}{sinx}}=\dfrac{cos^2x}{cosx}=cosx\)

4.

\(\dfrac{tanx}{1-tan^2x}.\dfrac{cot^2x-1}{cotx}=\dfrac{tanx}{1-tan^2x}.\dfrac{\dfrac{1}{tan^2x}-1}{\dfrac{1}{tanx}}=\dfrac{tanx}{1-tan^2x}.\dfrac{1-tan^2x}{tanx}=1\)

5.

\(\dfrac{1+sin^2x}{1-sin^2x}=\dfrac{1+sin^2x}{cos^2x}=\dfrac{1}{cos^2x}+tan^2x=\dfrac{sin^2x+cos^2x}{cos^2x}+tan^2x\)

\(=tan^2x+1+tan^2x=1+2tan^2x\)

câu 1 : ta có : \(A=\left(sin^4x+cos^4x+sin^2x.cos^2x\right)^2-\left(sin^8x+cos^8x\right)\)

\(=\left(1-sin^2x.cos^2x\right)^2-\left(1-3sin^2x.cos^2x\right)\)

\(=\left(1-sin^2x.cos^2x\right)^2-\left(1-sin^2x.cos^2x\right)+2sin^2xcos^2x\)

\(=-sin^2x.cos^2x\left(1-sin^2x.cos^2x\right)+2sin^2x.cos^2x\)

\(=sin^2x.cos^2x\left(1+sin^2x.cos^2x\right)\)

tới đây mk xin sử dụng kiến thức lớp 10 một chút

\(=\dfrac{sin^22x}{4}\left(1+\dfrac{sin^22x}{4}\right)=\dfrac{sin^22x}{4}+\dfrac{sin^42x}{16}\)

vẩn phụ thuộc vào x \(\Rightarrow\) đề sai .

câu 1 : câu này bn có thể tìm trong trang của mk , mk nhớ đã làm nó rồi nhưng tìm hoài không đc . nếu đc bn có thể chờ mk đi hok về mk sẽ kiếm cho bn hoắc có thể là lm lại cho bn nha :)

câu 2 : https://hoc24.vn/hoi-dap/question/657072.html

câu 3 : https://hoc24.vn/hoi-dap/question/657069.html

câu 4 : https://hoc24.vn/hoi-dap/question/656635.html

câu 5 : https://hoc24.vn/hoi-dap/question/657071.html

a.

Thực hiện phép biến đổi tương đương:

\(\dfrac{sinx+cosx-1}{1-cosx}=\dfrac{2cosx}{sinx-cosx+1}\)

\(\Leftrightarrow\left(sinx+cosx-1\right)\left(sinx-cosx+1\right)=2cosx\left(1-cosx\right)\)

\(\Leftrightarrow sin^2x-\left(cosx-1\right)^2=2cosx-2cos^2x\)

\(\Leftrightarrow sin^2x-cos^2x+2cosx-1=2cosx-2cos^2x\)

\(\Leftrightarrow1-cos^2x-cos^2x-1=-2cos^2x\)

\(\Leftrightarrow-2cos^2x=-2cos^2x\) (luôn đúng)

Vậy đẳng thức đã cho được chứng minh

b.

\(cot^2x-cos^2x=\dfrac{cos^2x}{sin^2x}-cos^2x=cos^2x\left(\dfrac{1}{sin^2x}-1\right)=\dfrac{cos^2x\left(1-sin^2x\right)}{sin^2x}=cot^2x.cos^2x\)

a: tan x(cot^2x-1)

\(=\dfrac{1}{cotx}\left(cot^2x-cotx\cdot tanx\right)\)

=cotx-tanx/cotx=cotx(1-tan^2x)

b: \(tan^2x-sin^2x=\dfrac{sin^2x}{cos^2x}-sin^2x\)

\(=sin^2x\left(\dfrac{1}{cos^2x}-1\right)=sin^2x\cdot\dfrac{sin^2x}{cos^2x}=sin^2x\cdot tan^2x\)

c: \(\dfrac{cos^2x-sin^2x}{cot^2x-tan^2x}=\dfrac{cos^2x-sin^2x}{\dfrac{cos^2x}{sin^2x}-\dfrac{sin^2x}{cos^2x}}\)

\(=\left(cos^2x-sin^2x\right):\dfrac{cos^4x-sin^4x}{sin^2x\cdot cos^2x}\)

\(=\dfrac{sin^2x\cdot cos^2x}{1}=sin^2x\cdot cos^2x\)

=>sin^2x*cos^2x-cos^2x=cos^2x(sin^2x-1)

=-cos^2x*cos^2x=-cos^4x

=>ĐPCM

1: \(=\dfrac{cotx+1+tanx+1}{\left(tanx+1\right)\left(cotx+1\right)}\)

\(=\dfrac{\dfrac{1}{cotx}+cotx+2}{2+tanx+cotx}\)

\(=1\)

2: \(VT=\dfrac{cos^2x+cosxsinx+sin^2x-sinx\cdot cosx}{sin^2x-cos^2x}\)

\(=\dfrac{1}{sin^2x-cos^2x}\)

\(VP=\dfrac{1+cot^2x}{1-cot^2x}=\left(1+\dfrac{cos^2x}{sin^2x}\right):\left(1-\dfrac{cos^2x}{sin^2x}\right)\)

\(=\dfrac{1}{sin^2x}:\dfrac{sin^2x-cos^2x}{sin^2x}=\dfrac{1}{sin^2x-cos^2x}\)

=>VT=VP

Bạn kiểm tra lại đề bài câu 1, câu này chỉ có thể rút gọn đến \(2cot^2x+2cotx+1\) nên biểu thức ko hợp lý

Đồng thời kiểm tra luôn đề câu 2, trong cả 2 căn thức đều xuất hiện \(6sin^2x\) rất không hợp lý, chắc chắn phải có 1 cái là \(6cos^2x\)

Câu 1 đề vẫn có vấn đề:

\(=\dfrac{1+cotx}{1-cotx}-\dfrac{2\left(1+cot^2x\right)cot^2x}{\left(tanx-1\right)\left(tan^2x+1\right)cot^2x}=\dfrac{1+cotx}{1-cotx}-\dfrac{2cot^2x}{tanx-1}\)

\(=\dfrac{1+cotx}{1-cotx}-\dfrac{2cot^3x}{1-cotx}=\dfrac{1+cotx-2cot^3x}{1-cotx}\)

\(=\dfrac{\left(1-cotx\right)\left(1+2cotx+2cot^2x\right)}{1-cotx}=1+2cotx+2cot^2x\)

Có thể coi như ko thể rút gọn tiếp

2.

\(\sqrt{\left(1-cos^2x\right)^2+6cos^2x+3cos^4x}+\sqrt{\left(1-sin^2x\right)^2+6sin^2x+3sin^4x}\)

\(=\sqrt{4cos^4x+4cos^2x+1}+\sqrt{4sin^4x+4sin^2x+1}\)

\(=\sqrt{\left(2cos^2x+1\right)^2}+\sqrt{\left(2sin^2x+1\right)^2}\)

\(=2\left(cos^2x+sin^2x\right)+2=4\)

b)

(sin2x + cos2x)cosx + 2cos2x - sinx = 0

⇔ cos2x (cosx + 2) + sinx (2cos2 x – 1) = 0

⇔ cos2x (cosx + 2) + sinx.cos2x = 0

⇔ cos2x (cosx + sinx + 2) = 0

⇔ cos2x  = 0

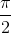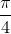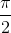⇔ 2x =  + kπ ⇔ x =  + k  (k ∈ )

c) 

Đáp án:

x=+ k2

và x= +k2 (k∈Z)

Lời giải:

sin2x-cos2x+3sinx-cosx-1=0

⇔ 2sinxcosx-(1-2sin²x) +3sinx-cosx-1=0

⇔ 2sin²x+2sinxcosx+3sinx-cosx-2=0

⇔ (2sin²x+3sinx-2)+ cosx(2sinx-1)=0

⇔ (2sinx-1)(sinx+2)+cosx(2sinx-1)=0

⇔ (2sinx-1)(sinx+cosx+2)=0

⇔ sinx=

⇔ x=+ k2

hoặc x= +k2 (k∈Z)

(sinx+cosx+2)=0 (vô nghiệm do sinx+cosx+2=sin(x+)+2>0)

Nhầm, câu c