Giải phương trình 9(x+2)-3x(x+2) = 0

Những câu hỏi liên quan
Bài 1: Giải phương trình và bất phương trình sau: 1. 5.(2-3x). (x-2) = 3.( 1-3x) 2. 4x^2 + 4x + 1= 0 3. 4x^2 - 9= 0 4. 5x^2 - 10=0 5. x^2 - 3x= -2 6. |x-5| - 3= 0
giải phương trình:
\(\left(x^2-3x-9\right)^2-\left(3x-17\right)^2=0\)
\(\Leftrightarrow\left(x^2-3x-9-3x+17\right)\left(x^2-3x-9+3x-17\right)=0\)
\(\Leftrightarrow\left(x^2-6x+8\right)\left(x^2-26\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x^2-6x+8=0\\x^2-26=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x_1=4;x_2=2\\x^2=26\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x_1=4;x_2=2\\x=\sqrt{26}\end{matrix}\right.\)
Vậy \(S=\left\{4;2;\sqrt{26}\right\}\)
Đúng 1
Bình luận (1)
Giúp e với ạ Giải bất phương trình: (x ^ 2 - 3x + 2)/(9 - x ^ 2) >0
\(\Leftrightarrow\dfrac{\left(x-1\right)\left(x-2\right)}{\left(3-x\right)\left(3+x\right)}>0\)
Bảng xét dấu:
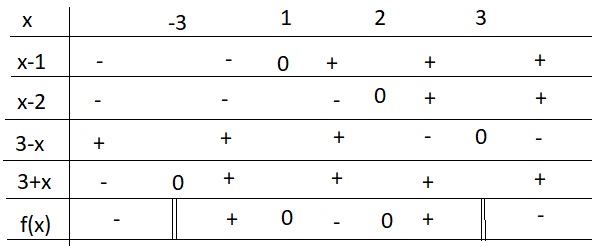
Từ bảng xét dấu ta có nghiệm của BPT là: \(x\in\left(-3;1\right)\cup\left(2;3\right)\)
Đúng 2
Bình luận (0)
Giải Phương trình
\(x-\dfrac{x+2}{3}< 3x+\dfrac{x}{2}+5
\)
\(\dfrac{x}{2}+\dfrac{1-x}{3}>0\)
\(\left(x-9\right)^2-x\left(x+9\right)< 0\)
a. \(x-\dfrac{x+2}{3}< 3x+\dfrac{x}{2}+5\)
\(\Leftrightarrow\dfrac{6x}{6}-\dfrac{2\left(x+2\right)}{6}< \dfrac{18x}{6}+\dfrac{3x}{6}+\dfrac{30}{6}\)
\(\Rightarrow6x-2x-4-18x-3x-30< 0\)
\(\Leftrightarrow-17x< 34\)
\(\Leftrightarrow x>-2\)
b. \(\dfrac{x}{2}+\dfrac{1-x}{3}>0\)
\(\Leftrightarrow3x+2-2x>0\)
\(\Leftrightarrow x>-2\)
c. \(\left(x-9\right)^2-x\left(x+9\right)< 0\)
\(\Leftrightarrow x^2-18x+81-x^2-9x< 0\)
\(\Leftrightarrow-27x< -81\)
\(\Leftrightarrow x>3\)
Đúng 2
Bình luận (0)
Giải bất phương trình
a) 3x2-3x(-2+x)<hoặc= 36
b) (x+2)2-9>0
`a,3x^2-3x(-2+x) <= 36`
`<=> 3x^2 + 6x -3x^2 <= 36`
`<=> 6x <= 36`
`<=> x <= 6`
Vậy bpt đã cho có tập nghiệm `x <= 6`
`b, (x+2)^2 -9>0`
`<=> (x+2)^2 > 9`
`<=>(x+2)^2 > 3^2`
`<=> x+2> +- 3`
`<=> x>1;-5`
Vậy bpt đã cho có tập nghiệm `x>1` hoặc `x> -5`
Đúng 2
Bình luận (0)
a: =>3x^2+6x-3x^2<=36
=>6x<=36
=>x<=6
b: =>(x-1)(x+5)>0
=>x>1 hoặc x<-5
Đúng 0
Bình luận (0)
a) \(2\left(x^2-2x\right)+\sqrt{x^2-2x-3}-9=0\)
b) \(3\sqrt{2+x}-6\sqrt{2-x}+4\sqrt{4-x^2}=10-3x\)
c) Cho phương trình: \(\sqrt{x}+\sqrt{9-x}=\sqrt{-x^2+9x+m}\)
+) Giải phương trình khi m=9
+) Tìm m để phương trình có nghiệm
a, ĐK: \(x\le-1,x\ge3\)
\(pt\Leftrightarrow2\left(x^2-2x-3\right)+\sqrt{x^2-2x-3}-3=0\)
\(\Leftrightarrow\left(2\sqrt{x^2-2x-3}+3\right).\left(\sqrt{x^2-2x-3}-1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}\sqrt{x^2-2x-3}=-\dfrac{3}{2}\left(l\right)\\\sqrt{x^2-2x-3}=1\end{matrix}\right.\)
\(\Leftrightarrow x^2-2x-3=1\)
\(\Leftrightarrow x^2-2x-4=0\)
\(\Leftrightarrow x=1\pm\sqrt{5}\left(tm\right)\)
Đúng 1
Bình luận (0)
b, ĐK: \(-2\le x\le2\)
Đặt \(\sqrt{2+x}-2\sqrt{2-x}=t\Rightarrow t^2=10-3x-4\sqrt{4-x^2}\)
Khi đó phương trình tương đương:
\(3t-t^2=0\)
\(\Leftrightarrow\left[{}\begin{matrix}t=0\\t=3\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}\sqrt{2+x}-2\sqrt{2-x}=0\\\sqrt{2+x}-2\sqrt{2-x}=3\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}2+x=8-4x\\2+x=17-4x+12\sqrt{2-x}\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{6}{5}\left(tm\right)\\5x-15=12\sqrt{2-x}\left(1\right)\end{matrix}\right.\)
Vì \(-2\le x\le2\Rightarrow5x-15< 0\Rightarrow\left(1\right)\) vô nghiệm
Vậy phương trình đã cho có nghiệm \(x=\dfrac{6}{5}\)
Đúng 1
Bình luận (0)
c, ĐK: \(0\le x\le9\)
Đặt \(\sqrt{9x-x^2}=t\left(0\le t\le\dfrac{9}{2}\right)\)
\(pt\Leftrightarrow9+2\sqrt{9x-x^2}=-x^2+9x+m\)
\(\Leftrightarrow-\left(-x^2+9x\right)+2\sqrt{9x-x^2}+9=m\)
\(\Leftrightarrow-t^2+2t+9=m\)
Khi \(m=9,pt\Leftrightarrow-t^2+2t=0\Leftrightarrow\left[{}\begin{matrix}t=0\\t=2\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}9x-x^2=0\\9x-x^2=4\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=0\left(tm\right)\\x=9\left(tm\right)\\x=\dfrac{9\pm\sqrt{65}}{2}\left(tm\right)\end{matrix}\right.\)
Phương trình đã cho có nghiệm khi phương trình \(m=f\left(t\right)=-t^2+2t+9\) có nghiệm
\(\Leftrightarrow minf\left(t\right)\le m\le maxf\left(t\right)\)
\(\Leftrightarrow-\dfrac{9}{4}\le m\le10\)
Đúng 1
Bình luận (0)
Bàil: Giải phương trình sau a) 2x - 3 3 - x b) 7x - 4 3x + 12 c) 3x - 6 + x 9 - x d) 10x - 12 - 3x 6 + x Bài 2: Giải bất phương trình sau và biểu diễn tập nghiệm trên trục số: a) 4x + 6 2x - 2 b) 3x + 15 0 c) 3x - 3 x + 5 d) x - 4 - 2x + 5 Bài3: a) Một người đi xe máy từ 4 đến B với vận tốc 25km/h. Lúc về người đó đi với vận tốc 30km/h, nên thời gian về ít hơn thời gian đi là 20 phút. Tính AB ? b) Một người đi xe đạp từ A đến B với vận tốc 15 km/h. Sau đó quay về từ B về A với vận tốc 12...
Đọc tiếp
Bàil: Giải phương trình sau a) 2x - 3 = 3 - x b) 7x - 4 = 3x + 12 c) 3x - 6 + x = 9 - x d) 10x - 12 - 3x = 6 + x Bài 2: Giải bất phương trình sau và biểu diễn tập nghiệm trên trục số: a) 4x + 6 <= 2x - 2 b) 3x + 15 < 0 c) 3x - 3 > x + 5 d) x - 4 > - 2x + 5 Bài3: a) Một người đi xe máy từ 4 đến B với vận tốc 25km/h. Lúc về người đó đi với vận tốc 30km/h, nên thời gian về ít hơn thời gian đi là 20 phút. Tính AB ? b) Một người đi xe đạp từ A đến B với vận tốc 15 km/h. Sau đó quay về từ B về A với vận tốc 12 km/h. Cả đi lẫn về hết 4 giờ 30 phút. Tính quãng đường 4B Bài 4: Cho tam giác ABC vuông tại A với AB = 3cm AC= 4cm vẽ đường cao AE. a) Chứng minh rằng AABC đồng dạng với AEBA. b) Tia phân giác của góc ABC cắt AC tại F. Tính BF Bài 5: Cho tam giác ABC có AC = 8cm, AC = 16cm Gọi D và E là hai điểm lần lượt trên cạnh AB và AC sao cho BD = 2cm CE = 13cm Chứng minh rằng a. AAEB AADC b. AED= ABC, cho DE = 5cm Tính BC? C. AE AC AD AB
1:
a: =>3x=6
=>x=2
b: =>4x=16
=>x=4
c: =>4x-6=9-x
=>5x=15
=>x=3
d: =>7x-12=x+6
=>6x=18
=>x=3
2:
a: =>2x<=-8
=>x<=-4
b: =>x+5<0
=>x<-5
c: =>2x>8
=>x>4
Đúng 0
Bình luận (0)
giải phương trình sau:
a)\(\sqrt{x^2-9}\) - 3\(\sqrt{x-3}\) =0 b)\(\sqrt{4x^2-12x+9}\) =x - 3
c)\(\sqrt{x^2+6x+9}\) =3x-1
a)√x2−9 - 3√x−3 =0
<=> (√x-3)(√x+3)-3√x-3=0
<=> (√x-3)(√x+3-3)=0
<=> (√x-3)√x=0
<=> √x-3=0
<=>x=9
b)√4x2−12x+9=x - 3
<=> √(2x -3)2 =x-3
<=> 2x-3=x-3
<=>2x-x=-3+3
<=>x=0
c)√x2+6x+9=3x-1
<=> √(x+3)2 =3x-1
<=> x+3=3x-1
<=> -2x=-4
<=> x=2
Nhớ cho mình 1 tim nha bạn
Đúng 2
Bình luận (1)
Lời giải:
a. ĐKXĐ: $x\geq 3$
PT $\Leftrightarrow \sqrt{(x-3)(x+3)}-3\sqrt{x-3}=0$
$\Leftrightarrow \sqrt{x-3}(\sqrt{x+3}-3)=0$
$\Leftrightarrow \sqrt{x-3}=0$ hoặc $\sqrt{x+3}-3=0$
$\Leftrightarrow \sqrt{x-3}=0$ hoặc $\sqrt{x+3}=3$
$\Leftrightarrow x=3$ hoặc $x=6$ (tm)
b.
PT \(\Rightarrow \left\{\begin{matrix} x-3\geq 0\\ 4x^2-12x+9=(x-3)^2=x^2-6x+9\end{matrix}\right.\Leftrightarrow \left\{\begin{matrix} x\geq 3\\ 3x^2-6x=0\end{matrix}\right.\)
\(\Leftrightarrow \left\{\begin{matrix} x\geq 3\\ 3x(x-2)=0\end{matrix}\right.\)
$\Rightarrow$ không có giá trị $x$ nào thỏa mãn
Vậy pt vô nghiệm.
c.
PT \(\Rightarrow \left\{\begin{matrix} 3x-1\geq 0\\ x^2+6x+9=(3x-1)^2\end{matrix}\right.\Leftrightarrow \left\{\begin{matrix} x\geq \frac{1}{3}\\ x^2+6x+9=9x^2-6x+1\end{matrix}\right.\)
\(\Leftrightarrow \left\{\begin{matrix} x\geq \frac{1}{3}\\ 8x^2-12x-8=0\end{matrix}\right.\Leftrightarrow \left\{\begin{matrix} x\geq \frac{1}{3}\\ 4(x-2)(2x+1)=0\end{matrix}\right.\Leftrightarrow x=2\)
Đúng 0
Bình luận (0)
Giải các bất phương trình sau
a) 3x2-3x(-2+x) < hoặc = 36
b) (x+2)2-9 > 0
\(a,3x^2-3x\left(-2+x\right)\le36\)
\(\Leftrightarrow3x^2+6x-3x^2-36\le0\)
\(\Leftrightarrow6x\le36\)
\(\Leftrightarrow x\le6\)
\(b,\left(x+2\right)^2-9>0\)
\(\Leftrightarrow\left(x+2\right)^2-3^2>0\)
\(\Leftrightarrow\left(x+2-3\right)\left(x+2+3\right)>0\)
\(\Leftrightarrow\left\{{}\begin{matrix}x-1>0\\x+5>0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x>1\\x>-5\end{matrix}\right.\)
Đúng 2
Bình luận (0)
b: =>(x+2-3)(x+2+3)>0
=>(x+5)(x-1)>0
=>x-1>0 hoặc x+5<0
=>x>1 hoặc x<-5
Đúng 0
Bình luận (0)






















