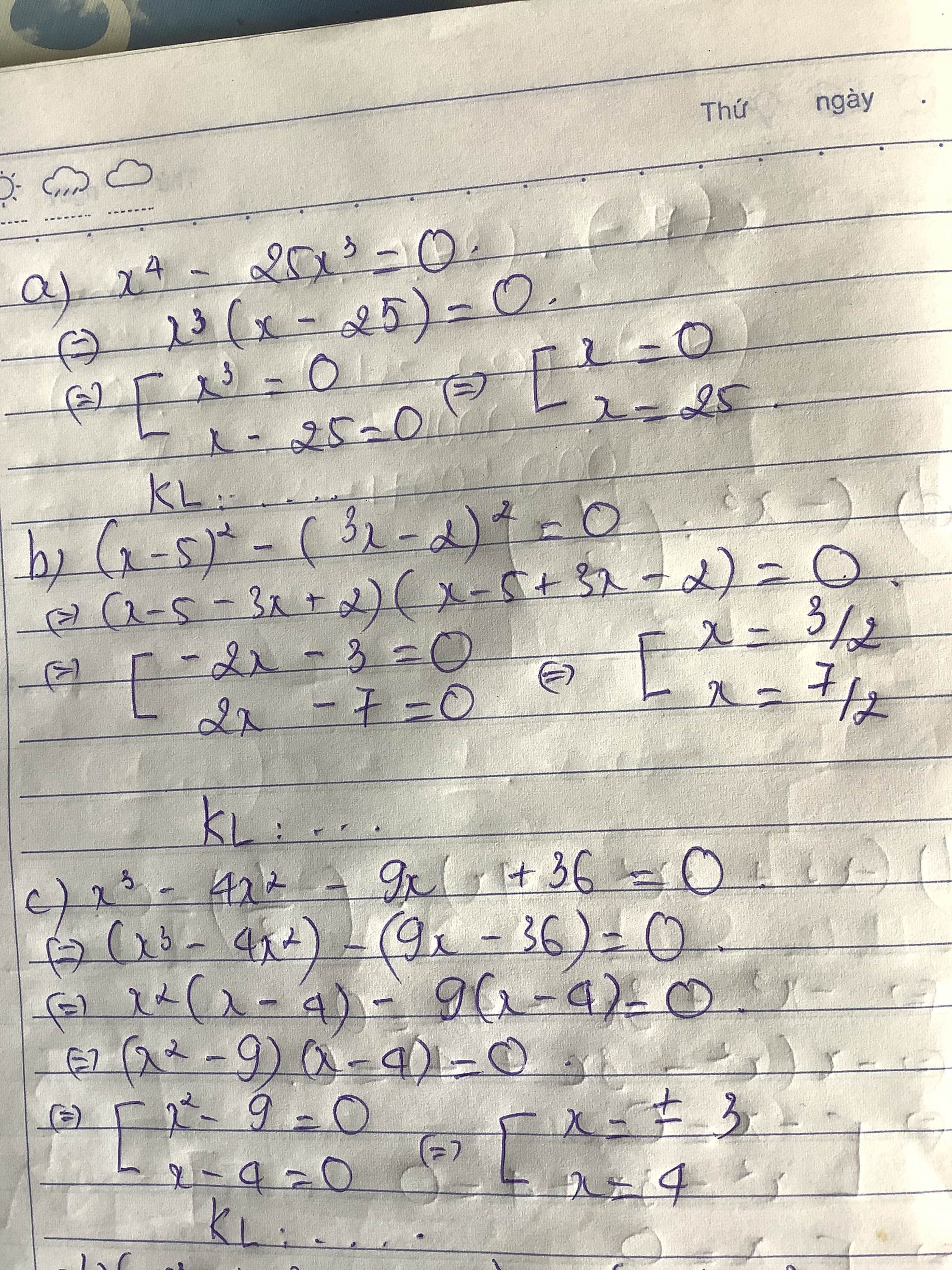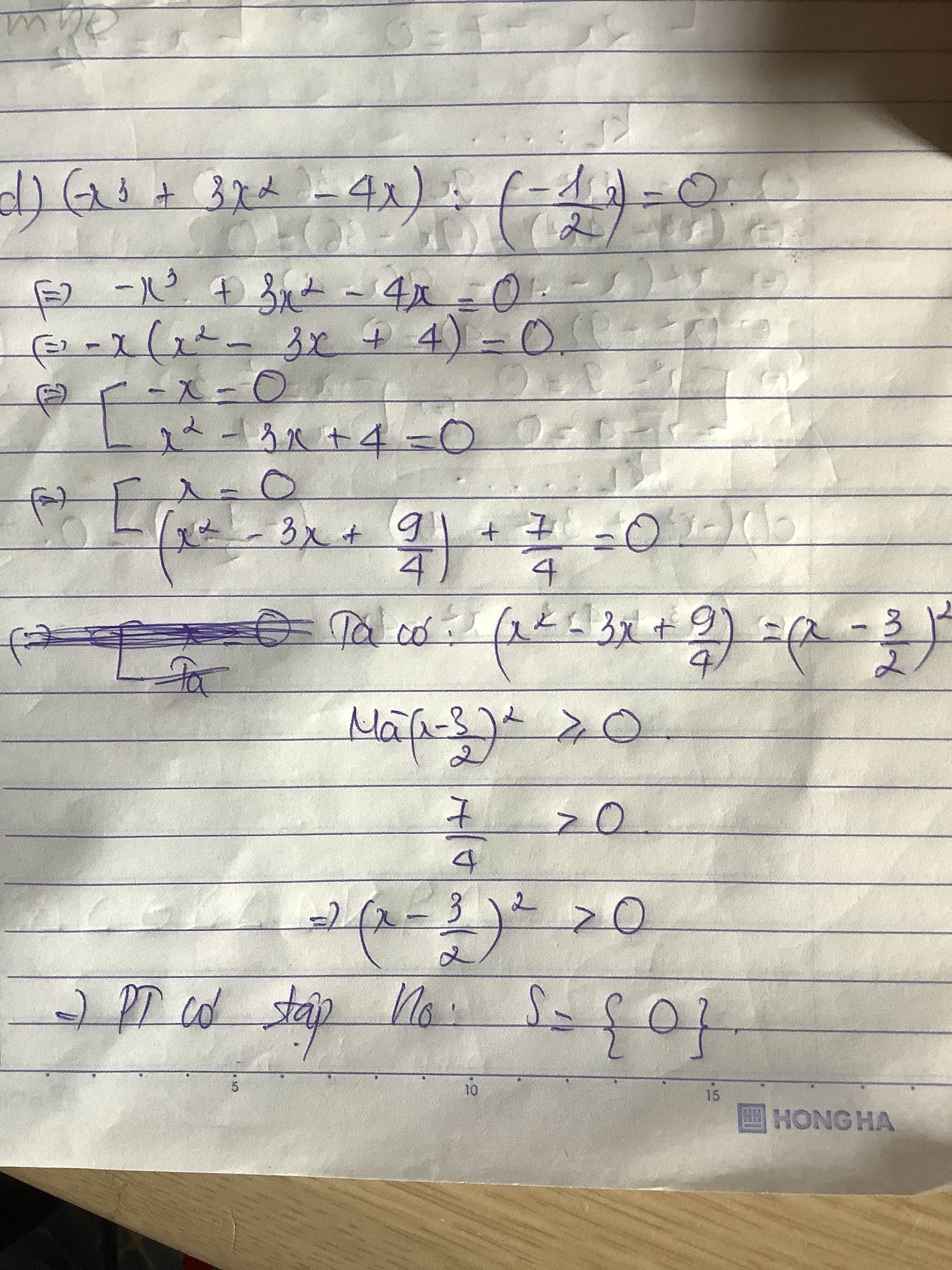cho mik hỏi rằng là 3x2 + 4x = 0 hay  3x² + 4x = 0

ông ơi mấy bài này bấm máy tính là ra mà ông

a) \(3x^2+4x=0\Leftrightarrow\left(3x+4\right)x=0\Leftrightarrow\left[{}\begin{matrix}x=0\\3x+4=0\Leftrightarrow x=-\dfrac{4}{3}\end{matrix}\right.\)

   ➤\(x\in\left\{0;-\dfrac{4}{3}\right\}\)

b) \(-2x^2-8=0\Leftrightarrow-2x^2+\left(-2\right)\cdot4=0\)

                           \(\Leftrightarrow\left(x^2+4\right)\cdot\left(-2\right)=0\\ \Leftrightarrow x^2+4=0\\\Rightarrow x^2=\varnothing\Leftrightarrow x=\varnothing \) 

                          vì với mọi x, ta luôn đúng với: \(x^2\ge0\Leftrightarrow x^2+4\ge4>0\)

➤\(x=\varnothing\)

c)\(2x^2-7x^2+5=0\)

+) \(a+b+c=2+\left(-7\right)+5=7-7=0\)

Do đó, phương trình có 2 nghiệm sau:

\(x=1\) và \(x=\dfrac{5}{2}=2,5\)

➤\(x\in\left\{1;2,5\right\}\)

d) \(x^2-8x-48=0\)

+)\(\Delta=\left(-8\right)^2-4\cdot1\cdot\left(-48\right)=64+192=266>0\)

\(\Leftrightarrow\sqrt{\Delta}=\sqrt{266}\)

➢Do đó, ta có: \(\left[{}\begin{matrix}x=\dfrac{\sqrt{266}-\left(-8\right)}{2\cdot2}=\dfrac{\sqrt{266}+8}{4}\\x=\dfrac{-\sqrt{266}-\left(-8\right)}{2\cdot2}=\dfrac{8-\sqrt{266}}{4}\end{matrix}\right.\)

➤ \(x\in\left\{\dfrac{8+\sqrt{266}}{4};\dfrac{8-\sqrt{266}}{4}\right\}\)

\(x^2-4x-3=0\)

Theo Vi-ét, ta có :
\(\left\{{}\begin{matrix}x_1+x_2=-\dfrac{b}{a}=4\\x_1x_2=\dfrac{c}{a}=-3\end{matrix}\right.\)

Ta có :

\(B=3x_1^2+3x_2^2-5x_1x_2\)

\(=3\left(x_1^2+x_2^2\right)-5x_1x_2\)

\(=3\left[\left(x_1+x_2\right)^2-2x_1x_2\right]-5x_1x_2\)

\(=3[4^2-2.\left(-3\right)]-5.\left(-3\right)\)

\(=81\)

a: 3x^2-4x+1=0

a=3; b=-4; c=1

Vì a+b+c=0 nên phương trình có hai nghiệm là:

x1=1 và x2=c/a=1/3

b: -x^2+6x-5=0

=>x^2-6x+5=0

a=1; b=-6; c=5

Vì a+b+c=0 nên phương trình có hai nghiệm là;
x1=1; x2=5/1=5

\(x^3-3x^2-4x+12=0\Leftrightarrow\left(x+2\right)\left(x-3\right)\left(x-2\right)=0\)

\(\Leftrightarrow\left[{}\begin{matrix}x=-2\\x=3\\x=2\end{matrix}\right.\)

\(x^3-3x^2-4x+12=0\)

\(\Leftrightarrow x^2\left(x-3\right)-4\left(x-3\right)=0\)

\(\Leftrightarrow\left(x-3\right)\left(x-2\right)\left(x+2\right)=0\)

\(\Leftrightarrow\left[{}\begin{matrix}x=3\\x=2\\x=-2\end{matrix}\right.\)

a.

$x^4-25x^3=0$

$\Leftrightarrow x^3(x-25)=0$

\(\Leftrightarrow \left[\begin{matrix} x^3=0\\ x-25=0\end{matrix}\right.\Leftrightarrow \left[\begin{matrix} x=0\\ x=25\end{matrix}\right.\)

b.

$(x-5)^2-(3x-2)^2=0$

$\Leftrightarrow (x-5-3x+2)(x-5+3x-2)=0$

$\Leftrightarrow (-2x-3)(4x-7)=0$
\(\Leftrightarrow \left[\begin{matrix} -2x-3=0\\ 4x-7=0\end{matrix}\right.\Leftrightarrow \left[\begin{matrix} x=\frac{-3}{2}\\ x=\frac{7}{4}\end{matrix}\right.\)

c.

$x^3-4x^2-9x+36=0$

$\Leftrightarrow x^2(x-4)-9(x-4)=0$

$\Leftrightarrow (x-4)(x^2-9)=0$

$\Leftrightarrow (x-4)(x-3)(x+3)=0$

\(\Leftrightarrow \left[\begin{matrix} x-4=0\\ x-3=0\\ x+3=0\end{matrix}\right.\Leftrightarrow \left[\begin{matrix} x=4\\ x=3\\ x=-3\end{matrix}\right.\)

d. ĐK: $x\neq 0$

$(-x^3+3x^2-4x):(\frac{-1}{2}x)=0$

$\Leftrightarrow x(-x^2+3x-4):(\frac{-1}{2}x)=0$

$\Leftrightarrow -2(-x^2+3x-4)=0$

$\Leftrightarrow x^2-3x+4=0$

$\Leftrightarrow (x-1,5)^2=-1,75< 0$ (vô lý)

Vậy pt vô nghiệm.

\(PT\Leftrightarrow\left(\sqrt{4x^2-20x+28}-2\right)=3x^2-15x+18\\ \Leftrightarrow\dfrac{4x^2-20x+24}{\sqrt{4x^2-20x+28}+2}=3\left(x-2\right)\left(x-3\right)\\ \Leftrightarrow\dfrac{4\left(x-2\right)\left(x-3\right)}{\sqrt{4x^2-20x+28}+2}-3\left(x-2\right)\left(x-3\right)=0\\ \Leftrightarrow\left(x-2\right)\left(x-3\right)\left(\dfrac{4}{\sqrt{4x^2-20x+28}+2}-3\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x=2\\x=3\\\dfrac{4}{\sqrt{4x^2-20x+28}+2}-3=0\left(1\right)\end{matrix}\right.\)

Vì \(\dfrac{4}{\sqrt{4x^2-20x+28}+2}\le2\Leftrightarrow\dfrac{4}{\sqrt{4x^2-20x+28}+2}-3\le-1< 0\)

Do đó \(\left(1\right)\) vô nghiệm

Vậy PT có nghiệm \(x=2;x=3\)

Lời giải:

ĐKXĐ: $x\geq \frac{1}{2}$

PT $\Leftrightarrow (3x^2-10x-25)=2(x+3)(\sqrt{2x-1}-3)$

$\Leftrightarrow (x-5)(3x+5)=2(x+3).\frac{2(x-5)}{\sqrt{2x-1}+3}$

\(\Leftrightarrow (x-5)\left[(3x+5)-\frac{4(x+3)}{\sqrt{2x-1}+3}\right]=0\)

Xét biểu thức trong ngoặc vuông:

\(\Leftrightarrow (3x+5)(\sqrt{2x-1}+3)=4(x+3)\)

\(\Leftrightarrow (3x+5)\sqrt{2x-1}=-(3+5x)\)

Dễ thấy điều này vô lý vì với $x\geq \frac{1}{2}$ thì vế trái không âm còn vế phải âm.

Vậy $x-5=0\Leftrightarrow x=5$

\(3x^2-2\left(x^2+4x\right)+3x+2=0\)

\(\Leftrightarrow3x^2-2x^2-8x+3x+2=0\)

\(\Leftrightarrow x^2-5x+2=0\)

a.

\(\Leftrightarrow\left(x-1\right)^3=10^3\)

\(\Leftrightarrow x-1=10\)

\(\Rightarrow x=11\)

b.

\(\Leftrightarrow x^2-4x+4=25\)

\(\Leftrightarrow\left(x-2\right)^2=5^2\)

\(\Leftrightarrow\left[{}\begin{matrix}x-2=5\\x-2=-5\end{matrix}\right.\)

\(\Leftrightarrow\left[{}\begin{matrix}x=7\\x=-3\end{matrix}\right.\)

a) x³-3x²+3x-1=1000

⇒(x-1)³=1000

⇒x-1=10

⇒x=11

b) x²-4x-21=0

⇒ x²-7x+3x-21=0

⇒x(x-7)+3(x-7)=0

⇒(x+3)(x-7)=0

⇒ hoặc x+3 = 0 ⇒ x=-3

hoặc x-7=0⇒x=7

vậy x={-3; 7}

a) Có: \(x^3-3x^2.1+3.1^2x-1^3=1000\)

          \(\Rightarrow\left(x-3\right)^3=1000\)

          \(\Rightarrow\left[{}\begin{matrix}x-3=10\\x-3=-10\end{matrix}\right.\)\(\Rightarrow\left[{}\begin{matrix}x=13\\x=-7\end{matrix}\right.\)

b) Có: \(x^2-4x+4-25=0\)

     \(\Rightarrow\left(x-2\right)^2=25\)

     \(\Rightarrow\left[{}\begin{matrix}x-2=5\\x-2=-5\end{matrix}\right.\Rightarrow\left[{}\begin{matrix}x=7\\x=-3\end{matrix}\right.\)