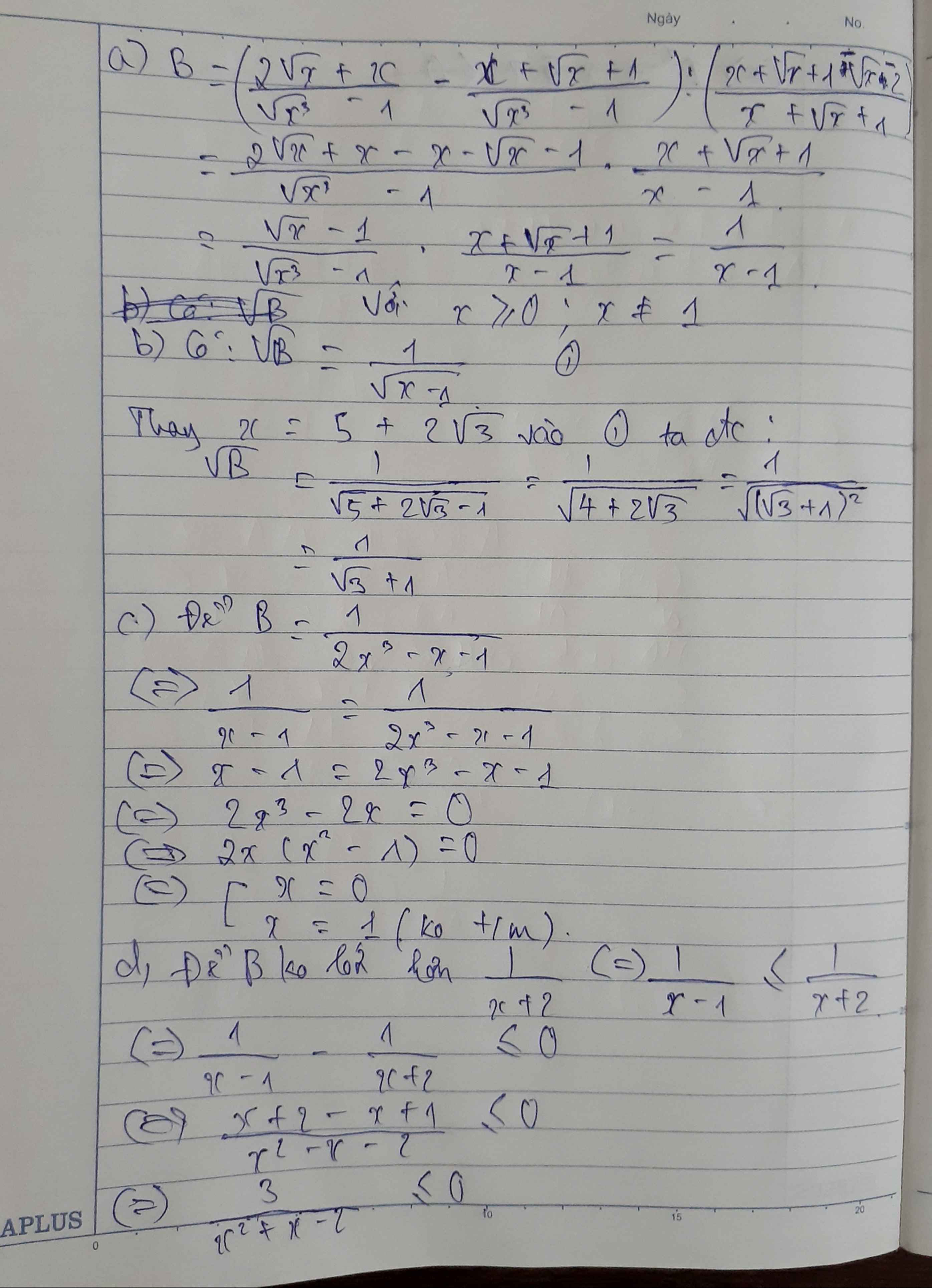Tìm x để A > B \(2\sqrt{x}+5\)

Những câu hỏi liên quan
Tìm x để A>B \(2\sqrt{x}+5\) biết \(A=\dfrac{\sqrt{x}+2}{\sqrt{x}-5}\) và \(B=\dfrac{1}{\sqrt{x}-5}\)
ĐKXĐ: \(\left\{{}\begin{matrix}x>=0\\x< >25\end{matrix}\right.\)
\(A>B\left(2\sqrt{x}+5\right)\)
=>\(\dfrac{\sqrt{x}+2}{\sqrt{x}-5}>=\dfrac{2\sqrt{x}+5}{\sqrt{x}-5}\)
=>\(\dfrac{\sqrt{x}+2-2\sqrt{x}-5}{\sqrt{x}-5}>=0\)
=>\(\dfrac{-\sqrt{x}-3}{\sqrt{x}-5}>=0\)
=>\(\sqrt{x}-5< 0\)
=>\(\sqrt{x}< 5\)
=>0<=x<25
Đúng 2
Bình luận (0)
Cho \(D=\left(\dfrac{x-2}{x+2}+\dfrac{1}{\sqrt{x}+2}\right).\dfrac{\sqrt{x}+1}{\sqrt{x}-1}\) với \(x>0; x\ne1\)
a) Tìm x để \(2D=2\sqrt{x}+5\)
b) Tìm x để D<1
c) Tìm x nguyên để D nguyên
cho A= \(\dfrac{2\sqrt{x}-9}{x-5\sqrt{x}+6}-\dfrac{\sqrt{x}+3}{\sqrt{x}-2}-\dfrac{2\sqrt{x}+1}{3-\sqrt{x}}\)
1, rút gọn A, tìm ĐKXĐ
2, tìm x để A< 1
3 Tìm GTNN khi B= (x-9). A
1: ĐKXĐ: \(\left\{{}\begin{matrix}x\ge0\\x\notin\left\{4;9\right\}\end{matrix}\right.\)
Ta có: \(A=\dfrac{2\sqrt{x}-9-x+9+2x-4\sqrt{x}+\sqrt{x}-2}{\left(\sqrt{x}-3\right)\left(\sqrt{x}-2\right)}\)
\(=\dfrac{\sqrt{x}+1}{\sqrt{x}-3}\)
Đúng 1
Bình luận (0)
\(1,A=\dfrac{2\sqrt{x}-9-x+9+2x-3\sqrt{x}-2}{\left(\sqrt{x}-2\right)\left(\sqrt{x}-3\right)}\\ A=\dfrac{x-\sqrt{x}-2}{\left(\sqrt{x}-2\right)\left(\sqrt{x}-3\right)}=\dfrac{\left(\sqrt{x}-2\right)\left(\sqrt{x}+1\right)}{\left(\sqrt{x}-2\right)\left(\sqrt{x}-3\right)}\\ A=\dfrac{\sqrt{x}+1}{\sqrt{x}-3}\left(x\ge0;x\ne4;x\ne9\right)\\ 2,A< 1\Leftrightarrow\dfrac{\sqrt{x}+1-\sqrt{x}+3}{\sqrt{x}-3}< 0\\ \Leftrightarrow\dfrac{4}{\sqrt{x}-3}< 0\Leftrightarrow\sqrt{x}-3< 0\Leftrightarrow0\le x< 9\)
Đúng 0
Bình luận (0)
B=\(\dfrac{\sqrt{x}-1}{2+\sqrt{x}}\)
a) Tính B khi x=\(6+2\sqrt{5}\)
b) Tìm x nguyên để B nguyên
a.
\(x=6+2\sqrt{5}=\left(\sqrt{5}+1\right)^2\) \(\Rightarrow\sqrt{x}=\sqrt{5}+1\)
\(\Rightarrow B=\dfrac{\sqrt{5}+1-1}{2+\sqrt{5}+1}=\dfrac{\sqrt{5}}{\sqrt{5}+3}=\dfrac{3\sqrt{5}-5}{4}\)
b.
\(B=\dfrac{\sqrt{x}+2-3}{\sqrt{x}+2}=1-\dfrac{3}{\sqrt{x}+2}\)
B nguyên \(\Rightarrow\dfrac{3}{\sqrt{x}+2}\in Z\Rightarrow\sqrt{x}+2=Ư\left(3\right)\)
Mà \(\sqrt{x}+2\ge2\Rightarrow\sqrt{x}+2=3\)
\(\Leftrightarrow\sqrt{x}=1\Rightarrow x=1\)
Đúng 1
Bình luận (1)
cho hai biểu thức
A=\(\dfrac{\sqrt{x}}{\sqrt{x}+5}\) và B = \(\dfrac{2\sqrt{x}}{\sqrt{x}-2}-\dfrac{\sqrt{x}-1}{\sqrt{x}+2}-\dfrac{2-5\sqrt{x}}{4-x}\) (\(x\ge0;x\ne4\))
a, tìm giá trị của A khi x = 25
b, rút gọn biểu thức B
c, tìm số tự nhiên x để \(\dfrac{B}{A}\le\dfrac{1}{3}\)
A= \(\frac{\sqrt{x}-3}{\sqrt{x}-2}-\frac{2\sqrt{x}-1}{\sqrt{x}-1}-\frac{x-6\sqrt{5}+5}{2x+7\sqrt{x}-4}\)
a) Tìm TXĐ của A
b) Rút gọn A
c) Tìm x để A >\(\frac{1}{2}\)
d) Tìm x thuộc Z để A thuộc Z
đè hinh như là 6\(\sqrt{x}\) nhi bạn
Đúng 0
Bình luận (0)
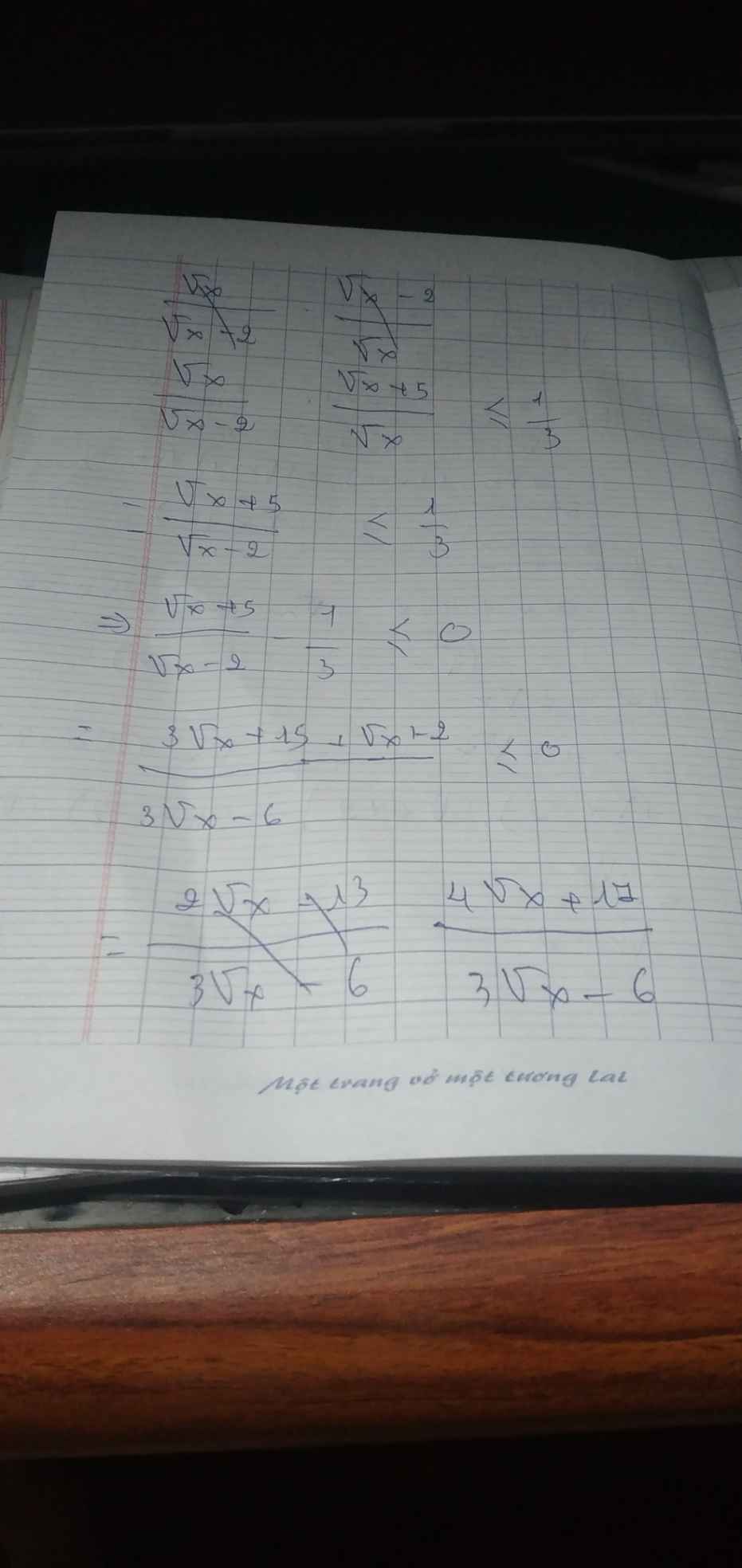
cho Bleft(dfrac{2sqrt{x}+x}{xsqrt{x}-1}-dfrac{1}{sqrt{x}-1}right)divleft(1-dfrac{sqrt{x}+2}{x+sqrt{x}+1}right)a. rút gọn Bb. tính sqrt{B} khi x5+2sqrt{3}c. tìm x để B dfrac{1}{2x^3-x-1}d. tìm giá trị của x để giá trị của B không lớn hơn giá trị biểu thức dfrac{1}{x+2}Lm nhanh giúp mk nhé mk đang cần gấp
Đọc tiếp
cho B=\(\left(\dfrac{2\sqrt{x}+x}{x\sqrt{x}-1}-\dfrac{1}{\sqrt{x}-1}\right)\div\left(1-\dfrac{\sqrt{x}+2}{x+\sqrt{x}+1}\right)\)
a. rút gọn B
b. tính \(\sqrt{B}\) khi \(x=5+2\sqrt{3}\)
c. tìm x để B= \(\dfrac{1}{2x^3-x-1}\)
d. tìm giá trị của x để giá trị của B không lớn hơn giá trị biểu thức \(\dfrac{1}{x+2}\)
Lm nhanh giúp mk nhé mk đang cần gấp
a) \(B=\left(\dfrac{2\sqrt{x}+x}{x\sqrt{x}-1}-\dfrac{1}{\sqrt{x}-1}\right):\left(1-\dfrac{\sqrt{x}+2}{x+\sqrt{x}+1}\right)\left(x\ge0,x\ne1\right)\)
\(=\left(\dfrac{2\sqrt{x}+x}{\left(\sqrt{x}-1\right)\left(x+\sqrt{x}+1\right)}-\dfrac{1}{\sqrt{x}-1}\right):\dfrac{x+\sqrt{x}+1-\sqrt{x}-2}{x+\sqrt{x}+1}\)
\(=\dfrac{2\sqrt{x}+x-x-\sqrt{x}-1}{\left(\sqrt{x}-1\right)\left(x+\sqrt{x}+1\right)}:\dfrac{x-1}{x+\sqrt{x}+1}\)
\(=\dfrac{\sqrt{x}-1}{\left(\sqrt{x}-1\right)\left(x+\sqrt{x}+1\right)}.\dfrac{x+\sqrt{x}+1}{x-1}=\dfrac{1}{x-1}\)
Đúng 1
Bình luận (0)
a) Ta có: \(B=\left(\dfrac{2\sqrt{x}+x}{x\sqrt{x}-1}-\dfrac{1}{\sqrt{x}-1}\right):\left(1-\dfrac{\sqrt{x}+2}{x+\sqrt{x}+1}\right)\)
\(=\dfrac{x+2\sqrt{x}-x-\sqrt{x}-1}{\left(\sqrt{x}-1\right)\left(x+\sqrt{x}+1\right)}:\dfrac{x+\sqrt{x}+1-\sqrt{x}-2}{\left(x+\sqrt{x}+1\right)}\)
\(=\dfrac{1}{x+\sqrt{x}+1}\cdot\dfrac{x+\sqrt{x}+1}{x-1}\)
\(=\dfrac{1}{x-1}\)
Đúng 0
Bình luận (0)
cho B= \(\frac{2\sqrt{x}-9}{x-5\sqrt{x}+6}-\frac{\sqrt{x}+3}{\sqrt{x}-2}-\frac{2\sqrt{x}+1}{3-\sqrt{x}}\)
a,xác định x để B có nghĩa
b,rút gọn B
c,tìm x để B>1
d,tìm x để B thuộc Z
\(\frac{2\sqrt{x}-9}{x-5\sqrt{x}+6}+\frac{2\sqrt{x}+1}{\sqrt{x}-3}+\frac{\sqrt{x}+3}{2-\sqrt{x}}\)
a, tìm gtri của x để bthuc M có nghĩa và rút gọn bthức M
b, tìm x thuộc Z để M=5
Để M có nghĩa thì \(\hept{\begin{cases}\sqrt{x}-3\ne0\\2-\sqrt{x}\ne0\\x\ge0\end{cases}\Leftrightarrow\hept{\begin{cases}x\ge0\\x\ne4\\x\ne9\end{cases}}}\)
ta có \(M=\frac{2\sqrt{x}-9+\left(2\sqrt{x}+1\right)\left(\sqrt{x}-2\right)-\left(\sqrt{x}+3\right)\left(\sqrt{x}-3\right)}{\left(\sqrt{x}-2\right)\left(\sqrt{x}-3\right)}\)
\(M=\frac{x-\sqrt{x}-2}{\left(\sqrt{x}-2\right)\left(\sqrt{x}-3\right)}=\frac{\sqrt{x}+1}{\sqrt{x}-3}\)
b.\(M=5=\frac{\sqrt{x}+1}{\sqrt{x}-3}\Leftrightarrow\sqrt{x}=4\Leftrightarrow x=16\)
Cho biểu thức A=\(\dfrac{\sqrt{x}+2}{\sqrt{x}-5}\) và B=\(\dfrac{3}{\sqrt{x}+5}+\dfrac{20-2\sqrt{x}}{x-25}\)
a) Tính giá trị của A khi x=9
b) Chứng minh B=\(\dfrac{1}{\sqrt{x}-5}\)
c) Tìm tất cả giá trị của x để A=B./x-4/
`A)đk:x>=0,x ne 25`
`A=9=>A=(3+2)/(3-5)=-5/2`
`B)B=(3sqrtx-15+20-2sqrtx)/(x-25)`
`=(sqrtx+5)/(x-25)`
`=1/(sqrtx-5)`
`A=B.|x-4|`
`<=>A/B=|x-4|`
`<=>\sqrtx+2=|x-4|`
`<=>\sqrtx+2=(sqrtx+2)|sqrtx-2|`
`<=>|sqrtx-2|=1`
`+)sqrtx-2=1<=>x=9(tm)`
`+)sqrtx-2=-1<=>x=1(tm)`
Vậy `S={1,9}`
Đúng 3
Bình luận (1)
a, Thay x=9 vào biểu thức A ta có
\(A=\dfrac{\sqrt{9}+2}{\sqrt{9}-5}\)
\(A=\dfrac{3+2}{3-5}=\dfrac{5}{-2}=-2,5\)
Vậy A =-2,5 khi x=9
Đúng 0
Bình luận (0)
a. A=\(\dfrac{\sqrt{x}+2}{\sqrt{x}-5}\)
=\(\dfrac{\sqrt{9}+2}{\sqrt{9}-5}=\dfrac{-5}{2}\)
Đúng 0
Bình luận (0)
Xem thêm câu trả lời