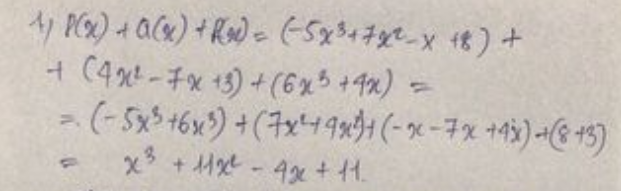bạn tham khảo nha

Đặt \(\sqrt{x^2+7x+8}=t\left(t\ge0\right)\)

ĐK: ` x \ne 2/7`

`(2x+3)((3x+8)/(2-7x)+1)=(x-5)((3x+8)/(2-7x)+1)`

`<=> ((3x+8)(2-7x)+1)(2x+3-x+5)=0`

`<=> ((3x+8)/(2-7x)+1)(x+8)=0`

 \(\Leftrightarrow\left[{}\begin{matrix}\dfrac{3x+8}{2-7x}=-1\\x+8=0\end{matrix}\right.\)

\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{5}{2}\\x=-8\end{matrix}\right.\)

Vậy `S={5/2 ; -8}`.

a) ĐKXĐ: \(x\notin\left\{-1;0\right\}\)

Ta có: \(\dfrac{x+3}{x+1}+\dfrac{x-2}{x}=2\)

\(\Leftrightarrow\dfrac{x\left(x+3\right)}{x\left(x+1\right)}+\dfrac{\left(x+1\right)\left(x-2\right)}{x\left(x+1\right)}=\dfrac{2x\left(x+1\right)}{x\left(x+1\right)}\)

Suy ra: \(x^2+3x+x^2-3x+2=2x^2+2x\)

\(\Leftrightarrow2x^2+2-2x^2-2x=0\)

\(\Leftrightarrow-2x+2=0\)

\(\Leftrightarrow-2x=-2\)

hay x=1(nhận)

Vậy: S={1}

b) ĐKXĐ: \(x\notin\left\{-7;\dfrac{3}{2}\right\}\)

Ta có: \(\dfrac{3x-2}{x+7}=\dfrac{6x+1}{2x-3}\)

\(\Leftrightarrow\left(3x-2\right)\left(2x-3\right)=\left(6x+1\right)\left(x+7\right)\)

\(\Leftrightarrow6x^2-9x-4x+6=6x^2+42x+x+7\)

\(\Leftrightarrow6x^2-13x+6-6x^2-43x-7=0\)

\(\Leftrightarrow-56x-1=0\)

\(\Leftrightarrow-56x=1\)

hay \(x=-\dfrac{1}{56}\)(nhận)

Vậy: \(S=\left\{-\dfrac{1}{56}\right\}\)

c) ĐKXĐ: \(x\ne-\dfrac{2}{3}\)

Ta có: \(\dfrac{5}{3x+2}=2x-1\)

\(\Leftrightarrow5=\left(3x+2\right)\left(2x-1\right)\)

\(\Leftrightarrow6x^2-3x+4x-2-5=0\)

\(\Leftrightarrow6x^2+x-7=0\)

\(\Leftrightarrow6x^2-6x+7x-7=0\)

\(\Leftrightarrow6x\left(x-1\right)+7\left(x-1\right)=0\)

\(\Leftrightarrow\left(x-1\right)\left(6x+7\right)=0\)

\(\Leftrightarrow\left[{}\begin{matrix}x-1=0\\6x+7=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=1\\6x=-7\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=1\left(nhận\right)\\x=-\dfrac{7}{6}\left(nhận\right)\end{matrix}\right.\)

Vậy: \(S=\left\{1;-\dfrac{7}{6}\right\}\)

d) ĐKXĐ: \(x\ne\dfrac{2}{7}\)

Ta có: \(\left(2x+3\right)\cdot\left(\dfrac{3x+8}{2-7x}+1\right)=\left(x-5\right)\left(\dfrac{3x+8}{2-7x}+1\right)\)

\(\Leftrightarrow\left(2x+3\right)\cdot\left(\dfrac{3x+8+2-7x}{2-7x}\right)-\left(x-5\right)\left(\dfrac{3x+8+2-7x}{2-7x}\right)=0\)

\(\Leftrightarrow\left(2x+3-x+5\right)\cdot\dfrac{-4x+6}{2-7x}=0\)

\(\Leftrightarrow\left(x+8\right)\cdot\left(-4x+6\right)=0\)(Vì \(2-7x\ne0\forall x\) thỏa mãn ĐKXĐ)

\(\Leftrightarrow\left[{}\begin{matrix}x+8=0\\-4x+6=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=-8\\-4x=-6\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=-8\left(nhận\right)\\x=\dfrac{3}{2}\left(nhận\right)\end{matrix}\right.\)

Vậy: \(S=\left\{-8;\dfrac{3}{2}\right\}\)

a) ĐKXĐ: \(x\ne1\)

Ta có: \(\dfrac{7x-3}{x-1}=\dfrac{2}{3}\)

\(\Leftrightarrow3\left(7x-3\right)=2\left(x-1\right)\)

\(\Leftrightarrow21x-9=2x-2\)

\(\Leftrightarrow21x-2x=-2+9\)

\(\Leftrightarrow19x=7\)

\(\Leftrightarrow x=\dfrac{7}{19}\)

Vậy: \(S=\left\{\dfrac{7}{19}\right\}\)

\(x^2-7x+\sqrt{x^2-7x+8}=12\)

\(x^2-7x-12+\sqrt{x^2-7x+8}=0\)

\(x^2-7x+8-20+\sqrt{x^2-7x+8}=0\)

Đặt : \(\sqrt{x^2-7x+8}=t\left(đk:t>0\right)\)

\(\Rightarrow x^2-7x+8=t^2\)

\(\Rightarrow\)Phương trình trở thành : \(t^2+t-20=0\)

\(\Rightarrow\orbr{\begin{cases}t=4\left(tm\right)\\t=-5\left(L\right)\end{cases}}\)

Với \(t=4\Rightarrow\sqrt{x^2-7x+8}=4\)

\(\Rightarrow x^2-7x+8=16\)

\(\Rightarrow x^2-7x+8-16=0\)

\(\Rightarrow x^2-7x-8=0\)

\(\Rightarrow\orbr{\begin{cases}x=8\\x=-1\end{cases}}\)

\(x^2-7x+\sqrt{x^2-7x+8}=12\)

\(\Leftrightarrow\sqrt{x^2-7x+8}=12-x^2+7x\)

\(\Leftrightarrow\sqrt{x^2-7x+8}-4=8-x^2+7x\)

\(\Leftrightarrow\frac{x^2-7x+8-16}{\sqrt{x^2-7x+8}+4}=-\left(x-8\right)\left(x+1\right)\)

\(\Leftrightarrow\frac{\left(x-8\right)\left(x+1\right)}{\sqrt{x^2-7x+8}+4}+\left(x-8\right)\left(x+1\right)=0\)

\(\Leftrightarrow\left(x-8\right)\left(x+1\right)\left(\frac{1}{\sqrt{x^2-7x+8}+4}+1\right)=0\)

Dễ thấy: \(\frac{1}{\sqrt{x^2-7x+8}+4}+1>0\)

\(\Rightarrow\orbr{\begin{cases}x-8=0\\x+1=0\end{cases}}\)\(\Rightarrow\orbr{\begin{cases}x=8\\x=-1\end{cases}}\)

Câu 1: 

Ta có: \(x^2-5x+4=0\)

\(\Leftrightarrow x^2-x-4x+4=0\)

\(\Leftrightarrow\left(x-1\right)\left(x-4\right)=0\)

\(\Leftrightarrow\left[{}\begin{matrix}x=1\\x=4\end{matrix}\right.\)

Vậy: S={1;4}

Câu 2: 

Ta có: \(3x^2-7x+3=0\)

\(\Delta=\left(-7\right)^2-4\cdot3\cdot3=49-36=13\)

Vì Δ>0 nên phương trình có hai nghiệm phân biệt là:

\(\left\{{}\begin{matrix}x_1=\dfrac{7-\sqrt{13}}{6}\\x_2=\dfrac{7+\sqrt{13}}{6}\end{matrix}\right.\)

Vậy: \(S=\left\{\dfrac{7-\sqrt{13}}{6};\dfrac{7+\sqrt{13}}{6}\right\}\)

Câu 3: 

Ta có: \(5x^2-x-4=0\)

\(\Leftrightarrow\left(x-1\right)\left(5x+4\right)=0\)

\(\Leftrightarrow\left[{}\begin{matrix}x=1\\x=-\dfrac{4}{5}\end{matrix}\right.\)

Vậy: \(S=\left\{1;-\dfrac{4}{5}\right\}\)

Câu 4: 

Ta có: \(7x^2+x-8=0\)

\(\Leftrightarrow\left(x-1\right)\left(7x+8\right)=0\)

\(\Leftrightarrow\left[{}\begin{matrix}x=1\\x=-\dfrac{8}{7}\end{matrix}\right.\)

Vậy: \(S=\left\{1;-\dfrac{8}{7}\right\}\)

Câu 1x^2-5x+4=0

<=>(x-1)(x-4)=0

<=>[x=1;x=4

Câu 2 3x^2-7x+3=0

x=7/6-căn bậc hai(13)/6, x=căn bậc hai(13)/6+7/6

x=7/6-căn bậc hai(13)/6, x=căn bậc hai(13)/6+7/6

Câu 3 5*x^2 -x-4 = 0

x=-4/5, x=1

Câu 4 7*x^2 +x-8 = 0

x=-8/7, x=1

bn ơi mk giải thế có chỗ nào ko hiểu bn có thể hỏi mk nhé

a) 

\(=x^2+x+6x+6\)

\(=x\left(x+1\right)+6\left(x+1\right)\)

\(=\left(x+1\right)\left(x+6\right)\)