Ta có: M =  x 2  +  y 2  – x + 6y + 10 = ( y 2  + 6y + 9) + ( x 2  – x + 1)

= y + 3 2  + ( x 2  – 2.1/2 x + 1/4) + 3/4 =  y + 3 2  + x - 1 / 2 2  + 3/4

Vì  y + 3 2  ≥ 0 và  x - 1 / 2 2  ≥ 0 nên  y + 3 2  +  x - 1 / 2 2  ≥ 0

⇒ M =  y + 3 2  +  x - 1 / 2 2  + 3/4 ≥ 3/4

⇒ M = 3/4 khi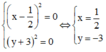 

Vậy M = 3/4 là giá trị nhỏ nhất tại y = -3 và x = 1/2

\(M=x^2+y^2-2x+6y+28=\left(x^2-2x+1\right)+\left(y^2+6y+9\right)+18=\left(x-1\right)^2+\left(y+3\right)^2+18\ge18\)

\(minM=18\Leftrightarrow\)\(\left\{{}\begin{matrix}x=1\\y=-3\end{matrix}\right.\)

\(a,P=x^2-2x+5=\left(x^2-2x+1\right)+4=\left(x-1\right)^2+4\ge4\)

Dấu \("="\Leftrightarrow x=1\)

\(b,Q=2x^2-6x=2\left(x^2-2\cdot\dfrac{3}{2}x+\dfrac{9}{4}-\dfrac{9}{4}\right)=2\left(x-\dfrac{3}{2}\right)^2-\dfrac{9}{2}\ge-\dfrac{9}{2}\)

Dấu \("="\Leftrightarrow x=\dfrac{3}{2}\)

\(c,M=\left(x^2-x+\dfrac{1}{4}\right)+\left(y^2+6y+9\right)+\dfrac{3}{4}=\left(x-\dfrac{1}{2}\right)^2+\left(y+3\right)^2+\dfrac{3}{4}\ge\dfrac{3}{4}\)

Dấu \("="\Leftrightarrow\left\{{}\begin{matrix}x=\dfrac{1}{2}\\y=-3\end{matrix}\right.\)

a: Ta có: \(P=x^2-2x+5\)

\(=x^2-2x+1+4\)

\(=\left(x-1\right)^2+4\ge4\forall x\)

Dấu '=' xảy ra khi x=1

a) \(P=x^2-2x+5\)

\(=x^2-2x+1+4\)

\(=\left(x-1\right)^2+4\ge4\)

\(MinP=4\Leftrightarrow x-1=0\Rightarrow x=1\)

b) \(Q=2x^2-6x\)

\(=2\left(x^2-3x\right)\)

\(=2\left(x^2-2.x.\frac{3}{2}+\frac{9}{4}-\frac{9}{4}\right)\)

\(=2\left(\left(x-\frac{3}{2}\right)^2-\frac{9}{4}\right)\)

\(=-\frac{9}{2}-2\left(x-\frac{3}{2}\right)^2\le\frac{-9}{2}\)

\(MinQ=\frac{-9}{2}\Leftrightarrow x-\frac{3}{2}=0\Rightarrow x=\frac{3}{2}\)

M=x^2+y^2-x+6y+10

M=(x^2-x+1/4)+(y^2+6y+9)+3/4

M=(x-1/2)^2+(y+3)^2+3/4

\(minM=\frac{3}{4}\Leftrightarrow\hept{\begin{cases}x=\frac{1}{2}\\y=-3\end{cases}}\)

\(a,-x^2+2x+5=-\left(x^2-2x-5\right)=-\left(x^2-2x+1-6\right)=-\left(x-1\right)^2+6\le6\)

dấu'=' xảy ra<=>x=1=>Max A=6

\(b,B=-x^2-y^2+4x+4y+2=-x^2+4x-4-y^2+4x-4+10\)

\(=-\left(x^2-4x+4\right)-\left(y^2-4x+4\right)+10\)

\(=-\left(x-2\right)^2-\left(y-2\right)^2+10=-\left[\left(x-2\right)^2+\left(y-2\right)^2\right]+10\le10\)

dấu"=" xảy ra<=>x=y=2=>Max B=10

\(c,C=x^2+y^2-2x+6y+12=\left(x-1\right)^2+\left(y+3\right)^2+2\ge2\)

dấu'=' xảy ra<=>x=1,y=-3=>MinC=2

a, xem lại đề 

\(b,x^2-4x+y^2-6y+1\\ =\left(x^2-4x+4\right)+\left(y^2-6y+9\right)-12\\ =\left(x-2\right)^2+\left(y-3\right)^2-12\ge-12\)

Dấu "=" xảy ra\(\Leftrightarrow\left\{{}\begin{matrix}x=2\\y=3\end{matrix}\right.\)

Vậy ...

\(c,x^2-4xy+5y^2-2y+5\\ =\left(x^2-4xy+4y^2\right)+\left(y^2-2y+1\right)+4\\ =\left(x-2y\right)^2+\left(y-1\right)^2+4\ge4\)

Dấu "=" xảy ra\(\Leftrightarrow\left\{{}\begin{matrix}x=2\\y=1\end{matrix}\right.\)

Vậy ...

a, 

Dấu "=" xảy ra⇔{x=2y=3⇔{x=2y=3

Vậy ...

Dấu "=" xảy ra⇔{x=2y=1⇔{x=2y=1

Vậy ...

cho M(x) =0

\(=>x^3-25x=0=>x\left(x^2-25\right)=0\)

\(=>\left[{}\begin{matrix}x=0\\x^2-25=0\end{matrix}\right.=>\left[{}\begin{matrix}x=0\\x^2=25=>\left[{}\begin{matrix}x=5\\x=-5\end{matrix}\right.\end{matrix}\right.\)

M(x) =0

\(=>x^5+27x^2=0=>x^2\left(x^3+27\right)=0\)

\(=>\left[{}\begin{matrix}x^2=0\\x^3=-27\end{matrix}\right.=>\left[{}\begin{matrix}x=0\\x=-3\end{matrix}\right.\)

M(x)=x³-25x=x.(x²-25)=x.(x-5)(x+5)