\(x^2-2x+8< 0\)

\(\Leftrightarrow x^2-2x+1+7< 0\)

\(\Leftrightarrow\left(x-1\right)^2+7< 0\)

PTVN.

`x^2 - 2x + 8 < 0`

`<=> (x-1)^2 + 7 < 0`

`<=> (x-1)^2 < -7`

Vì `(x-1)^2 > -7` với mọi `x`

`=>` vô nghiệm 

Vậy `x \in RR`

cái này là lớp mấy thế bạn

a) thay m = 3 ta có pt:

x² + 10x + 3 = 0 

<=> xét delta phẩy 

25 - 3 = 22 

\(\left[{}\begin{matrix}x1=-5+\sqrt{22}\\x2=-5-\sqrt{22}\end{matrix}\right.\)

vậy S={ \(-5+\sqrt{22}\);\(-5-\sqrt{22}\)}

b) xét delta phẩy 

(m+2)² - m² + 6

= 4m +10 

để phương trình có 2 nghiệm x1;x2 thì delta phẩy ≥ 0 

=> m ≥ \(\dfrac{-10}{4}\)

theo Vi-ét ta có:

\(\left\{{}\begin{matrix}x1+x2=-2m-4\\x1x2=m^2-6\end{matrix}\right.\)

theo bài ra ta có:

x1² + x2² = 16

<=> (x1+x2)² - 2x1x2 = 16

=> 4m² + 16m + 16 - 2m² + 12 = 16

<=> 2m² + 16m + 12 = 0 

<=> m² + 8m + 6 = 0 

giải ra \(\left[{}\begin{matrix}m=-4+\sqrt{10}\\m=-4-\sqrt{10}\end{matrix}\right.\)

vậy m = \(-4+\sqrt{10}\) để pt có 2 nghiệm thỏa mãn hệ thức x1² + x2² = 16

( m = -4-\(\sqrt{10}\) loại)

pt <=> (x^4+2x^3-3x^2)+(x^2+2x-3) = 0

<=> (x^2+2x-3).(x^2+1) = 0

<=> x^2+2x-3 = 0 ( vì x^2+1 > 0 )

<=> (x^2-x)+(3x-3) = 0

<=> (x-1).(x+3) = 0

<=> x-1=0 hoặc x+3=0

<=> x=1 hoặc x=-3

Vậy .........

Tk mk nha

a) 2(x + 3)(x – 4) = (2x – 1)(x + 2) – 27

⇔ 2(x2 – 4x + 3x – 12) = 2x2 + 4x – x – 2 – 27

⇔ 2x2 – 2x – 24 = 2x2 + 3x – 29

⇔ -2x – 3x = 24 – 29

⇔ - 5x = - 5 ⇔ x = -5/-5 ⇔ x = 1

Tập nghiệm của phương trình : S = {1}

b) x2 – 4 – (x + 5)(2 – x) = 0

⇔ x2 – 4 + (x + 5)(x – 2) = 0 ⇔ (x – 2)(x + 2 + x + 5) = 0

⇔ (x – 2)(2x + 7) = 0 ⇔ x – 2 = 0 hoặc 2x + 7 = 0

⇔ x = 2 hoặc x = -7/2

Tập nghiệm của phương trình: S = {2; -7/2 }

c) ĐKXĐ : x – 2 ≠ 0 và x + 2 ≠ 0 (khi đó : x2 – 4 = (x – 2)(x + 2) ≠ 0)

⇔ x ≠ 2 và x ≠ -2

Quy đồng mẫu thức hai vế :

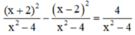
Khử mẫu, ta được : x2 + 4x + 4 – x2 + 4x – 4 = 4

⇔ 8x = 4 ⇔ x = 1/2( thỏa mãn ĐKXĐ)

Tập nghiệm của phương trình : S = {1/2}

d) ĐKXĐ : x – 1 ≠ 0 và x + 3 ≠ 0 (khi đó : x2 + 2x – 3 = (x – 1)(x + 3) ≠ 0)

⇔ x ≠ 1 và x ≠ -3

Quy đồng mẫu thức hai vế :

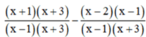
Khử mẫu, ta được : x2 + 3x + x + 3 – x2 + x – 2x + 2 + 4 = 0

⇔ 3x = -9 ⇔ x = -3 (không thỏa mãn ĐKXĐ)

Tập nghiệm của phương trình : S = ∅

\(2\left(x+3\right)\left(x-4\right)=\left(2x-1\right)\left(x+2\right)-27\)

\(< =>2\left(x^2-x-12\right)=2x^2+3x-2-27\)

\(< =>2x^2-2x-24=2x^2+3x-2-27\)

\(< =>5x=-24+29=5\)

\(< =>x=\frac{5}{5}=1\)

\(x^2-4-\left(x+5\right)\left(2-x\right)=0\)

\(< =>\left(x-2\right)\left(x+2\right)+\left(x+5\right)\left(x-2\right)=0\)

\(< =>\left(x-2\right)\left(x+2+x+5\right)=0\)

\(< =>\left(x-2\right)\left(2x+7\right)=0\)

\(< =>\orbr{\begin{cases}x-2=0\\2x+7=0\end{cases}}< =>\orbr{\begin{cases}x=2\\x=-\frac{7}{2}\end{cases}}\)

\(\Leftrightarrow\left(x^2+2x\right)^2+5\left(x^2+2x\right)+6-2=0\)

\(\Leftrightarrow\left(x^2+2x\right)^2+5\left(x^2+2x\right)+4=0\)

\(\Leftrightarrow\left(x^2+2x+1\right)\left(x^2+2x+4\right)=0\)

=>x+1=0

hay x=-1

\(\left(x^2+2x+2\right)\left(x^2+2x+3\right)-2=0\\ \Leftrightarrow\left(x^2+2x+2\right)\left[\left(x^2+2x+2\right)+1\right]-2=0\\ \Leftrightarrow\left(x^2+2x+2\right)^2+\left(x^2+2x+2\right)-2=0\\ \Leftrightarrow\left[\left(x^2+2x+2\right)^2+2\left(x^2+2x+2\right)\right]-\left[\left(x^2+2x+2\right)+2\right]=0\\ \Leftrightarrow\left(x^2+2x+2\right)\left(x^2+2x+2+2\right)-\left(x^2+2x+2+2\right)=0\\ \Leftrightarrow\left(x^2+2x+2-1\right)\left(x^2+2x+4\right)=0\\ \Leftrightarrow\left(x^2+2x+1\right)\left(x^2+2x+4\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x^2+2x+1=0\\x^2+2x+4=0\end{matrix}\right.\)

\(\Leftrightarrow\left[{}\begin{matrix}\left(x+1\right)^2=0\\\left(x+1\right)^2+3=0\left(vô.lí\right)\end{matrix}\right.\\ \Leftrightarrow x+1=0\\ \Leftrightarrow x=-1\)

Đặt \(x^2+2x+2=t\)đk t > 0 

\(t\left(t+1\right)-2=0\Leftrightarrow t^2+t-2=0\Leftrightarrow t=1;t=2\left(ktm\right)\)

Với t = 1 \(x^2+2x+1=0\Leftrightarrow\left(x+1\right)^2=0\Leftrightarrow x=-1\)

`a,(2x-5)(12+5x)=0`

\(\Leftrightarrow\left[{}\begin{matrix}2x-5=0\\12+5x=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}2x=5\\5x=-12\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{5}{2}\\x=-\dfrac{12}{5}\end{matrix}\right.\)

`b, (x-3)(x-4)-2(x-3)=0`

`<=>(x-3)(x-4-2)=0`

`<=>(x-3)(x-6)=0`

\(\Leftrightarrow\left[{}\begin{matrix}x-3=0\\x-6=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=3\\x=6\end{matrix}\right.\)

`c, x(x-1)(x+1)=0`

\(\Leftrightarrow\left[{}\begin{matrix}x=0\\x-1=0\\x+1=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=0\\x=1\\x=-1\end{matrix}\right.\)

`d, (2x)/3 +(2x-1)/6=0`

`<=> (4x)/6 +(2x-1)/6=0`

`<=> (4x+2x-1)/6=0`

`<=> (6x-1)/6=0`

`<=> 6x-1=0`

`<=> 6x=1`

`<=>x=1/6` ( đề là vậy à bạn )

 a) \(\left(2x-5\right)\left(12+5x\right)=0\)

\(\Leftrightarrow\left[{}\begin{matrix}2x-5=0\\12+5x=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}2x=5\\5x=-12\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=2,5\\x=-2,4\end{matrix}\right.\)

b) \(\left(x-3\right)\left(x-4\right)-2\left(x-3\right)=0\)

\(\Leftrightarrow\left(x-3\right)\left[\left(x-4\right)-2\right]=0\)

\(\Leftrightarrow\left(x-3\right)\left(x-6\right)\)

\(\Leftrightarrow\left[{}\begin{matrix}x-3=0\\x-6=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=3\\x=6\end{matrix}\right.\)

c) \(x\left(x-1\right)\left(x+1\right)=0\)

\(\Leftrightarrow\left[{}\begin{matrix}x+1=0\\x-1=0\\x=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=-1\\x=1\\x=0\end{matrix}\right.\)

d) \(\dfrac{2x}{3}+\dfrac{2x-1}{6}=0\)

\(\Leftrightarrow\dfrac{4x+2x-1}{6}=0\)

\(\Leftrightarrow6x-1=0\)

\(\Leftrightarrow6x=1\Leftrightarrow x=\dfrac{1}{6}\)

\(a,\dfrac{x-3}{x}=\dfrac{x-3}{x+3}\)\(\left(đk:x\ne0,-3\right)\)

\(\Leftrightarrow\dfrac{x-3}{x}-\dfrac{x-3}{x+3}=0\)

\(\Leftrightarrow\dfrac{\left(x-3\right)\left(x+3\right)-x\left(x-3\right)}{x\left(x+3\right)}=0\)

\(\Leftrightarrow x^2-9-x^2+3x=0\)

\(\Leftrightarrow3x-9=0\)

\(\Leftrightarrow3x=9\)

\(\Leftrightarrow x=3\left(n\right)\)

Vậy \(S=\left\{3\right\}\)

\(b,\dfrac{4x-3}{4}>\dfrac{3x-5}{3}-\dfrac{2x-7}{12}\)

\(\Leftrightarrow\dfrac{4x-3}{4}-\dfrac{3x-5}{3}+\dfrac{2x-7}{12}>0\)

\(\Leftrightarrow\dfrac{3\left(4x-3\right)-4\left(3x-5\right)+2x-7}{12}>0\)

\(\Leftrightarrow12x-9-12x+20+2x-7>0\)

\(\Leftrightarrow2x+4>0\)

\(\Leftrightarrow2x>-4\)

\(\Leftrightarrow x>-2\)