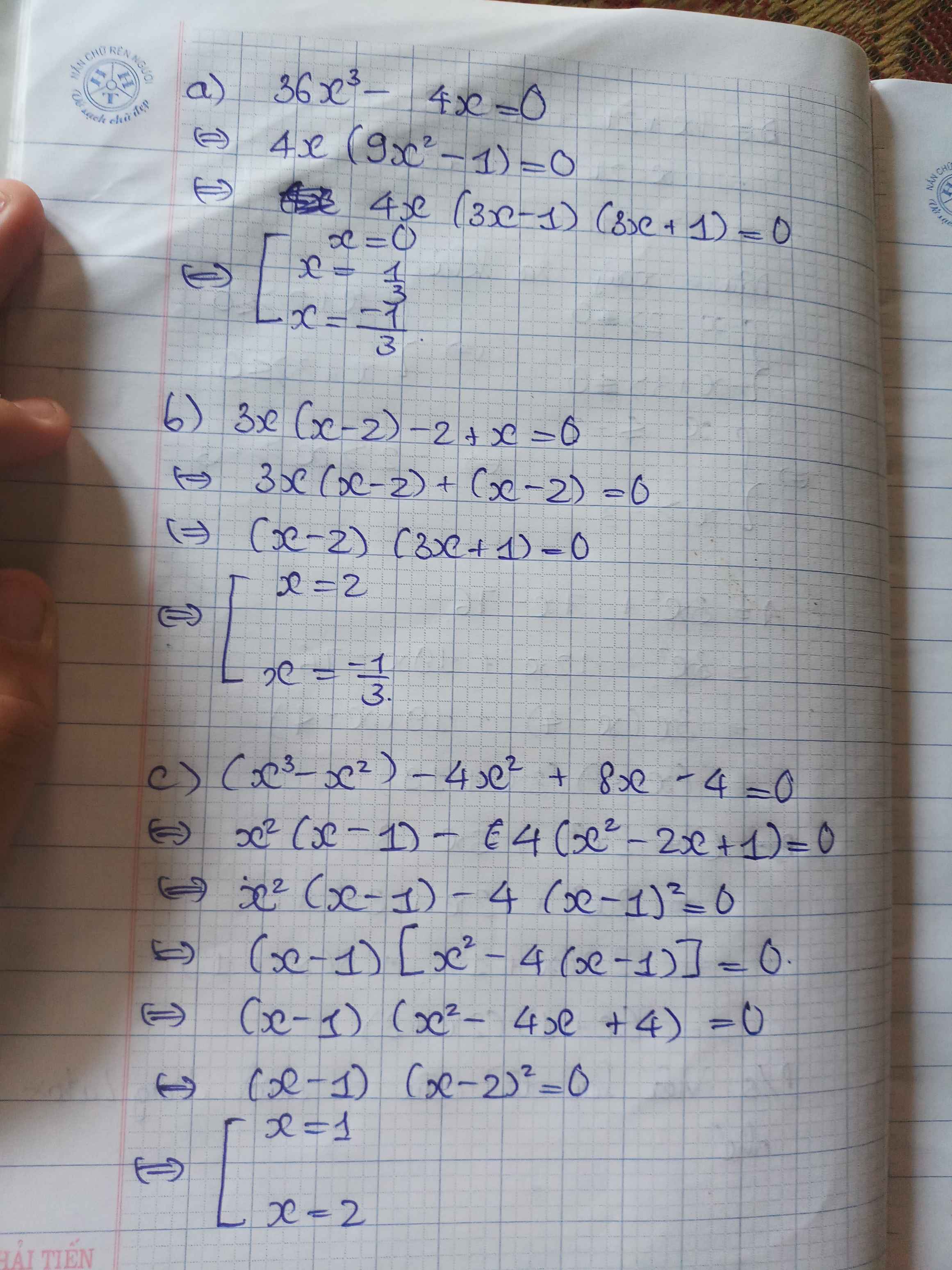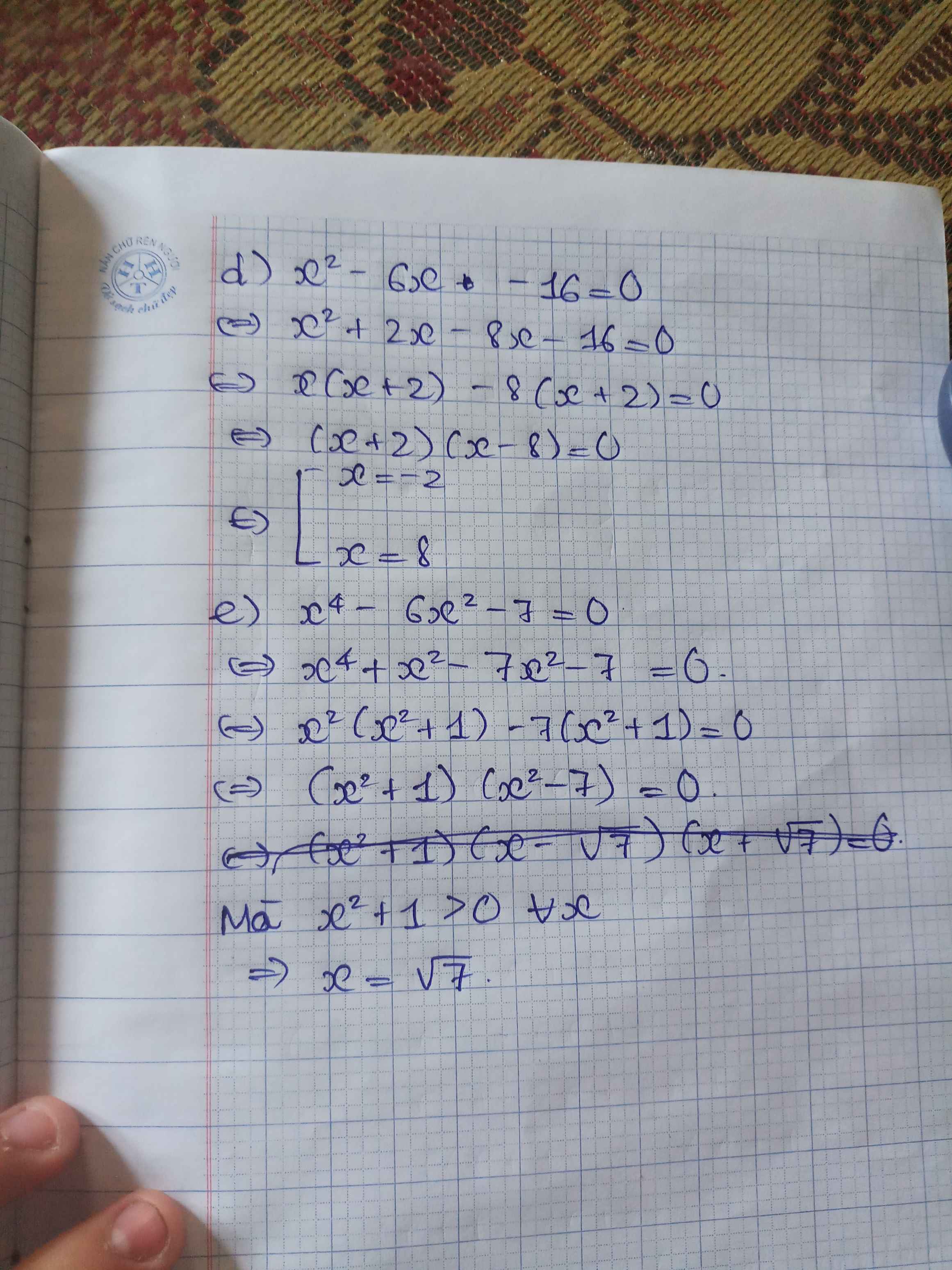tinh
a)(3-x).(x+4)=0b)4x-x2>hoăc=05x211010x10x< hoặc=0
Những câu hỏi liên quan
Giải phương trình:
a) x4 - 2x3 + x2 - 4x +4 = 0
b) x4 + 2x3 - 3 = 0
c) 2x4 - 100x + 98 = 0
d) (x + 1)(x + 2)(x + 3)(x + 4) = 24
d: Ta có: \(\left(x+1\right)\left(x+2\right)\left(x+3\right)\left(x+4\right)=24\)
\(\Leftrightarrow\left(x^2+5x+4\right)\left(x^2+5x+6\right)-24=0\)
\(\Leftrightarrow x\left(x+5\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=0\\x=-5\end{matrix}\right.\)
Đúng 0
Bình luận (0)
tìm x, biết:
a) 9x2+36=0
b) 3(x+4)-x2-4x=0
c) x(2x-1)-(x-2)(2x+1)=0
d) (2x-3)2-4x2=00
e)1 phần 3.x2-3x=0
f) x3-x2-x+1=0
ráng giúp mình nha
\(a,\Leftrightarrow9x^2=-36\Leftrightarrow x\in\varnothing\\ b,\Leftrightarrow3\left(x+4\right)-x\left(x+4\right)=0\\ \Leftrightarrow\left(3-x\right)\left(x+4\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x=3\\x=-4\end{matrix}\right.\\ c,\Leftrightarrow2x^2-x-2x^2+3x+2=0\\ \Leftrightarrow2x=-2\Leftrightarrow x=-1\\ d,\Leftrightarrow\left(2x-3-2x\right)\left(2x-3+2x\right)=0\\ \Leftrightarrow-3\left(4x-3\right)=0\\ \Leftrightarrow x=\dfrac{3}{4}\\ e,\Leftrightarrow\dfrac{1}{3}x\left(x-9\right)=0\Leftrightarrow\left[{}\begin{matrix}x=0\\x=9\end{matrix}\right.\\ f,\Leftrightarrow x^2\left(x-1\right)-\left(x-1\right)=0\\ \Leftrightarrow\left(x^2-1\right)\left(x-1\right)=0\\ \Leftrightarrow\left(x-1\right)^2\left(x+1\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x=1\\x=-1\end{matrix}\right.\)
Đúng 1
Bình luận (0)
Tìm x biết:
a, 16x² – 9(x + 1)²= 0
b, x2 (x – 1) – 4x2 + 8x – 4 = 0
c, x(2x – 3) – 2(3 – 2x) = 0
d, (x – 3)(x² + 3x + 9) – x(x + 2)(x – 2) = 1
e, 4x² + 4x – 6 = 2
f, 2x² + 7x + 3 = 0
e: ta có: \(4x^2+4x-6=2\)
\(\Leftrightarrow4x^2+4x-8=0\)
\(\Leftrightarrow\left(x+2\right)\left(x-1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=-2\\x=1\end{matrix}\right.\)
f: Ta có: \(2x^2+7x+3=0\)
\(\Leftrightarrow\left(x+3\right)\left(2x+1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=-3\\x=-\dfrac{1}{2}\end{matrix}\right.\)
Đúng 2
Bình luận (0)
Giải phương trình:
a)x2-4x+4=0
b)2x2-x=0
c)x2-5x+6=0
d)x2+y2=0
e)x2+6x+10=0
\(a.x^2-4x+4=0\)
\(\left(x-2\right)^2=0\)
=>x=2
b) \(2x^2-x=0\)
\(x\left(2x-1\right)=0\)
=> \(\left[{}\begin{matrix}x=0\\x=\dfrac{1}{2}\end{matrix}\right.\)
c) \(x^2-5x+6=0\)
\(x^2-2x-3x+6=0\)
\(\left(x-2\right)\left(x-3\right)=0\)
=> \(\left[{}\begin{matrix}x=2\\x=3\end{matrix}\right.\)
d) \(x^2+y^2=0\)
Vì \(x^2,y^2\ge0\forall x,y\)
=>x=y=0
e) \(x^2+6x+10=0\)
\(\left(x+3\right)^2+1=0\)
Vì \(\left(x+3\right)^2\ge0\forall x\)
=> VT>0 \(\forall x\)
=> phương trình vô nghiệm
Đúng 3
Bình luận (0)
a) \(x^2-4x+4=0\)
\(\Leftrightarrow\left(x-2\right)^2=0\)
\(\Leftrightarrow x-2=0\)
\(\Leftrightarrow x=2\)
b) \(2x^2-x=0\)
\(\Leftrightarrow x\left(2x-1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=0\\2x-1=0\end{matrix}\right.\) \(\Leftrightarrow\left[{}\begin{matrix}x=0\\x=\dfrac{1}{2}\end{matrix}\right.\)
c) \(x^2-5x+6=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=2\\x=3\end{matrix}\right.\) \(\left(a+b+c=0\right)\)
d) \(x^2+y^2=0\)
\(\Leftrightarrow\left\{{}\begin{matrix}x^2=0\\y^2=0\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}x=0\\y=0\end{matrix}\right.\)
e) \(x^2+6x+10=0\)
\(\Leftrightarrow x^2+6x+9+1=0\)
\(\Leftrightarrow\left(x+3\right)^2+1=0\left(1\right)\)
mà \(\left(x+3\right)^2+1\ge1>0,\forall x\in R\)
Nên phương trình (1) vô nghiệm
Đúng 0
Bình luận (0)
giải các Phương trình sau
a) (5x+3)(x2+1)(x-1)=0
b) (4x-1)(x-3)-(x-3)(5x+2)=0
c) (x+6)(3x-1)+x2-36 =0
a: =>(5x+3)(x-1)=0
=>x=1 hoặc x=-3/5
b: =>(x-3)(4x-1-5x-2)=0
=>(x-3)(-x-3)=0
=>x=-3 hoặc x=3
c: =>(x+6)(3x-1+x-6)=0
=>(x+6)(4x-7)=0
=>x=7/4 hoặc x=-6
Đúng 0
Bình luận (0)
a. (2x - 5)2 + (4x - 10)(2 + x) + x2 + 4x + 4 = 0
b. ( 3 – x2 + 5x )( x2 – 5x + 3) = 9
\(a,\Rightarrow\left(2x-5\right)^2+2\left(2x-5\right)\left(x+2\right)+\left(x+2\right)^2=0\\ \Rightarrow\left(2x-5+x+2\right)^2=0\\ \Rightarrow3x-3=0\\ \Rightarrow x=1\\ b,\Rightarrow9-\left(x^2-5x\right)^2=9\\ \Rightarrow x^2-5x=0\\ \Rightarrow x\left(x-5\right)=0\Rightarrow\left[{}\begin{matrix}x=0\\x=5\end{matrix}\right.\)
Đúng 0
Bình luận (0)
giải các phương trình sau
a) x2+4x-5=0
b) x2-x-12=0
c) (2x-7)2-6(2x-7)(x-3)=0
`a,x^2 +4x-5=0`
`<=> x^2-x+5x-5=0`
`<=> x(x-1)+5(x-1)=0`
`<=>(x-1)(x+5)=0`
\(\Leftrightarrow\left[{}\begin{matrix}x-1=0\\x+5=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=1\\x=-5\end{matrix}\right.\)
`b, x^2 -x-12=0`
`<=> x^2 +3x-4x-12=0`
`<=>(x^2+3x)-(4x+12)=0`
`<=>x(x+3)-4(x+3)=0`
`<=>(x+3)(x-4)=0`
\(\Leftrightarrow\left[{}\begin{matrix}x+3=0\\x-4=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=-3\\x=4\end{matrix}\right.\)
`c, (2x-7)^2 - 6(2x-7)(x-3)=0`
`<=>(2x-7)(2x-7 -6x+18)=0`
`<=>(2x-7) ( -4x+11)=0`
\(\Leftrightarrow\left[{}\begin{matrix}2x-7=0\\-4x+11=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}2x=7\\-4x=-11\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{7}{2}\\x=\dfrac{11}{4}\end{matrix}\right.\)
Đúng 2
Bình luận (0)
a: =>(x+5)(x-1)=0
=>x=1 hoặc x=-5
b: =>(x-4)(x+3)=0
=>x=4 hoặc x=-3
c: =>(2x-7)(2x-7-6x+18)=0
=>(2x-7)(-4x+11)=0
=>x=11/4 hoặc x=7/2
Đúng 0
Bình luận (0)
Giải phương trình bằng cách đặt ẩn phụ:
a
)
3.
x
2
+
x
2
−
2
x
2
+
x...
Đọc tiếp
Giải phương trình bằng cách đặt ẩn phụ:
a ) 3. x 2 + x 2 − 2 x 2 + x − 1 = 0 b ) x 2 − 4 x + 2 2 + x 2 − 4 x − 4 = 0 c ) x − x = 5 x + 7 d ) x x + 1 − 10 ⋅ x + 1 x = 3
a)
3 · x 2 + x 2 - 2 x 2 + x - 1 = 0 ( 1 )
Đặt t = x 2 + x ,
Khi đó (1) trở thành : 3 t 2 – 2 t – 1 = 0 ( 2 )
Giải (2) : Có a = 3 ; b = -2 ; c = -1
⇒ a + b + c = 0
⇒ (2) có hai nghiệm t 1 = 1 ; t 2 = c / a = - 1 / 3 .
+ Với t = 1 ⇒ x 2 + x = 1 ⇔ x 2 + x – 1 = 0 ( * )
Có a = 1; b = 1; c = -1 ⇒ Δ = 1 2 – 4 . 1 . ( - 1 ) = 5 > 0
(*) có hai nghiệm
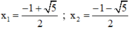
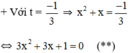
Có a = 3; b = 3; c = 1 ⇒ Δ = 3 2 – 4 . 3 . 1 = - 3 < 0
⇒ (**) vô nghiệm.
Vậy phương trình (1) có tập nghiệm 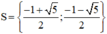
b)
x 2 − 4 x + 2 2 + x 2 − 4 x − 4 = 0 ⇔ x 2 − 4 x + 2 2 + x 2 − 4 x + 2 − 6 = 0 ( 1 )
Đặt x 2 – 4 x + 2 = t ,
Khi đó (1) trở thành: t 2 + t – 6 = 0 ( 2 )
Giải (2): Có a = 1; b = 1; c = -6
⇒ Δ = 1 2 – 4 . 1 . ( - 6 ) = 25 > 0
⇒ (2) có hai nghiệm
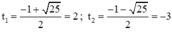
+ Với t = 2 ⇒ x 2 – 4 x + 2 = 2
⇔ x 2 – 4 x = 0
⇔ x(x – 4) = 0
⇔ x = 0 hoặc x = 4.
+ Với t = -3 ⇒ x 2 – 4 x + 2 = - 3
⇔ x2 – 4x + 5 = 0 (*)
Có a = 1; b = -4; c = 5 ⇒ Δ ’ = ( - 2 ) 2 – 1 . 5 = - 1 < 0
⇒ (*) vô nghiệm.
Vậy phương trình ban đầu có tập nghiệm S = {0; 4}.
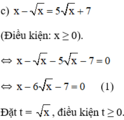
Khi đó (1) trở thành: t 2 – 6 t – 7 = 0 ( 2 )
Giải (2): Có a = 1; b = -6; c = -7
⇒ a – b + c = 0
⇒ (2) có nghiệm t 1 = - 1 ; t 2 = - c / a = 7 .
Đối chiếu điều kiện chỉ có nghiệm t = 7 thỏa mãn.
+ Với t = 7 ⇒ √x = 7 ⇔ x = 49 (thỏa mãn).
Vậy phương trình đã cho có nghiệm x = 49.
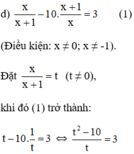
⇔ t 2 – 10 = 3 t ⇔ t 2 – 3 t – 10 = 0 ( 2 )
Giải (2): Có a = 1; b = -3; c = -10
⇒ Δ = ( - 3 ) 2 - 4 . 1 . ( - 10 ) = 49 > 0
⇒ (2) có hai nghiệm:
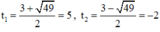
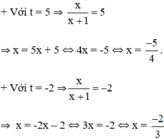
Cả hai nghiệm đều thỏa mãn điều kiện xác định.
Vậy phương trình đã cho có tập nghiệm 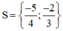
Đúng 0
Bình luận (0)
Tìm x:
a) 36x3-4x=0
b) 3x(x-2)-2+x=0
c) (x3-x2)-4x2+8x-4=0
d) x2-6x-16=0
e) x4-6x2-7=0