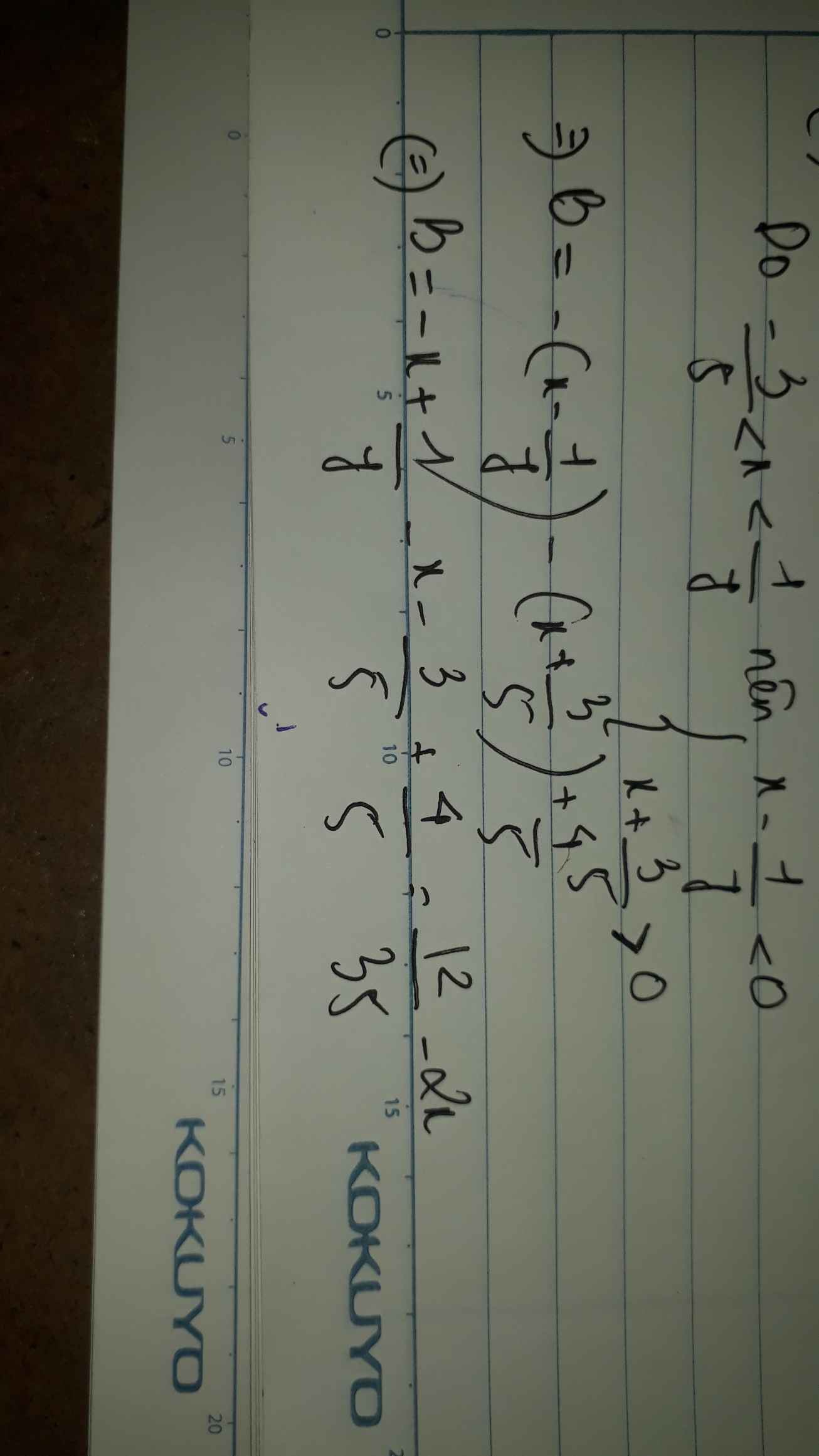Rút gọn biểu thức: x 4 + 15 x + 7 2 x 3 + 2 . x 14 x 2 + 1 . 4 x 3 + 4 x 4 + 15 x + 7

Những câu hỏi liên quan
BÀI 6
\(A=\dfrac{x+15}{x^2-9}-\dfrac{2}{x+3}\)
a) viết điều kiện xác định của biểu thức A
b)rút gọn phân thức
c)tìm giá trị của Akhi x=-1
BÀI 7
\(A=\dfrac{x+2}{x-2}+\dfrac{x-1}{x+2}\dfrac{x^2-4x}{4-x^2}\)với x2-4≠0
a)rút gọn biểu thức A
b)tính giá trị cua A khi x=4
a) ĐKXĐ:
\(\left\{{}\begin{matrix}x^2-9\ne0\\x+3\ne0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x\ne\pm3\\x\ne-3\end{matrix}\right.\Leftrightarrow x\ne\pm3\)
b) \(A=\dfrac{x+15}{x^2-9}-\dfrac{2}{x+3}\)
\(A=\dfrac{x+15}{\left(x+3\right)\left(x-3\right)}-\dfrac{2\left(x-3\right)}{\left(x+3\right)\left(x-3\right)}\)
\(A=\dfrac{x+15-2x+6}{\left(x+3\right)\left(x-3\right)}\)
\(A=\dfrac{21-x}{\left(x+3\right)\left(x-3\right)}\)
c) Thay x = - 1 vào A ta có:
\(A=\dfrac{21-\left(-1\right)}{\left(-1+3\right)\left(-1-3\right)}=\dfrac{21+1}{2\cdot-4}=\dfrac{22}{-8}=-\dfrac{11}{4}\)
Đúng 4
Bình luận (0)
Rút gọn biểu thức sau:
C= |15-x| + 2|9-x| - 5| x-9| -7
Câu1: Rút gọn biểu thức
a) 3x(x-3)-5x(x+7)
b) 1/5x(10x -15)-2x(x-5)-12
a) \(3x\left(x-3\right)-5x\left(x+7\right)\)
\(=3x^2-9x-5x^2-35x\)
\(=-2x^2-44x\)
b) \(\dfrac{1}{5}x\left(10x-15\right)-2x\left(x-5\right)-12\)
\(=2x^2-3x-2x^2+10x-12\)
\(=7x-12\)
Đúng 2
Bình luận (0)
b=|x-1/7|-|x+3/5|+4/5 voi -3/5<x<1/7 rút gọn biểu thức
Ta có: \(B=\left|x-\dfrac{1}{7}\right|-\left|x+\dfrac{3}{5}\right|+\dfrac{4}{5}\)
\(=-x+\dfrac{1}{7}-x-\dfrac{3}{5}+\dfrac{4}{5}\)
\(=-2x+\dfrac{12}{35}\)
Đúng 0
Bình luận (0)
rút gọn biểu thức :
[-15 + (-x )] +[ 25 - (-x)]
Rút gọn biểu thức
-3.(x - 4) + 2.(-y).(4 - x) + 7.(x - 4) - 5.(-y).(4 - x)
Cho A=|x-4|+|x+15|.rút gọn biểu thức trên
Rút gọn biểu thức A= \(\dfrac{-7\sqrt{x}+6}{x-4}+\dfrac{\sqrt{x}}{\sqrt{x}-2}\)
`A=(-7sqrtx+6)/(x-4)+sqrtx/(sqrtx-2)`
`=(-7sqrtx+6)/(x-4)+(x+2sqrtx)/(x-4)`
`=(x+2sqrtx-7sqrtx+6)/(x-4)`
`=(x-5sqrtx+6)/(x-4)`
`=((sqrtx-2)(sqrtx-3))/((sqrtx-2)(sqrtx+2))`
`=(sqrtx-3)/(sqrtx+2)`
Đúng 1
Bình luận (0)
Câu 1: Pttnta) 25x^2(x-3y)-15(3y-x) b) x^4-5x^2+4Bài2 cho biểu thức a) Tìm điều kiện xác định của biểu thức Ab) Rút gọn biểu thức Ac) Tính giá trị của biểu thức A khi x2024
Đọc tiếp
Câu 1: Pttnt
a) 25x^2(x-3y)-15(3y-x) b) x^4-5x^2+4
Bài2 cho biểu thức 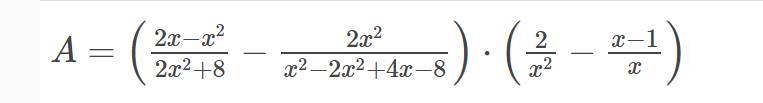
a) Tìm điều kiện xác định của biểu thức A
b) Rút gọn biểu thức A
c) Tính giá trị của biểu thức A khi x=2024
Câu 2:
a: ĐKXĐ: \(x\notin\left\{0;2\right\}\)
b: Sửa đề: \(A=\left(\dfrac{2x-x^2}{2x^2+8}-\dfrac{2x^2}{x^3-2x^2+4x-8}\right)\cdot\left(\dfrac{2}{x^2}-\dfrac{x-1}{x}\right)\)
\(=\left(\dfrac{2x-x^2}{2\left(x^2+4\right)}-\dfrac{2x^2}{\left(x-2\right)\left(x^2+4\right)}\right)\cdot\dfrac{2-x\left(x-1\right)}{x^2}\)
\(=\left(\dfrac{\left(2x-x^2\right)\left(x-2\right)-4x^2}{2\left(x^2+4\right)\left(x-2\right)}\right)\cdot\dfrac{2-x^2+x}{x^2}\)
\(=\dfrac{\left(x^2-2x\right)\left(x-2\right)+4x^2}{2\left(x^2+4\right)\left(x-2\right)}\cdot\dfrac{x^2-x-2}{x^2}\)
\(=\dfrac{x^3-2x^2-2x^2+4x+4x^2}{2\left(x^2+4\right)\left(x-2\right)}\cdot\dfrac{\left(x-2\right)\left(x+1\right)}{x^2}\)
\(=\dfrac{x^3+4x}{2\left(x^2+4\right)}\cdot\dfrac{x+1}{x^2}\)
\(=\dfrac{x\left(x^2+4\right)\left(x+1\right)}{2\left(x^2+4\right)\cdot x^2}=\dfrac{x+1}{2x}\)
c: Khi x=2024 thì \(A=\dfrac{2024+1}{2\cdot2024}=\dfrac{2025}{4048}\)
Câu 1:
a: \(25x^2\left(x-3y\right)-15\left(3y-x\right)\)
\(=25x^2\left(x-3y\right)+15\left(x-3y\right)\)
\(=\left(x-3y\right)\left(25x^2+15\right)\)
\(=\left(x-3y\right)\cdot5\cdot\left(5x^2+3\right)\)
b: \(x^4-5x^2+4\)
\(=x^4-x^2-4x^2+4\)
\(=\left(x^4-x^2\right)-\left(4x^2-4\right)\)
\(=x^2\left(x^2-1\right)-4\left(x^2-1\right)\)
\(=\left(x^2-1\right)\left(x^2-4\right)=\left(x-1\right)\left(x+1\right)\left(x-2\right)\left(x+2\right)\)
Đúng 1
Bình luận (0)
RÚT GỌN BIỂU THỨC:
15) \(A= \left(1 - \dfrac{4}{\sqrt{x} + 1} + \dfrac{1}{x - 1} \right) : \dfrac{x - 2\sqrt{x}}{x - 1} \)
\(A=\left(1-\dfrac{4}{\sqrt{x}+1}+\dfrac{1}{\left(\sqrt{x}-1\right)\cdot\left(\sqrt{x}+1\right)}\right)\cdot\dfrac{x-1}{x-2\sqrt{x}}\)
\(=\dfrac{x-1-4\left(\sqrt{x}-1\right)+1}{x-1}\cdot\dfrac{x-1}{x-2\sqrt{x}}\)
\(=\dfrac{x-4\sqrt{x}+4}{x-2\sqrt{x}}=\dfrac{\left(\sqrt{x}-2\right)^2}{\sqrt{x}\left(\sqrt{x}-2\right)}=\dfrac{\sqrt{x}-2}{\sqrt{x}}\)
Đúng 1
Bình luận (0)
\(A=\left(1-\dfrac{4}{\sqrt{x}+1}+\dfrac{1}{x-1}\right):\dfrac{x-2\sqrt{x}}{x-1}\) (ĐK: \(x>0;x\ne1;x\ne4\))
\(A=\left[1-\dfrac{4}{\sqrt{x}+1}+\dfrac{1}{\left(\sqrt{x}\right)^2-1^2}\right]:\dfrac{\sqrt{x}\left(\sqrt{x}-2\right)}{\left(\sqrt{x}\right)^2-1^2}\)
\(A=\left[\dfrac{\left(\sqrt{x}+1\right)\left(\sqrt{x}-1\right)}{\left(\sqrt{x}+1\right)\left(\sqrt{x}-1\right)}-\dfrac{4\left(\sqrt{x}-1\right)}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}+\dfrac{1}{\left(\sqrt{x}+1\right)\left(\sqrt{x}-1\right)}\right]:\dfrac{\sqrt{x}\left(\sqrt{x}-2\right)}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}\)
\(A=\dfrac{x-1-4\sqrt{x}+4+1}{\left(\sqrt{x}+1\right)\left(\sqrt{x}-1\right)}\cdot\dfrac{\left(\sqrt{x}+1\right)\left(\sqrt{x}-1\right)}{\sqrt{x}\left(\sqrt{x}-2\right)}\)
\(A=\dfrac{x-4\sqrt{x}+4}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}\cdot\dfrac{\left(\sqrt{x}+1\right)\left(\sqrt{x}-1\right)}{\sqrt{x}\left(\sqrt{x}-2\right)}\)
\(A=\dfrac{\left(\sqrt{x}-2\right)^2}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}\cdot\dfrac{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}{\sqrt{x}\left(\sqrt{x}-2\right)}\)
\(A=\dfrac{\sqrt{x}-2}{\sqrt{x}}\)
Đúng 2
Bình luận (0)
Bước 1: Đặt y = √x Khi đó, biểu thức A sẽ trở thành: A = (1 - 4y + 1/y) / (y^2 - 2y + 1)
Bước 2: Nhân mẫu và tử số với y^2 để loại bỏ phân số: A = (y^2 - 4y^3 + y^2) / (y^4 - 2y^3 + y^2)
Bước 3: Kết hợp các thành phần tương đồng: A = (2y^2 - 4y^3) / (y^4 - 2y^3 + y^2)
Bước 4: Rút gọn tử số và mẫu: A = 2y^2(1 - 2y) / y^2(y^2 - 2y + 1)
Bước 5: Đặt z = y^2 - 2y + 1 Khi đó, biểu thức A sẽ trở thành: A = 2(1 - 2y) / z
Vậy, biểu thức rút gọn của A là: A = 2(1 - 2y) / (y^2 - 2y + 1)
Đúng 0
Bình luận (0)
Xem thêm câu trả lời