a: (x+2)(x-3)>0

nên x+2;x-3 cùng dấu

=>x>3 hoặc x<-2

b: (x-1)(x+4)<=0

nên x-1 và x+4 khác dấu

=>-4<=x<=1

a) Ta có: \(x^2-x-12=0\)

\(\Leftrightarrow\left(x-4\right)\left(x+3\right)=0\)

\(\Leftrightarrow\left[{}\begin{matrix}x=4\\x=-3\end{matrix}\right.\)

b) Ta có: \(x^2+3x-18=0\)

\(\Leftrightarrow\left(x+6\right)\left(x-3\right)=0\)

\(\Leftrightarrow\left[{}\begin{matrix}x=-6\\x=3\end{matrix}\right.\)

\(a,\Leftrightarrow x\left(x+9\right)=0\Leftrightarrow\left[{}\begin{matrix}x=0\\x=-9\end{matrix}\right.\\ b,\Leftrightarrow\left(x+4-4\right)\left(x+4+4\right)=0\\ \Leftrightarrow x\left(x+8\right)=0\Leftrightarrow\left[{}\begin{matrix}x=0\\x=-8\end{matrix}\right.\\ c,\Leftrightarrow x\left(x-4\right)\left(x+4\right)=0\Leftrightarrow\left[{}\begin{matrix}x=0\\x=4\\x=-4\end{matrix}\right.\\ d,\Leftrightarrow\left(x-5\right)^2=0\Leftrightarrow x=5\)

a) \(\Leftrightarrow x\left(x+9\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x=0\\x=-9\end{matrix}\right.\)

b) \(\Leftrightarrow x\left(x+8\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x=0\\x=-8\end{matrix}\right.\)

c) \(\Leftrightarrow x\left(x-4\right)\left(x+4\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x=0\\x=4\\x=-4\end{matrix}\right.\)

d) \(\Leftrightarrow\left(x-5\right)^2=0\\ \Leftrightarrow x=5\)

\(a,\Leftrightarrow\left(9x^2-18x+9\right)+\left(y^2-6y+9\right)+\left(2z^2+4z+2\right)=0\\ \Leftrightarrow9\left(x-1\right)^2+\left(y-3\right)^2+2\left(z+1\right)^2=0\\ \Leftrightarrow\left\{{}\begin{matrix}x=1\\y=3\\z=-1\end{matrix}\right.\)

\(b,\Leftrightarrow\left(4x^2+8xy+4y^2\right)+\left(x^2-2x+1\right)+\left(y^2+2y+1\right)=0\\ \Leftrightarrow4\left(x+y\right)^2+\left(x-1\right)^2+\left(y+1\right)^2=0\\ \Leftrightarrow\left\{{}\begin{matrix}x=-y\\x=1\\y=-1\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=1\\y=-1\end{matrix}\right.\)

\(c,\Leftrightarrow\left(4x^2+4xy+y^2\right)+\left(x^2-2x+1\right)+\left(y^2+4y+4\right)=0\\ \Leftrightarrow\left(2x+y\right)^2+\left(x-1\right)^2+\left(y+2\right)^2=0\\ \Leftrightarrow\left\{{}\begin{matrix}2x=-y\\x=1\\y=-2\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=1\\y=-2\end{matrix}\right.\)

a,9x^2+y^2+2z^2−18x+4z−6y+20=0

⇔9(x−1)^2+(y−3)^2+2(z+1)^2=0

⇔x=1;y=3;z=−1

b,5x^2+5y^2+8xy+2y−2x+2=0

⇔4(x+y)2+(x−1)2+(y+1)2=0

⇔x=−y;x=1y=−1⇔x=1y=−1

c,5x^2+2y^2+4xy−2x+4y+5=0

⇔(2x+y)^2+(x−1)^2+(y+2)^2=0

⇔2x=−y;x=1;y=−2

⇔x=1;y=−2

⇔(x−1)^2+(2y−3)^2+(z+2)^2=0

\(d,\Leftrightarrow\left(x^2-2x+1\right)+\left(4y^2-12y+9\right)+\left(z^2+4z+4\right)=0\\ \Leftrightarrow\left(x-1\right)^2+\left(2y-3\right)^2+\left(z+2\right)^2=0\\ \Leftrightarrow\left\{{}\begin{matrix}x=1\\y=\dfrac{3}{2}\\z=-2\end{matrix}\right.\)

\(e,x^2+y^2-6x+4y+2=0\\ \Leftrightarrow\left(x-3\right)^2+\left(y+2\right)^2=11\)

\(\Rightarrow\)PT vô nghiệm vì 11 không phải là tổng 2 số chính phương

a) Ta có: \(36x^3-4x=0\)

\(\Leftrightarrow4x\left(9x^2-1\right)=0\)

\(\Leftrightarrow x\left(3x-1\right)\left(3x+1\right)=0\)

\(\Leftrightarrow\left[{}\begin{matrix}x=0\\x=\dfrac{1}{3}\\x=\dfrac{-1}{3}\end{matrix}\right.\)

b) Ta có: \(3x\left(x-2\right)+x-2=0\)

\(\Leftrightarrow\left(x-2\right)\left(3x+1\right)=0\)

\(\Leftrightarrow\left[{}\begin{matrix}x=2\\x=\dfrac{-1}{3}\end{matrix}\right.\)

d) Ta có: \(x^2-6x-16=0\)

\(\Leftrightarrow\left(x-8\right)\left(x+2\right)=0\)

\(\Leftrightarrow\left[{}\begin{matrix}x=8\\x=-2\end{matrix}\right.\)

e) Ta có: \(x^4-6x^2-7=0\)

\(\Leftrightarrow\left(x^2-7\right)\left(x^2+1\right)=0\)

\(\Leftrightarrow x\in\left\{\sqrt{7};-\sqrt{7}\right\}\)

a) TXĐ: R

y′ = 6x − 24 x 2  = 6x(1 − 4x)

y' = 0 ⇔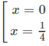 

y' > 0 trên khoảng (0; 1/4) , suy ra y đồng biến trên khoảng (0; 1/4)

y' < 0 trên các khoảng ( - ∞ ; 0 ); (14; + ∞ ), suy ra y nghịch biến trên các khoảng ( - ∞ ;0 ); (14; + ∞ )

b) TXĐ: R

y′ = 16 + 4x − 16 x 2  − 4 x 3  = −4(x + 4)( x 2  − 1)

y' = 0 ⇔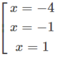 

Bảng biến thiên:

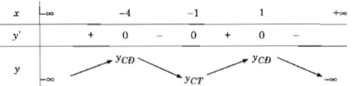
Vậy hàm số y đã cho đồng biến trên các khoảng ( - ∞ ; -4) và (-1; 1), nghịch biến trên các khoảng (-4; -1) và (1; + ∞ )

c) TXĐ: R

y′ = 3 x 2 − 12x + 9

y' = 0

y' > 0 trên các khoảng ( - ∞ ; 1), (3;  + ∞ ) nên y đồng biến trên các khoảng ( - ∞ ; 1), (3;  + ∞ )

y'< 0 trên khoảng (1; 3) nên y nghịch biến trên khoảng (1; 3)

d) TXĐ: R

y′ = 4 x 3  + 16 = 4x( x 2  + 4)

y' = 0 ⇔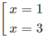 

y' > 0 trên khoảng (0;  + ∞ ) ⇒ y đồng biến trên khoảng (0;  + ∞ )

y' < 0 trên khoảng ( - ∞ ; 0) ⇒ y nghịch biến trên khoảng ( - ∞ ; 0)