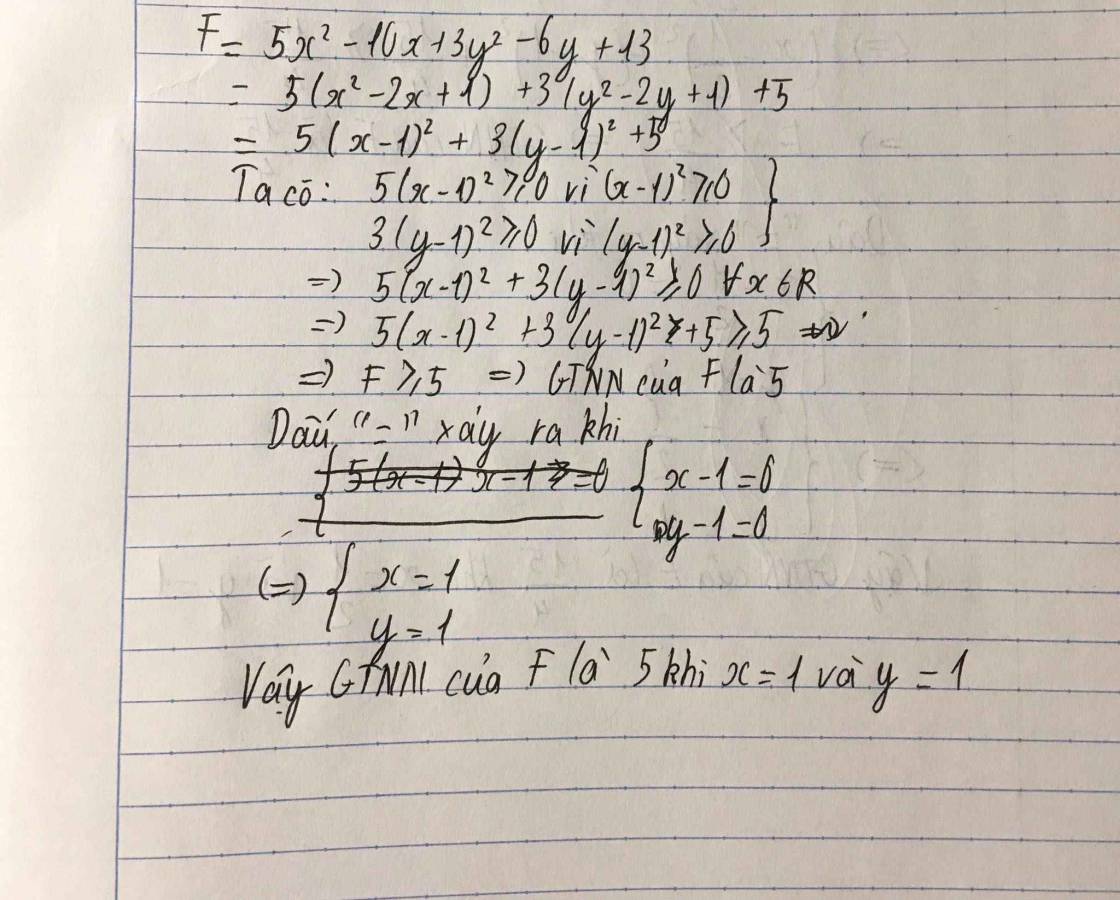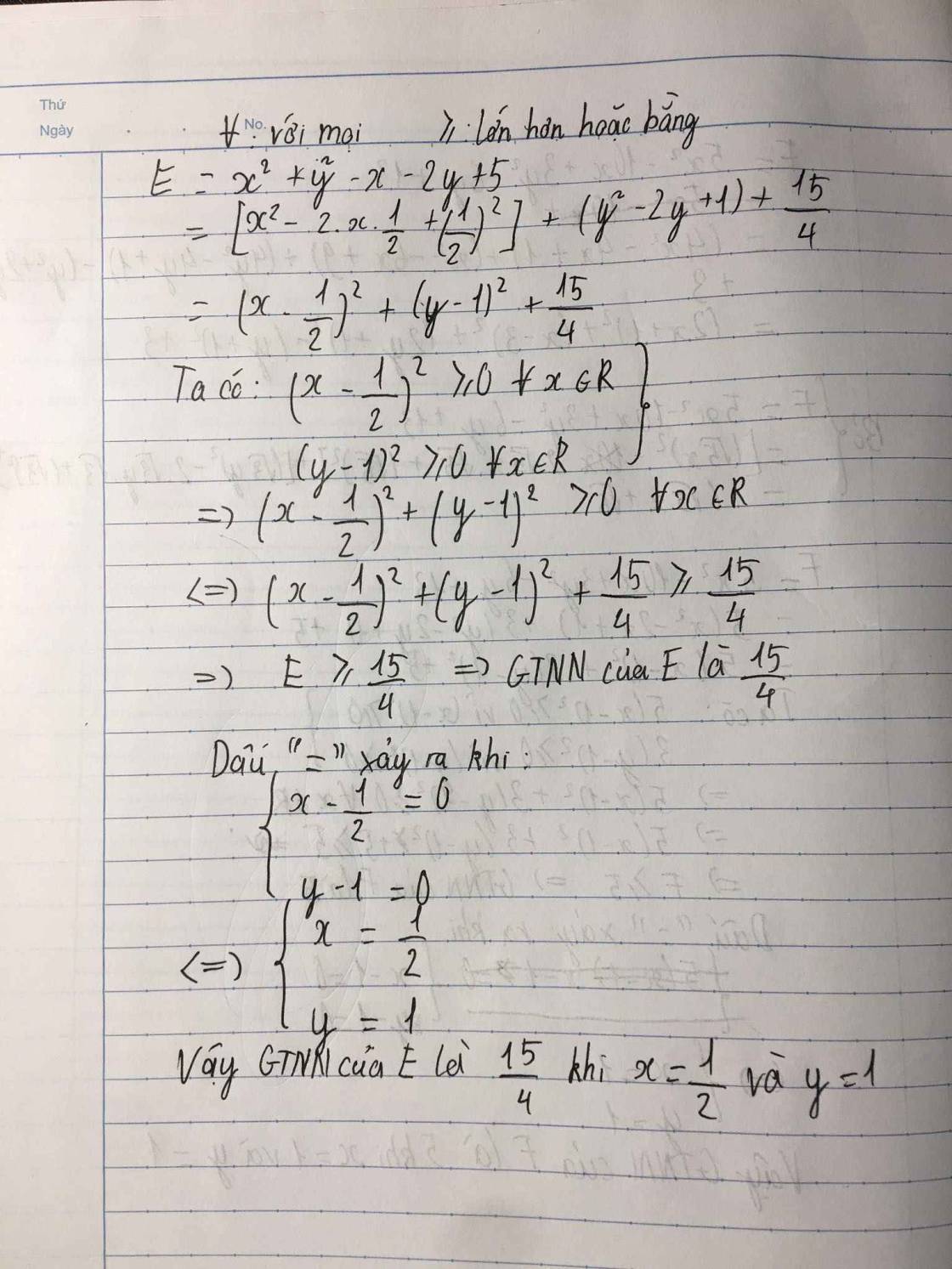a.

\(1-4x^2=\left(1-2x\right)\left(1+2x\right)\)

b.

\(8-27x^3=\left(2\right)^3-\left(3x\right)^3=\left(2-3x\right)\left(4+6x+9x^2\right)\)

c.

\(27+27x+9x^2+x^3=x^3+3.x^2.3+3.3^2.x+3^3\)

\(=\left(x+3\right)^3\)

d.

\(2x^3+4x^2+2x=2x\left(x^2+2x+1\right)=2x\left(x+1\right)^2\)

e.

\(x^2-y^2-5x+5y=\left(x-y\right)\left(x+y\right)-5\left(x-y\right)\)

\(=\left(x-y\right)\left(x+y-5\right)\)

f.

\(x^2-6x+9-y^2=\left(x-3\right)^2-y^2=\left(x-3-y\right)\left(x-3+y\right)\)

g. 10x(x-y)-6y(y-x)

=10x(x-y)+6y(x-y)

=(x-y)(10x+6y)

h.x²-4x-5

=(x-5)(x+1)

i.x⁴-y⁴ = (x²-y²)(x²+y²)

B2.

a.5(x-2)=x-2

⇔5(x-2)-(x-2)=0

⇔4(x-2)=0

⇔x=2

b.3(x-5)=5-x

⇔3(x-5)+(x-5)=0

⇔4(x-5)=0

⇔x=5

c.(x+2)²-(x+2)(x-2)=0

⇔(x+2)[(x+2)-(x-2)]=0

⇔4(x+2)=0

⇔x=-2

\(x^2+3y^2-4x+6y+7=0\\ \Leftrightarrow\left(x^2-4x+4\right)+\left(3y^2+6y+3\right)=0\\ \Leftrightarrow\left(x-2\right)^2+3\left(y+1\right)^2=0\\ \Leftrightarrow\left\{{}\begin{matrix}x-2=0\\y+1=0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=2\\y=-1\end{matrix}\right.\)

\(3x^2+y^2+10x-2xy+26=0\\ \Leftrightarrow\left(x^2-2xy+y^2\right)+\left(2x^2+10x+\dfrac{25}{8}\right)+\dfrac{183}{8}=0\\ \Leftrightarrow\left(x-y\right)^2+2\left(x^2+2\cdot\dfrac{5}{2}x+\dfrac{25}{4}\right)+\dfrac{183}{8}=0\\ \Leftrightarrow\left(x-y\right)^2+2\left(x+\dfrac{5}{2}\right)^2+\dfrac{183}{8}=0\\ \Leftrightarrow x,y\in\varnothing\)

Sửa đề: \(3x^2+6y^2-12x-20y+40=0\)

\(\Leftrightarrow\left(3x^2-12x+12\right)+\left(6y^2-20y+\dfrac{50}{3}\right)+\dfrac{34}{3}=0\\ \Leftrightarrow3\left(x-2\right)^2+6\left(y^2-2\cdot\dfrac{5}{3}y+\dfrac{25}{9}\right)+\dfrac{34}{3}=0\\ \Leftrightarrow3\left(x-2\right)^2+6\left(y-\dfrac{5}{3}\right)^2+\dfrac{34}{3}=0\\ \Leftrightarrow x,y\in\varnothing\)

\(2\left(x^2+y^2\right)=\left(x+y\right)^2\\ \Leftrightarrow2x^2+2y^2=x^2+2xy+y^2\\ \Leftrightarrow x^2-2xy+y^2=0\\ \Leftrightarrow\left(x-y\right)^2=0\Leftrightarrow x-y=0\Leftrightarrow x=y\)

xy là x.y hay là x và y vậy bn

X và y là số nguyên phải ko

a) (a - 2b)x(a + 2b)
b) x²-(y-3)²
 => (x-y+3)(x+y-3)
c) (2a + b - a)(2a + b + a)
=> (a+b)(3a+b)
d) (4(x - 1))² - (5(x + y))²
⇔ (4x - 4 - 5x - 5y)(4x - 4 + 5x + 5y)
⇔ -(x + 5y + 4)(9x + 5y + -4)
e) (x + 5)²
f) (5x - 2y)²
h) (x - 5)(x² + 5x + 25)

k) (x + 5)³

a: \(x^2+3y^2-4x+6y+7=0\)

\(\Leftrightarrow x^2-4x+4+3y^2+6y+3=0\)

\(\Leftrightarrow\left(x-2\right)^2+3\left(y+1\right)^2=0\)

\(\Leftrightarrow\left(x,y\right)=\left(-2;1\right)\)

Có thể thay đề bài từ tìm nghiệm nguyên thành tìm nghiệm.

Ta có: \(x^2-10x+29=\left(x-5\right)^2+4\ge4>0;y^2+6y+14=\left(y+3\right)^2+5\ge5>0\).

Từ đó \(\left(x^2-10x+29\right)\left(y^2+6y+14\right)\ge4.5=20\).

Do đẳng thức xảy ra nên ta phải có: \(\left\{{}\begin{matrix}\left(x-5\right)^2=0\\\left(y+3\right)^2=0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=5\\y=-3\end{matrix}\right.\).

Vậy...

a: ta có: \(P=x^2+10x+27\)

\(=x^2+10x+25+2\)

\(=\left(x+5\right)^2+2\ge2\forall x\)

Dấu '=' xảy ra khi x=-5