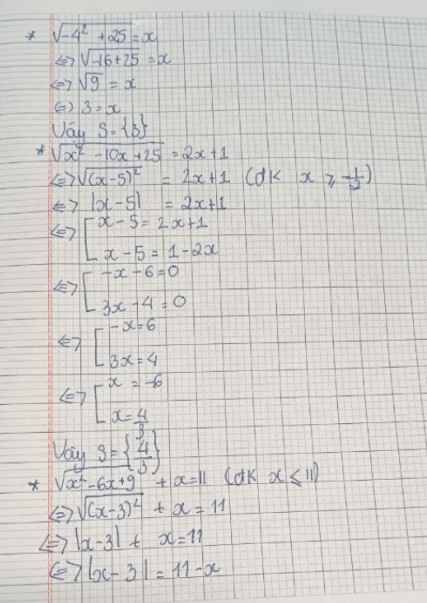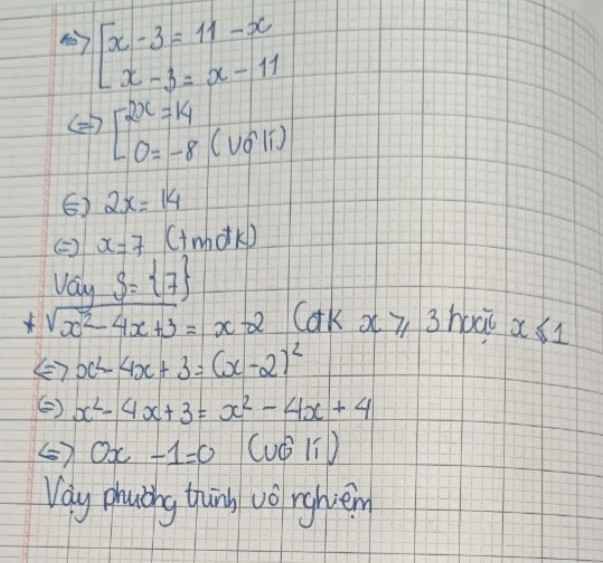1) ĐKXĐ: \(x\ge\dfrac{5}{2}\)

\(\sqrt{x^2}=2x-5\\ \Rightarrow\left|x\right|=2x-5\\ \Rightarrow\left[{}\begin{matrix}x=2x-5\\x=5-2x\end{matrix}\right.\\ \Rightarrow\left[{}\begin{matrix}x=5\left(tm\right)\\x=\dfrac{5}{3}\left(ktm\right)\end{matrix}\right.\)

2) ĐKXĐ: \(x\ge3\)

\(\sqrt{25x^2-10x+1}=2x-6\\ \Rightarrow\left|5x-1\right|=2x-6\\ \Rightarrow\left[{}\begin{matrix}5x-1=2x-6\\5x-1=6-2x\end{matrix}\right.\\ \Rightarrow\left[{}\begin{matrix}x=-\dfrac{5}{3}\left(ktm\right)\\x=1\left(tm\right)\end{matrix}\right.\)

3) ĐKXĐ: \(x\ge\dfrac{5}{2}\)

\(\sqrt{25-10x+x^2}=2x-5\\ \Rightarrow\left|x-5\right|=2x-5\\ \Rightarrow\left[{}\begin{matrix}x-5=2x-5\\x-5=5-2x\end{matrix}\right.\\ \Rightarrow\left[{}\begin{matrix}x=0\left(ktm\right)\\x=\dfrac{10}{3}\left(tm\right)\end{matrix}\right.\)

4) ĐKXĐ: \(x\ge\dfrac{1}{2}\)

\(\sqrt{1-2x+x^2}=2x-1\\ \Rightarrow\left|x-1\right|=2x-1\\ \Rightarrow\left[{}\begin{matrix}x-1=2x-1\\x-1=1-2x\end{matrix}\right.\\ \Rightarrow\left[{}\begin{matrix}x=0\left(ktm\right)\\x=\dfrac{2}{3}\left(tm\right)\end{matrix}\right.\)

a) Ta có: \(\sqrt{x-2\sqrt{x-1}}-\sqrt{x-1}=1\)

\(\Leftrightarrow\left|\sqrt{x-1}-1\right|=\sqrt{x-1}+1\)

\(\Leftrightarrow\sqrt{x-1}=\sqrt{x-1}+1+1\)(Vô lý)

Vậy: \(S=\varnothing\)

b) Ta có: \(\sqrt{x^4+2x^2+1}=\sqrt{x^2+10x+25}-10x+22\)

\(\Leftrightarrow x^2+1=\left|x+5\right|-10x+22\)

\(\Leftrightarrow\left|x+5\right|=x^2+1+10x-22=x^2+10x-21\)

\(\Leftrightarrow\left[{}\begin{matrix}x+5=x^2+10x-21\left(x\ge-5\right)\\-x-5=x^2+10x-21\left(x< -5\right)\end{matrix}\right.\)

\(\Leftrightarrow\left[{}\begin{matrix}x^2+10x-21-x-5=0\\x^2+10x-21+x+5=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x^2+9x-26=0\\x^2+11x-16=0\end{matrix}\right.\)

\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{-9+\sqrt{185}}{2}\\x=\dfrac{-11-\sqrt{185}}{2}\end{matrix}\right.\)

a: TH1: x>=2

A=x+x-2=2x-2

TH2: x<2

A=x+2-x=2

b: TH1: x>=3

A=x-3-x=-3

TH2: x<3

A=3-x-x=-2x+3

c: TH1: x>=1

C=x-x+1=1

TH2: x<1

C=x+x-1=2x-1

d: TH1: m>=3

C=m-3-2m=-3-m

TH2: m<3

C=-m+3-2m=-3m+3

e: TH1: m>=1

E=m-m+1=1

TH2: m<1

E=m+m-1=2m-1

√(x² + x + 1) = 1

⇔ x² + x + 1 = 1

⇔ x² + x = 0

⇔ x(x + 1) = 0

⇔ x = 0 hoặc x + 1 = 0

*) x + 1 = 0

⇔ x = -1

Vậy x = 0; x = -1

--------------------

√(x² + 1) = -3

Do x² ≥ 0 với mọi x

⇒ x² + 1 > 0 với mọi x

⇒ x² + 1 = -3 là vô lý

Vậy không tìm được x thỏa mãn yêu cầu

--------------------

√(x² - 10x + 25) = 7 - 2x

⇔ √(x - 5)² = 7 - 2x

⇔ |x - 5| = 7 - 2x  (1)

*) Với x ≥ 5, ta có 

(1) ⇔ x - 5 = 7 - 2x

⇔ x + 2x = 7 + 5

⇔ 3x = 12

⇔ x = 4 (loại)

*) Với x < 5, ta có:

(1) ⇔ 5 - x = 7 - 2x

⇔ -x + 2x = 7 - 5

⇔ x = 2 (nhận)

Vậy x = 2

--------------------

√(2x + 5) = 5

⇔ 2x + 5 = 25

⇔ 2x = 20

⇔ x = 20 : 2

⇔ x = 10

Vậy x = 10

-------------------

√(x² - 4x + 4) - 2x +5 = 0

⇔ √(x - 2)² - 2x + 5 = 0

⇔ |x - 2| - 2x + 5 = 0 (2)

*) Với x ≥ 2, ta có: 

(2) ⇔  x - 2 - 2x + 5 = 0

⇔ -x + 3 = 0

⇔ x = 3 (nhận)

*) Với x < 2, ta có:

(2) ⇔ 2 - x - 2x + 5 = 0

⇔ -3x + 7 = 0

⇔ 3x = 7

⇔ x = 7/3 (loại)

Vậy x = 3

1)

\(\Leftrightarrow x^2+x+1=1^2=1\\ \Leftrightarrow x^2+x=0\\ \Leftrightarrow x\left(x+1\right)=0\\ \Rightarrow\left\{{}\begin{matrix}x=0\\x=-1\end{matrix}\right.\)

2) Do \(x^2+1>0\forall x\) nên \(x\in\varnothing\)

3) 

\(\Leftrightarrow\sqrt{\left(x-5\right)^2}=7-2x\\ \Leftrightarrow\left|x-5\right|=7-2x\)

Nếu \(x\ge5\) thì

\(\Leftrightarrow x-5-7+2x=0\\ \Leftrightarrow3x-12=0\\ \Leftrightarrow3x=12\\ \Rightarrow x=4\)

=> Loại trường hợp này

Nếu \(x< 5\) thì

\(\Leftrightarrow5-x-7+2x=0\\ \Leftrightarrow x-2=0\\ \Rightarrow x=2\)

=> Nhận trường hợp này

Vậy x = 2 

4)

\(\Leftrightarrow2x+5=5^2=25\\ \Leftrightarrow2x=25-5=20\\ \Rightarrow x=\dfrac{20}{2}=10\)

5)

\(\Leftrightarrow\sqrt{\left(x-2\right)^2}-2x+5=0\\ \Leftrightarrow\left|x-2\right|-2x+5=0\)

Nếu \(x\ge2\) thì

\(\Leftrightarrow x-2-2x+5=0\\ \Leftrightarrow3-x=0\\ \Rightarrow x=3\)

=> Nhận trường hợp này

Nếu \(x< 2\) thì

\(\Leftrightarrow2-x-2x+5=0\\ \Leftrightarrow7-3x=0\\ \Leftrightarrow3x=7\\ \Rightarrow x=\dfrac{7}{3}\)

=> Loại trường hợp này

Vậy x = 3

Thiếu soát gì mog bạn thông cảm :]

do \(x^2+x+1=x^2+2.\frac{1}{2}x+\frac{1}{4}+\frac{3}{4}=\left(x+\frac{1}{2}\right)^2+\frac{3}{4}>0\forall x\)

\(\Rightarrow\sqrt{x^2+x+1}>0\forall x\)

voi dk \(x\ge-1\) ta co 

\(x^2+x+1=x^2+2x+1\Rightarrow x=0\)(tm)

b,\(\sqrt{4x^2-20x+25}+2x=5\)

\(\Leftrightarrow\sqrt{\left(2x-5\right)^2}+2x=5\)

    \(\Leftrightarrow\left|2x-5\right|+2x=5\)

th1 \(2x-5\ge0\Leftrightarrow x\ge\frac{5}{2}\) ta co\(2x-5+2x=5\Leftrightarrow4x=10\Rightarrow x=2.5\left(tm\right)\)

th2 \(2x-5< 0\Leftrightarrow x< \frac{5}{2}\) \(5-2x+2x=5\Leftrightarrow5=5\)

\(\Rightarrow\) dung voi moi \(x< \frac{5}{2}\)

kl \(x\le\frac{5}{2}\)

c, \(\left|x-1\right|=4\) \(\Rightarrow\orbr{\begin{cases}x-1=4\left(x\ge1\right)\\x-1=-4\left(x< 1\right)\end{cases}\Leftrightarrow\orbr{\begin{cases}x=5\left(tm\right)\\x=-3\left(tm\right)\end{cases}}}\)

d.\(\sqrt{3\left(x^2+2x+1\right)+4}+\sqrt{5\left(x^2+2x+1\right)+16}\)

 =\(\sqrt{3\left(x+1\right)^2+4}+\sqrt{5\left(x+1\right)^2+16}\ge\sqrt{4}+\sqrt{16}=6\)

ma \(-x^2-2x+5=-\left(x^2+2x+1\right)+6=-\left(x+1\right)^2+6\le6\)

dau = xay ra \(\Leftrightarrow x=-1\)

1) \(\sqrt{5-2x}=6\left(đk:x\le\dfrac{5}{2}\right)\)

\(\Leftrightarrow5-2x=36\)

\(\Leftrightarrow2x=-31\Leftrightarrow x=-\dfrac{31}{2}\left(tm\right)\)

2) \(\sqrt{2-x}=\sqrt{x+1}\left(đk:2\ge x\ge-1\right)\)

\(\Leftrightarrow2-x=x+1\)

\(\Leftrightarrow2x=1\Leftrightarrow x=\dfrac{1}{2}\left(tm\right)\)

3) \(\Leftrightarrow\sqrt{\left(2x+1\right)^2}=6\)

\(\Leftrightarrow\left|2x+1\right|=6\)

\(\Leftrightarrow\left[{}\begin{matrix}2x+1=6\\2x+1=-6\end{matrix}\right.\)

\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{5}{2}\\x=-\dfrac{7}{2}\end{matrix}\right.\)

4) \(\sqrt{x^2-10x+25}=x-2\left(đk:x\ge2\right)\)

\(\Leftrightarrow\sqrt{\left(x-5\right)^2}=x-2\)

\(\Leftrightarrow\left|x-5\right|=x-2\)

\(\Leftrightarrow\left[{}\begin{matrix}x-5=x-2\left(x\ge5\right)\\x-5=2-x\left(2\le x< 5\right)\end{matrix}\right.\)

\(\Leftrightarrow\left[{}\begin{matrix}5=2\left(VLý\right)\\x=\dfrac{7}{2}\left(tm\right)\end{matrix}\right.\)

a, \(\sqrt{4x^2+20x+25}\) + \(\sqrt{x^2-8x+16}\) = \(\sqrt{x^2+18x+81}\)

⇔ 4x² + 20x + 25 + \(2\sqrt{\left(4x^2+20x+25\right)\left(x^2-8x+16\right)}\) = x² + 18x + 81

⇔ 4x² + 20x + 25 - x² - 18x - 81 + \(2\sqrt{\left(2x+5\right)^2.\left(x-4\right)^2}\) = 0

⇔ 3x² + 2x - 56 + 2.(2x + 5) . (x - 4) = 0

⇔ 3x² + 2x - 56 + (4x + 10) . (x - 4) = 0

⇔ 3x² + 2x - 56 + 4x² - 16x + 10x - 40 = 0

⇔ 7x² - 4x - 96 = 0

x₁ = 4 ( nhận )

x₂ = \(\frac{-24}{7}\) ( nhận )

Vậy: S = {4; \(\frac{-24}{7}\)}