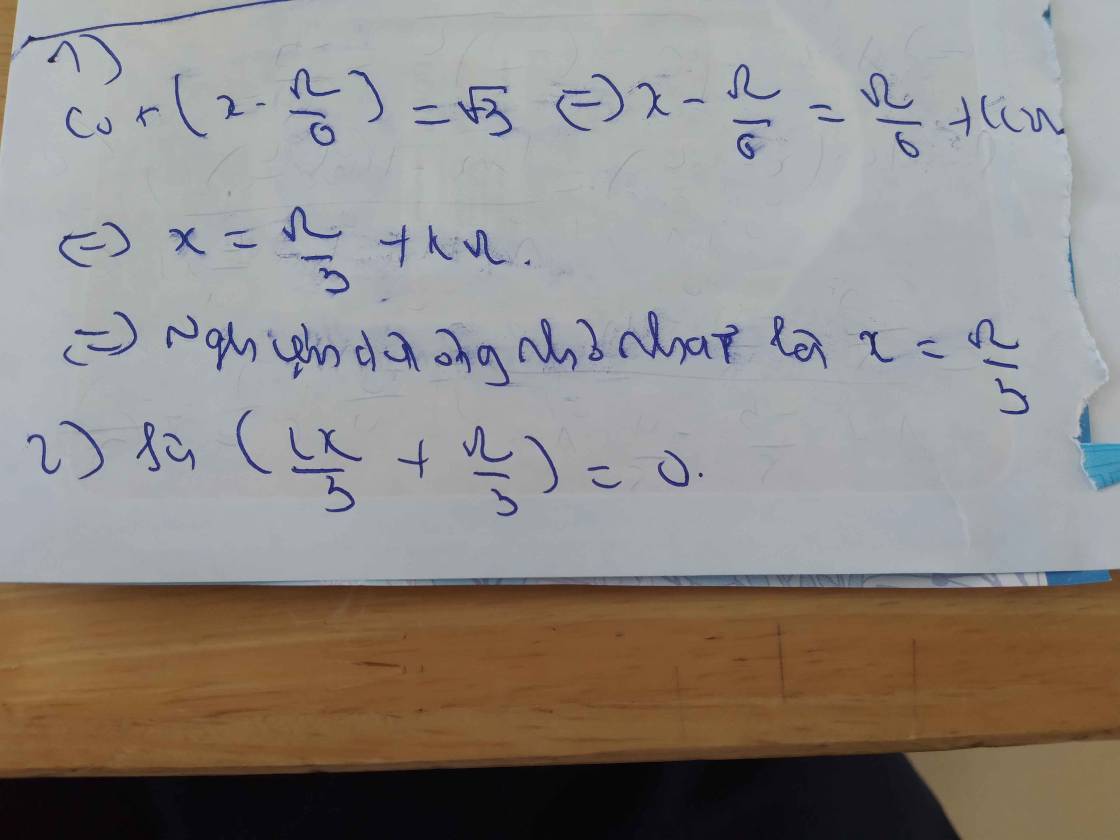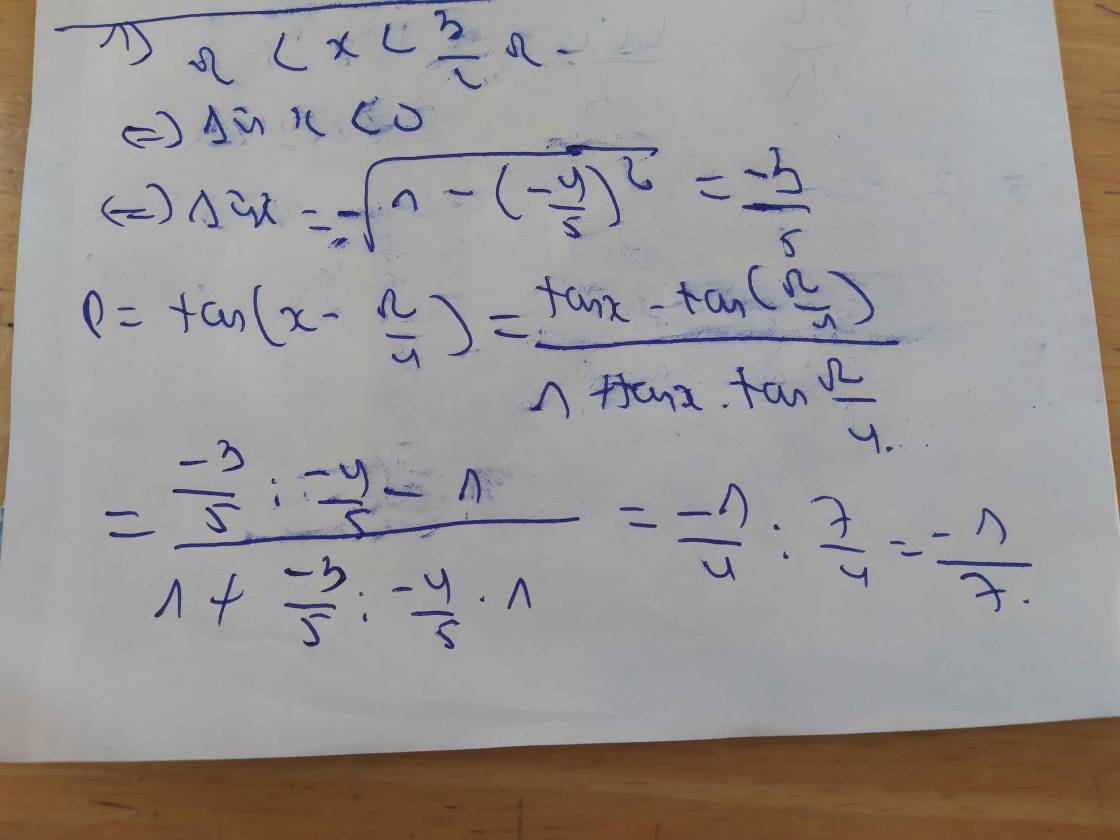Gỉai nghiệm của phương trình: y= 3cos + 3sin(x+\(\dfrac{\pi}{7}\)) = 0

Những câu hỏi liên quan
Câu 1: Phương trình lượng giác: sin^2 x - 3cos x - 4 = 0 có nghiệm là: A. x=- pi 2 +k 2 pi B. x=- pi+k2 pi C. x = pi/6 + k*pi D.Vô nghiệm
1.D
sin2x - 3cosx - 4 = 0
1-cos2x - 3cosx - 4 = 0
cos2x + 3 cosx + 3 = 0
Vô nghiệm
Đúng 0
Bình luận (0)
1) nghiệm dương nhỏ nhất của phương trình \(cot\left(x-\dfrac{\pi}{6}\right)=\sqrt{3}\) là
2) phương trình \(sin\left(\dfrac{2x}{3}+\dfrac{\pi}{3}\right)=0\) có nghiệm là
3) họ nghiệm của phương trình \(cot\)(2x - 30 độ) = \(\sqrt{3}\) là
Số nghiệm của phương trình : \(\sin3x+\cos3x+2\cos x=0\) thuộc \(\left[-\dfrac{\pi}{2};\dfrac{\pi}{2}\right]\) là
\(\Leftrightarrow3sinx-4sin^3x+4cos^3x-3cosx+2cosx=0\)
\(\Leftrightarrow3sinx-cosx-4sin^3x+4cos^3x=0\)
Với \(cosx=0\) ko phải nghiệm, với \(cosx\ne0\) chia 2 vế cho \(cos^3x\)
\(\Leftrightarrow3tanx\left(1+tan^2x\right)-\left(1+tan^2x\right)-4tan^3x+4=0\)
\(\Leftrightarrow-tan^3x-tan^2x+3tanx+3=0\)
\(\Leftrightarrow-tan^2x\left(tanx+1\right)+3\left(tanx+1\right)=0\)
\(\Leftrightarrow\left(tanx+1\right)\left(3-tan^2x\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}tanx=-1\\tanx=\sqrt{3}\\tanx=-\sqrt{3}\end{matrix}\right.\)
Tới đây chắc bạn hoàn thành được phần còn lại
Đúng 1
Bình luận (1)
Câu 1: Tích các nghiệm trên khoảng left(dfrac{pi}{4};dfrac{7pi}{4}right)của phương trình cos2x-3cosx+20 Câu 2: Tìm tất cả các giá trị thực của tham số m để phương trình 2cos^23x+left(3-2mright)cos3x+m-20 có đúng 3 nghiệm thuộc khoảng left(-dfrac{pi}{6};dfrac{pi}{3}right).Câu 3: Tính tổng T tất cả các nghiệm của phương trình 2sin^2dfrac{x}{4}-3cosdfrac{x}{4}0 trên đoạn left[0;8piright].Câu 4: Giá trị của m để phương trình cos2x-left(2m+1right)sinx-m-10 có nghiệm trên khoảng left(0;piright) là min...
Đọc tiếp
Câu 1: Tích các nghiệm trên khoảng \(\left(\dfrac{\pi}{4};\dfrac{7\pi}{4}\right)\)của phương trình \(cos2x-3cosx+2=0\)
Câu 2: Tìm tất cả các giá trị thực của tham số m để phương trình \(2cos^23x+\left(3-2m\right)cos3x+m-2=0\) có đúng 3 nghiệm thuộc khoảng \(\left(-\dfrac{\pi}{6};\dfrac{\pi}{3}\right)\).
Câu 3: Tính tổng T tất cả các nghiệm của phương trình \(2sin^2\dfrac{x}{4}-3cos\dfrac{x}{4}=0\) trên đoạn \(\left[0;8\pi\right]\).
Câu 4: Giá trị của m để phương trình \(cos2x-\left(2m+1\right)sinx-m-1=0\) có nghiệm trên khoảng \(\left(0;\pi\right)\) là \(m\in[a;b)\) thì a+b là?
Câu 5: Điều kiện cần và đủ để phương trình \(msinx-3cosx=5\) có nghiệm là \(m\in(-\infty;a]\cup[b;+\infty)\) với \(a,b\in Z\). Tính a+b.
Câu 6: Điều kiện để phương trình \(msinx-3cosx=5\) có nghiệm là?
Câu 7: Số nghiệm để phương trình \(sin2x+\sqrt{3}cos2x=\sqrt{3}\) trên khoảng \(\left(0;\dfrac{\pi}{2}\right)\) là?
Câu 8: Tập giá trị của hàm số \(y=\dfrac{sinx+2cosx+1}{sinx+cosx+2}\) là?
Câu 9: Có bao nhiêu giá trị nguyên của tham số m thuộc đoạn \(\left[-2018;2018\right]\) dể phương trình \(\left(m+1\right)sin^2-sin2x+cos2x=0\) có nghiệm?
Câu 10: Có bao nhiêu giá trị nguyên của tham số m để phương trình \(sin2x-cos2x+|sinx+cosx|-\sqrt{2cos^2x+m}-m=0\) có nghiệm thực?
1.
\(cos2x-3cosx+2=0\)
\(\Leftrightarrow2cos^2x-3cosx+1=0\)
\(\Leftrightarrow\left[{}\begin{matrix}cosx=1\\cosx=\dfrac{1}{2}\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=k2\pi\\x=\pm\dfrac{\pi}{3}+k2\pi\end{matrix}\right.\)
\(x=k2\pi\in\left[\dfrac{\pi}{4};\dfrac{7\pi}{4}\right]\Rightarrow\) không có nghiệm x thuộc đoạn
\(x=\pm\dfrac{\pi}{3}+k2\pi\in\left[\dfrac{\pi}{4};\dfrac{7\pi}{4}\right]\Rightarrow x_1=\dfrac{\pi}{3};x_2=\dfrac{5\pi}{3}\)
\(\Rightarrow P=x_1.x_2=\dfrac{5\pi^2}{9}\)
Đúng 1
Bình luận (0)
2.
\(pt\Leftrightarrow\left(cos3x-m+2\right)\left(2cos3x-1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}cos3x=\dfrac{1}{2}\left(1\right)\\cos3x=m-2\left(2\right)\end{matrix}\right.\)
\(\left(1\right)\Leftrightarrow x=\pm\dfrac{\pi}{9}+\dfrac{k2\pi}{3}\)
Ta có: \(x=\pm\dfrac{\pi}{9}+\dfrac{k2\pi}{3}\in\left(-\dfrac{\pi}{6};\dfrac{\pi}{3}\right)\Rightarrow x=\pm\dfrac{\pi}{9}\)
Yêu cầu bài toán thỏa mãn khi \(\left(2\right)\) có nghiệm duy nhất thuộc \(\left(-\dfrac{\pi}{6};\dfrac{\pi}{3}\right)\)
\(\Leftrightarrow\left[{}\begin{matrix}m-2=0\\m-2=1\\m-2=-1\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}m=2\\m=3\\m=1\end{matrix}\right.\)
TH1: \(m=2\)
\(\left(2\right)\Leftrightarrow cos3x=0\Leftrightarrow x=\dfrac{\pi}{6}+\dfrac{k2\pi}{3}\in\left(-\dfrac{\pi}{6};\dfrac{\pi}{3}\right)\Rightarrow x=\dfrac{\pi}{6}\left(tm\right)\)
\(\Rightarrow m=2\) thỏa mãn yêu cầu bài toán
TH2: \(m=3\)
\(\left(2\right)\Leftrightarrow cos3x=0\Leftrightarrow x=\dfrac{k2\pi}{3}\in\left(-\dfrac{\pi}{6};\dfrac{\pi}{3}\right)\Rightarrow x=0\left(tm\right)\)
\(\Rightarrow m=3\) thỏa mãn yêu cầu bài toán
TH3: \(m=1\)
\(\left(2\right)\Leftrightarrow cos3x=-1\Leftrightarrow x=\dfrac{\pi}{3}+\dfrac{k2\pi}{3}\in\left(-\dfrac{\pi}{6};\dfrac{\pi}{3}\right)\Rightarrow\left[{}\begin{matrix}x=\pm\dfrac{1}{3}\\x=-1\\x=-\dfrac{5}{3}\end{matrix}\right.\)
\(\Rightarrow m=2\) không thỏa mãn yêu cầu bài toán
Vậy \(m=2;m=3\)
Đúng 2
Bình luận (0)
3.
\(2sin^2\dfrac{x}{4}-3cos\dfrac{x}{4}=0\)
\(\Leftrightarrow2cos^2\dfrac{x}{4}+3cos\dfrac{x}{4}-2=0\)
\(\Leftrightarrow\left[{}\begin{matrix}cos\dfrac{x}{4}=\dfrac{1}{2}\\cos\dfrac{x}{4}=-2\left(l\right)\end{matrix}\right.\)
\(\Leftrightarrow x=\pm\dfrac{4\pi}{3}+k8\pi\in\left[0;8\pi\right]\)
\(\Rightarrow\left[{}\begin{matrix}x=\dfrac{4\pi}{3}\\x=\dfrac{20\pi}{3}\end{matrix}\right.\)
\(\Rightarrow T=\dfrac{4\pi}{3}+\dfrac{20\pi}{3}=8\pi\)
Đúng 0
Bình luận (0)
Nghiệm của phương trình \(sin^4x+cos^4x+cos\left(x-\dfrac{\pi}{4}\right).sin\left(3x-\dfrac{\pi}{4}\right)-\dfrac{3}{2}=0\)
\(\Leftrightarrow\left(sin^2x+cos^2x\right)^2-2sin^2x.cos^2x+\dfrac{1}{2}sin\left(4x-\dfrac{\pi}{2}\right)+\dfrac{1}{2}sin2x-\dfrac{3}{2}=0\)
\(\Leftrightarrow1-\dfrac{1}{2}sin^22x-\dfrac{1}{2}cos4x+\dfrac{1}{2}sin2x-\dfrac{3}{2}=0\)
\(\Leftrightarrow1-\dfrac{1}{2}\left(\dfrac{1-cos4x}{2}\right)-\dfrac{1}{2}cos4x+\dfrac{1}{2}sin2x-\dfrac{3}{2}=0\)
\(\Leftrightarrow-\dfrac{3}{4}-\dfrac{1}{4}cos4x+\dfrac{1}{2}sin2x=0\)
\(\Leftrightarrow-\dfrac{3}{4}-\dfrac{1}{4}\left(1-2sin^22x\right)+\dfrac{1}{2}sin2x=0\)
\(\Leftrightarrow...\)
Đúng 2
Bình luận (0)
1) cho góc x thỏa mãn \(cosx=-\dfrac{4}{5}\) và \(\pi< x< \dfrac{3\pi}{2}\) tính \(P=tan\left(x-\dfrac{\pi}{4}\right)\)
2) giải phương trình \(2cosx-\sqrt{2}=0\)
3) phương trình lượng giác \(cos3x=cos\dfrac{\pi}{15}\) có nghiệm là
Phương trình cos5x.cosx = cos4x.cos2x + 3cos^2x - 1 có các nghiệm thuộc (-pi: pi) là
Nghiệm của phương trình 2sin2 x- 3sin x+ 1 0 thỏa điều kiện:
0
≤
x
π
2
A. x
π
/6 B. x
π
/4 C. x
π
/2 D. x -
π
/2
Đọc tiếp
Nghiệm của phương trình 2sin2 x- 3sin x+ 1= 0 thỏa điều kiện: 0 ≤ x < π 2
A. x = π /6
B. x = π /4
C. x = π /2
D. x = - π /2

Vì 0 ≤ x < π 2 nên nghiệm của phương trình là x = π /6
Đúng 0
Bình luận (0)
Cho phương trình \(2cos^2x+cos4x=0\). Trong các số sau, số nào là họ nghiệm của phương trình trên:
\(I.x=\dfrac{\pi}{6}+k\dfrac{\pi}{4}II.x=-\dfrac{\pi}{6}+k\dfrac{\pi}{2}III.x=\dfrac{\pi}{6}+k\dfrac{\pi}{2}IV.x=-\dfrac{\pi}{6}+k\dfrac{\pi}{4}\)
Chọn câu trả lời đúng nhất.
A. Chỉ I, IV đúng
B. Chỉ I đúng
C. Chỉ IV đúng
D. I, II, III, IV cùng đúng
\(\Leftrightarrow cos2x+1+2cos^22x-1=0\)
\(\Leftrightarrow cos2x\left(2cos2x+1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}cos2x=0\\cos2x=-\dfrac{1}{2}\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}2x=\dfrac{\pi}{2}+k\pi\\2x=\pm\dfrac{2\pi}{3}+k2\pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{\pi}{4}+\dfrac{k\pi}{2}\\x=\pm\dfrac{\pi}{3}+k\pi\end{matrix}\right.\)
Không nghiệm nào trong 4 đáp án đúng
Hoặc là đề sai, hoặc là đáp án sai
Đúng 1
Bình luận (0)