b) –x(x – 2) + 4 = (x + 1)(1 – x)
c) 16x^2 – (x – 1)^2 = 0
giúp mình vớii
c) (x2 + 1). (x + 2) > 0
d) (x + 4). (x – 3) < 0
e) ( x2 + 2). (x2 + 5) < 0
f) (x + 6). (x – 4) > 0
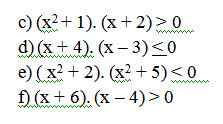 Giúp mình với, mình cần gấp ạ
Giúp mình với, mình cần gấp ạ
Hai bài bị trùng nhau nên các bạn nhìn ảnh hay văn bản đều như nhau ạ
c: =>x+2>0
hay x>-2
d: =>-4<=x<=3
e: =>\(x\in\varnothing\)
f: \(\Leftrightarrow\left[{}\begin{matrix}x>4\\x< -6\end{matrix}\right.\)
giải pt:
a) x^4+4x³+6x²+4x+ căn(x²+2x+10)=2
b) x²=căn(x³-x²)+căn(x²-x)
c) căn(x-1)+căn(3-x) + x²+2x-3- √2=0
GIÚP MÌNH
a) PT \(\Leftrightarrow\left(x+1\right)^4+\sqrt{\left(x+1\right)^2+9}=3\).
Ta có \(\left(x+1\right)^4+\sqrt{\left(x+1\right)^2+9}\ge\sqrt{9}=3\).
Đẳng thức xảy ra khi và chỉ khi x = -1.
Vậy..
b) \(x^2=\sqrt{x^3-x^2}+\sqrt{x^2-x}\)
Đk: \(\left\{{}\begin{matrix}x^3-x^2\ge0\\x^2-x\ge0\end{matrix}\right.\)\(\Leftrightarrow\left\{{}\begin{matrix}x^2\left(x-1\right)\ge0\\x\left(x-1\right)\ge0\end{matrix}\right.\)\(\Leftrightarrow\left\{{}\begin{matrix}\left[{}\begin{matrix}x\ge1\\x=0\end{matrix}\right.\\\left[{}\begin{matrix}x\ge1\\x\le0\end{matrix}\right.\end{matrix}\right.\)\(\Leftrightarrow\left[{}\begin{matrix}x=0\\x\ge1\end{matrix}\right.\)
Thay x=0 vào pt thấy thỏa mãn => x=0 là một nghiệm của pt
Xét \(x\ge1\)
Pt \(\Leftrightarrow x^4=\left(\sqrt{x^3-x^2}+\sqrt{x^2-x}\right)^2\le2\left(x^3-x\right)\) (Theo bđt bunhiacopxki)
\(\Leftrightarrow x^4\le2x\left(x^2-1\right)\le\left(x^2+1\right)\left(x^2-1\right)=x^4-1\)
\(\Leftrightarrow0\le-1\) (vô lí)
Vậy x=0
c) \(\sqrt{x-1}+\sqrt{3-x}+x^2+2x-3-\sqrt{2}=0\) (đk: \(1\le x\le3\))
Xét x-1=0 <=> x=1 thay vào pt thấy thỏa mãn => x=1 là một nghiệm của pt
Xét \(x\ne1\)
Pt\(\Leftrightarrow\dfrac{x-1}{\sqrt{x-1}}+\dfrac{1-x}{\sqrt{3-x}+\sqrt{2}}+\left(x-1\right)\left(x+3\right)=0\)
\(\Leftrightarrow\left(x-1\right)\left(\dfrac{1}{\sqrt{x-1}}-\dfrac{1}{\sqrt{3-x}+\sqrt{2}}+x+3\right)=0\) (1)
Xét \(\dfrac{1}{\sqrt{x-1}}-\dfrac{1}{\sqrt{3-x}+\sqrt{2}}+x+3\)
Có \(\sqrt{3-x}+\sqrt{2}\ge\sqrt{2}\)
\(\Leftrightarrow\dfrac{-1}{\sqrt{3-x}+\sqrt{2}}\ge-\dfrac{1}{\sqrt{2}}\)
Có \(\left\{{}\begin{matrix}\dfrac{1}{\sqrt{x-1}}>0\\x+3\ge4\end{matrix}\right.\) \(\Rightarrow\dfrac{1}{\sqrt{x-1}}-\dfrac{1}{\sqrt{3-x}+\sqrt{2}}+x+3>0-\dfrac{1}{\sqrt{2}}+4>0\)
Từ (1) => x-1=0 <=> x=1
Vậy pt có nghiệm duy nhất x=1
(x^2+1)(x^2-4)=0
Giúp mình với mình đang gấp
Vì \(x^2+1>0\) nên \(x^2-4=0\)
\(\Leftrightarrow x^2=4\Leftrightarrow\left[{}\begin{matrix}x=2\\x=-2\end{matrix}\right.\)
Bài1: giải các pt sau:
a, 3-4x+24+6x= x+27+3x
b, 5-(6-x)=4(3-2x)
c, x-(x+1)/3 = (2x+1)/5
d,(2x-1)/3 - (5x+2)/7 = x+13
Bài 2:
a, (x-1)(3x+1)=0
b, (x-5)(7-x)=0
c, ( x-1)(x+5)(-3x+8)=0
d, x(x^2 - 1 )=0
Giúp mình 2 bài này với , mình đang cần gấp , CẢM ƠN M.N ạ><
2:
a: =>x-1=0 hoặc 3x+1=0
=>x=1 hoặc x=-1/3
b: =>x-5=0 hoặc 7-x=0
=>x=5 hoặc x=7
c: =>\(\left[{}\begin{matrix}x-1=0\\x+5=0\\3x-8=0\end{matrix}\right.\Leftrightarrow x\in\left\{1;-5;\dfrac{8}{3}\right\}\)
d: =>x=0 hoặc x^2-1=0
=>\(x\in\left\{0;1;-1\right\}\)
cho biểu thức a=(4/x-1):(1-x-3/x^2+x+1) với x khác 1 1 rút gọn a 2 tính giá trị a với x thỏa mãn x^4 -7x^2-4x+20=0
giúp mình với helppp
a: \(A=\left(\dfrac{4}{x}-1\right):\left(1-\dfrac{x-3}{x^2+x+1}\right)\)
\(=\dfrac{4-x}{x}:\dfrac{x^2+x+1-x+3}{x^2+x+1}\)
\(=\dfrac{4-x}{x}\cdot\dfrac{x^2+x+1}{x^2+4}=\dfrac{\left(4-x\right)\left(x^2+x+1\right)}{x\left(x^2+4\right)}\)
b: x^4-7x^2-4x+20=0
=>(x-2)^2(x^2+4x+5)=0
=>x=2
Khi x=2 thì \(A=\dfrac{\left(4-2\right)\left(4+2+1\right)}{2\left(4+4\right)}=\dfrac{7}{8}\)
a) (3x-1).(2x+7)-(x+1).(6x-5)=7
b) (x+1)^3-x.(x-2)^2+x-1=0
c) (x+2).(x^2-2x+4)-x(x^2+3)
d) 2x.(x-3)-5.(3-x)=0
giúp mình ạ cần gấp
b) (x+1)^3-x(x-2)^2+x-1=0
⇔x^3+3x^2+3x+1-(x^3-4x^2+4x)=0
⇔ x^3+3x^2+3x+1-x^3+4x^2-4x+x-1=0
⇔7x^2-2=0
⇔7x^2=2
⇔7x^2=-2⇔x=-3
⇔7x^2=2⇔x=-căn 5
Bài 1: Tìm x
a) x - 452 = 77 + 48
b) x + 58 = 64 + 58
c) x - 1 - 2 - 3 - 4 = 0
Giúp mình với
a) x - 452 = 77 + 48
x - 452 = 125
x= 125 + 452
x= 577
b) x + 58 = 64 + 58
x + 58 = 122
x= 122 - 58
x= 64
c) x - 1 - 2 - 3 - 4 = 0
x= 0 + 4 + 3 + 2 +1
x= 10
a)x-452=77+48
x-452=125
x=125+452
x=577
b)x+58=64+58
x+58=122
x=122-58
x=64
c)x-1-2-3-4=0
x=0+4+3+2+1
x=10
Nhớ tick cho mk nha^^
a) x^2 - 25 - 3 *(x - 5) =0
b) (3x + 1 )^2 = (2x - 5 )
c) 2x^2 - 7x + 6 = 0
giúp mình vs
*đừng giải tắt nha*
mình cần gấp
a. \(x^2-25-3.\left(x-5\right)=0\\ \Leftrightarrow\left(x-5\right)\left(x+5\right)-3.\left(x-5\right)=0\\ \Leftrightarrow\left(x-5\right)\left(x+5-3\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x-5=0\\x+2=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=5\\x=-2\end{matrix}\right.\)
b. \(\left(3x+1\right)^2=\left(2x-5\right)\\ \Leftrightarrow9x^2+6x+1=2x-5\\ \Leftrightarrow9x^2+6x-2x=-5-1\\ \Leftrightarrow9x^2+4x=-6\\ \Leftrightarrow x\left(9x+4\right)=-6\\ \Leftrightarrow\left[{}\begin{matrix}x=-6\\9x+4=-6\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=-6\\x=-\dfrac{10}{9}\end{matrix}\right.\)
c. \(2x^2-7x+6=0\\ \Leftrightarrow2x^2-7x=-6\\ \Leftrightarrow x\left(2x-7\right)=-6\\ \Leftrightarrow\left[{}\begin{matrix}x=-6\\x=\dfrac{1}{2}\end{matrix}\right.\)
a, \(\left(x-5\right)\left(x+5\right)-3\left(x-5\right)=0\Leftrightarrow\left(x-5\right)\left(x+2\right)=0\Leftrightarrow x=-2;x=5\)
b, bạn ktra lại đề, thường thường ngta hay cho 2 vế cùng bình phương
c, \(2x^2-7x+6=0\Leftrightarrow\left(2x-3\right)\left(x-2\right)=0\Leftrightarrow x=\dfrac{3}{2};x=2\)
\(\left(3x+1\right)^2=\left(2x-5\right)^2\)
\(\left[{}\begin{matrix}3x+1=2x-5\\3x+1=5-2x\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=-6\\5x=4\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=-6\\x=\dfrac{4}{5}\end{matrix}\right.\)
TÌM x
1, (3 – x)2 + (2x + 1)2 – (2 – x)2 – (2x + 1)2 = 0
2, (1 – 2x)2 – 3(x – 1)2 + (x + 1)2 – (1 – x)2 – (x – 1)2 = 0
GIÚP MÌNH VỚI, THANKS NHÌU NHÌU Ạ !!!^^
1: Ta có: \(\left(3-x\right)^2+\left(2x+1\right)^2-\left(2-x\right)^2-\left(2x+1\right)^2=0\)
\(\Leftrightarrow\left(x-3\right)^2-\left(x-2\right)^2=0\)
\(\Leftrightarrow\left(x-3+x-2\right)=0\)
\(\Leftrightarrow x=\dfrac{5}{2}\)
2: Ta có: \(\left(1-2x\right)^2-3\left(x-1\right)^2+\left(x+1\right)^2-\left(x-1\right)^2-\left(x-1\right)^2=0\)
\(\Leftrightarrow4x^2-4x+1-3x^2+6x-3+\left(x+1\right)^2-2\left(x-1\right)^2=0\)
\(\Leftrightarrow x^2+2x-2+x^2+2x+1-2\left(x^2-2x+1\right)=0\)
\(\Leftrightarrow2x^2+4x+1-2x^2+4x-2=0\)
\(\Leftrightarrow x=\dfrac{1}{8}\)