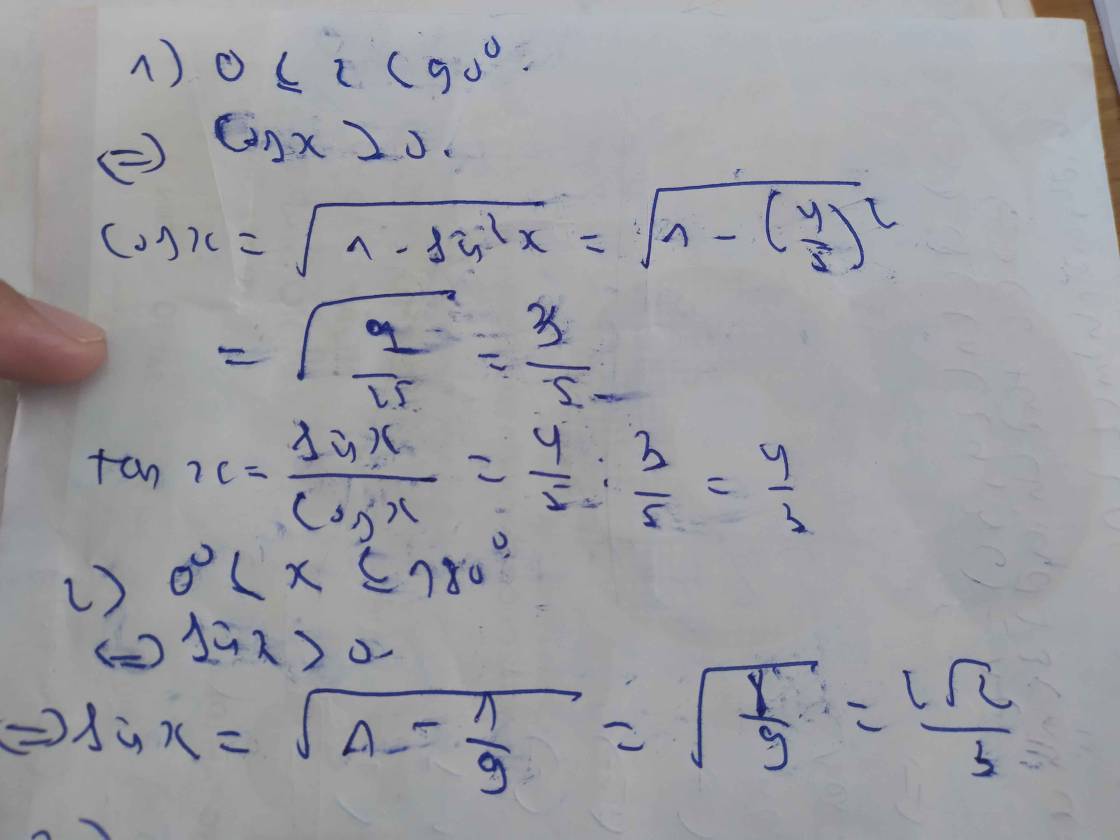Cho Sin x = 2/5 và 90° < x < 180° . Tính giá trị cosx (chỉ tui cách để tính bài này đi mn)

Những câu hỏi liên quan
1) cho góc x (0 độ le x 90 độ) thỏa mãn sinxdfrac{4}{5} giá trị của tanx là 2) cho góc x (0 độ le x le 180 độ) thỏa mãn cosxdfrac{1}{3} giá trị của sinx là3) cho cosxdfrac{1}{2} tính P3sin^2x+4cos^2x
Đọc tiếp
1) cho góc x (0 độ \(\le\) x < 90 độ) thỏa mãn \(sinx=\dfrac{4}{5}\) giá trị của \(tanx\) là
2) cho góc x (0 độ \(\le\) x \(\le\) 180 độ) thỏa mãn \(cosx=\dfrac{1}{3}\) giá trị của \(sinx\) là
3) cho \(cosx=\dfrac{1}{2}\) tính \(P=3sin^2x+4cos^2x\)
Cho tan²x+cot²x=2. Tính giá trị của biểu thức P=1/cosx–cosx/1+sin x
Xem chi tiết
\(tan^2x+cot^2x=2=2.tanx.cotx\)
\(\Leftrightarrow tan^2x+cot^2x-2tanx.cotx=0\)
\(\Leftrightarrow\left(tanx-cotx\right)^2=0\Leftrightarrow tanx=cotx=\dfrac{1}{tanx}\)
\(\Leftrightarrow tanx=\pm1\)
\(P=\dfrac{1}{cosx}-\dfrac{cosx}{1+sinx}=\dfrac{1+sinx-cos^2x}{cosx\left(1+sinx\right)}=\dfrac{sin^2x+sinx}{cosx\left(1+sinx\right)}\)
\(=\dfrac{sinx\left(1+sinx\right)}{cosx\left(1+sinx\right)}=tanx=\pm1\)
Đúng 1
Bình luận (0)
Tìm các giá trị lượng giác còn lại biết:
a) Cho sin \(x=-\dfrac{4}{5}\)và \(90^o< x< 180^o\)
b) Cho \(\sin x=\dfrac{\sqrt{3}}{2}\)và \(270^o< x< 360^o\)
c) Cho \(\cos x=-\dfrac{1}{3}\)và \(0^o< x< 90^o\)
a: Sửa đề: sin x=4/5
cosx=-3/5; tan x=-4/3; cot x=-3/4
b: 270 độ<x<360 độ
=>cosx>0
=>cosx=1/2
tan x=căn 3; cot x=1/căn 3
Đúng 0
Bình luận (0)
Cho Sin a (alpha) = 3/5 và 90° < a < 180°. Tính giá trị biểu thức A= 2cos²a - 5tan²a
\(90^0< a< 180^0\)
=>\(cosa< 0\)
\(sin^2a+cos^2a=1\)
=>\(cos^2a+\dfrac{9}{25}=1\)
=>\(cos^2a=1-\dfrac{9}{25}=\dfrac{16}{25}\)
mà cosa<0
nên \(cosa=-\dfrac{4}{5}\)
\(tana=\dfrac{sina}{cosa}=\dfrac{3}{5}:\dfrac{-4}{5}=-\dfrac{3}{4}\)
\(A=2\cdot cos^2a-5\cdot tan^2a\)
\(=2\cdot\left(-\dfrac{4}{5}\right)^2-5\cdot\left(-\dfrac{3}{4}\right)^2\)
\(=2\cdot\dfrac{16}{25}-5\cdot\dfrac{9}{16}\)
\(=\dfrac{32}{25}-\dfrac{45}{16}=\dfrac{-613}{400}\)
Đúng 0
Bình luận (0)
Câu 1 : Giá trị lớn nhất của biểu thức P= 3 sin x +4 cosx là
Câu 2: Tính giá trị của biểu thức P=(sin²x-1)tan²x+ (cos²x-1)cot²x
Câu 3: Tìm các giá trị của tham số m để phương trình (x-2)(x-2mx+1)=0 có 2 nghiệm phân biệt
Xem chi tiết
Tính giá trị biểu thức a. Cos(x- 45⁰) biết sin x = 4/5 và 180⁰
Cho cosx -3/5 , 𝝅/2 x𝝅. Nêu cách tính sinx, sin (x + 𝝅/3), (x+ 𝝅/4)
Đọc tiếp
Cho cosx = -3/5 , 𝝅/2 <x<𝝅. Nêu cách tính sinx, sin (x + 𝝅/3), (x+ 𝝅/4)
\(\dfrac{\pi}{2}< x< \pi\Rightarrow sinx>0\)
\(\Rightarrow sinx=\sqrt{1-cos^2x}=\sqrt{1-\left(-\dfrac{3}{5}\right)^2}=\dfrac{4}{5}\)
\(sin\left(x+\dfrac{\pi}{3}\right)=sinx.cos\left(\dfrac{\pi}{3}\right)+cosx.sin\left(\dfrac{\pi}{3}\right)=\dfrac{4}{5}.\dfrac{1}{2}+\left(-\dfrac{3}{5}\right).\dfrac{\sqrt{3}}{2}=\dfrac{4-3\sqrt{3}}{10}\)
\(cos\left(x+\dfrac{\pi}{4}\right)=cosx.cos\left(\dfrac{\pi}{4}\right)-sinx.sin\left(\dfrac{\pi}{4}\right)=-\dfrac{3}{5}.\dfrac{\sqrt{2}}{2}-\dfrac{4}{5}.\dfrac{\sqrt{2}}{2}=-\dfrac{7\sqrt{2}}{10}\)
Đúng 0
Bình luận (0)
Biết \(sinx=\dfrac{-2\sqrt{5}}{5},cosx=\dfrac{1}{\sqrt{5}},tanx=-2\). Tính giá trị của biểu thức: M = \(sin\left(\dfrac{\pi}{2}-x\right).cot\left(\pi+x\right)\)
\(sin(\dfrac{\pi}{2}-x)cot(\pi+x)=cosxcotx=\dfrac{cosx}{tanx}\\ =\dfrac{\dfrac{1}{\sqrt5}}{-2}=\dfrac{-\sqrt5}{10}\)
Đúng 0
Bình luận (0)
~Các bạn giúp mk làm bài này nhé! Cảm ơn các bạn nhiều ...~ Bài 1:Tính giá trị biểu thứca) A sin10°+sin20°+sin30°+sin40°-cos50°-cos60°-cos70°-cos80°b) C cos²52° sin45°+sin²52° cos45°c) E sin²5°+sin²15°+sinv25°+sin²35°+sin²45°+sin²55°+sin²65°+sin²75°+sin²85°Bài 2: C/m rằng với góc nhọn α ta luôn cóa) (sinα +cosα)²-(sinα -cosα)² 4sinα cosαb) cosα/1-sinα 1+sinα/cosαc) √̅s̅i̅n̅²̅x̅(̅1̅+̅̅c̅o̅t̅̅x̅)̅̅+̅c̅o̅s̅²̅x̅(̅1̅+̅t̅a̅n̅x̅)̅ sinx+cosxBài 3: Cho α là một góc nhọn a) Biết sinα 3/4. Tính cosα(90°-...
Đọc tiếp
~Các bạn giúp mk làm bài này nhé! Cảm ơn các bạn nhiều ...~
Bài 1:Tính giá trị biểu thức
a) A= sin10°+sin20°+sin30°+sin40°-cos50°-cos60°-cos70°-cos80°
b) C= cos²52° sin45°+sin²52° cos45°
c) E= sin²5°+sin²15°+sinv25°+sin²35°+sin²45°+sin²55°+sin²65°+sin²75°+sin²85°
Bài 2: C/m rằng với góc nhọn α ta luôn có
a) (sinα +cosα)²-(sinα -cosα)² = 4sinα cosα
b) cosα/1-sinα =1+sinα/cosα
c) √̅s̅i̅n̅²̅x̅(̅1̅+̅̅c̅o̅t̅̅x̅)̅̅+̅c̅o̅s̅²̅x̅(̅1̅+̅t̅a̅n̅x̅)̅ =sinx+cosx
Bài 3: Cho α là một góc nhọn
a) Biết sinα =3/4. Tính cosα(90°-α)
b) Biết tanα =2. Tính cotα(90°-α)