a \(\Leftrightarrow3x^2+9x+4x+12=0\Leftrightarrow3x\left(x+3\right)+4\left(x+3\right)=0\Leftrightarrow\left(x+3\right)\left(3x+4\right)=0\) \(\Leftrightarrow\left[{}\begin{matrix}x+3=0\\3x+4=0\end{matrix}\right.\)  \(\Leftrightarrow\left[{}\begin{matrix}x=-3\\x=-\dfrac{4}{3}\end{matrix}\right.\)

\(3x^2+13x+12=0\)

\(\Leftrightarrow3\left(x^2+\dfrac{13}{3}x+4\right)=0\Leftrightarrow x^2+\dfrac{13}{3}x+4=0\)

\(\Leftrightarrow x^2+3x+\dfrac{4}{3}x+4=0\)

\(\Leftrightarrow x\left(x+3\right)+\dfrac{4}{3}\left(x+3\right)=0\)

\(\Leftrightarrow\left(x+\dfrac{4}{3}\right)\left(x+3\right)=0\Leftrightarrow\left[{}\begin{matrix}x=-\dfrac{4}{3}\\x=-3\end{matrix}\right.\)

Ta có:

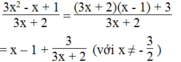
Vì x là số nguyên nên x – 1 là số nguyên.

Để biểu thức đã cho là số nguyên thì 3 ⋮ (3x + 2) và x ≠ -2/3

Suy ra: 3x + 2 ∈ Ư(3) = {-3; -1; 1; 3}

Ta có: 3x + 2 = -3 ⇒ x = -5/3 ∉ Z (loại)

3x + 2 = -1 ⇒ x = - 1

3x + 2 = 1 ⇒ x = -1/3 ∉ Z (loại)

3x + 2 = 3 ⇒ x = 1/3 ∉ Z (loại)

x = -1 khác -3/2

Vậy với x = - 1 thì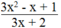có giá trị nguyên.

\(a,\Rightarrow\left(x-2000\right)\left(5x-1\right)=0\Rightarrow\left[{}\begin{matrix}x=2000\\x=\dfrac{1}{5}\end{matrix}\right.\\ b,\Rightarrow x\left(x^2-13\right)=0\Rightarrow\left[{}\begin{matrix}x=0\\x=\sqrt{13}\\x=-\sqrt{13}\end{matrix}\right.\\ c,\Rightarrow3x\left(x-2\right)=0\Rightarrow\left[{}\begin{matrix}x=0\\x=2\end{matrix}\right.\\ d,\Rightarrow\left(x-5\right)\left(x+3\right)=0\Rightarrow\left[{}\begin{matrix}x=5\\x=-3\end{matrix}\right.\\ e,\Rightarrow\left(3x-2\right)\left(3x+2\right)=0\Rightarrow\left[{}\begin{matrix}x=\dfrac{2}{3}\\x=-\dfrac{2}{3}\end{matrix}\right.\)

2: \(\Leftrightarrow\left(x^2+x\right)^2-5\left(x^2+x\right)-6=0\)

\(\Leftrightarrow x^2+x-6=0\)

=>(x+3)(x-2)=0

=>x=-3 hoặc x=2

5: \(\Leftrightarrow\left(x+2\right)\left(x-1\right)\left(x+1\right)=0\)

hay \(x\in\left\{-2;1;-1\right\}\)

a)  x + 30 % x = − 1 , 3

x 1 + 3 10 = − 13 10 13 10 x = − 13 10 x = − 1

b)  1 3 x + 2 5 x − 1 = 0

1 3 x + 2 5 x − 2 5 = 0 11 15 x = 2 5 x = 2 5 : 11 15 x = 6 11

c)  3 x − 1 2 − 5 x + 3 5 = − x + 1 5

3 x − 3 2 − 5 x − 3 = − x + 1 5 x = − 3 2 − 3 − 1 5 x = − 47 10

Ta có

3 x 2   +   13 x   +   10   =   0     ⇔   3 x 2   +   3 x   +   10 x   +   10   =   0

ó 3x(x + 1) + 10(x + 1) = 0

ó (x + 1)(3x + 10) = 0

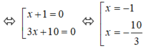
=> 2 x 1 x 2 =   2 . ( - 1 ) . - 10 3 = 20 3

Đáp án cần chọn là: B

Bài 1.

\(a,x^2=5\Leftrightarrow x=\pm\sqrt{5}\)

Vậy \(S=\left\{\pm\sqrt{5}\right\}\)

\(b,3x^2-12=0\Leftrightarrow3x^2=12\Leftrightarrow x^2=4\Leftrightarrow x=\pm2\)

Vậy \(S=\left\{\pm2\right\}\)

\(c,4x^2-3=-9\)

\(\Leftrightarrow4x^2=-6\)

\(\Leftrightarrow x^2=-\dfrac{3}{2}\) (loại)

Vậy pt vô nghiệm.

\(d,5x^2-3=-3\)

\(\Leftrightarrow5x^2=0\)

\(\Leftrightarrow x=0\)

Vậy \(S=\left\{0\right\}\)

a)

`x^2 =5`
`=>\(\left[{}\begin{matrix}x=\sqrt{5}\\x=-\sqrt{5}\end{matrix}\right.\)

b)

`3x^2 -12=0`

`<=>3x^2 =12`

`<=>x^2 =4`

\(< =>\left[{}\begin{matrix}x=2\\x=-2\end{matrix}\right.\)

c)

`4x^2 -3=-9`

`<=>4x^2 =-6`

`<=>x^2 =-3/2` (vô lí vì `x>=0AA x` )

d)

`5x^2 -3=3`

`<=>5x^2 =0`

`<=>x^2 =0`

`<=>x=0`