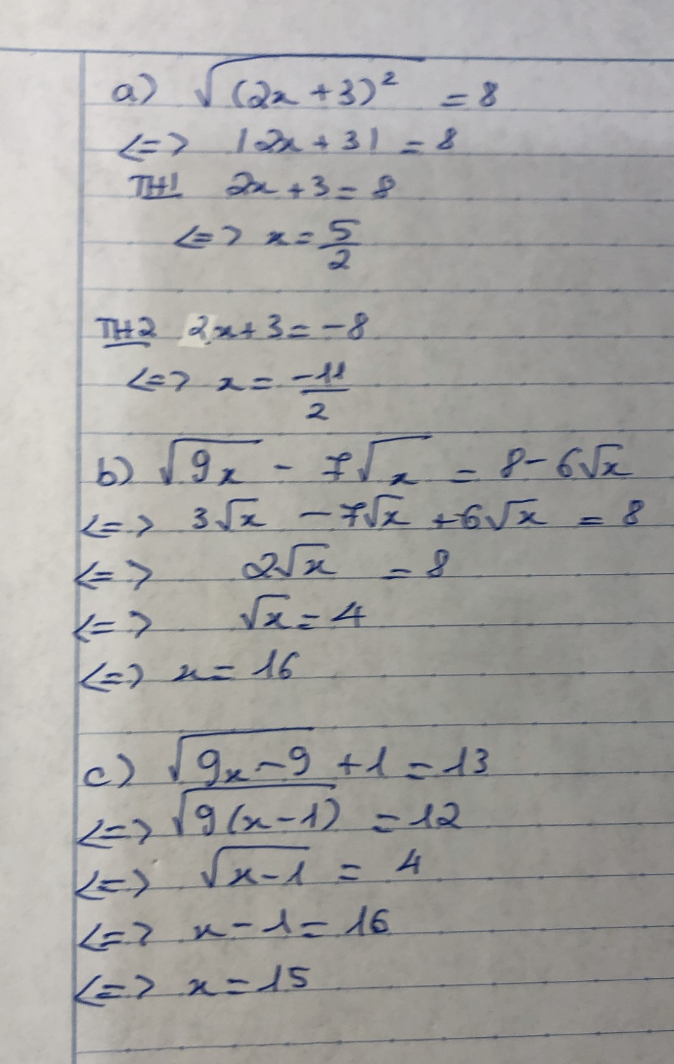x=8 nên x+1=9

\(F=x^{13}-9x^{12}+9x^{11}-9x^{10}+...-9x^2+9x-2\)

\(=x^{13}-x^{12}\left(x+1\right)+x^{11}\left(x+1\right)-x^{10}\left(x+1\right)+...-x^2\left(x+1\right)+x\left(x+1\right)-2\)

\(=x^{13}-x^{13}-x^{12}+x^{12}+...-x^3-x^2+x^2+x-2\)

=x-2

=8-2

=6

Để F(x) có nghiệm <=> x^10 - 9x^9 + ... + 9x^2 - 9x +8 = 0

<=> (x^10 - x^9) - (8x^9 - 8x^8) + (x^8 - x^7) - ... + (x^2 - x) - (8x - 8) = 0

<=> x^9(x - 1) - 8x^8(x - 1) + ... + x(x - 1) - 8(x - 1) = 0

<=> (x^9 - 8x^8 + ... + x - 8)(x - 1) = 0

<=> (  (x^9 - 8x^8) + (x^7 - 8x^6) + ... + (x - 8)  )(x - 1) = 0

<=> (x^8 + x^6 + ... + 1)(x - 8)(x - 1) = 0

Có nghiệm là 8 và 1

Với x = 8

=> x + 1 = 9 (1)

Thay (1) vào biểu thức ta được

\(x^{10}-9x^9+9x^8-9x^7+...+9x^2-9x-2\)

\(=x^{10}-\left(x+1\right)x^9+\left(x+1\right)x^8-\left(x+1\right)x^7+...+\left(x+1\right)x^2-\left(x+1\right)x-2\)

\(=x^{10}-x^{10}-x^9+x^9+x^8-x^8-x^7+...+x^3+x^2-x^2-x-2\)

\(=-x-2\)

\(=-8-2=-10\)

bn ơi kể ra có kết quả rồi 

\(\Leftrightarrow3\cdot9^2-7\left(9x-5\right)=26\)

=>7(9x-5)=217

=>9x-5=31

hay x=4

a) ⇔ |2x+3| = 8
⇒ \(\left[{}\begin{matrix}2x+3=8\\2x+3=-8\end{matrix}\right.\) ⇔ \(\left[{}\begin{matrix}2x=5\\2x=-11\end{matrix}\right.\) ⇔ \(\left[{}\begin{matrix}x=\dfrac{5}{2}\\x=-\dfrac{11}{2}\end{matrix}\right.\)

Vậy...

b) ĐKXĐ: \(x\ge0\)

\(\Leftrightarrow3\sqrt{x}-7\sqrt{x}+6\sqrt{x}=8\)

\(\Leftrightarrow2\sqrt{x}=8\)

\(\Leftrightarrow\sqrt{x}=4\)

\(\Leftrightarrow x=16\) (Vì \(x\ge0\) )

Vậy x = 16

c) ĐKXĐ: \(x\ge1\)

\(\Leftrightarrow\sqrt{9\left(x-1\right)}=12\)

\(\Leftrightarrow3\sqrt{x-1}=12\)

\(\Leftrightarrow\sqrt{x-1}=4\)

\(\Leftrightarrow x-1=16\)

\(\Leftrightarrow x=17\)(TM)

Vậy x = 17