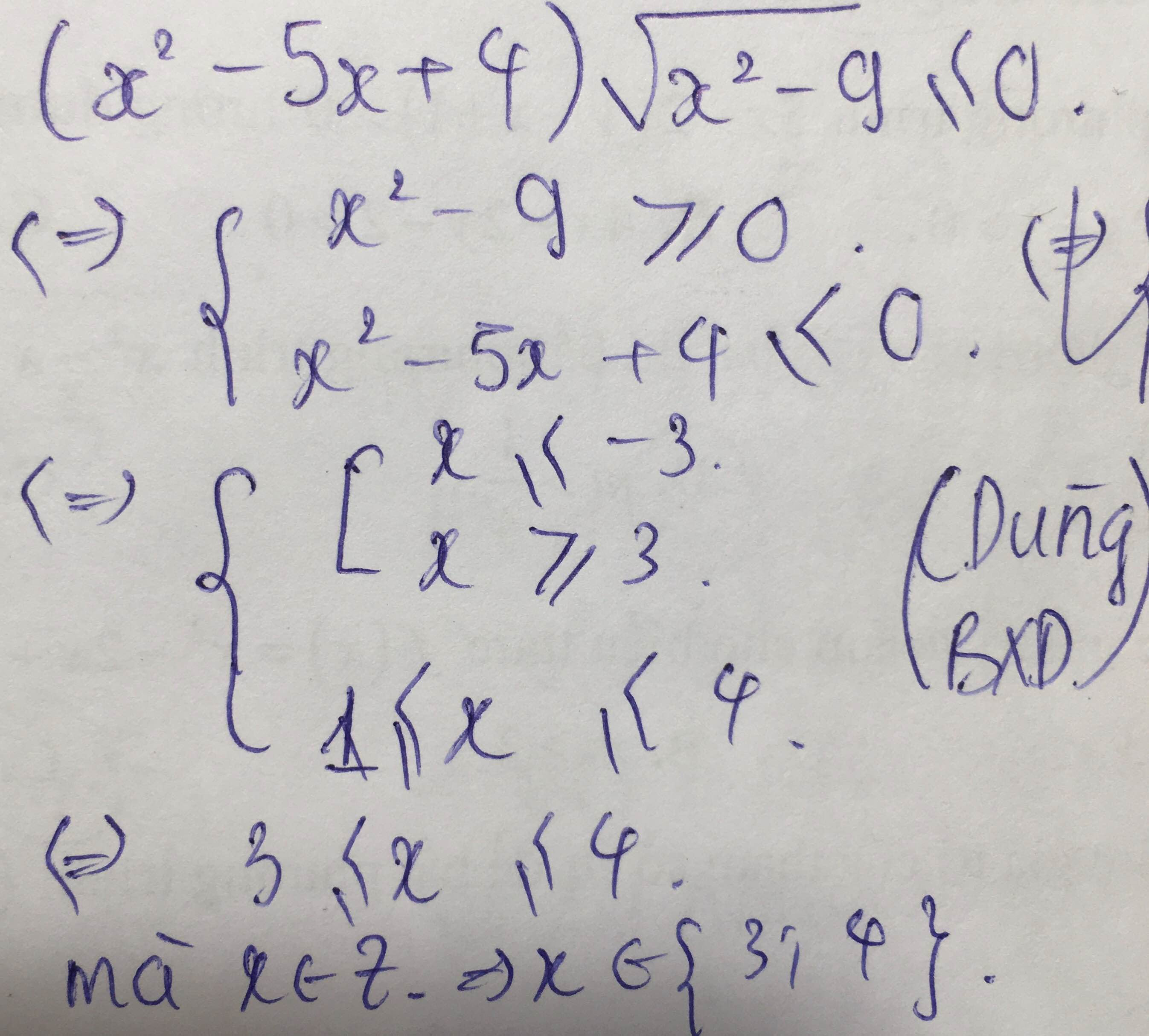Tập nghiệm của bất phương trình\(\left(x+2\right)\sqrt{x^2-9}\le0\)

Những câu hỏi liên quan
số nghiệm nguyên của bất phương trình \(\left(x^2-5x+4\right)\sqrt{x^2-9}\le0\) ?
Cho bất phương trình \(x^3+\left(3x^2-4x-4\right)\sqrt{x+1}\le0\) có tập nghiệm \(\left[a;b\right]\) . Tính a + b☘
Tập nghiệm của bất phương trình \(\dfrac{2x\left(x^2-1\right)}{3-2x-x^2}\le0\) là
Tập nghiệm của BPT là: \(\left[{}\begin{matrix}-3< x\le-1\\0\le x< 1\\x>1\end{matrix}\right.\)
Đúng 1
Bình luận (0)
Hệ bất phương trình \(\left\{{}\begin{matrix}x^2-5x+4\le0\\x^2-\left(m^2+3\right)x+2\left(m^2+1\right)\le0\end{matrix}\right.\) có tập nghiệm biểu diễn trên trục số có độ dài bằng 1, với giá trị của m bằng ?
gọi S là tập nghiệm của bất phương trình \(x^2-\left(2m-6\right)x+m^2-6m+5\le0\). tìm tất cả các giá trị của m sao cho (3;5) \(\subset\) S.
=>x^2-[(m-1)+(m-5)]x+m^2-6m+5<=0
=>x(x-m+1)-(m-5)(x-m+1)<=0
=>(x-m+1)(x-m+5)<=0
=>m-5<=x<=m-1
=>S=[m-5;m-1]
(3;5) là tập con của S
=>m-5>=3 và m-1<=5
=>m>=8 và m<=6
=>Loại
Đúng 0
Bình luận (0)
Phương trình sqrt{2-fleft(xright)}fleft(xright) có tập nghiệm A {1;2;3}. Phương trình sqrt{2.gleft(xright)-1}+sqrt[3]{3.gleft(xright)-2}2.gleft(xright) có tập nghiệm là B {0;3;4;5} . Hỏi tập nghiệm của phương trình sqrt{fleft(xright)-1}+sqrt{gleft(xright)-1}+fleft(xright).gleft(xright)+1fleft(xright)+gleft(xright)
có bao nhiêu phần tử?
A.1
B.4
C.6
D.7
Đọc tiếp
Phương trình \(\sqrt{2-f\left(x\right)}=f\left(x\right)\) có tập nghiệm A = {1;2;3}. Phương trình \(\sqrt{2.g\left(x\right)-1}+\sqrt[3]{3.g\left(x\right)-2}=2.g\left(x\right)\) có tập nghiệm là B = {0;3;4;5} . Hỏi tập nghiệm của phương trình \(\sqrt{f\left(x\right)-1}+\sqrt{g\left(x\right)-1}+f\left(x\right).g\left(x\right)+1=f\left(x\right)+g\left(x\right)\)
có bao nhiêu phần tử?
A.1
B.4 C.6 D.7
\(\sqrt{2-f\left(x\right)}=f\left(x\right)\Leftrightarrow\left\{{}\begin{matrix}f\left(x\right)\ge0\\f^2\left(x\right)+f\left(x\right)-2=0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}f\left(x\right)=1\\f\left(x\right)=-2< 0\left(loại\right)\end{matrix}\right.\)
\(\Rightarrow f\left(1\right)=f\left(2\right)=f\left(3\right)=1\)
\(\sqrt{2g\left(x\right)-1}+\sqrt[3]{3g\left(x\right)-2}=2.g\left(x\right)\)
\(VT=1.\sqrt{2g\left(x\right)-1}+1.1\sqrt[3]{3g\left(x\right)-2}\)
\(VT\le\dfrac{1}{2}\left(1+2g\left(x\right)-1\right)+\dfrac{1}{3}\left(1+1+3g\left(x\right)-2\right)\)
\(\Leftrightarrow VT\le2g\left(x\right)\)
Dấu "=" xảy ra khi và chỉ khi \(g\left(x\right)=1\)
\(\Rightarrow g\left(0\right)=g\left(3\right)=g\left(4\right)=g\left(5\right)=1\)
Để các căn thức xác định \(\Rightarrow\left\{{}\begin{matrix}f\left(x\right)-1\ge0\\g\left(x\right)-1\ge0\end{matrix}\right.\)
Ta có:
\(\sqrt{f\left(x\right)-1}+\sqrt{g\left(x\right)-1}+f\left(x\right).g\left(x\right)-f\left(x\right)-g\left(x\right)+1=0\)
\(\Leftrightarrow\sqrt{f\left(x\right)-1}+\sqrt{g\left(x\right)-1}+\left[f\left(x\right)-1\right]\left[g\left(x\right)-1\right]=0\)
\(\Leftrightarrow\left\{{}\begin{matrix}f\left(x\right)=1\\g\left(x\right)=1\end{matrix}\right.\) \(\Leftrightarrow x=3\)
Vậy tập nghiệm của pt đã cho có đúng 1 phần tử
Đúng 0
Bình luận (0)
Cho hệ bất phương trình : \(\hept{\begin{cases}x^2-6x+5\le0\\x^2-2\left(a+1\right)x+a^2+1\le0\end{cases}}\) Để hệ bất phương trình có nghiệm , giá trị của a là :
tập nghiệm của hệ bất phương trình\(\left\{{}\begin{matrix}x^2-5x+6\le0\\x^2-4\le0\end{matrix}\right.\)
\(x^2-5x+6\le0\Leftrightarrow\left(x-2\right)\left(x-3\right)\le0\Rightarrow2\le x\le3\) (1)
\(x^2-4\le0\Leftrightarrow\left(x-2\right)\left(x+2\right)\le0\Rightarrow-2\le x\le2\) (2)
(1);(2) \(\Rightarrow x=2\)
Tìm tập nghiệm của bất phương trình:\(2\left(x-4\right)\sqrt{2x+1}\ge x\sqrt{x^2+1}+x^3+x^2-3x-8\)