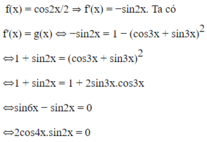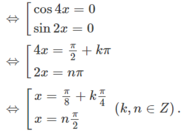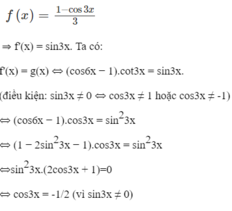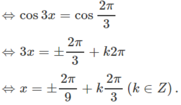Giải phương trình f′(x) = g(x), biết rằng f ( x ) = 1 2 sin 2 x ...

Hãy tham gia nhóm Học sinh Hoc24OLM

Bạn chưa đăng nhập. Vui lòng đăng nhập để hỏi bài

Chọn lớp:

Chọn môn:

Gửi câu hỏi ẩn danh

Pham Trong Bach 10 tháng 6 2019 lúc 6:44

Giải phương trình f′(x) g(x), biết rằng f ( x ) 1 2 sin 2 x + 5 cos x , g ( x ) 3 sin 2 x + 3 1 +...

Đọc tiếp

Giải phương trình f′(x) = g(x), biết rằng f ( x ) = 1 2 sin 2 x + 5 cos x , g ( x ) = 3 sin 2 x + 3 1 + tan 2 x

Lớp 11 Toán

Những câu hỏi liên quan

Bài 7

SGK trang 169

4 tháng 4 2017 lúc 14:06

Giải phương trình $f'\left(x\right)=0$ biết rằng :

a) $f\left(x\right)=3\cos x+4\sin x+5x$

b) $f\left(x\right)=1-\sin\left(\pi+x\right)+2\cos\left(\dfrac{2\pi+x}{2}\right)$

Xem chi tiết

Lớp 11 Toán Bài 3: Đạo hàm của hàm số lượng giác

Đặng Phương Nam

4 tháng 4 2017 lúc 17:13

a) f'(x) = - 3sinx + 4cosx + 5. Do đó

f'(x) = 0 <=> - 3sinx + 4cosx + 5 = 0 <=> 3sinx - 4cosx = 5

<=> $\frac{3}{5}$ sinx - $\frac{4}{5}$ cosx = 1. (1)

Đặt cos φ = $\frac{3}{5}$ , (φ ∈ $\left ( 0;\frac{\pi }{2} \right )$ ) => sin φ = $\frac{4}{5}$ , ta có:

(1) <=> sinx.cos φ - cosx.sin φ = 1 <=> sin(x - φ) = 1

<=> x - φ = $\frac{\pi }{2}$ + k2π <=> x = φ + $\frac{\pi }{2}$ + k2π, k ∈ Z.

b) f'(x) = - cos(π + x) - sin $\left (\pi + \frac{x}{2} \right )$ = cosx + sin $\frac{x }{2}$ .

f'(x) = 0 <=> cosx + sin $\frac{x }{2}$ = 0 <=> sin $\frac{x }{2}$ = - cosx <=> sin $\frac{x }{2}$ = sin $\left (\pi- \frac{x}{2} \right )$

<=> $\frac{x }{2}$ = $\pi -\frac{x}{2}$ + k2π hoặc $\frac{x }{2}$ = π - x + $\frac{\pi }{2}$ + k2π

<=> x = π - k4π hoặc x = π + k $\frac{4\pi }{3}$ , (k ∈ Z).

Đúng 0

Bình luận (0)

Pham Trong Bach

27 tháng 4 2017 lúc 14:53

Giải phương trình f′(x) g(x), biết rằng f ( x ) 1 2 cos 2 x , g ( x ) 1 - cos 3 x + sin 3...

Đọc tiếp

Giải phương trình f′(x) = g(x), biết rằng f ( x ) = 1 2 cos 2 x , g ( x ) = 1 - cos 3 x + sin 3 x 2

Xem chi tiết

Lớp 11 Toán

Cao Minh Tâm

27 tháng 4 2017 lúc 14:54

Giải sách bài tập Toán 11 | Giải sbt Toán 11

Đúng 0

Bình luận (0)

Pham Trong Bach

15 tháng 2 2019 lúc 3:52

Giải phương trình f′(x) g(x), biết rằng f ( x ) 1 - cos 3 x 3 ; g ( x ) cos 6 x -...

Đọc tiếp

Giải phương trình f′(x) = g(x), biết rằng f ( x ) = 1 - cos 3 x 3 ; g ( x ) = cos 6 x - 1 c o t 3 x

Xem chi tiết

Lớp 11 Toán

Cao Minh Tâm

15 tháng 2 2019 lúc 3:53

Giải sách bài tập Toán 11 | Giải sbt Toán 11

Đúng 0

Bình luận (0)

Nguyễn Phương Thùy

11 tháng 9 2021 lúc 7:49

cho hàm số $f\left(x\right)=x^3-3x^2+2$

a, giải bất phương trình $f'\left(x\right)\le0$

b, giải phương trình $f'=\left(x^2-3x+2\right)=0$

c, đặt $g\left(x\right)=f\left(1-2x\right)+x^2-x+2022$ giải bất phương trình$g'\left(x\right)\ge0$

Xem chi tiết

Lớp 11 Toán Ôn tập cuối năm môn Đại số 11

Nguyễn Hoàng Minh

11 tháng 9 2021 lúc 8:38

$a,f'\left(x\right)=3x^2-6x\\ f'\left(x\right)\le0\Leftrightarrow3x^2-6x\le0\\ \Leftrightarrow3x\left(x-2\right)\le0\Leftrightarrow0\le x\le2$

Đúng 2

Bình luận (0)

Akai Haruma Giáo viên

11 tháng 9 2021 lúc 8:44

Lời giải:

a. $f'(x)\leq 0$

$\Leftrightarrow 3x^2-6x\leq 0$

$\Leftrightarrow x(x-2)\leq 0$

$\Leftrightarrow 0\leq x\leq 2$

$f'(x)=x^2-3x+2=0$

$\Leftrightarrow 3x^2-6x=x^2-3x+2=0$

$\Leftrightarrow 3x(x-2)=(x-1)(x-2)=0$

$\Leftrightarrow x-2=0$

$\Leftrightarrow x=2$

$g(x)=f(1-2x)+x^2-x+2022$

$g'(x)=(1-2x)'f(1-2x)'_{1-2x}+2x-1$

$=-2[3(1-2x)^2-6(1-2x)]+2x-1$
$=-24x^2+2x+5$

$g'(x)\geq 0$

$\Leftrightarrow -24x^2+2x+5\geq 0$

$\Leftrightarrow (5-12x)(2x-1)\geq 0$

$\Leftrightarrow \frac{-5}{12}\leq x\leq \frac{1}{2}$

Đúng 1

Bình luận (0)

Bài 3.19

Sách bài tập trang 208

11 tháng 4 2017 lúc 10:53

Giải phương trình $f'\left(x\right)=g\left(x\right)$

a) Với $f\left(x\right)=1+\sin^43x$ và $g\left(x\right)=\sin6x$

b) Với $f\left(x\right)=4x\cos^2\left(\dfrac{x}{2}\right)$ và $g\left(x\right)=8\cos\dfrac{x}{2}-3-2x\sin x$

Xem chi tiết

Lớp 11 Toán Bài 3: Đạo hàm của hàm số lượng giác

Bài 2

Sách bài tập trang 214

11 tháng 4 2017 lúc 11:37

Giải phương trình $f'\left(x\right)=g\left(x\right)$ biết :

a) $f\left(x\right)=\dfrac{1-\cos3x}{3};g\left(x\right)=\left(\cos6x-1\right)\cot3x$

b) $f\left(x\right)=\dfrac{1}{2}\cos2x;g\left(x\right)=1-\left(\cos3x+\sin3x\right)^2$

c) $f\left(x\right)=\dfrac{1}{2}\sin2x+5\cos x;g\left(x\right)=3\sin^2x+\dfrac{3}{1+\tan^2x}$

Xem chi tiết

Lớp 11 Toán Bài 6: Ôn tập chương Đạo hàm

Bài 3.18

Sách bài tập trang 207

11 tháng 4 2017 lúc 10:51

Giải các phương trình :

a) $f'\left(x\right)=0$ với $f\left(x\right)=1-\sin\left(\pi+x\right)+2\cos\dfrac{3\pi+x}{2}$

b) $g'\left(x\right)=0$ với $g\left(x\right)=\sin3x-\sqrt{3}\cos3x+3\left(\cos x-\sqrt{3}\sin x\right)$

Xem chi tiết

Lớp 11 Toán Bài 3: Đạo hàm của hàm số lượng giác

Bài 12

SGK trang 47

31 tháng 3 2017 lúc 11:05

Cho hàm số: $f(x)=\dfrac{1}{3}x^3−\dfrac{1}{2}x^2−4x+6$

a) Giải phương trình $f’(\sin x) = 0$

b) Giải phương trình $f’’(\cos x) = 0$

c) Viết phương trình tiếp tuyến của đồ thị hàm số đã cho tại điểm có hoành độ là nghiệm của phương trình $f’’(x) = 0$

Xem chi tiết

Lớp 12 Toán Bài 5d: Bài tập ôn luyện

Bùi Thị Vân

29 tháng 5 2017 lúc 16:02

Đúng 0

Bình luận (0)

Pham Trong Bach

12 tháng 6 2017 lúc 8:15

Cho f ( x ) 2 x 3 + x − 2 v à g ( x ) 3 x 2 + x + 2 . Giải bất phương trìn...

Đọc tiếp

Cho f ( x ) = 2 x 3 + x − 2 v à g ( x ) = 3 x 2 + x + 2 .

Giải bất phương trình f′(x) > g′(x).

Xem chi tiết

Lớp 11 Toán

Cao Minh Tâm

12 tháng 6 2017 lúc 8:15

(−∞; 0) ∪ (1; +∞).

Đúng 0

Bình luận (0)

Câu hỏi

Khoá học trên OLM (olm.vn)