Thực hiện các phép tính sau: x 3 - 2 x 2 y + x y 2 2 x + y . 2 x y + y 2 x 3 - x y 2

Những câu hỏi liên quan
V . CÁC PHÉP TOÁN VỀ PHÂN THỨC :Bài 1 : Thực hiện các phép tính sau : b) x+3/x-2+4+x/2-x Bài 2 : Thức hiện các phép tính sau : a) x+1/2x+6+2x+3/x2+3x d) 3/2x2y +5/xy2 + x/y3 e) x/x-2y +x/x+2y + 4xy/4y2-x2 g) x+3/x+1 +2x-1/x-1 +x+5/X2-1 ;
Đọc tiếp
V . CÁC PHÉP TOÁN VỀ PHÂN THỨC :
Bài 1 : Thực hiện các phép tính sau :
b) x+3/x-2+4+x/2-x
Bài 2 : Thức hiện các phép tính sau :
a) x+1/2x+6+2x+3/x2+3x
d) 3/2x2y +5/xy2 + x/y3
e) x/x-2y +x/x+2y + 4xy/4y2-x2
g) x+3/x+1 +2x-1/x-1 +x+5/X2-1 ;
Bài 1:
b: \(=\dfrac{x+3-4-x}{x-2}=\dfrac{-1}{x-2}\)
Bài 2:
a: \(=\dfrac{x+1}{2\left(x+3\right)}+\dfrac{2x+3}{x\left(x+3\right)}\)
\(=\dfrac{x^2+x+4x+6}{2x\left(x+3\right)}=\dfrac{x^2+5x+6}{2x\left(x+3\right)}=\dfrac{x+2}{2x}\)
d: \(=\dfrac{3}{2x^2y}+\dfrac{5}{xy^2}+\dfrac{x}{y^3}\)
\(=\dfrac{3y^2+10xy+2x^3}{2x^2y^3}\)
e: \(=\dfrac{x^2+2xy+x^2-2xy-4xy}{\left(x+2y\right)\left(x-2y\right)}=\dfrac{2x^2-4xy}{\left(x+2y\right)\cdot\left(x-2y\right)}=\dfrac{2x}{x+2y}\)
Đúng 0
Bình luận (0)
Thực hiện các phép tính sau: b)(xy/2x- y)-( 2x² / y-2x) c) (3x² - x/ x-1) +( x + 2/1-x) + (3 -2x²/ x-1 )
b: \(\dfrac{xy}{2x-y}-\dfrac{2x^2}{y-2x}=\dfrac{xy}{2x-y}+\dfrac{2x^2}{2x-y}=\dfrac{xy+2x^2}{2x-y}\)
b: \(\dfrac{3x^2-x}{x-1}+\dfrac{x+2}{1-x}+\dfrac{3-2x^2}{x-1}\)
\(=\dfrac{3x^2-x-x-2+3-2x^2}{x-1}\)
\(=\dfrac{x^2-2x+1}{x-1}=x-1\)
Đúng 1
Bình luận (0)
thực hiện phép tính sau\(\dfrac{1}{x-y}-\dfrac{3xy}{x^3-y^3}+\dfrac{x-y}{x^2+xy+y^2}\)
MTC = (x - y)(x2 + xy + y2)
\(\dfrac{1}{x-y}-\dfrac{3xy}{x^3-y^3}+\dfrac{x-y}{x^2+xy+y^2}\)
\(=\dfrac{x^2+xy+y^2}{\left(x-y\right)\left(x^2+xy+y^2\right)}-\dfrac{3xy}{\left(x-y\right)\left(x^2+xy+y^2\right)}+\dfrac{\left(x-y\right)^2}{\left(x-y\right)\left(x^2+xy+y^2\right)}\)
\(=\dfrac{x^2+xy+y^2-3xy+\left(x-y\right)^2}{\left(x-y\right)\left(x^2+xy+y^2\right)}\)
\(=\dfrac{x^2+xy+y^2-3xy+x^2-2xy+y^2}{\left(x-y\right)\left(x^2+xy+y^2\right)}\)
\(=\dfrac{2x^2-4xy+2y^2}{\left(x-y\right)\left(x^2+xy+y^2\right)}\)
\(=\dfrac{2\left(x^2-2xy+y^2\right)}{\left(x-y\right)\left(x^2+xy+y^2\right)}\)
\(=\dfrac{2\left(x-y\right)^2}{\left(x-y\right)\left(x^2+xy+y^2\right)}\)
\(=\dfrac{2\left(x-y\right)}{x^2+xy+y^2}\)
Đúng 1
Bình luận (0)
1/x-y-3xy/x^3-y^3+x-y/x^2+xy+y^2
=1/x-y+-3xy/(x-y)(x^2+xy+y^2)+x-y/x^2+xy+y^2
=x^2+xy+y^2/(x-y)(x^2+xy+y^2)+-3xy/(x-y)(x^2+xy+y^2)+x^2-2xy+y^2/(x-y)(x^2+xy+y^2)
=x^2+xy+y^2-3xy+x^2-2xy-y^2/(x-y)(x^2+xy+y^2)
=2x^2-5xy/(x-y)(x^2+xy+y^2)
Đúng 0
Bình luận (0)
MTC = (x - y)(x2 + xy + y2)
\(\dfrac{1}{x-y}-\dfrac{3xy}{x^3-y^3}+\dfrac{x-y}{x^2+xy+y^2}\)
\(=\dfrac{x^2+xy+y^2}{\left(x-y\right)\left(x^2+xy+y^2\right)}-\dfrac{3xy}{\left(x-y\right)\left(x^2+xy+y^2\right)}+\dfrac{\left(x-y\right)^2}{\left(x-y\right)\left(x^2+xy+y^2\right)}\)
\(=\dfrac{x^2+xy+y^2-3xy+\left(x-y\right)^2}{\left(x-y\right)\left(x^2+xy+y^2\right)}\)
\(=\dfrac{x^2+xy+y^2-3xy+x^2-2xy+y^2}{\left(x-y\right)\left(x^2+xy+y^2\right)}\)
\(=\dfrac{2x^2-4xy+2y^2}{\left(x-y\right)\left(x^2+xy+y^2\right)}\)
\(=\dfrac{2\left(x^2-2xy+y^2\right)}{\left(x-y\right)\left(x^2+xy+y^2\right)}\)
\(=\dfrac{2\left(x-y\right)^2}{\left(x-y\right)\left(x^2+xy+y^2\right)}\)
\(=\dfrac{2\left(x-y\right)}{x^2+xy+y^2}\)
Đúng 0
Bình luận (0)
Thực hiện các phép tính sau: a) (x²/x²+3x)+(3/x+3)+(3/x) b) (2/x+2)+(-4/2-x)+(5x+2/4-x²) c) (1/x-y)+(3xy/y³-x³)+(x-y/x²+xy+y²) d) (3-3x/2x)+(3x-1/2x-1)+(11x-5/2x-4x²)
Thực hiện phép tính :bài 1 :thực hiện phép tính :
Bài 3: phân tích thành nhân tử:3/6x(x-y)-9y^2+9xyBài 4:Tìm x, biết:
1/ (x-1)(x^2+x+1)-x^3-6x=11
2/ 16x^2-(3x-4)^2=0
3/ x^3-x^2+3-3x=0
4/ x-1/x+2=x+2/x+1
5/1/x+2/x+1=0
6/ 9-x^2/x : (x-3)=1
Xem chi tiết
Bài 3: phân tích thành nhân tử:3/6x(x-y)-9y^2+9xyBài 4:Tìm x, biết:
1/ (x-1)(x^2+x+1)-x^3-6x=11
2/ 16x^2-(3x-4)^2=0
3/ x^3-x^2+3-3x=0
4/ x-1/x+2=x+2/x+1
5/1/x+2/x+1=0
6/ 9-x^2/x : (x-3)=1
Bài 3:
3: \(6x\left(x-y\right)-9y^2+9xy\)
\(=6x\left(x-y\right)+9xy-9y^2\)
\(=6x\left(x-y\right)+9y\left(x-y\right)\)
\(=\left(x-y\right)\left(6x+9y\right)\)
\(=3\left(2x+3y\right)\left(x-y\right)\)
Bài 4:
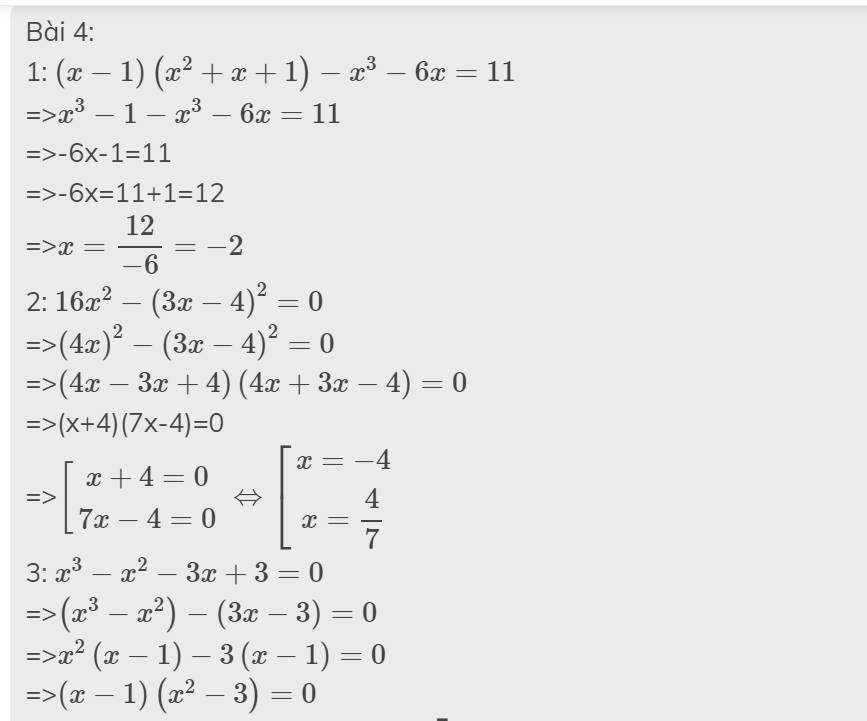

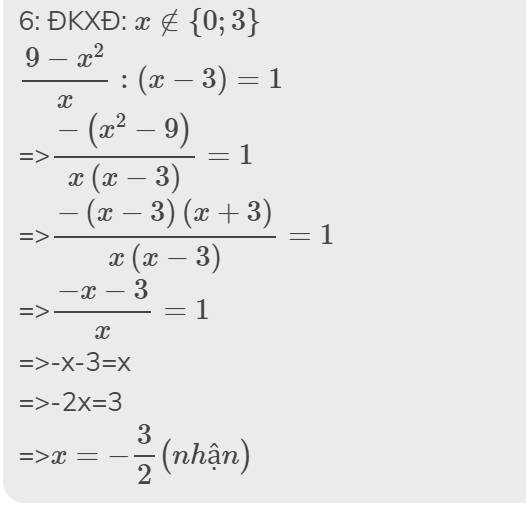
Đúng 1
Bình luận (0)
Thực hiện phép tính sau:
\(\dfrac{3}{2x^2+y}+\dfrac{5}{xy^2}+\dfrac{x}{y^3}\)
\(\dfrac{3}{2x^2+y}+\dfrac{5}{xy^2+}+\dfrac{x}{y^3}\)
=\(\dfrac{3xy^5}{xy^2.y^3\left(2x^2+y\right)+}+\dfrac{10y^3x^2+5y^4}{xy^2.y^3\left(2x^2+y\right)}+\dfrac{2x^4y^2+x^2y^3}{xy^2.y^3\left(2x^2+y\right)}\)
=\(\dfrac{3xy^5+10y^3x^2+5y^4+2x^4y^2+x^2y^3}{xy^5\left(2x^2+y\right)}\)
=\(\dfrac{3xy^5+11y^3x^2+5y^4+2x^4y^2}{xy^5\left(2x^2+y\right)}\)
ủa đáp án cứ sao sao:<
Đúng 0
Bình luận (0)
Thực hiện các phép tính sau:
a) \(18{x^4}{y^3}:12{\left( { - x} \right)^3}y\)
b) \({x^2}{y^2} - 2x{y^3}:\left( {\dfrac{1}{2}x{y^2}} \right)\)
a) \(18x^4y^3:12\left(-x\right)^3y\)
\(=\left(18:-12\right)\left(x^4:x^3\right)\left(y^3:y\right)\)
\(=-\dfrac{3}{2}xy^2\)
b) \(x^2y^2-2xy^3:\dfrac{1}{2}xy^2\)
\(=\dfrac{xy^2\left(x-2y\right)}{\dfrac{1}{2}xy^2}\)
\(=\dfrac{x-2y}{\dfrac{1}{2}}\)
\(=2x-4y\)
Đúng 1
Bình luận (0)
1) Thực hiện các phép tính sau ( giả thiết các phân thức đã cho có nghĩa).a)dfrac{x^3}{x-1}-dfrac{x^2}{x+1}-dfrac{1}{x-1}+dfrac{1}{x+1}b)dfrac{x+y}{2.left(x-yright)}-dfrac{x-y}{2.left(x+yright)}+dfrac{2y^2}{x^2-y^2}c)dfrac{x+5}{2x-4}.dfrac{4-2x}{x+2}d) dfrac{8}{x^2+2x-3}+dfrac{2}{x+3}+dfrac{1}{x-1}Mình đang cần gấp ah
Đọc tiếp
1) Thực hiện các phép tính sau ( giả thiết các phân thức đã cho có nghĩa).
a)\(\dfrac{x^3}{x-1}\)-\(\dfrac{x^2}{x+1}\)-\(\dfrac{1}{x-1}\)+\(\dfrac{1}{x+1}\)
b)\(\dfrac{x+y}{2.\left(x-y\right)}\)-\(\dfrac{x-y}{2.\left(x+y\right)}\)+\(\dfrac{2y^2}{x^2-y^2}\)
c)\(\dfrac{x+5}{2x-4}\).\(\dfrac{4-2x}{x+2}\)
d) \(\dfrac{8}{x^2+2x-3}\)+\(\dfrac{2}{x+3}\)+\(\dfrac{1}{x-1}\)
Mình đang cần gấp ah
a.
\(\dfrac{x^3}{x-1}-\dfrac{x^2}{x+1}-\dfrac{1}{x-1}+\dfrac{1}{x+1}=\dfrac{x^3-1}{x-1}-\dfrac{x^2-1}{x+1}\)
\(=\dfrac{\left(x-1\right)\left(x^2+x+1\right)}{x-1}-\dfrac{\left(x-1\right)\left(x+1\right)}{x+1}\)
\(=x^2+x+1-\left(x-1\right)=x^2+2\)
b.
\(\dfrac{x+y}{2\left(x-y\right)}-\dfrac{x-y}{2\left(x+y\right)}+\dfrac{2y^2}{x^2-y^2}\)
\(=\dfrac{\left(x+y\right)^2}{2\left(x-y\right)\left(x+y\right)}-\dfrac{\left(x-y\right)^2}{2\left(x-y\right)\left(x+y\right)}+\dfrac{4y^2}{2\left(x-y\right)\left(x+y\right)}\)
\(=\dfrac{\left(x+y\right)^2-\left(x-y\right)^2+4y^2}{2\left(x-y\right)\left(x+y\right)}\)
\(=\dfrac{4xy+4y^2}{2\left(x-y\right)\left(x+y\right)}=\dfrac{4y\left(x+y\right)}{2\left(x-y\right)\left(x+y\right)}\)
\(=\dfrac{2y}{x-y}\)
c.
\(\dfrac{x+5}{2x-4}.\dfrac{4-2x}{x+2}=\dfrac{x+5}{2x-4}.\dfrac{-\left(2x-4\right)}{x+2}=-\dfrac{x+5}{x+2}\)
d.
\(\dfrac{8}{x^2+2x-3}+\dfrac{2}{x+3}+\dfrac{1}{x-1}=\dfrac{8}{\left(x-1\right)\left(x+3\right)}+\dfrac{2\left(x-1\right)}{\left(x-1\right)\left(x+3\right)}+\dfrac{x+3}{\left(x-1\right)\left(x+3\right)}\)
\(=\dfrac{8+2\left(x-1\right)+x+3}{\left(x-1\right)\left(x+3\right)}=\dfrac{3x+9}{\left(x-1\right)\left(x+3\right)}\)
\(=\dfrac{3\left(x+3\right)}{\left(x-1\right)\left(x+3\right)}=\dfrac{3}{x-1}\)
Đúng 2
Bình luận (0)
Thực hiện các phép tính sau:
a) \({x^2}y\left( {5xy - 2{x^2}y - {y^2}} \right)\)
b) \(\left( {x - 2y} \right)\left( {2{x^3} + 4xy} \right)\)
a) \(x^2y\left(5xy-2x^2y-y^2\right)\)
\(=5x^3y^2-2x^4y^2-x^2y^3\)
b) \(\left(x-2y\right)\left(2x^3+4xy\right)\)
\(=2x^4+4x^2y-4x^3y-8xy^2\)
Đúng 1
Bình luận (0)
thực hiện các phép tính sau:
1. -3ab.(a^2-3b)
2,(x^2-2xy+y^2).(x-2y)
\(-3ab.\left(a^2-3b\right)\)
\(=-3a^3b+9ab^2\)
\(\left(x^2-2xy+y^2\right)\left(x-2y\right)\)
\(=x^3-2x^2y+xy^2-2x^2y+4xy^2-2y^3\)
\(=x^3-4x^2y+5xy^2-2y^3\)
Đúng 0
Bình luận (0)
a) \(-3ab.\left(a^2-3b\right)=-3ab.a^2+3ab.3b=-3a^3b+9ab^2\)
b) \(\left(x^2-2xy+y^2\right).\left(x-2y\right)=\left(x-2y\right).x^2-\left(x-2y\right).2xy+\left(x-2y\right).y^2\)
\(=xx^2-2yx^2-2xyx+2xy2y+xy^2-2yy^2\)
\(=x^3-\left(2yx^2+2yx^2\right)+\left(4xy^2+xy^2\right)-2y^3\)
\(=x^3-4yx^2+5xy^2-2y^3\)
mk chỉ có thể thu gọn đc thôi, mk ko tính đc đâu!
Đúng 0
Bình luận (0)
























