Giải bất phương trình sau và biểu diễn nghiệm trên trục số 3 x - 1 4 ≥ 2

Những câu hỏi liên quan
giải bất phương trình sau và biểu diễn tập nghiệm trên trục số:
\(\dfrac{x-2}{2}+1\)≤\(\dfrac{x-1}{3}\)
\(\dfrac{x-2}{2}+1\le\dfrac{x-1}{3}\)
\(\Leftrightarrow\dfrac{3\left(x-2\right)}{6}+\dfrac{1.6}{6}\le\dfrac{2\left(x-1\right)}{6}\)
`<=> 3x - 6 + 6 <= 2x-2`
`<=> 3x <= 2x-2`
`<=> 3x -2x <= -2`
`<=> x <= -2`
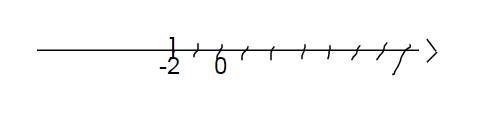
Đúng 2
Bình luận (2)
\(\dfrac{x-2}{2}\)+1≤\(\dfrac{x-1}{3}\)
<=>\(\dfrac{3x-6}{6}\)+\(\dfrac{6}{6}\)≤\(\dfrac{2x-1}{6}\)
<=>3x-6+6≤2x-1
<=>x<-1
Đúng 0
Bình luận (0)
Giải bất phương trình và biểu diễn tập nghiệm trên trục số
(x-2)(2x-3)+3(x+4)<2(x+1)2-4x
=>2x^2-3x-4x+6+3x+8<2x^2+4x+2-4x
=>2x^2-4x+14<2x^2+2
=>-4x<-12
=>x>3
Đúng 0
Bình luận (0)
\(\dfrac{1-2x}{2}-\dfrac{x+1}{3}\le2\) . Giải bất phương trình sau và biểu diễn tập nghiệm trên trục số
\(\Leftrightarrow3\left(1-2x\right)-2\left(x+1\right)< =6\)
=>3-6x-2x-2<=6
=>-8x+1<=6
=>-8x<=5
hay x>=5/8
Đúng 0
Bình luận (1)
Giải bất phương trình sau và biểu diễn nghiệm trên trục số
x-2/6 - x-1/3 < x/2
`(x-2)/6 -(x-1)/3 < x/2`
`<=> (x-2)/6 -(2(x-1))/6 < (3x)/6`
`<=> x-2 - (2x-2) <3x`
`<=> x-2-2x+2<3x`
`<=> -x <3x`
`<=> -x-3x<0`
`<=> -4x<0`
`<=> x>0`
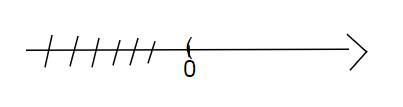
Đúng 2
Bình luận (1)
\(\dfrac{x-2}{6}\)-\(\dfrac{x-1}{3}\)<\(\dfrac{x}{2}\)
\(\dfrac{x-2}{6}\)-\(\dfrac{2\left(x-1\right)}{6}\)<\(\dfrac{6x}{6}\)
<=>x-2-2x+2<6x
<=>-7x<0
<=>x>0
vậy tập nghiệm của bất phương trình là
\(\left\{x|x>0\right\}\)
Đúng 0
Bình luận (0)
\(\dfrac{x-2}{6}-\dfrac{x-1}{3}< \dfrac{x}{2}\\ \Leftrightarrow\dfrac{x-2}{6}-\dfrac{\left(x-1\right)2}{3.2}< \dfrac{x.3}{2.3}\\ \Leftrightarrow x-2-2x+2< 3x\\ \Leftrightarrow x-2x-3x< -2\\ \Leftrightarrow-4x< -2\\ \Leftrightarrow x>\dfrac{1}{2}\)
Vậy bất phương trình có tập nghiệm là:
\(S=\left\{x|x>\dfrac{1}{2}\right\}\)
Biểu diễn:
Đúng 0
Bình luận (1)
Giải các bất phương trình sau và biểu diễn tập nghiệm trên trục số a)(4x-1)(x²+12)(-x+4)>0
=>(4x-1)(-x+4)>0
=>(4x-1)(x-4)<0
=>1/4<x<4
Đúng 0
Bình luận (0)
giải bất phương trình sau và biểu diễn tập nghiệm trên trục số x + 3 > 6
\(x+3>6\)
\(\Leftrightarrow x>6-3\)
\(\Leftrightarrow x>3\)
Biểu diễn trên trục số:
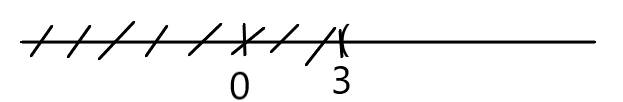
Đúng 0
Bình luận (0)
Giải bất phương trình và biểu diễn tập nghiệm trên trục số
\(\dfrac{x+4}{5}\) - \(\dfrac{x-2}{3}\) > 2
`(x+4)/5 - (x-2)/3 > 2`
`=> (3x+12 - 5x + 10)/15 > 2`
`=> 24 - 2x > 30`
`=> -2x > 6`
`=> x < -3`.
Đúng 2
Bình luận (14)
\(\dfrac{x+4}{5}\) \(-\) \(\dfrac{x-2}{3}\) \(>\) \(2\)
\(=\) \(\dfrac{3x+12-5x+10}{15}\) \(>\) \(2\)
\(=\) \(24-2x>30\)
\(=\) \(-2x>6\)
\(=\) \(x< -3\)
Đúng 0
Bình luận (1)
Giải các bất phương trình sau và biểu diễn tập nghiệm trên trục số:a)
(
2
x
+
3
)
(
2
x
−
1
)
(
2
x
−
5
)
2
b)
(
x
−
1
)
(
x
+
2
)
(...
Đọc tiếp
Giải các bất phương trình sau và biểu diễn tập nghiệm trên trục số:
a) ( 2 x + 3 ) ( 2 x − 1 ) < ( 2 x − 5 ) 2
b) ( x − 1 ) ( x + 2 ) < ( x − 1 ) 2 + 3
Giải bất phương trình sau và biểu diễn nghiệm trên trục số 3x – (7x + 2) > 5x + 4
\(3x-\left(7x+2\right)>5x+4\\ \Leftrightarrow3x-7x-2-5x-4>0\\ \Leftrightarrow-9x-6>0\\ \Leftrightarrow-9x>6\\ \Leftrightarrow x< -\dfrac{2}{3}\)
Đúng 1
Bình luận (0)
Giải bất phương trình sau và biểu diễn tập nghiệm trên trục số:
\(\dfrac{1-2x}{4}-2< \dfrac{1-5x}{8}\)
\(\dfrac{1-2x}{4}-2< \dfrac{1-5x}{8}\)
\(\Leftrightarrow\dfrac{2\left(1-2x\right)-16}{8}< \dfrac{1-5x}{8}\)
\(\Leftrightarrow2\left(1-2x\right)-16< 1-5x\)
\(\Leftrightarrow2-4x-16< 1-5x\)
\(\Leftrightarrow x< 15\)
Vậy \(S=\left\{x|x< 15\right\}\)
Đúng 3
Bình luận (1)




























