Tìm giá trị nhỏ nhất của biểu thức A=/x-13/+/x-14/+/x-15/+/x-16/+/x-17/-10
giúp mình với!
Tìm giá trị nhỏ nhất của biểu thức:
\(A=\left|x-13\right|+\left|x-14\right|+\left|x-15\right|+\left|x-16\right|+\left|x-17\right|-10\)
giải ik mk tictick cho
\(A=\left(|x-13|+|x-17|\right)+\left(|x-14|+|x-16|\right)+|x-15|-10\)
\(\ge\left(x-13+17-x\right)+\left(x-14+16-x\right)+0-10=4+2-10=-4\)
\(\Rightarrow A_{min}=-4\Leftrightarrow x=15\)
Tìm giá trị nhỏ nhất của biểu thức:
\(A=\left|x-13\right|+\left|x-14\right|+\left|x-15\right|+\left|x-16\right|+\left|x-17\right|-10\)
giải giúp mk vs các bn :
mk đg cần gấp
\(A=\left|x-13\right|+\left|x-14\right|+\left|x-15\right|+\left|x-16\right|+\left|x-17\right|-10\)
\(=\left(\left|x-13\right|+\left|x-16\right|\right)+\left(\left|x-14\right|+\left|x-17\right|\right)-10+\left|x-15\right|\)
\(=\left(\left|x-13\right|+\left|16-x\right|\right)+\left(\left|x-14\right|+\left|17-x\right|\right)-10+\left|x-15\right|\)
\(\Rightarrow A\ge\left|x-13+16-x\right|+\left|x-14+17-x\right|-10+\left|x-15\right|\)
\(=\left|3\right|+\left|3\right|-10+\left|x-15\right|\)\(=3+3-10+\left|x-15\right|=-6+\left|x-15\right|\)
Vì \(\left|x-15\right|\ge0\forall x\)\(\Rightarrow A\ge-6\)
Dấu " = " xảy ra \(\Leftrightarrow\hept{\begin{cases}\left(x-13\right)\left(16-x\right)\ge0\\\left(x-14\right)\left(17-x\right)\ge0\\x-15=0\end{cases}}\Leftrightarrow\hept{\begin{cases}13\le x\le16\\14\le x\le17\\x=15\end{cases}}\Leftrightarrow x=15\)
Vậy \(minA=-6\Leftrightarrow x=15\)
Bài 4. Tìm giá trị nhỏ nhất của các biểu thức:
a) A = 2x2 – 15 ; b) B = 2(x + 1)2 – 17.
Bài 5. Tìm giá trị lớn nhất của các biểu thức:
a) A = 14 – x2; b) B = 25 – (x – 2)2
mik sẽ tick nha
Bài 4:
\(A=2x^2-15\ge-15\\ A_{min}=-15\Leftrightarrow x=0\\ B=2\left(x+1\right)^2-17\ge-17\\ B_{min}=-17\Leftrightarrow x=-1\)
Bài 5:
\(A=-x^2+14\le14\\ A_{max}=14\Leftrightarrow x=0\\ B=25-\left(x-2\right)^2\le25\\ B_{max}=25\Leftrightarrow x=2\)
1,Tính giá trị biểu thức
a, 13 . 17 - 256 : 16 + 14 : 7 - 1
b, 15 . 24 - 14 . 5 . (145 : 5 - 27)
c, 15 . 8 - (17 - 30 + 83) - 144 : 6
d,18 - 4 . (27 - 90 + 73) : 10
2,Tìm x
a, 315 - (135 - x)=215
b, x - 320 : 32 = 25 . 16
c, 3 . x - 2018 : 2 = 23
d, 280 - 9 . x - x = 80
e, 38 . x - 12 . x - x . 16 = 40
Giúp mình với nhé,mình đang cần gấp !!
2,
a) \(315-\left(135-x\right)=215\)
\(\Rightarrow135-x=315-215\)
\(\Rightarrow135-x=100\)
\(\Rightarrow x=135-100\)
\(\Rightarrow x=35\)
b) \(x-320:32=25\cdot16\)
\(\Rightarrow x-10=5^2\cdot4^2\)
\(\Rightarrow x-10=20^2\)
\(\Rightarrow x-10=400\)
\(\Rightarrow x=410\)
c) \(3\cdot x-2018:2=23\)
\(=3\cdot x-1009=23\)
\(\Rightarrow3\cdot x=1032\)
\(\Rightarrow x=1032:3\)
\(\Rightarrow x=344\)
d) \(280-9\cdot x-x=80\)
\(\Rightarrow280-x\cdot\left(9+1\right)=80\)
\(\Rightarrow280-10\cdot x=80\)
\(\Rightarrow10\cdot x=280-80\)
\(\Rightarrow10\cdot x=200\)
\(\Rightarrow x=20\)
e) \(38\cdot x-12\cdot x-x\cdot16=40\)
\(\Rightarrow x\cdot\left(38-12-16\right)=40\)
\(\Rightarrow x\cdot10=40\)
\(\Rightarrow x=40:10\)
\(\Rightarrow x=4\)
Tính giá trị của biểu thức tại x=11\(A=x^{17}-12.x^{16}+12.x^{15}-12.x^{14}+12.x^{13}-12.x^{12}+...-12.x^2+12.x-1\)
x=11 suy ra 12=x+1 thay vào A ta có:
A=x^17- (x+1)x^16 + (x+1)x^15 - (x+1)x^14 + .....- (x+1)x^2+(x+1)x -1
= x^17 - x^17 -x^16 + x^16 + x^15 - x^15 - x^14 +.....- x^3 -x^2 + x^2 +x -1
= x-1= 11-1=10
tìm giá trị nhỏ nhất của biểu thức P =|x-17| + |x-16| +... +|x-2 | + |x-1|
Tìm giá trị nguyên của x để biểu thức sau đạt giá trị nhỏ nhất
A=|x-9|+10
giúp mik na mik đag cần rất là gấp
`|x-9|>=0`
`=>|x-9|+10>=10`
Dấu "=" xảy ra khi `x-9=0<=>x=9(TM\ x in Z)`
x−9|≥0|x-9|≥0
⇒|x−9|+10≥10⇒|x-9|+10≥10
Dấu "=" xảy ra khi x−9=0⇔x=9(TM x∈Z)
Giải:
A=|x-9|+10
Xét thấy: |x-9| ≥ 0 với mọi x
⇒|x-9|+10 ≥ 0+10
A ≥ 10
A nhỏ nhất =10 khi và chỉ khi
|x-9|=0
x-9=0
x=0+9
x=9
Chúc bạn học tốt!
Câu 14. Cho biểu thức P = x2 + xy + y2 – 3(x + y) + 3. Chứng minh rằng giá trị nhỏ nhất của P bằng 0.
Câu 15. Chứng minh rằng không có giá trị nào của x, y, z thỏa mãn đẳng thức sau:
x2 + 4y2 + z2 – 2a + 8y – 6z + 15 = 0
Câu 16. Tìm giá trị lớn nhất của biểu thức:![]()
Câu 17. So sánh các số thực sau (không dùng máy tính):
Câu 18. Hãy viết một số hữu tỉ và một số vô tỉ lớn hơn √2 nhưng nhỏ hơn √3
Câu 19. Giải phương trình: ![]() .
.
Câu 20. Tìm giá trị lớn nhất của biểu thức A = x2y với các điều kiện x, y > 0 và 2x + xy = 4.
Câu 21. Cho ![]() .
.
Hãy so sánh S và ![]() .
.
Câu 22. Chứng minh rằng: Nếu số tự nhiên a không phải là số chính phương thì √a là số vô tỉ.
Câu 23. Cho các số x và y cùng dấu. Chứng minh rằng: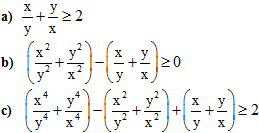
Câu 24. Chứng minh rằng các số sau là số vô tỉ:
Câu 25. Có hai số vô tỉ dương nào mà tổng là số hữu tỉ không?
Câu 26. Cho các số x và y khác 0. Chứng minh rằng:
![]()
Câu 27. Cho các số x, y, z dương. Chứng minh rằng:
![]()
Câu 28. Chứng minh rằng tổng của một số hữu tỉ với một số vô tỉ là một số vô tỉ.
Câu 29. Chứng minh các bất đẳng thức:
a) (a + b)2 ≤ 2(a2 + b2)
b) (a + b + c)2 ≤ 3(a2 + b2 + c2)
c) (a1 + a2 + ….. + an)2 ≤ n(a12 + a22 + ….. + an2).
Câu 30. Cho a3 + b3 = 2. Chứng minh rằng a + b ≤ 2.
Câu 29:
a: \(\left(a+b\right)^2\le2\left(a^2+b^2\right)\)
\(\Leftrightarrow-a^2+2ab-b^2\le0\)
\(\Leftrightarrow-\left(a-b\right)^2\le0\)(luôn đúng)
Câu 14. Cho biểu thức P = x2 + xy + y2 – 3(x + y) + 3. Chứng minh rằng giá trị nhỏ nhất của P bằng 0.
Câu 15. Chứng minh rằng không có giá trị nào của x, y, z thỏa mãn đẳng thức sau:
x2 + 4y2 + z2 – 2a + 8y – 6z + 15 = 0
Câu 16. Tìm giá trị lớn nhất của biểu thức:![]()
Câu 17. So sánh các số thực sau (không dùng máy tính):
Câu 18. Hãy viết một số hữu tỉ và một số vô tỉ lớn hơn √2 nhưng nhỏ hơn √3
Câu 19. Giải phương trình: ![]() .
.
Câu 20. Tìm giá trị lớn nhất của biểu thức A = x2y với các điều kiện x, y > 0 và 2x + xy = 4.
Câu 21. Cho ![]() .
.
Hãy so sánh S và ![]() .
.
Câu 22. Chứng minh rằng: Nếu số tự nhiên a không phải là số chính phương thì √a là số vô tỉ.
Câu 23. Cho các số x và y cùng dấu. Chứng minh rằng: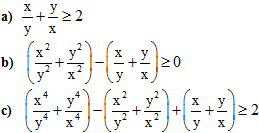
Câu 24. Chứng minh rằng các số sau là số vô tỉ:
Câu 25. Có hai số vô tỉ dương nào mà tổng là số hữu tỉ không?
Câu 26. Cho các số x và y khác 0. Chứng minh rằng:
![]()
Câu 27. Cho các số x, y, z dương. Chứng minh rằng:
![]()
Câu 28. Chứng minh rằng tổng của một số hữu tỉ với một số vô tỉ là một số vô tỉ.
Câu 29. Chứng minh các bất đẳng thức:
a) (a + b)2 ≤ 2(a2 + b2)
b) (a + b + c)2 ≤ 3(a2 + b2 + c2)
c) (a1 + a2 + ….. + an)2 ≤ n(a12 + a22 + ….. + an2).
Câu 30. Cho a3 + b3 = 2. Chứng minh rằng a + b ≤ 2.
\(14,P=x^2+xy+y^2-3x-3y+3\\ P=\left(x^2+xy+\dfrac{1}{4}y^2\right)-3\left(x+\dfrac{1}{2}y\right)+\dfrac{3}{4}y^2-\dfrac{3}{2}y+3\\ P=\left(x+\dfrac{1}{2}y\right)^2-3\left(x+\dfrac{1}{2}y\right)+\dfrac{9}{4}+\dfrac{3}{4}\left(y^2-2y+1\right)\\ P=\left(x+\dfrac{1}{2}y-\dfrac{3}{2}\right)^2+\dfrac{3}{4}\left(y-1\right)^2\ge0\)