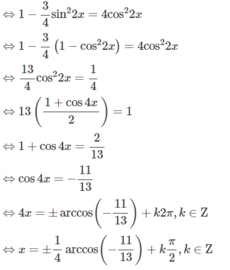giải phương trình
sin8x -cos6x = \(\sqrt{3}\)( sin6x + cos8x )
giải các phương trình sau:
1) \(\left(\sqrt{3}-1\right)sinx-\left(\sqrt{3}+1\right)cosx=1-\sqrt{3}\)
2) \(sin8x-cos6x=\sqrt{3}\left(sin6x+cos8x\right)\)
3) \(sinx+cosx=2\sqrt{2}sinx.cosx\)
4) \(2sin^2x+\sqrt{3}sin2x=3\)
Phương trình sin 8 x - cos 6 x = 3 sin 6 x + cos 8 x có các họ nghiệm là:
A. x = π 4 + k π hoặc x = π 12 + kπ 7
B. x = π 3 + k π hoặc x = π 6 + kπ 2
C. x = π 5 + k π hoặc x = π 7 + kπ 2
D. x = π 8 + k π hoặc x = π 9 + kπ 3
Giải các pt:
a) \(cos3x-sinx=\sqrt{3}\left(cosx-sin3x\right)\)
b) \(2cos^2x-3\sqrt{3}sin2x-4sin^2x=-4\)
c) \(\sqrt{3}\left(cos2x+sin3x\right)=sin2x+cos8x\)
d) \(cos2x-\sqrt{3}sin2x=\sqrt{3}sinx+cosx\)
e) \(sin8x-cos6x=\sqrt{3}\left(sin6x+cos8x\right)\)
a/
\(\Leftrightarrow\frac{\sqrt{3}}{2}sin3x+\frac{1}{2}cos3x=\frac{1}{2}sinx+\frac{\sqrt{3}}{2}cosx\)
\(\Leftrightarrow sin\left(3x+\frac{\pi}{6}\right)=sin\left(x+\frac{\pi}{3}\right)\)
\(\Rightarrow\left[{}\begin{matrix}3x+\frac{\pi}{6}=x+\frac{\pi}{3}+k2\pi\\3x+\frac{\pi}{6}=\pi-x-\frac{\pi}{3}+k2\pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\frac{\pi}{12}+k\pi\\x=\frac{\pi}{8}+\frac{k\pi}{2}\end{matrix}\right.\)
b/
\(\Leftrightarrow2\left(\frac{1+cos2x}{2}\right)-3\sqrt{3}sin2x-4\left(\frac{1-cos2x}{2}\right)=-4\)
\(\Leftrightarrow3cos2x-3\sqrt{3}sin2x=-3\)
\(\Leftrightarrow\frac{\sqrt{3}}{2}sin2x-\frac{1}{2}cos2x=1\)
\(\Leftrightarrow sin\left(2x-\frac{\pi}{6}\right)=1\)
\(\Leftrightarrow2x-\frac{\pi}{6}=\frac{\pi}{2}+k2\pi\)
\(\Leftrightarrow x=\frac{\pi}{3}+k\pi\)
c/
Ủa đề câu này bạn ghi đúng ko? Nhìn kì kì, cos8x hay cos3x bên vế phải vậy?
d/
\(\Leftrightarrow\frac{1}{2}cos2x-\frac{\sqrt{3}}{2}sin2x=\frac{\sqrt{3}}{2}sinx+\frac{1}{2}cosx\)
\(\Leftrightarrow cos\left(2x+\frac{\pi}{3}\right)=cos\left(x-\frac{\pi}{3}\right)\)
\(\Leftrightarrow\left[{}\begin{matrix}2x+\frac{\pi}{3}=x-\frac{\pi}{3}+k2\pi\\2x+\frac{\pi}{3}=\frac{\pi}{3}-x+k2\pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=-\frac{2\pi}{3}+k2\pi\\x=\frac{k2\pi}{3}\end{matrix}\right.\)
e/
\(\Leftrightarrow\frac{1}{2}sin8x-\frac{\sqrt{3}}{2}cos8x=\frac{\sqrt{3}}{2}sin6x+\frac{1}{2}cos6x\)
\(\Leftrightarrow sin\left(8x-\frac{\pi}{3}\right)=sin\left(6x+\frac{\pi}{6}\right)\)
\(\Rightarrow\left[{}\begin{matrix}8x-\frac{\pi}{3}=6x+\frac{\pi}{6}+k2\pi\\8x-\frac{\pi}{3}=\pi-6x-\frac{\pi}{6}+k2\pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\frac{\pi}{4}+k\pi\\x=\frac{\pi}{28}+\frac{k\pi}{7}\end{matrix}\right.\)
Rút gọn biểu thức: 3(sin8x - cos8x) + 4(cos6x - 2sin6x) + 6sin4x
M = 3(sin^8x-cos^8x) + 4(cos^6x-2sin^6x)+6sin^4x
Ta có:
sin^8(x) - cos^8(x) = [sin^4(x) ]² - [cos^4(x)]²
= (sin²x + cos²x)(sin²x -cos²x).[ sin^4(x) + cos^4(x) ]
= (sin²x -cos²x)[ sin^4(x) + cos^4(x) ]
= sin^6(x) - cos^6(x) + sin²x.cos^4(x) -cos²x.sin^4(x)
Lúc đó M viết lại là:
M = 3.[sin^6(x) - cos^6(x) + sin²x.cos^4(x) -cos²x.sin^4(x) ] + 4.[ cos^6(x) -2sin^6(x) ] + 6sin^4(x)
M = -5sin^6(x) + cos^6(x) -3sin^4(x).cos²x + 3sin²x.cos^4(x) +6sin^4(x)
M = -3sin^(6)x - 3cos²x.sin^4(x) + cos^4(x).sin²x + cos^6(x) - 2sin^6(x) + 2sin²x.cos^4(x) + 6sin^4(x)
M = -3sin^4(x).(sin²x + cos²x ) + cos^4(x).[sin²x + cos²x ] -2sin²x.[sin^4(x) - cos^4(x) ] + 6sin^4(x)
M = 3sin^4(x) + cos^4(x) -2sin²x.[sin²x - cos²x]
M = 3sin^4(x) + cos^4(x) -2sin^4(x) + 2sin²x.cos²x
M = sin^4(x) + 2sin²x.cos²x + cos^4(x)
M = [sin²x + cos²x ]² = 1
9. Rút gọn các biểu thức sau
A= cos7x - cos8x - cos9x + cos10x / sin7x - sin8x - sin9x + sin10x
B = sin2x + 2sin3x + sin4x / sin3x +2sin4x + sin5x
C= 1+cosx + cos2x + cos3x / cosx + 2cos^2 . x -1
D = sin4x + sin5x + sin6x / cos4x + cos5x + cos6x
\(D=\frac{sin4x+sin5x+sin6x}{cos4x+cos5x+cos6x}\)
\(=\frac{\left(sin4x+sin6x\right)+sin5x}{\left(cos4x+cos6x\right)+cos5x}\)
\(=\frac{2sin\frac{4x+6x}{2}.cos\frac{4x-6x}{2}+sin5x}{2cos\frac{4x+6x}{2}.cos\frac{4x-6x}{2}+cos5x}\)
\(=\frac{2sin5x.cos\left(-x\right)+sin5x}{2cos5x.cos\left(-x\right)+cos5x}=\frac{sin5x\left(2.cos\left(-x\right)+1\right)}{cos5x\left(2.cos\left(-x\right)+1\right)}=\frac{sin5x}{cos5x}=tan5x\)
Giải phương trình y = s i n 6 x + c o s 6 x = 4 c o s 2 2 x . Nghiệm của phương trình là
A. y = a r c cos - 11 3 + k π 2
B. y = ± 1 4 a r c cos - 11 3 + k π 2
C. y = ± 1 4 a r c cos - 5 13 + k π 2
D. y = ± 1 4 a r c cos 1 3 + k π 2
Giải phương trình y= sin 6 x + cos 6 x = 4 cos 2 2 x Nghiệm của phương trình là
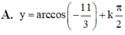
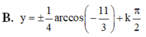
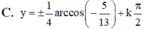

Giải phương trình y = sin 6 x + cos 6 x = 4 cos 2 2 x . Nghiệm của phương trình là
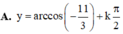
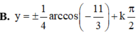
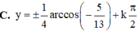

Giải các phương trình sau sin 6 x + cos 6 x = 4 cos 2 2 x
sin 6 x + cos 6 x = 4 cos 2 2 x ⇔ sin 2 x + cos 2 x 3 - 3 sin 2 x . cos 2 x ( sin 2 x + cos 2 x ) = 4 cos 2 2 x