Giúp mk câu này với ạ

Những câu hỏi liên quan
 Mn giúp mk với mấy câu này với ạ . mk cảm mơn ạ
Mn giúp mk với mấy câu này với ạ . mk cảm mơn ạ
1 send - will receive
2 do - will improve
3 find - will give
4 will go - has
5 will go- gets
6 doesn't phone - will leave
7 don't study - won't pass
8 rains - won't have to
9 won't be able - watch
10 can't move - isn't
11 study - will pass
Đúng 6
Bình luận (0)
Giúp mk câu này với mk cần gấp ạ!
Giúp mk câu này với mk cần gấp ạ!
Giúp mk câu này với ạ
1. sociable
2. happy
3. polite
4. famous
5. crowded
6. beautiful
7. quiet
8. selfish
9. traditional
10. patient
Đúng 1
Bình luận (0)
1. sociable
2. happy
3. polite
4. famous
5. crowded
6. beautiful
7. quiet
8. selfish
Đúng 0
Bình luận (0)
Giúp mk 2 câu trắc nhiệm này với mk cần gấp ạ!!!
Giải giúp mk 2 bài này với ạ mk đag cần gấp . dịch giúp mk 4 câu của part 7
P6:
1. big
2. been
3. bought
4. people
P7:
1. T
2. F
3. F
4. F
Đúng 1
Bình luận (1)
Giúp câu này với ạ, mk hứa sẽ tick

Giúp mk mấy câu này với ạ mk cảm ơn ( ko phải thi nha )
23. Please take the form from your teacher and ask her to sign.
24. Can you show me the nearest way to the post office
25. We are going to plant the flowers in the parks and water them in the afternoon.
26. My brother is very good at repairing household appliances.
Đúng 0
Bình luận (0)
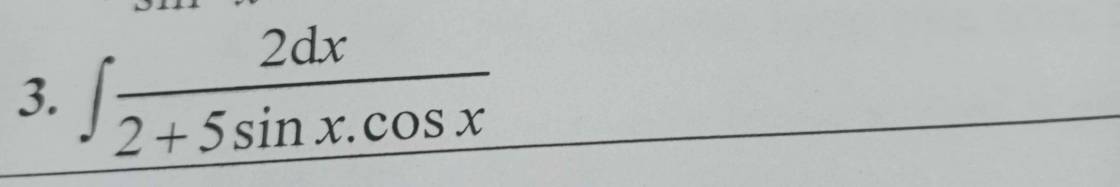 Mọi người giúp mk giải chi tiết câu này với ạ. Mk cảm ơn
Mọi người giúp mk giải chi tiết câu này với ạ. Mk cảm ơn
\(I=\int\dfrac{2}{2+5sinxcosx}dx=\int\dfrac{2sec^2x}{2sec^2x+5tanx}dx\\ =\int\dfrac{2sec^2x}{2tan^2x+5tanx+2}dx\)
We substitute :
\(u=tanx,du=sec^2xdx\\ I=\int\dfrac{2}{2u^2+5u+2}du\\ =\int\dfrac{2}{2\left(u+\dfrac{5}{4}\right)^2-\dfrac{9}{8}}du\\ =\int\dfrac{1}{\left(u+\dfrac{5}{4}\right)^2-\dfrac{9}{16}}du\\ \)
Then,
\(t=u+\dfrac{5}{4}\\I=\int\dfrac{1}{t^2-\dfrac{9}{16}}dt\\ =\int\dfrac{\dfrac{2}{3}}{t-\dfrac{3}{4}}-\dfrac{\dfrac{2}{3}}{t+\dfrac{3}{4}}dt\)
Finally,
\(I=\dfrac{2}{3}ln\left(\left|\dfrac{t-\dfrac{3}{4}}{t+\dfrac{3}{4}}\right|\right)+C=\dfrac{2}{3}ln\left(\left|\dfrac{tanx+\dfrac{1}{2}}{tanx+2}\right|\right)+C\)
Đúng 1
Bình luận (0)
























