\(A=sin42^0-cos48^0=cos\left(90^0-42^0\right)-cos48^0=cos48^0-cos48^0=0\)

\(B=cot56^0-tan34^0=tan\left(90^0-56^0\right)-tan34^0=tan34^0-tan34^0=0\)

\(C=sin30^0-cot50^0-cos60^0+tan40^0\)

\(=cos\left(90^0-30^0\right)-tan\left(90^0-50^0\right)-cos60^0+tan40^0\)

\(=cos60^0-tan40^0-cos60^0+tan40^0=0\)

\(A=\sin42^0-\cos48^0=\sin42^0-\sin42^0=0\)

\(B=\cot56^0-\tan34^0=\tan34^0-\tan34^0=0\)

\(sin60^0=cos\left(90^0-60^0\right)=cos30^0\)

\(cos75^0=sin\left(90^0-75^0\right)=sin15^0\)

\(cot82^03'=tan\left(90^0-82^03'\right)=tan\left(7^057'\right)\)

\(tan80^0=cot\left(90^0-80^0\right)=cot10^0\)

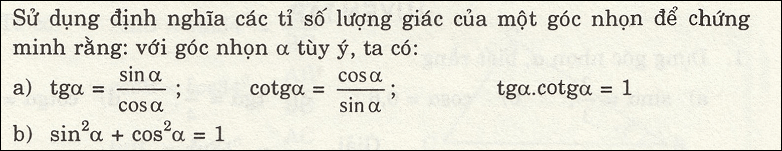
Bài 1:

\(\cos60^0=\sin30^0;\sin67^0=\cos23^0;\tan80^0=\cot10^0;\cot20^0=\cot20^0\)

Bài 2:

Xét tam giác ABC vuông tại A

\(a,\dfrac{\sin\alpha}{\cos\alpha}=\dfrac{AC}{BC}:\dfrac{AB}{BC}=\dfrac{AC}{AB}=\tan\alpha\\ \cot\alpha=\dfrac{1}{\tan\alpha}=\dfrac{1}{\dfrac{\sin\alpha}{\cos\alpha}}=\dfrac{\cos\alpha}{\sin\alpha}\\ \tan\alpha\cdot\cot\alpha=\dfrac{AC}{AB}\cdot\dfrac{AB}{AC}=1\\ b,\sin^2\alpha+\cos^2\alpha=\dfrac{AC^2}{BC^2}+\dfrac{AB^2}{BC^2}=\dfrac{AB^2+AC^2}{BC^2}=\dfrac{BC^2}{BC^2}=1\left(định.lí.pytago\right)\)

sin75=cos15

cos53=sin37

tan71=cot19

cot47=tan43

sin57 độ 25'=cos 32 độ 35'

tan 68 độ35'=cot 21 độ 25'

cos 87 độ 12 p=sin 2 độ 48'

a) \(A=2sin30^o+3cos45^o-sin60^0\)

\(\Leftrightarrow A=2.\dfrac{1}{2}+3.\dfrac{\sqrt[]{2}}{2}-\dfrac{\sqrt[]{3}}{2}\)

\(\Leftrightarrow A=1+\dfrac{3\sqrt[]{2}}{2}-\dfrac{\sqrt[]{3}}{2}\)

\(\Leftrightarrow A=1+\dfrac{\sqrt[]{3}\left(\sqrt[]{6}-1\right)}{2}\)

b) \(B=3cos30^o+3sin45^o-cos45^o\)

\(\Leftrightarrow B=3\dfrac{\sqrt[]{3}}{2}+3\dfrac{\sqrt[]{2}}{2}-\dfrac{\sqrt[]{2}}{2}\)

\(\Leftrightarrow B=\dfrac{3\sqrt[]{3}}{2}+\dfrac{2\sqrt[]{2}}{2}\)

\(\Leftrightarrow B=\dfrac{3\sqrt[]{3}}{2}+\sqrt[]{2}\)

đáp án

A=Sin 42^o - cos 48^o =cos(90^o - 42^o) - cos 48^o= cos48^o - cos48^o=0

hok tốt

B=cos56^o-tan34^o=tan(90^o - 56^o) - tan34^o=tan34^o - tan34^o=0

a: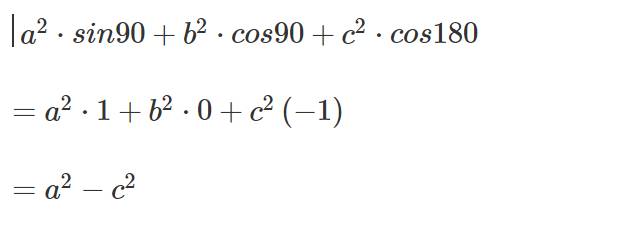 

b: \(B=3-sin^290^0+2\cdot cos^260^0-3\cdot tan^245^0\)

\(=3-1+2\cdot\left(\dfrac{1}{2}\right)^2-3\cdot1^2\)

\(=2-3+2\cdot\dfrac{1}{4}=-1+\dfrac{1}{2}=-\dfrac{1}{2}\)

c: \(C=sin^245^0-2\cdot sin^250^0+3\cdot cos^245^0-2\cdot sin^240^0+4\cdot tan55\cdot tan35\)

\(=\left(\dfrac{\sqrt{2}}{2}\right)^2+3\cdot\left(\dfrac{\sqrt{2}}{2}\right)^2-2\cdot\left(sin^250^0+sin^240^0\right)+4\)

\(=\dfrac{1}{2}+3\cdot\dfrac{1}{2}-2+4\)

\(=2-2+4=4\)

Thứ tự tăng dần :

1) cos 62 độ 25 phút; sin 35 độ; cos 47 độ; sin 53 độ 30 phút; sin 74 độ.

2) tan 11 độ; cot 63 độ = tan 27 độ; cot 57 độ 30 phút; tan 55 độ