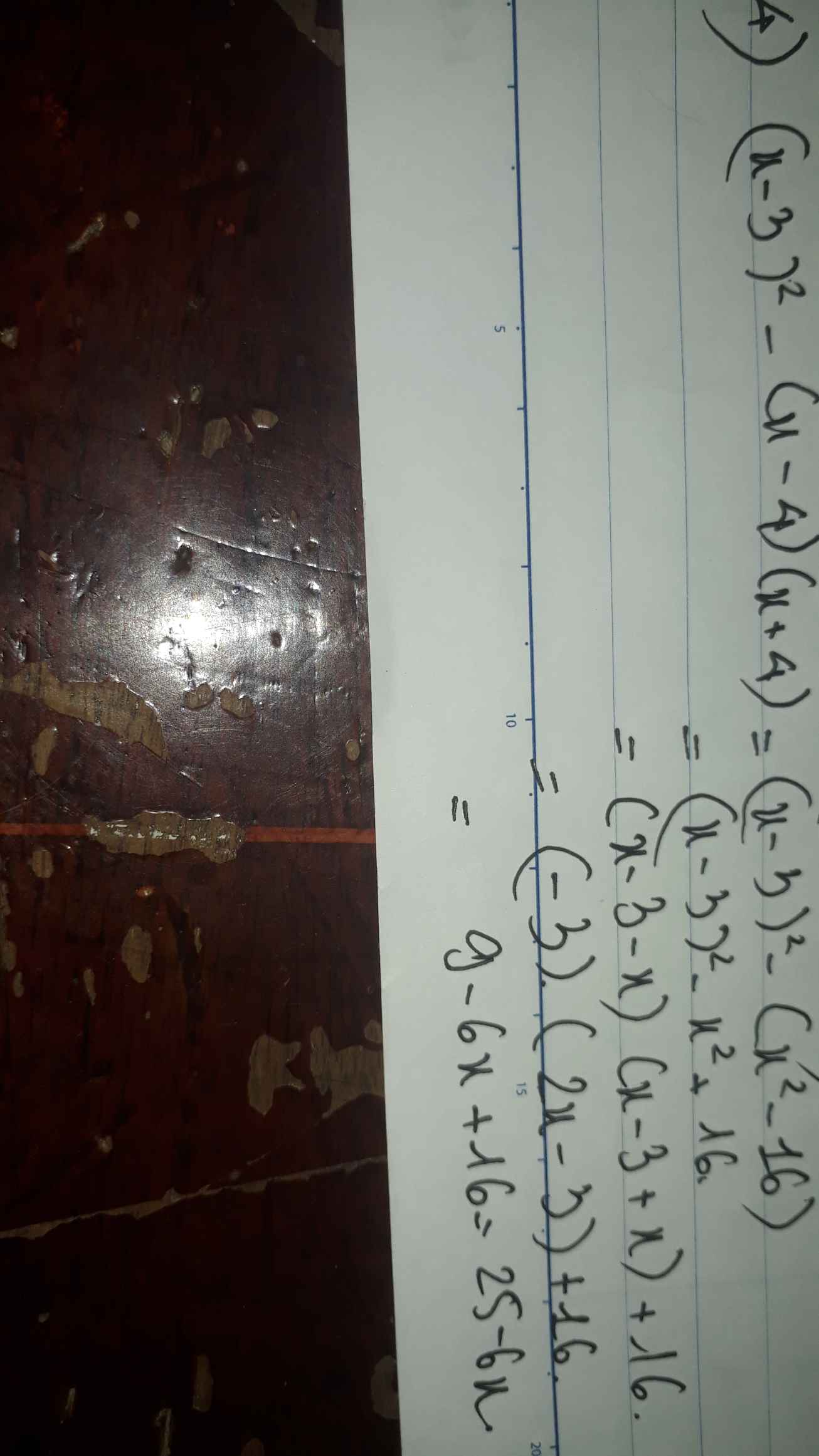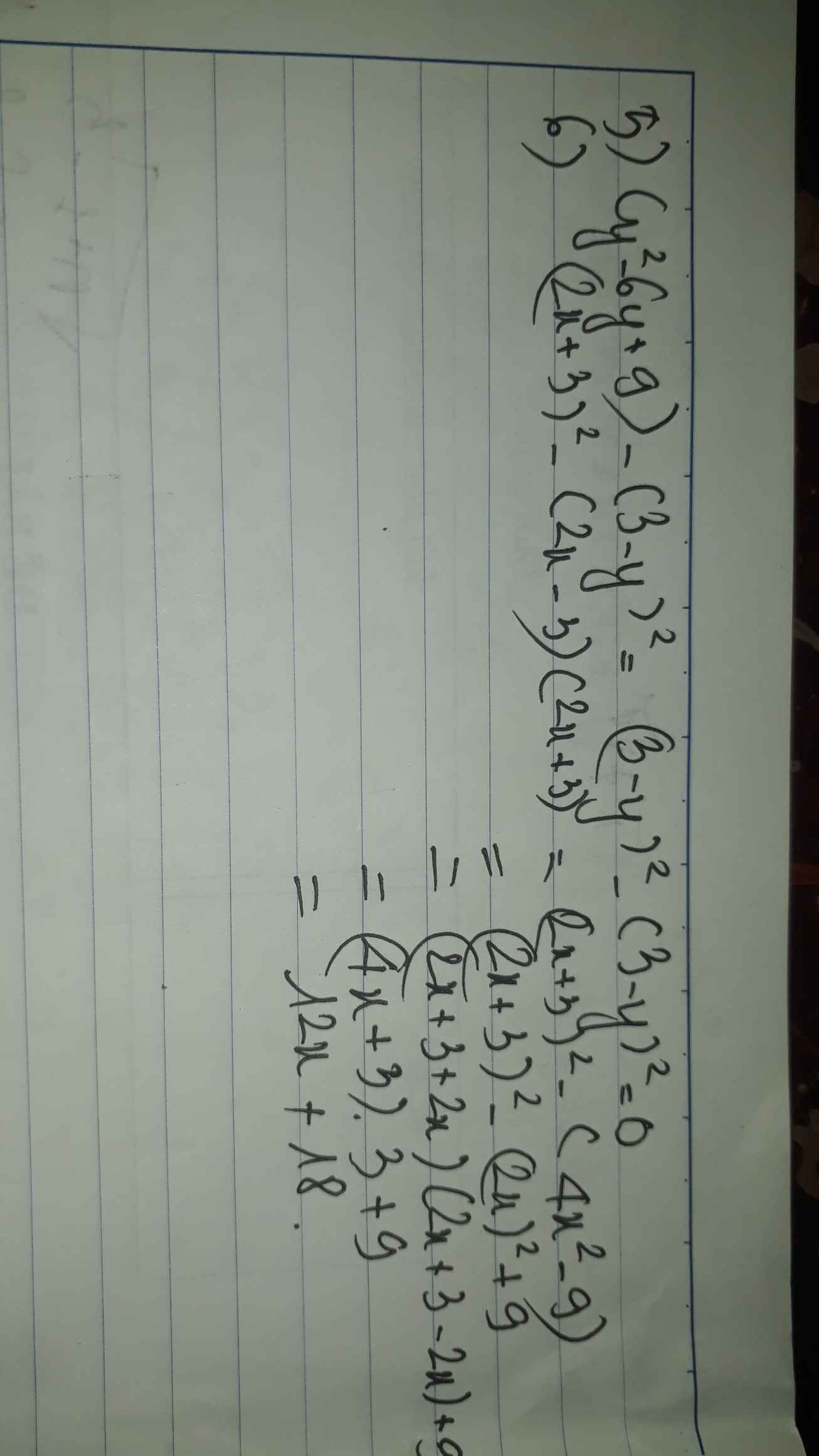1) Ta có: \(\left(x+2\right)^2+\left(x-3\right)^2\)

\(=x^2+4x+4+x^2-6x+9\)

\(=2x^2-2x+13\)

2) Ta có: \(\left(4-x\right)^2-\left(x-3\right)^2\)

\(=\left(4-x-x+3\right)\left(4-x+x-3\right)\)

\(=-2x+7\)

3) Ta có: \(\left(x-5\right)\left(x+5\right)-\left(x+5\right)^2\)

\(=x^2-25-x^2-10x-25\)

=-10x-50

4) Ta có: \(\left(x-3\right)^2-\left(x-4\right)\left(x+4\right)\)

\(=x^2-6x+9-x^2+16\)

=-6x+25

5) Ta có: \(\left(y^2-6y+9\right)-\left(y-3\right)^2\)

\(=y^2-6y+9-y^2+6y-9\)

=0

6) Ta có: \(\left(2x+3\right)^2-\left(2x-3\right)\left(2x+3\right)\)

\(=4x^2+12x+9-4x^2+9\)

=12x+18

1) Ta có: \(\left(x+2\right)^2+\left(x-3\right)^2\)

\(=x^2+4x+4+x^2-6x+9\)

\(=2x^2-2x+13\)

2) Ta có: \(\left(4-x\right)^2-\left(x-3\right)^2\)

\(=\left(4-x-x+3\right)\left(4-x+x-3\right)\)

\(=\left(-2x+7\right)\cdot1\)

\(=-2x+7\)

3) Ta có: \(\left(x-5\right)\left(x+5\right)-\left(x+5\right)^2\)

\(=x^2-25-x^2-10x-25\)

\(=-10x-50\)

a: 2x-3/2+3/4=-4

=>2x-3/4=-4

=>2x=-13/4

hay x=-13/8

b: \(\left(-\dfrac{2}{3}x-\dfrac{3}{5}\right)\cdot\left(\dfrac{-3}{2}-\dfrac{10}{3}\right)=\dfrac{2}{5}\)

\(\Leftrightarrow-\dfrac{2}{3}x-\dfrac{3}{5}=\dfrac{2}{5}:\dfrac{-29}{6}=\dfrac{-2}{5}\cdot\dfrac{6}{29}=\dfrac{-12}{145}\)

=>2/3x+3/5=12/145

=>2/3x=-15/29

hay x=-45/58

c: \(\dfrac{x}{2}-\left(\dfrac{3}{5}x-\dfrac{13}{5}\right)=-\left(\dfrac{7}{10}x+\dfrac{7}{5}\right)\)

=>1/2x-3/5x+13/5=-7/10x-7/5

=>-1/10x+7/10x=-7/5-13/5

=>3/5x=-2

hay x=-2:3/5=-10/3

1)  (2x + 1)(3x – 2) = (5x – 8)(2x + 1)

⇔ (2x + 1)(3x – 2) – (5x – 8)(2x + 1) = 0

⇔ (2x + 1).[(3x – 2) – (5x – 8)] = 0

⇔ (2x + 1).(3x – 2 – 5x + 8) = 0

⇔ (2x + 1)(6 – 2x) = 0

⇔\(\left[{}\begin{matrix}2x+1=0\\6-2x=0\end{matrix}\right.\) ⇔ \(\left[{}\begin{matrix}x=\dfrac{-1}{2}\\x=3\end{matrix}\right.\)

Vậy.....

2)  4x² -1 = (2x + 1)(3x - 5)

⇔ (2x-1)(2x+1)-(2x+1)(3x-5)=0

⇔ (2x+1)(2x-1-3x+5)=0

⇔ (2x+1)(4-x)=0

⇔ \(\left[{}\begin{matrix}2x+1=0\\4-x=0\end{matrix}\right.\) ⇔ \(\left[{}\begin{matrix}x=\dfrac{-1}{2}\\x=4\end{matrix}\right.\)

Vậy...

3)  

(x + 1)² = 4(x² – 2x + 1)

⇔ (x + 1)² - 4(x² – 2x + 1) = 0

⇔ x² + 2x +1- 4x² + 8x – 4 = 0

⇔ - 3x² + 10x – 3 = 0

⇔ (- 3x² + 9x) + (x – 3) = 0

⇔ -3x (x – 3)+ ( x- 3) = 0

⇔ ( x- 3) ( - 3x + 1) = 0

⇔\(\left[{}\begin{matrix}x-3=0\\-3x+1=0\end{matrix}\right.\) ⇔\(\left[{}\begin{matrix}x=3\\x=\dfrac{1}{3}\end{matrix}\right.\)

Vậy......

4) 2x³+5x²-3x=0

⇒2x³-x²+6x²-3x=0

⇒(2x³-x²)+(6x²-3x)=0

⇒x²(2x-1)+3x(2x-1)=0

⇒(x²+3x)(2x-1)=0

⇒ hoặc x²+3x=0⇒x(x+3)=0⇒hoặc x=0 hoặc x=-3

hoặc 2x-1=0⇒x=0,5

Vậy ...

5)2x=3x-2

⇒2x-3x=-2

⇒-x=-2

⇒x=2

6) x+15=3x-1

⇒x-3x=-1-15

⇒-2x=-16

⇒x=8

7)2-x=0,5x-4

⇒-x-0,5x=-4-2

⇒-1,5x=-6

⇒x=4

a)\(5\left(2x-1\right)-4\left(8-3x\right)=7\)

\(\Leftrightarrow10x-5+12x-32=7\)

\(\Leftrightarrow22x-37=7\)

\(\Leftrightarrow22x=44\Rightarrow x=2\)

b)\(5x\left(x-5\right)-x\left(2x+3\right)=26\)

\(\Leftrightarrow5x^2-25x-2x^2-3x=26\)

\(\Leftrightarrow3x^2-28x-26=0\)

\(\Leftrightarrow3\left(x-\dfrac{14}{3}\right)^2-\dfrac{274}{3}=0\)

\(\Rightarrow x=\dfrac{14}{3}\pm\dfrac{\sqrt{274}}{3}\)

ủng hộ mình lên 330 điểm nha các bạn

\(\Leftrightarrow\dfrac{233}{286}\left(2x-x^2\right)=\dfrac{-233}{572}\\ \Leftrightarrow x\left(2-x\right)=\dfrac{-1}{2}\\ \Leftrightarrow\left[{}\begin{matrix}x=\dfrac{-1}{2}\\2-x=\dfrac{-1}{2}\Leftrightarrow x=\dfrac{5}{2}\end{matrix}\right.\)